Soft landing performance optimization for novel lander based on multiple working conditions
-
摘要:
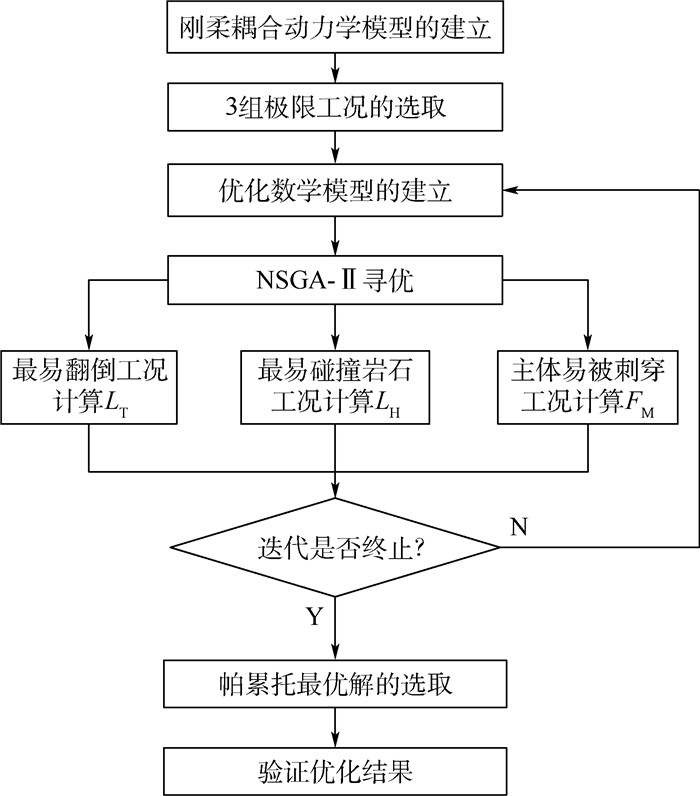
以新型腿式着陆器为研究对象,建立其刚柔耦合动力学分析模型,实现着陆器软着陆过程的仿真。通过仿真计算,确定着陆器最易翻倒、底面最易与星球表面岩石碰撞、主体承受最大碰撞力的3组恶劣着陆工况。分析着陆器缓冲机构构型选取设计变量,基于仿真得到的3组恶劣工况,应用第二代非劣排序遗传算法(NSGA-Ⅱ)实现着陆器软着陆性能的优化,优化目标为增强着陆器抗翻倒能力、降低着陆器底面与星球表面岩石碰撞的可能性、降低着陆器主体最大受力值。将优化所得参数代入模型重新进行仿真,着陆器不再发生翻倒,着陆平台底面与星球表面最小距离提高4.2%,主体最大受力值降低12.1%。
Abstract:A rigid-flexible coupled dynamics model was established for simulating and analyzing the soft landing process of the novel leg type lander. Three groups of bad landing conditions, in which the lander most easily overturns, bottom surface of the lander most easily collides with rocks on the surface of the planet, and body of the lander bears the greatest impact force, were found by iterating over landing parameters. According to the configuration of buffering mechanism, design variables of optimization were selected. Based on the three groups of bad landing conditions and non-dominated sorting genetic algorithm Ⅱ (NSGA-Ⅱ), a multi-objective optimization method was applied to enhance the ability of the lander to resist overturning, reduce the possibility that the bottom surface of lander collides with rocks on the surface of the planet, and reduce the impact on the body of lander. In the simulation using optimized parameters, the model does not overturn any longer. The minimum distance between bottom surface of the landing platform of the lander and surface of the planet increases by 4.2%, and the impact on the body of lander reduces by 12.1%.
-
表 1 着陆工况各参数取值
Table 1. Value of parameters of landing condition
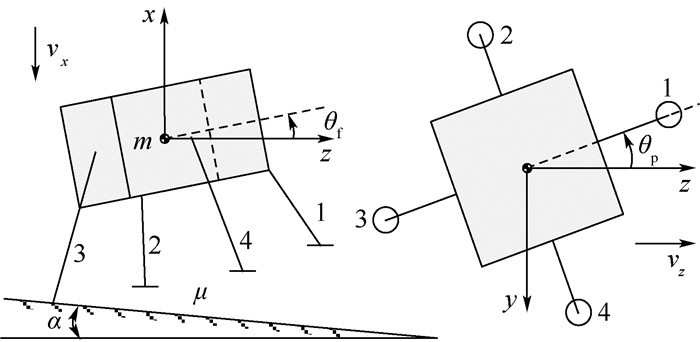
参数 取值 μ 0.3,0.8 α/(°) 7 n 0,1,2,3 vx/(m·s-1) 3.5 vz/(m·s-1) -1,+1 θp/(°) 0~45 θf/(°) -3,+3 m/kg 700,800 表 2 极限工况计算结果
Table 2. Calculation results of the worst landing condition
极限指标值 μ n vz/(m·s-1) θp/(°) θf/(°) m/kg LT=1.11 mm 0.8 2 1 9 3 700 LH=734.23 mm 0.3 3 -1 0 -3 800 FM=27.22 kN 0.8 3 -1 0 3 800 表 3 设计变量取值范围
Table 3. Value range of design variables
设计变量 取值范围 kx 1~2 kz 1~1.5 dz/m 1.37~1.45 参数 种群规模 进化代数 交叉指数 变异指数 交叉概率 取值 12 20 10 20 0.9 表 5 帕累托最优解集
Table 5. Pareto optimal solution aggregate
编号 kz kx dz/m LH/mm FM/kN LT/mm 1 1.46 1.07 1.45 757.91 24.07 413.92 2 1.46 1.23 1.45 757.97 26.31 416.59 3 1.49 1.13 1.44 761.38 25.57 378.00 4 1.49 1.03 1.43 763.07 23.36 349.08 5 1.49 1.05 1.41 765.81 23.95 258.71 6 1.49 1.22 1.42 764.49 27.31 306.33 7 1.49 1.08 1.43 762.92 24.07 352.49 8 1.49 1.04 1.43 762.57 23.50 360.83 9 1.49 1.03 1.42 764.15 23.46 311.13 10 1.48 1.19 1.43 761.46 25.72 365.11 表 6 优化前后性能对比
Table 6. Comparison of performance before and after optimization
对比项 kx kz dz/m LT/mm LH/mm FM/kN 优化前 1 1 1.36 1.11 734.23 27.22 优化后 1.05 1.49 1.41 234.36 765.81 23.95 -
[1] 杨建中, 满剑锋, 曾福明, 等."嫦娥三号"着陆缓冲机构的研究成果及其应用[J].航天返回与遥感, 2014, 35(6):20-27. http://www.cnki.com.cn/Article/CJFDTOTAL-HFYG201406004.htmYANG J Z, MAN J F, ZENG F M, et al.Achievements and applications of landing gear for Chang'e-3 lander[J].Spacecraft Recovery & Remote Sensing, 2014, 35(6):20-27(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HFYG201406004.htm [2] 李萌. 腿式着陆缓冲装置吸能特性及软着陆过程动力学仿真研究[D]. 哈尔滨: 哈尔滨工业大学, 2013: 1-10.LI M.Research on energy absorbers of legged-type lander and dynamic simulation on its soft landing process[D].Harbin:Harbin Institute of Technology, 2013:1-10(in Chinese). [3] 蒋万松, 黄伟, 沈祖炜, 等.月球探测器软着陆动力学仿真[J].宇航学报, 2011, 32(3):462-469. http://www.cnki.com.cn/Article/CJFDTOTAL-YHXB201002007.htmJIANG W S, HUANG W, SHEN Z W, et al.Soft landing dynamic simulation for lunar explorer[J].Journal of Astronautics, 2011, 32(3):462-469(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-YHXB201002007.htm [4] ADAM D S, MIGUEL S M, TOMMASO P R, et al.Mars science laboratory entry, descent, and landing system development challenges[J].Journal of Spacecraft and Rockets, 2014, 51(4):994-1003. doi: 10.2514/1.A32866 [5] DING J Z, WANG C J.Fast modeling for lunar landing dynamics analysis:AIAA-2016-1191[R].Reston:AIAA, 2016. [6] 刘晓宇. 着陆器软着陆缓冲稳定性仿真分析[D]. 长沙: 湖南大学, 2013: 19-28.LIU X Y.Simulation analysis on soft landing's buffer and stability of lunar exploration[D].Changsha:Hunan University, 2013:19-28(in Chinese). [7] 陈金宝, 聂宏, 万峻麟.深空探测着陆器数字化设计及着陆性能影响因素[J].航空学报, 2014, 35(2):541-554. http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201402026.htmCHEN J B, NIE H, WAN J L.Digital design and landing performance influence factors of deep space lander[J].Acta Aeronautica et Astronautica Sinica, 2014, 35(2):541-554(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201402026.htm [8] 罗昌杰, 邓宗全, 刘荣强, 等.基于零力矩点理论的腿式着陆器着陆稳定性研究[J].机械工程学报, 2010, 46(9):38-45. http://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201009008.htmLUO C J, DENG Z Q, LIU R Q, et al.Landing stability investigation of legged-type spacecraft lander based on zero moment point theory[J].Journal of Mechanical Engineering, 2010, 46(9):38-45(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-JXXB201009008.htm [9] 罗松柏, 赵永嘉.月球软着陆动力学分析与仿真[J].北京航空航天大学学报, 2012, 38(2):185-190.LUO S B, ZHAO Y J.Dynamic analysis and simulation of soft-landing for lunar lander[J].Journal of Beijing University of Aeronautics and Astronautics, 2012, 38(2):185-190(in Chinese). [10] 逯运通, 宋顺广, 王春洁.基于刚柔耦合模型的月球着陆器动力学分析[J].北京航空航天大学学报, 2010, 36(11):1348-1352.LU Y T, SONG S G, WANG C J.Dynamic analysis for lunar lander based on rigid-flexible coupled model[J].Journal of Beijing University of Aeronautics and Astronautics, 2010, 36(11):1348-1352(in Chinese). [11] 王家俊, 王春洁, 宋顺广.基于响应面法的月球着陆器软着陆性能优化[J].北京航空航天大学学报, 2014, 40(5):707-711.WANG J J, WANG C J, SONG S G.Performance optimization of lunar lander based on response surface methodology[J].Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(5):707-711(in Chinese). [12] BRIAN C S, MELISSA S, PETER I.Telecommunications performance during entry, descent, and landing of the Mars science laboratory[J].Journal of Spacecraft and Rockets, 2014, 51(4):1237-1250. doi: 10.2514/1.A32790 [13] ZUPP G A, DOIRON H H.A mathematical procedure for predicting the touchdown dynamics of a soft-landing vehicle:NASA-TN-D-7045[R].Washington, D.C.:NASA, 1971. [14] MARLER R T, ARORA J S.Survey of multi-objective optimization methods for engineering[J].Structural and Multidisciplinary Optimization, 2004, 26(6):369-395. doi: 10.1007/s00158-003-0368-6 [15] 黄晶晶, 郑龙席, 刘钢旗, 等.双盘转子系统优化算法与试验[J].航空动力学报, 2016, 31(1):65-71. http://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201601010.htmHUANG J J, ZHENG L X, LIU G Q, et al.Optimization algorithm and experiment of two-disk rotor system[J].Journal of Aerospace Power, 2016, 31(1):65-71(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201601010.htm -








 下载:
下载: