Novel second-order sliding mode control based 3D guidance law with impact angle constraints
-
摘要:
针对导弹在三维空间中攻击地面机动目标问题,提出了一种带落角约束的三维有限时间制导律。为提高收敛速度和抑制抖振现象,基于非齐异快速终端滑模面和二阶滑模控制理论设计了含耦合项的非奇异快速终端二阶滑模三维制导律,设计过程中无需对系统模型作线性化处理并且避免了奇异问题的出现。针对目标机动信息和视线角耦合带来的总扰动,设计了非齐次干扰观测器进行估计并补偿。并对制导律的稳定性和有限时间收敛特性进行了严格的数学证明。仿真验证了本文提出制导律的有效性和优越性。
Abstract:To deal with the problem of missile for attacking ground maneuvering target in 3D space, a 3D finite-time guidance law with impact angle constraints is proposed. In order to improve convergence speed and suppress chattering problem, the nonsingular fast terminal second-order sliding mode control based 3D guidance law with coupling terms is designed based on the nonsingular fast terminal sliding surface and the second-order sliding mode control theory. System model linearization is not needed and singular problem is avoided in the design process. A nonhomogeneous disturbance observer is designed to estimate and compensate the total disturbance, which is caused by target maneuvering information and coupling terms of line of sight. And the stability and finite-time convergent characteristics of the proposed guidance law are proved mathematically. The effectiveness and superiority of the proposed guidance law are verified by numerical simulation.
-
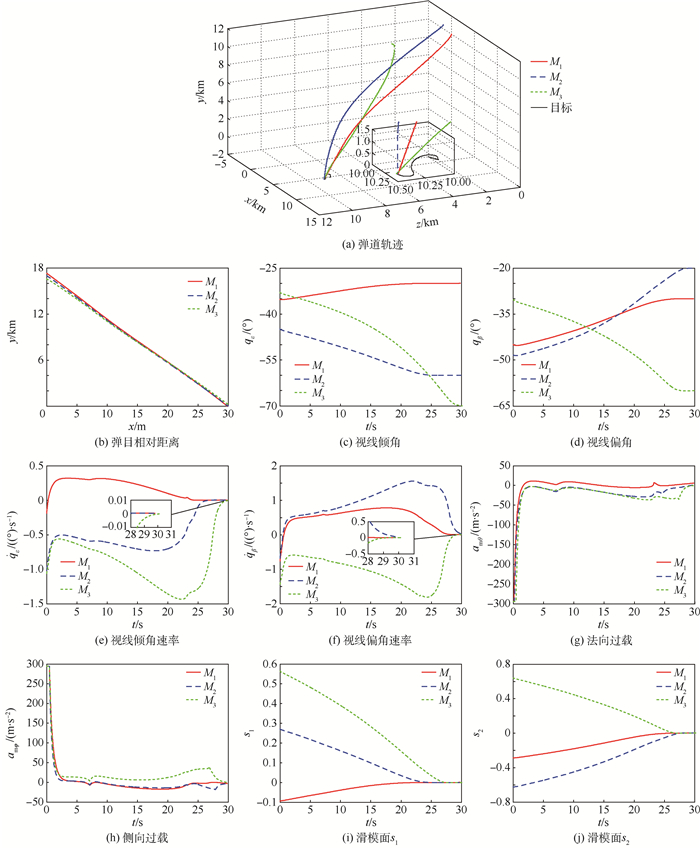
表 1 导弹初始参数和期望落角
Table 1. Initial parameters and expected impact angles of missiles
导弹 初始坐标/km θm0/(°) φm0/(°) qεd/(°) qβd/(°) M1 (0, 10, 0) -25 -20 -30 -30 M2 (2, 12, 1) -10 -30 -60 -20 M3 (-2, 9, 3) 10 10 -70 -60 表 2 3枚导弹攻击目标主要仿真参数
Table 2. Main simulation parameters of 3 missiles attacking targets
导弹 脱靶量/m 视线倾角误差/(°) 视线偏角误差/(°) 飞行时间/s M1 0.206 9 0.011 6 0.025 7 29.768 0 M2 0.077 6 0.010 0 0.025 0 29.979 0 M3 0.296 2 0.029 3 0.015 9 30.362 0 表 3 3种制导律仿真主要参数对比
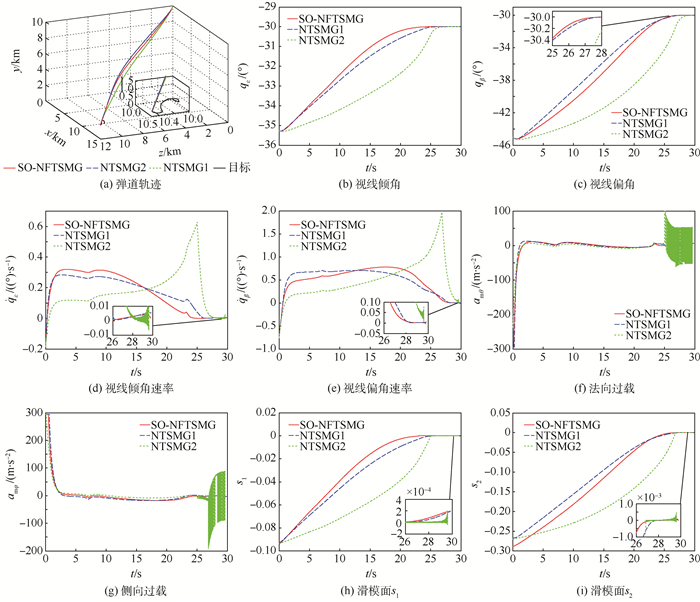
Table 3. Main simulation parameters comparison of 3 guidance laws
制导律 脱靶量/m 视线倾角误差/(°) 视线偏角误差/(°) 飞行时间/s SO-NFTSMG 0.206 9 0.011 6 0.025 7 29.768 0 NTSMG1 0.268 1 0.023 6 0.026 1 29.706 0 NTSMG2 0.242 3 0.011 4 0.027 3 29.923 0 -
[1] 黄诘, 张友安, 刘永新.一种有撞击角和视场角约束的运动目标的偏置比例导引算法[J].宇航学报, 2016, 37(2):195-202. doi: 10.3873/j.issn.1000-1328.2016.02.009HUANG J, ZHANG Y A, LIU Y X.A biased proportional guidance algorithm for moving target with impact angle and field-of-view constraints[J].Journal of Astronautics, 2016, 37(2):195-202(in Chinese). doi: 10.3873/j.issn.1000-1328.2016.02.009 [2] ERER K S, TEKIN R.Impact time and angle control based on constrained optimal solutions[J].Journal of Guidance, Control, and Dynamics, 2016, 39(10):1-7. [3] TAUB I, SHIMA T.Intercept angle missile guidance under time varying acceleration bounds[J].Journal of Guidance, Control, and Dynamics, 2013, 36(3):686-699. doi: 10.2514/1.59139 [4] LEE C H, KIM T H, TAHK M J.Interception angle control guidance using proportional navigation with error feedback[J].Journal of Guidance, Control, and Dynamics, 2013, 36(5):1556-1561. doi: 10.2514/1.58454 [5] WANG H, LIN D, CHENG Z, et al.Optimal guidance of extended trajectory shaping[J].Chinese Journal of Aeronautics, 2014, 27(5):1259-1272. doi: 10.1016/j.cja.2014.03.022 [6] 王晓芳, 王紫扬, 林海.一种同时具有攻击时间和攻击角度约束的协同制导律[J].弹道学报, 2017, 29(4):1-8. doi: 10.3969/j.issn.1004-499X.2017.04.001WANG X F, WANG Z Y, LIN H.A cooperative guidance law with constraints of impact time and impact angle[J].Journal of Ballistics, 2017, 29(4):1-8(in Chinese). doi: 10.3969/j.issn.1004-499X.2017.04.001 [7] 张小件, 刘明雍, 李洋.基于反演滑模和扩张观测器的带角度约束制导律设计[J].系统工程与电子技术, 2017, 39(6):1311-1316.ZHANG X J, LIU M Y, LI Y.Backstepping sliding mode control and extended state observer based guidance law design with angles[J].Systems Engineering and Electronics, 2017, 39(6):1311-1316(in Chinese). [8] WANG X, HONG Y.Finite-time consensus for multi-agent networks with second-order agent dynamics[C]//Proceedings of the IFAC World Congress.Laxenburg: IFCA, 2018: 15185-15190. [9] 熊少锋, 王卫红, 王森.带攻击角度约束的非奇异快速终端滑模制导律[J].控制理论与应用, 2014, 31(3):269-278.XIONG S F, WANG W H, WANG S.Nonsingular fast terminal sliding-mode guidance with intercept angle constraint[J].Control Theory & Applications, 2014, 31(3):269-278(in Chinese). [10] 赵斌, 周军, 卢晓东, 等.考虑终端角度约束的自适应积分滑模制导律[J].控制与决策, 2017, 32(11):1966-1972.ZHAO B, ZHOU J, LU X D, et al.Adaptive integral sliding mode guidance law considering impact angel constraint[J].Control and Decision, 2017, 32(11):1966-1972(in Chinese). [11] 赵曜, 李璞, 刘娟, 等.带碰撞角约束的三维有限时间滑模制导律[J].北京航空航天大学学报, 2018, 44(2):273-279.ZHAO Y, LI P, LIU J, et al.Finite-time sliding mode control based 3D guidance law with impact angle constraints[J].Journal of Beijing University of Aeronautics and Astronautics, 2018, 44(2):273-279(in Chinese). [12] 司玉洁, 宋申民.拦截高超声速飞行器的三维有限时间制导律设计[J].中国惯性技术学报, 2017, 25(3):405-414.SI Y J, SONG S M.Design of three-dimensional finite-time guidance law for intercepting hypersonic vehicle[J].Journal of Chinese Inertial Technology, 2017, 25(3):405-414(in Chinese). [13] ARIE L.Principles of 2-sliding mode design[J].Automatica, 2007, 43(4):576-586. doi: 10.1016/j.automatica.2006.10.008 [14] SUN L H, WANG W H, YI R, et al.A noval guidance law using fast terminal sliding mode control with impact angle constraints[J].ISA Transactions, 2016, 64:12-23. doi: 10.1016/j.isatra.2016.05.004 [15] LI P, PENG X F, MA J J, et al.Non-homogeneous disturbance observer-based second order sliding mode control for a tailless aircraft[C]//Proceedings of Chinese Automation Congress.Piscataway, NJ: IEEE Press, 2013: 120-125. [16] BHAT S P, BERNSTEIN D S.Finite-time stability of continuous autonomous systems[J].SIAM Journal on Control and Optimization, 2000, 38(3):751-766. doi: 10.1137/S0363012997321358 [17] 周慧波.基于有限时间和滑模理论的导引律及多导弹协同制导研究[D].哈尔滨: 哈尔滨工业大学, 2015: 41-44.ZHOU H B.Study on guidance law and cooperative guidance for multi-missiles based on finite-time and sliding mode theory[D].Harbin: Harbin Institute of Technology, 2015: 41-44(in Chinese). 期刊类型引用(1)
1. 尚涛,孙海正,刘建伟. 基于斐波那契编码的测量设备无关量子密钥分发方案. 航空科学技术. 2021(03): 71-78 .  百度学术
百度学术其他类型引用(2)
-







 下载:
下载:
 百度学术
百度学术

