-
摘要:
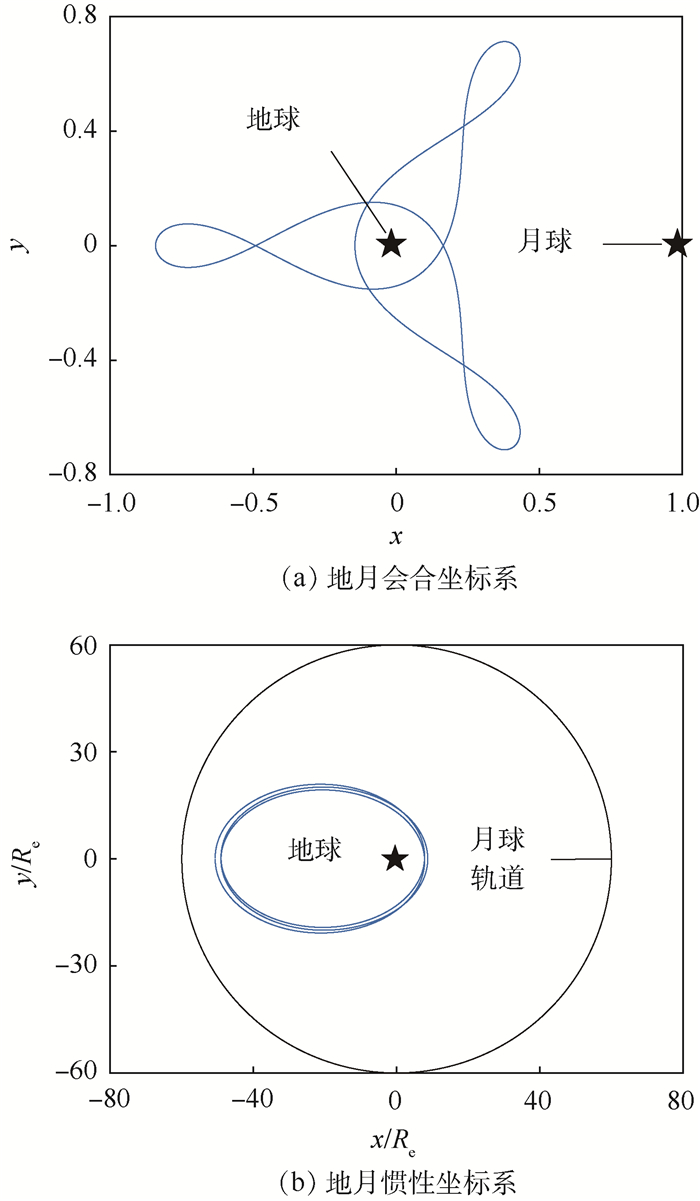
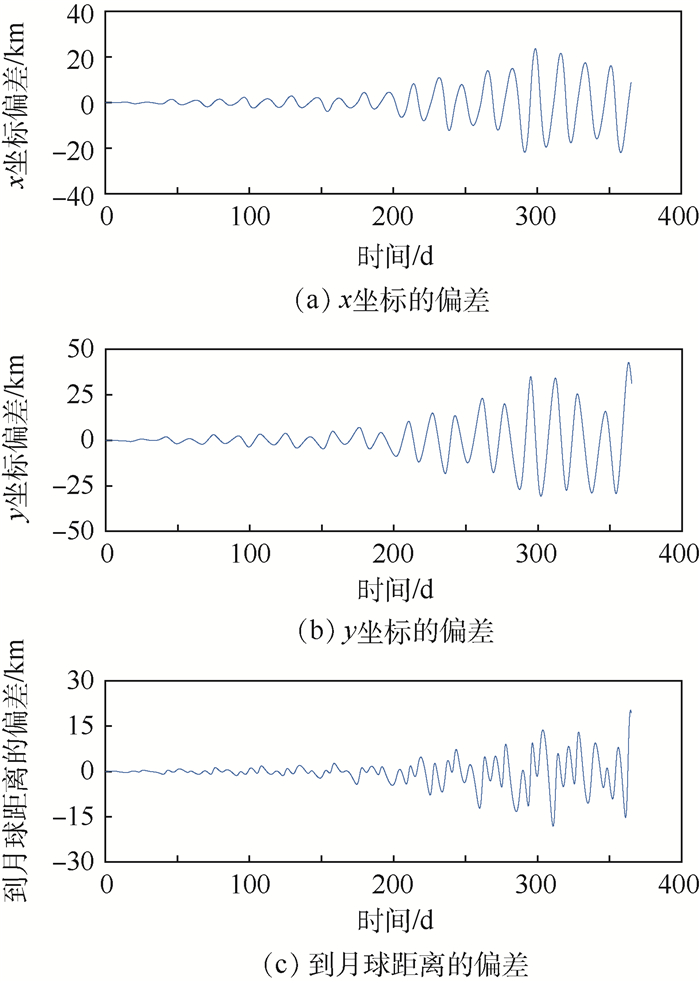
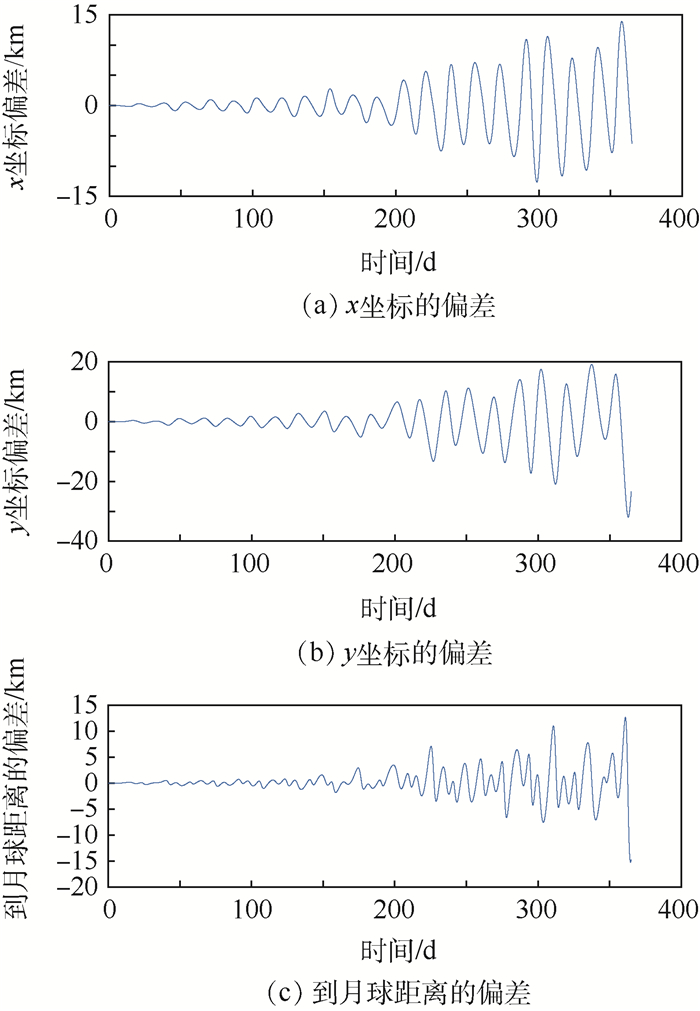
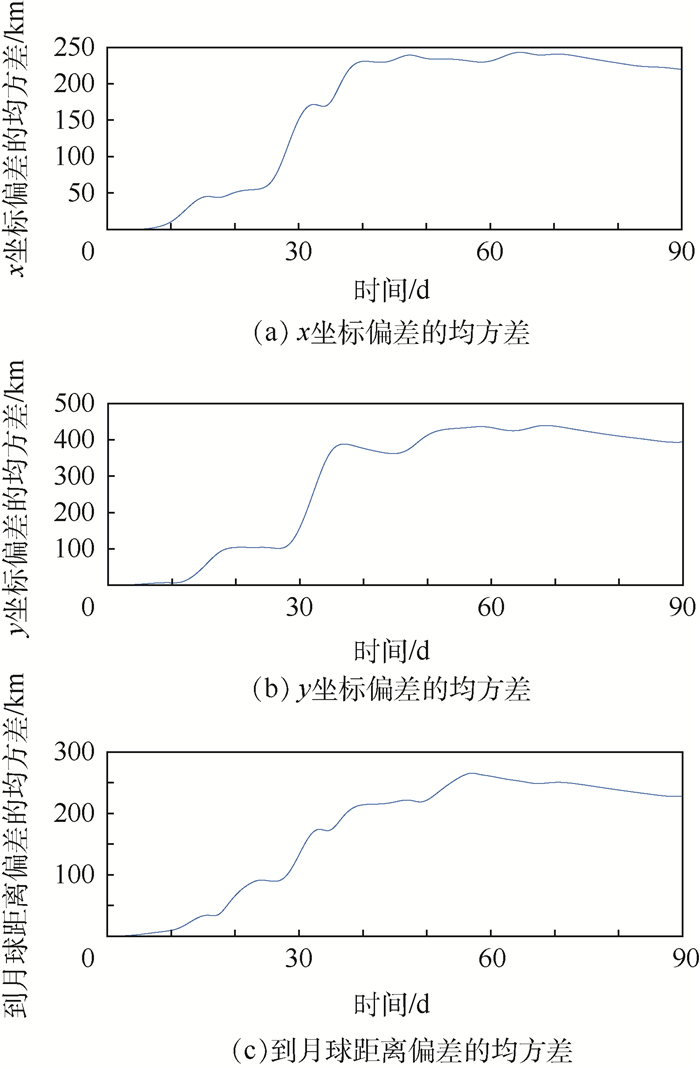
针对远距逆行轨道（DRO）的航天工程应用问题，研究了DRO的计算方法以及轨道特性，分析了DRO在实际力环境中的主要摄动因素，为DRO的精确建模和标称轨道设计奠定一定的理论基础。首先，利用仿真算例验证流函数法在计算DRO周期轨道族中的有效性。然后，利用该方法，通过改变雅可比常数，延拓计算DRO周期轨道族，获得不同共振比的DRO，仿真结果表明整数共振比的DRO在地月惯性坐标系中的轨迹是封闭的曲线，而共振比非整数的DRO则不封闭。最后，通过轨道外推分析影响DRO稳定性的主要摄动因素，仿真结果表明太阳引力和月球轨道偏心率是影响DRO稳定性的主要摄动因素。在动力学模型中，使用标准星历表示行星的运动状态，当积分时间多于10天时模型误差为km量级，因此在地月系这样大尺度的空间范围内，可以使用星历模型近似的分析DRO在真实力环境中的运动状态，为任务轨道设计提供依据。
-
关键词:
- 圆形限制性三体问题 /
- 流函数 /
- 拟周期轨道 /
- 远距逆行轨道(DRO) /
- 共振轨道
Abstract:For the aerospace engineering application of Distant Retrograde Orbit (DRO), a calculation method and orbit characteristics are studied, and the main perturbation factors of DRO in actual force environment are analyzed to provide a theoretical foundation for DRO's precise modeling and nominal orbit design. Firstly, the effectiveness of the stream function method in calculating the DRO periodic orbit family is verified by simulation examples. Secondly, the DRO periodic orbit family is calculated by adjusting the Jacobi constant, and the DRO orbits with different resonance ratios are obtained. The simulation results show that the trajectory of DRO with an integer resonance ratio in the Earth-Moon inertial coordinate system is a closed curve, while DRO orbits with non-integer resonance ratio are not closed. Finally, the main perturbation factors affecting DRO stability are analyzed by orbit extrapolation. The simulation results show that solar gravitation and lunar orbit eccentricity are the main perturbation factors that affect stability of DRO. In the dynamic model, the standard ephemeris is used to represent the motion state of the planet. When the integration time reaches more than 10 days, the model error is about kilometer-scale. Therefore, in the large spatial scale of the Earth-Moon system, the ephemeris model can be used to analyze the motion state of the DRO approximately in the real force environment, which could provide a basis for mission orbit design.
-
图 1 地月系的φ*(x)曲线和以点P为初值迭代得到的DRO(C=2.93)
Figure 1. φ*(x) curve of the Earth-Moon system and DRO obtained by iteration with point P as initial value(C=2.93)
图 4 DRO在地月惯性坐标系中的运动轨迹
Figure 4. Motion trajectory of DRO in the Earth-Moon inertial coordinate system
图 5 摄动力模型下地月拟周期DRO
Figure 5. The Earth-Moon quasi-periodic DRO under perturbative force model
图 6 模型1和模型2在x、y坐标及到月球距离的偏差
Figure 6. Deviation of x, y coordinate and distance to the Moon between Model 1 and Model 2
图 7 模型1和模型3在x、y坐标及到月球距离的偏差
Figure 7. Deviation of x, y coordinate and distance to the Moon between Model 1 and Model 3
图 8 模型1和模型4在x、y坐标及到月球距离的偏差
Figure 8. Deviation of x, y coordinate and distance to the Moon between Model 1 and Model 4
图 9 模型1和模型5在x、y坐标及到月球距离的偏差
Figure 9. Deviation of x, y coordinate and distance to the Moon between Model 1 and Model 5
图 10 星历模型和全力模型x、y坐标及到月球距离偏差的均方差
Figure 10. Mean square error of deviation between ephemeris model and full force model of x, y coordinate and distance to the Moon
表 1 摄动平均量级
Table 1. Average magnitude of perturbation
加速度源 加速度平均量级/(m·s-2) 月球中心引力 1.00×10-3 地球引力 1.33×10-3 太阳引力 5.60×10-6 太阳光压 8.66×10-8 月球非对称引力 1.88×10-10 金星引力 6.63×10-10 木星引力 7.16×10-11 
下载: 导出CSV
表 2 不同摄动模型轨道外推精度的均方差统计结果
Table 2. Statistical results of mean square error of trajectory extrapolation precision for different perturbation models
km 积分时间 偏差方向 轨道外推精度的均方差 不考虑月球
非对称引力不考虑木星
引力和金星引力不考虑
太阳光压不考虑
太阳引力前1个月 x坐标 0.304 9 0.160 5 98.683 1 597.5 y坐标 0.370 4 0.191 5 170.10 2 859.2 到月球距离 0.112 2 0.064 8 82.730 1 705.4 前2个月 x坐标 0.625 2 0.286 1 168.15 1 696.5 y坐标 0.899 3 0.413 6 295.72 3 161.1 到月球距离 0.239 6 0.122 6 161.87 1 792.0 前3个月 x坐标 0.902 3 0.374 4 334.18 2 448.4 y坐标 1.183 3 0.499 9 513.36 5 126.9 到月球距离 0.321 4 0.154 6 254.59 2 784.8
下载: 导出CSV
-
[1] HENON M.Numerical exploration of the restricted problem.V.Hill's case: Periodic orbits and their stability[J].Astronomy and Astrophysics, 1969, 1: 223-238. doi: 10.1023/A:1022518422926 [2] 钱霙婧.地月空间拟周期轨道上航天器自主导航与轨道保持研究[D].哈尔滨: 哈尔滨工业大学, 2013.QIAN Y J.Research on autonomous navigation and stationkeeping for quasi-periodic orbit in the Earth-Moon system[D].Harbin: Harbin Institute of Technology, 2013(in Chinese). [3] SZEBEHELY V. Theory of orbits:The restricted problem of three bodies[M].New York:Academic Press, 1967:381-442. [4] HOU X Y, LIU L.On quasi-periodic motions around the triangular libration points of the real Earth-Moon system[J].Celestial Mechanics and Dynamics Astronomy, 2010, 108:301-313. doi: 10.1007/s10569-010-9305-3 [5] HOU X Y, LIU L.On quasi-periodic motions around the collinear libration points of the real Earth-Moon system[J].Celestial Mechanics and Dynamics Astronomy, 2011, 110:71-98. doi: 10.1007/s10569-011-9340-8 [6] BEZROUK C J, PARKER J.Long duration stability of distant retrograde orbits: AIAA-2014-4424[R].Reston: AIAA, 2014. [7] TURNER G.Results of long-duration simulation of distant retrograde orbits[J].Aerospace, 2016, 3(4):37. doi: 10.3390/aerospace3040037 [8] LAM T, WHIFFEN G J.Exploration of distant retrograde orbits around Europa[C]//Proceedings of the AAS/AIAA Space Flight Mechanics Meeting.San Diego: AAS Publications Office, 2005: AAS05-110. [9] MAZANEK D D, MERRILL R G, BROPHY J R, et al.Asteroid redirect mission concept:A bold approach for utilizing space resources[J].Acta Astronautica, 2015, 117:163-171. doi: 10.1016/j.actaastro.2015.06.018 [10] STRANGE N, DAMON L, MCELRATH T, et al.Overview of mission design for NASA asteroid redirect robotic mission concept[C]//33rd International Electric Propulsion Conference, 2013: 6-10. [11] BEZROUK C, PARKER J S.Ballistic capture into distant retrograde orbits from interplanetary space[C]//Proceedings of the AAS/AIAA Space Flight Mechanics Meeting.San Diego: AAS Publications Office, 2015: AAS15-302. [12] BROPHY J R, FRIEDMAN L, CULICK F, et al.Asteroid retrieval feasibility study[C]//Proceedings of 2012 IEEE Aerospace Conference.Piscataway: IEEE Press, 2012: 1-16. [13] OCAMPO C A, ROSBOROUGH G W.Transfer trajectories for distant retrograde orbiters of the Earth[C]//Proceedings of the 3rd Annual Spaceflight Mechanics Meeting.Washington, D.C.: NASA, 1993: 1177-1200. [14] DEMEYER J, GURFIL P.Transfer to distant retrograde orbits using manifold theory[J].Journal of Guidance, Control, and Dynamics, 2007, 30(5):1261-1267. doi: 10.2514/1.24960 [15] SCOTT C J, SPENCER D B.Calculating transfer families to periodic distant retrograde orbits using differential correction[J].Journal of Guidance, Control, and Dynamics, 2010, 33(5):1592-1605. doi: 10.2514/1.47791 [16] STRAMACCHIA M, COLOMBO C, BERNELLI Z F.Distant retrograde orbits for space-based near Earth objects detection[J].Advances in Space Research, 2016, 58(6):967-988. doi: 10.1016/j.asr.2016.05.053 [17] XU M, XU S J.Exploration of distant retrograde orbits around Moon[J].Acta Astronautica, 2009, 65(5-6):853-860. doi: 10.1016/j.actaastro.2009.03.026 [18] MURAKAMI N, YAMANAKA K.Trajectory design for rendezvous in lunar distant retrograde orbit[C]//Proceedings of 2015 IEEE Aerospace Conference.Piscataway: IEEE Press, 2015: 1-13. [19] CONTE D, CARLO M D, HO K, et al.Earth-Mars transfers through Moon distant retrograde orbits[J].Acta Astronautica, 2018, 143:372-379. doi: 10.1016/j.actaastro.2017.12.007 [20] LARA M.Nonlinear librations of distant retrograde orbits:A perturbative approach-The Hill problem case[J].Nonlinear Dynamics, 2018, 93(4):2019-2038. doi: 10.1007%2Fs11071-018-4304-0 [21] LARA M.Design of distant retrograde orbits based on a higher order analytical solutiont[C]//Proceedings of the International Astronautical Congress (IAC).Bremen: International Astronautical Federation(IAF), 2018: 562-578. [22] BEZROUK C, PARKER J S.Long term evolution of distant retrograde orbits in the Earth-Moon system[J].Astrophysics and Space Science, 2017, 362:176. doi: 10.1007/s10509-017-3158-0 [23] 李明涛.共线平动点任务节能轨道设计与优化[D].北京: 中国科学院空间科学与应用研究中心, 2010.LI M T.Low energy trajectory design and optimization for collinear libration points missions[D].Beijing: Center for Space Science and Applied Research, Chinese Academy of Sciences, 2010(in Chinese). [24] DICHMANN D J, LEBOIS R, CARRICO J P.Dynamics of orbits near 3: 1 resonance in the Earth-Moon system[J].The Journal of the Astronautical Sciences, 2013, 60(1):51-86. doi: 10.1007/s40295-014-0009-x [25] ANATOLE K, BORIS H.Introduction to the modern theory of dynamical systems[M].Cambridge:Cambridge University, 1996:451-488. [26] CHRISTIAN B, LORENZO J D, MARELO V.Dynamics beyond uniform hyperbolicity:A global geometric and probabilistic perspective[M].Berlin:Springer, 2005:13-24. 期刊类型引用(7)
1. 张晨. 基于数值延拓的日月综合借力DRO入轨策略. 北京航空航天大学学报. 2024(04): 1176-1186 . 
本站查看2. 黄逸丹，黄勇，樊敏，李培佳. 基于地基测量数据的月球DRO轨道定轨精度分析. 深空探测学报(中英文). 2024(04): 405-413 . 
百度学术3. 王波，薛璐瑶，彭玉明，段晓闻，张嵬，谢攀，陆希. 日地DRO近地小行星资源勘探普查任务轨道设计与监测效能分析. 上海航天(中英文). 2024(S1): 253-260 . 百度学术4. 刘佳，宋叶志，黄乘利，胡小工，谭龙玉. 地月DRO星载光学测量近地小行星轨道确定. 天文学报. 2023(06): 83-100 . 百度学术5. 陈天冀，周晚萌，和星吉，彭祺擘，徐明，吕纪远. 考虑环月交会约束的地月转移轨道设计. 宇航学报. 2023(12): 1830-1838 . 百度学术6. 陈冠华，杨驰航，张晨，张皓. 地月空间的远距离逆行轨道族及其分岔研究. 北京航空航天大学学报. 2022(12): 2576-2588 . 本站查看7. 刘文芳，胡诗杨，刘福窑. 圆型限制性三体问题的动力学特征. 上海工程技术大学学报. 2021(03): 272-280 . 百度学术其他类型引用(3)
-
下载:
百度学术
点击查看大图
计量
- 文章访问数: 2481
- HTML全文浏览量: 122
- PDF下载量: 360
- 被引次数: 10
