-
摘要:
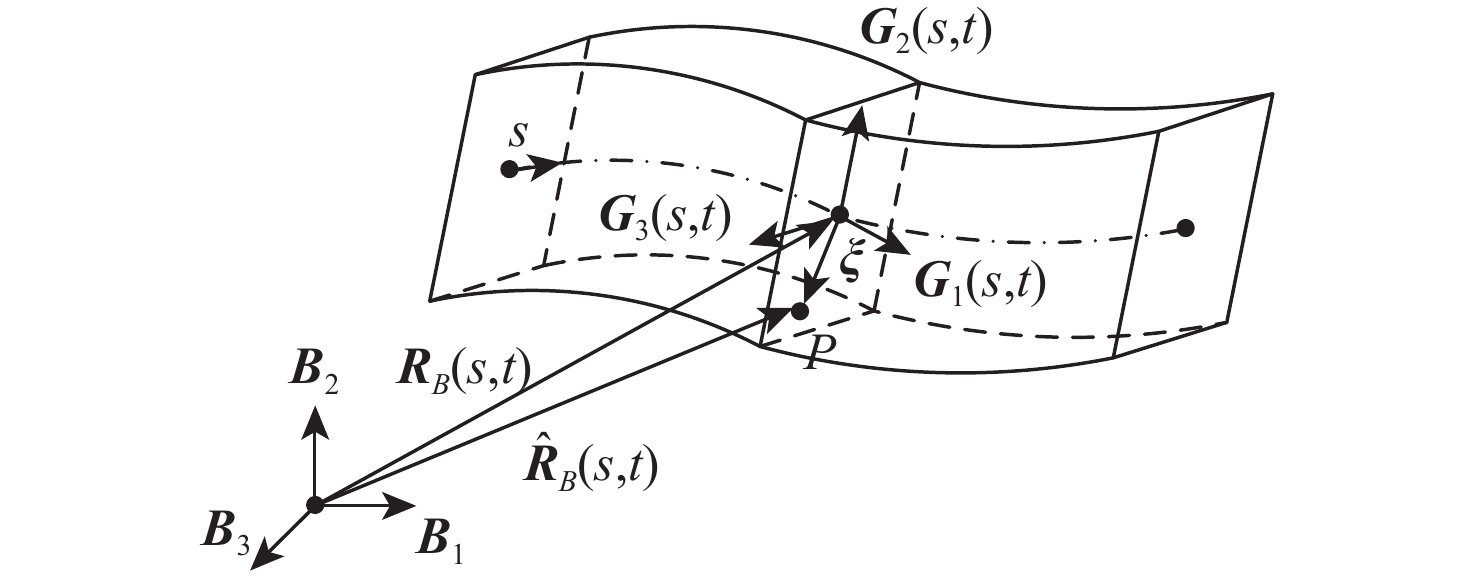
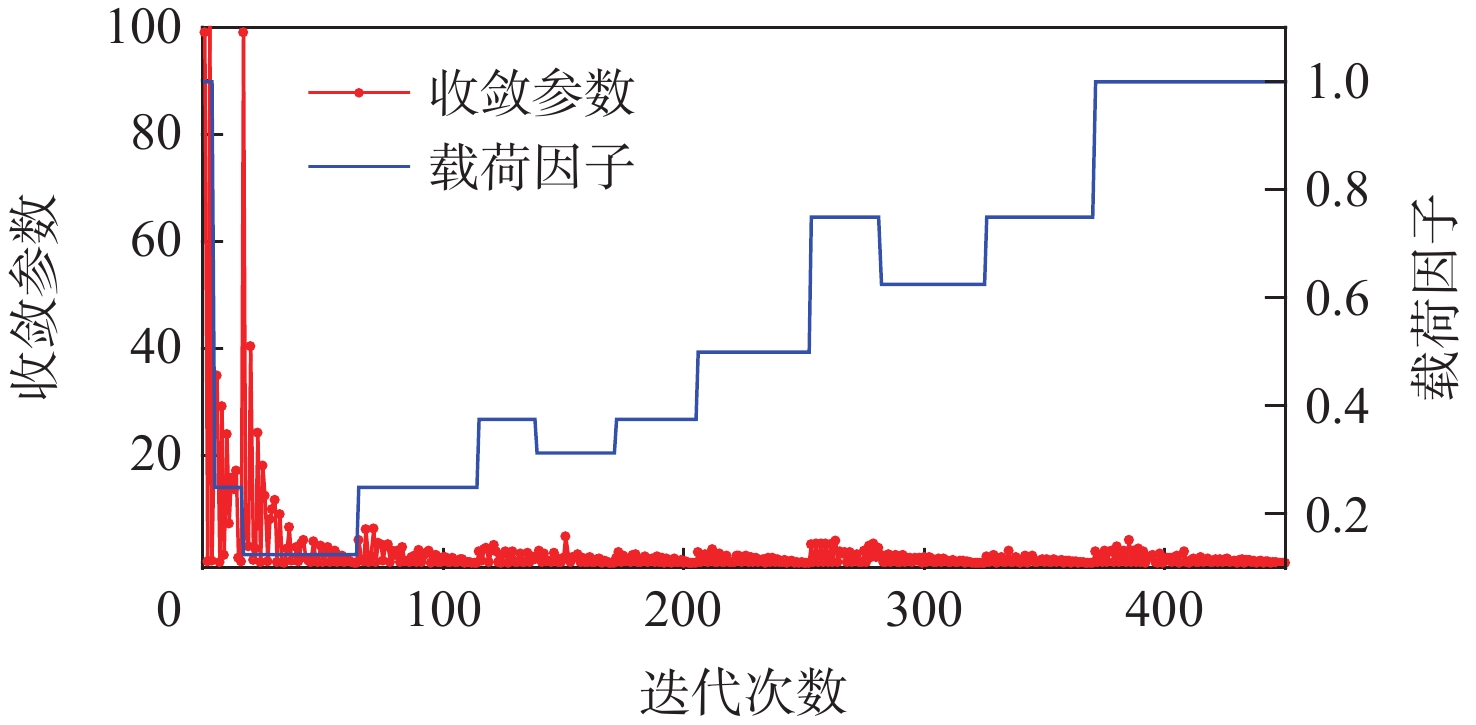
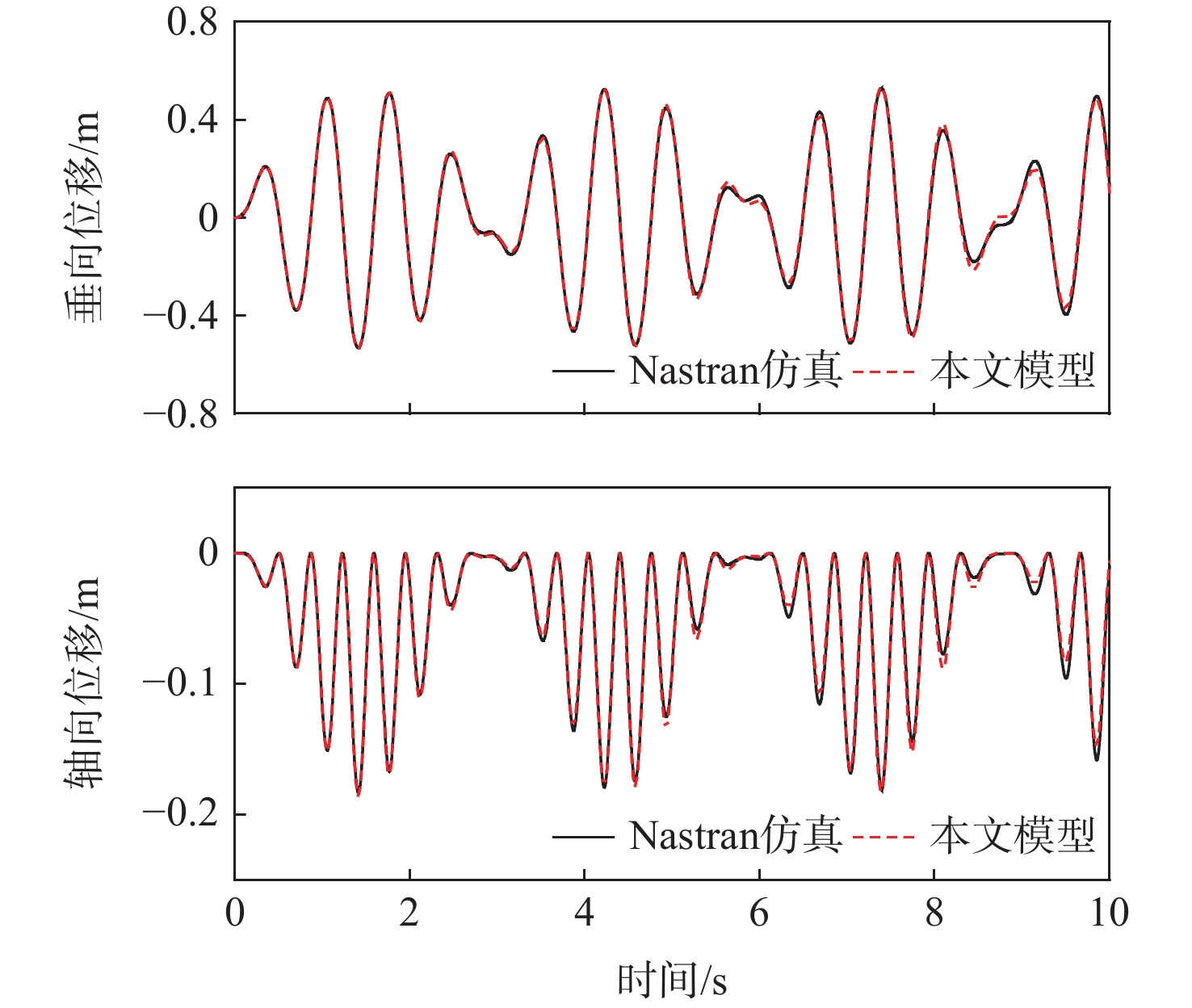
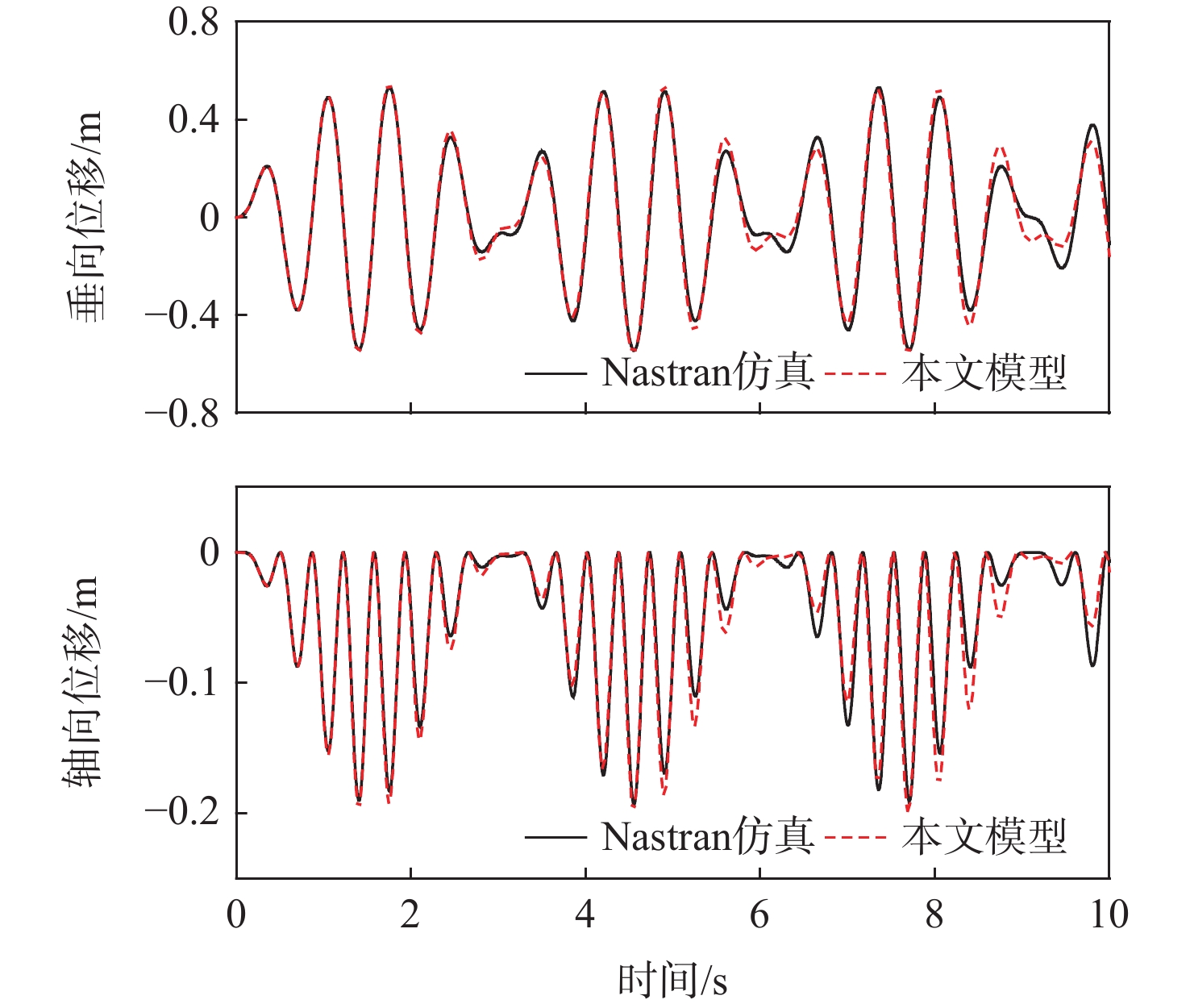
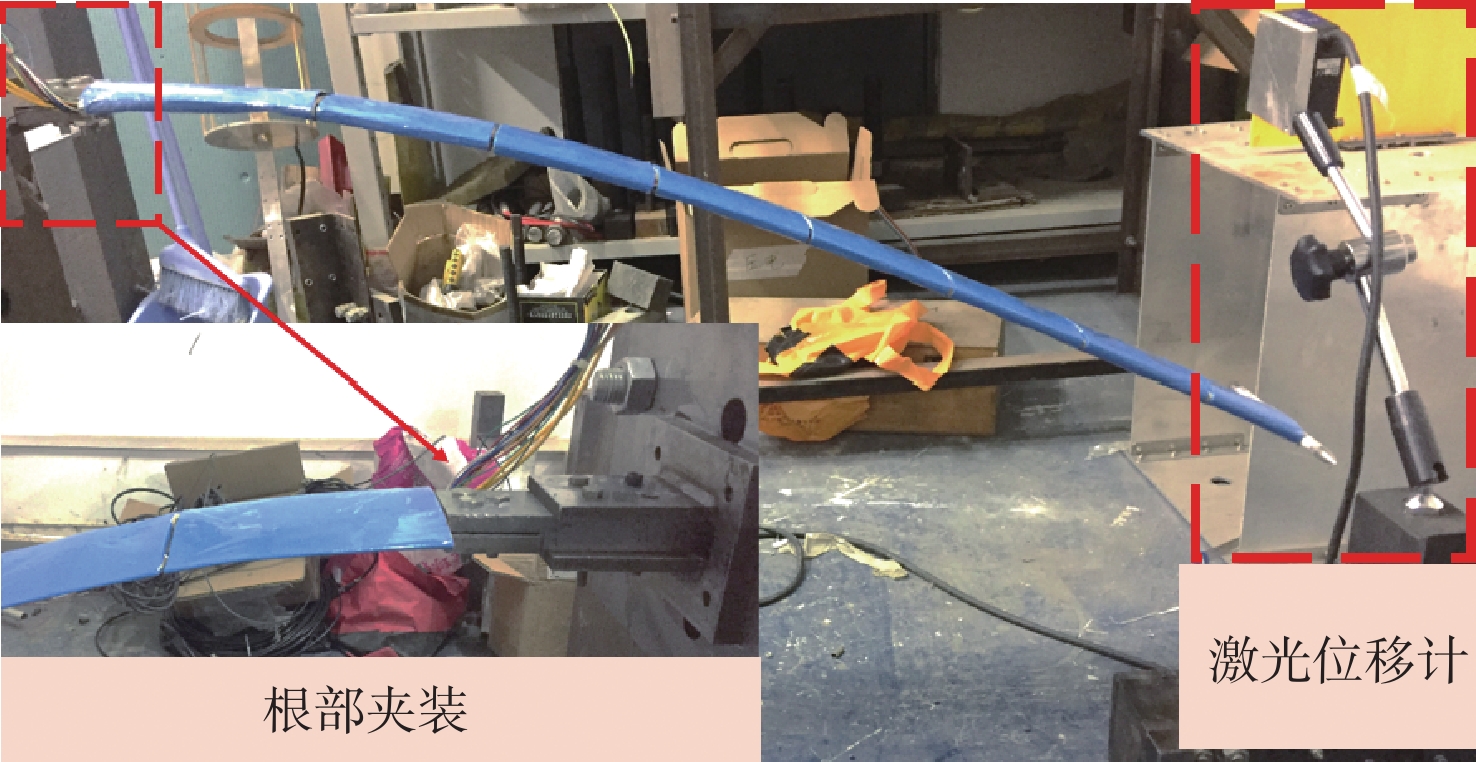
柔性机翼在气动载荷作用下产生较大变形,几何非线性因素不容忽视。利用机翼柔性特点,通常采用梁模型进行结构建模。从几何精确梁理论出发,结合Hamilton原理推导了几何非线性梁的动力学平衡方程。不同于经典的位移基有限元,采用梁广义应变作为插值变量,得到广义质量阵、广义阻尼阵、刚度阵及载荷列向量,建立非线性应变梁模型。结合Newmark数值算法和牛顿-拉夫森(Newdon-Raphson)迭代法建立了动力学方程求解算法。针对典型算例,开展静、动力学分析,并分别与有限元软件的仿真结果进行对比。结果表明:在相当计算精度下,所建模型收敛特性更好。为进一步验证所建模型在工程实际应用中的精度和有效性,开展针对典型大展弦比机翼的地面静力试验。试验表明:仿真结果与激光位移计和光纤传感设备测得的变形值具有较高的一致性,验证了所建模型具有较高精度。
Abstract:Under the action of aerodynamic forces, flexible wings undergo large deformations, for which geometric nonlinearity cannot be ignored. By taking advantage of their slenderness, flexible wings can be modeled as beams. This paper developed the dynamic equilibrium equations of nonlinear beams based on the geometrically exact beam theory and Hamilton principle. Different from the classical displacement-based finite element, this work took the generalized strains as interpolated variables and obtained the generalized mass matrix, damping matrix, stiffness matrix and force vectors, based on which a strain-based nonlinear beam model was proposed. The nonlinear dynamic equations were then solved by Newmark method combined with Newton-Raphson iterations with typical examples investigated from both static and dynamic perspectives. Next, the results were compared with those calculated by finite-element software, which proved that the strain-based beam model has better convergence with the comparable accuracy. The method was further verified by static ground tests of a large-aspect-ratio wing model, during which laser displacement sensor and fiber optic sensing technology were utilized to measure the structural deformation. The excellent agreement between numerical results calculated by the proposed method and the test results further validated the accuracy of the strain-based formulation.
-
Key words:
- geometric nonlinearity /
- strain /
- beam /
- structural dynamics /
- finite-element method
-
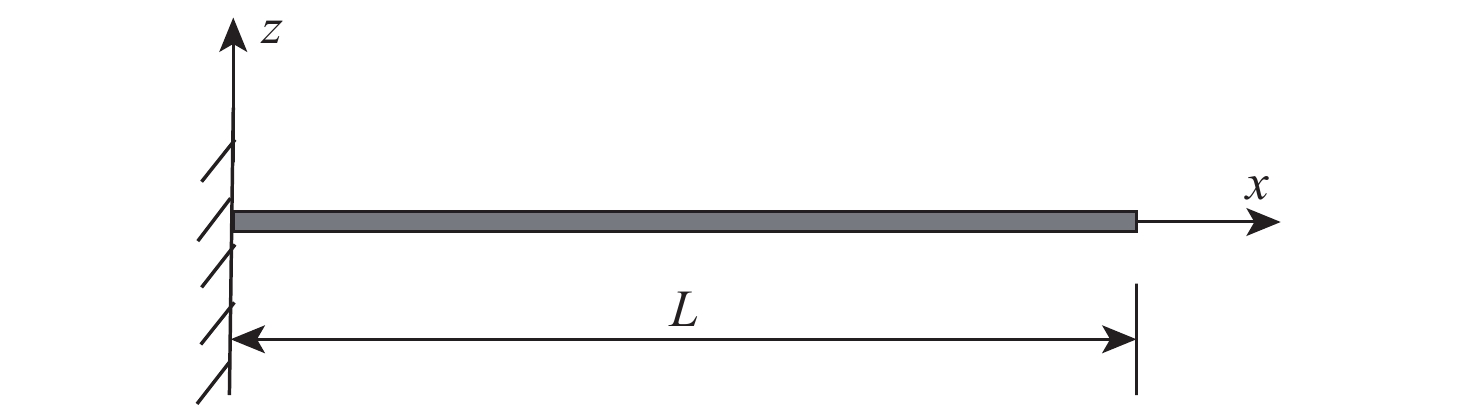
表 1 悬臂梁模型物理属性
Table 1. Properties of cantilevered beam
参数 数值 长度L/m 1.00 弹性模量E/GPa 210 剪切模量G/GPa 84.2 横截面宽度b/mm 35 横截面高度h/mm 1.5 密度ρ/(kg·m−3) 7.75×103 扭转惯量Ix/(kg·m) 5.37×10−9 面外弯曲惯量Iy/(kg·m) 9.84×10−12 面内弯曲惯量Iz/(kg·m) 5.36×10−9 表 2 不同端部载荷作用下端部垂向位移
Table 2. Tip vertical displacements with respect to different tip forces
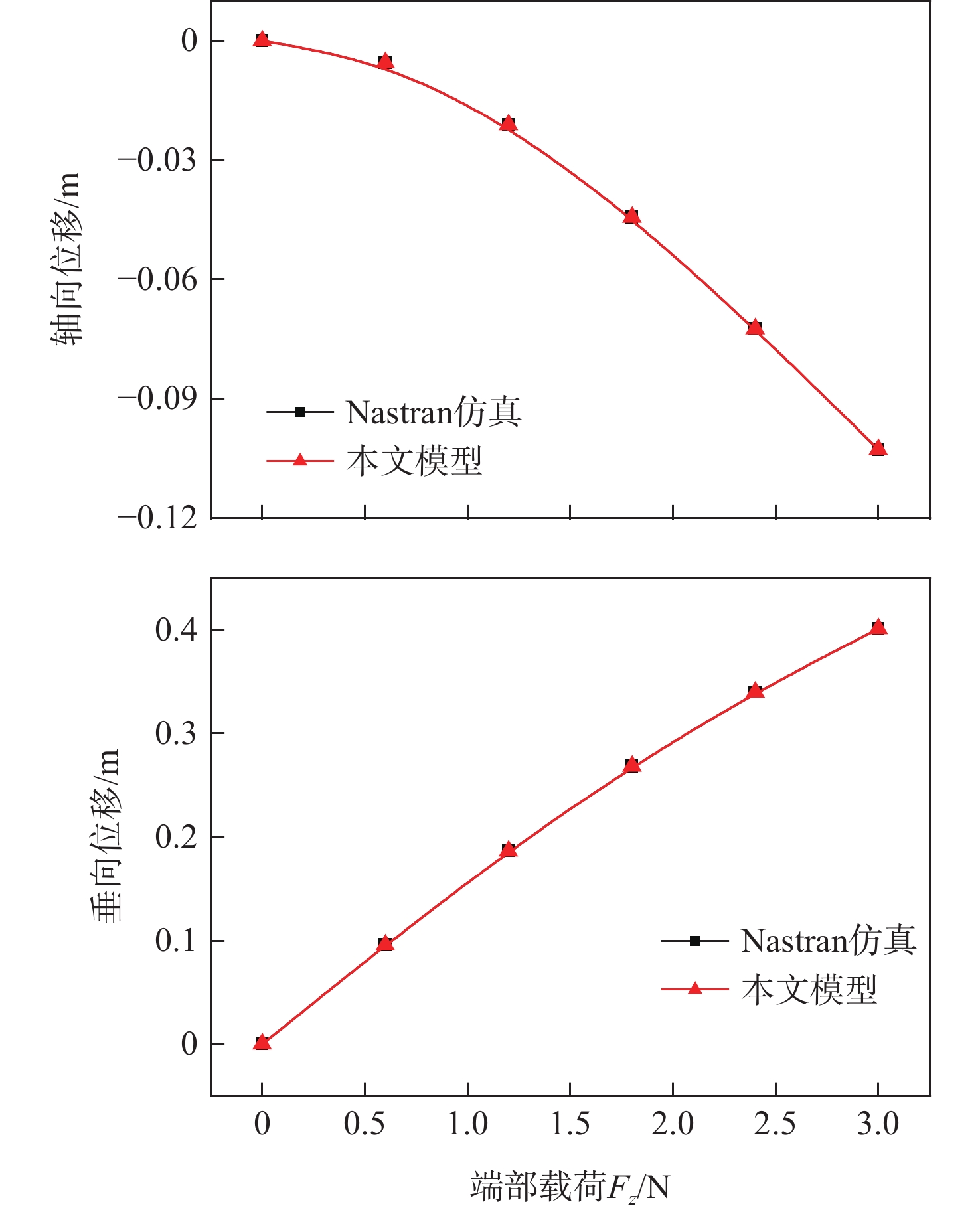
端部载荷/N 端部垂向位移/m 相对误差/% Nastran仿真 本文模型 0.6 0.09584 0.09578 −0.063 1.2 0.18655 0.18643 −0.064 1.8 0.26852 0.26836 −0.060 2.4 0.34020 0.33999 −0.062 3.0 0.40165 0.40140 −0.062 表 3 不同端部载荷作用下端部轴向位移
Table 3. Tip axial displacements with respect to different tip forces
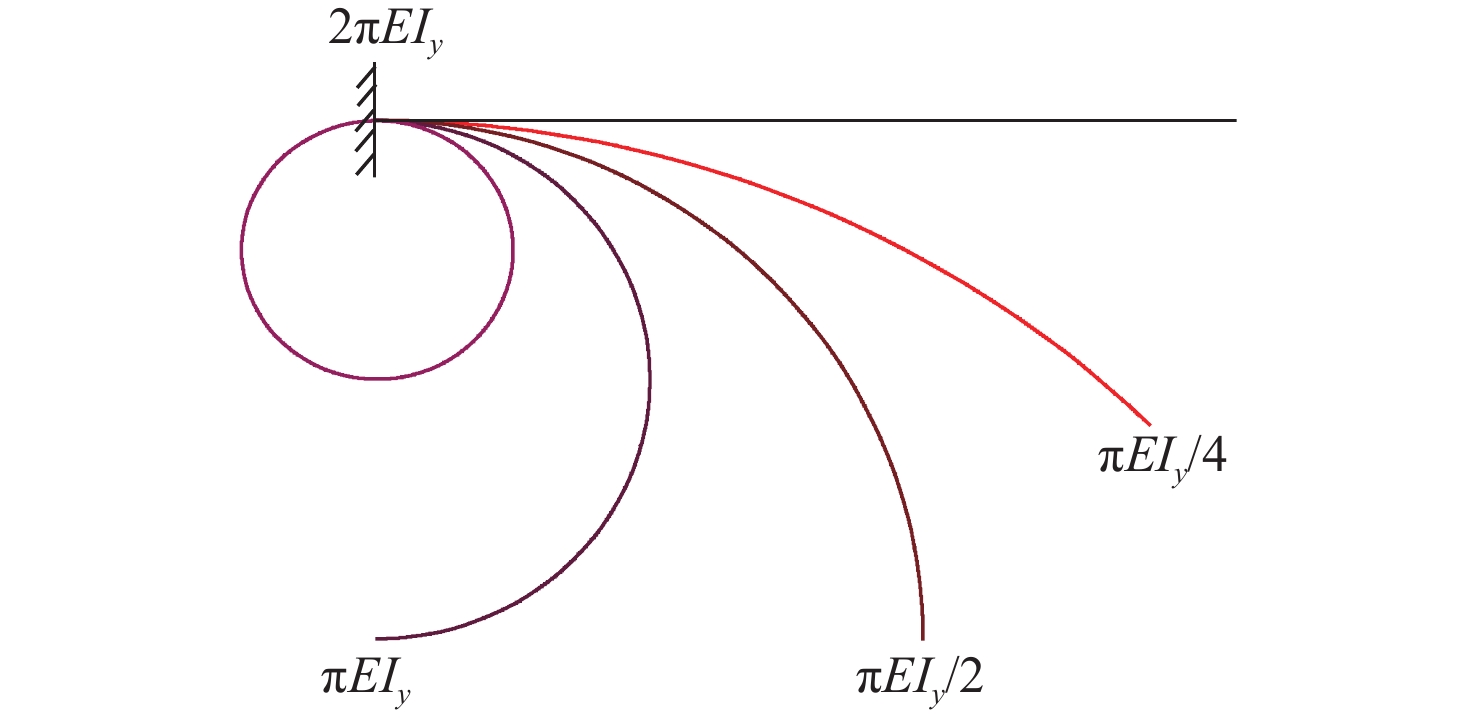
端部载荷/N 端部轴向位移/m 相对误差/% Nastran仿真 本文模型 0.6 −0.005525 −0.005523 −0.040 1.2 −0.021120 −0.021110 −0.047 1.8 −0.044360 −0.044340 −0.045 2.4 −0.072380 −0.072350 −0.041 3.0 −0.102720 −0.102670 −0.049 表 4 本文模型各迭代步端部垂向位移
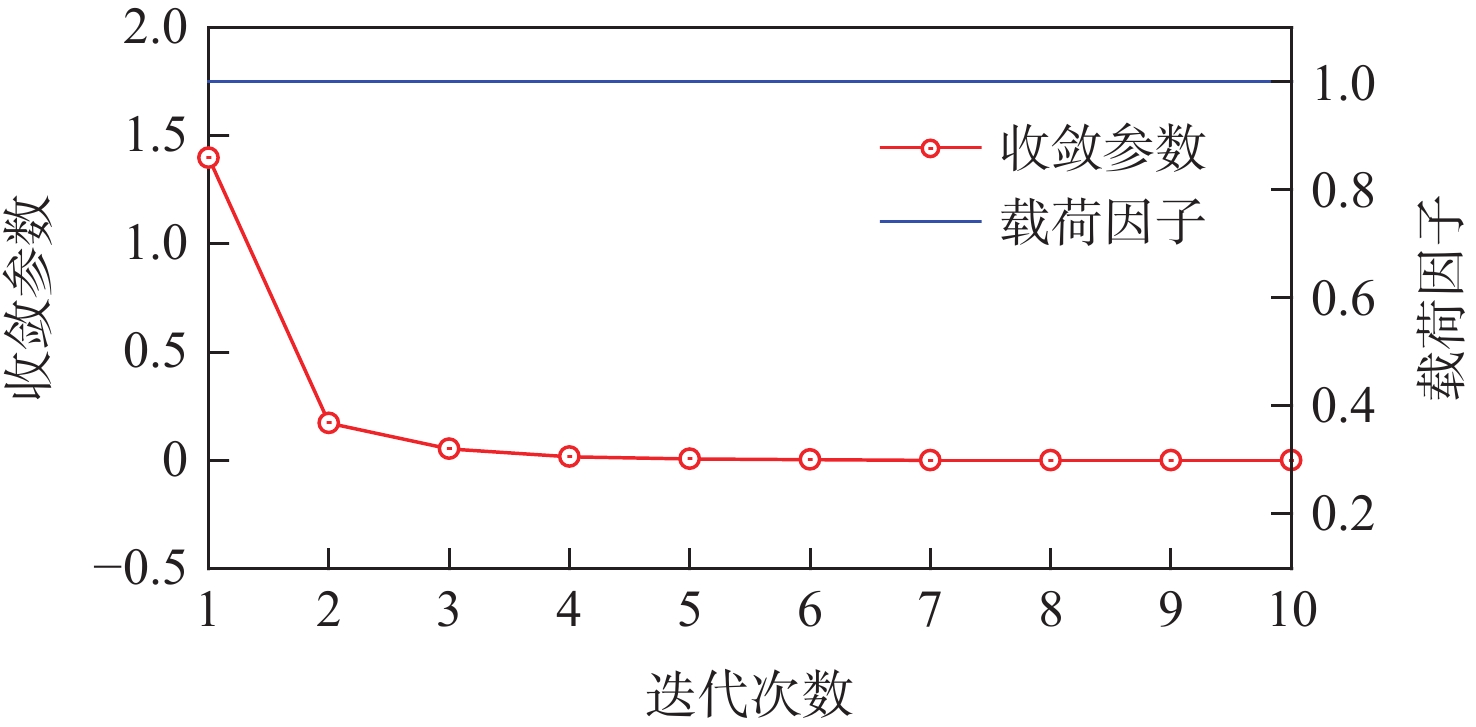
Table 4. Tip vertical displacements of each iteration step in proposed model
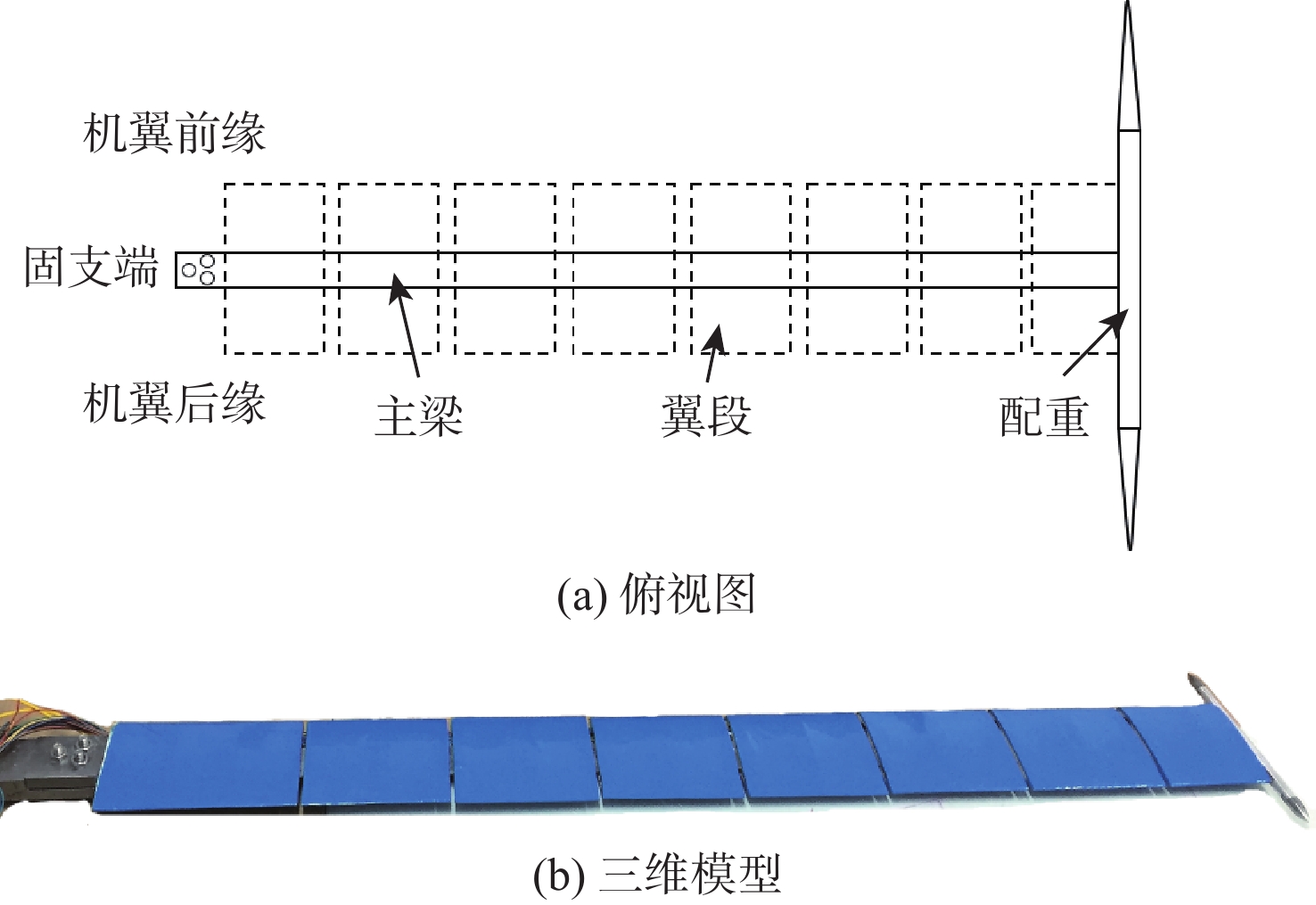
迭代次数 端部垂向位移/m 1 0.447575 2 0.386251 3 0.405881 4 0.400023 5 0.401817 6 0.401272 7 0.401438 8 0.401388 9 0.401403 10 0.401398 表 5 机翼模型主要参数
Table 5. Main parameters of wing model
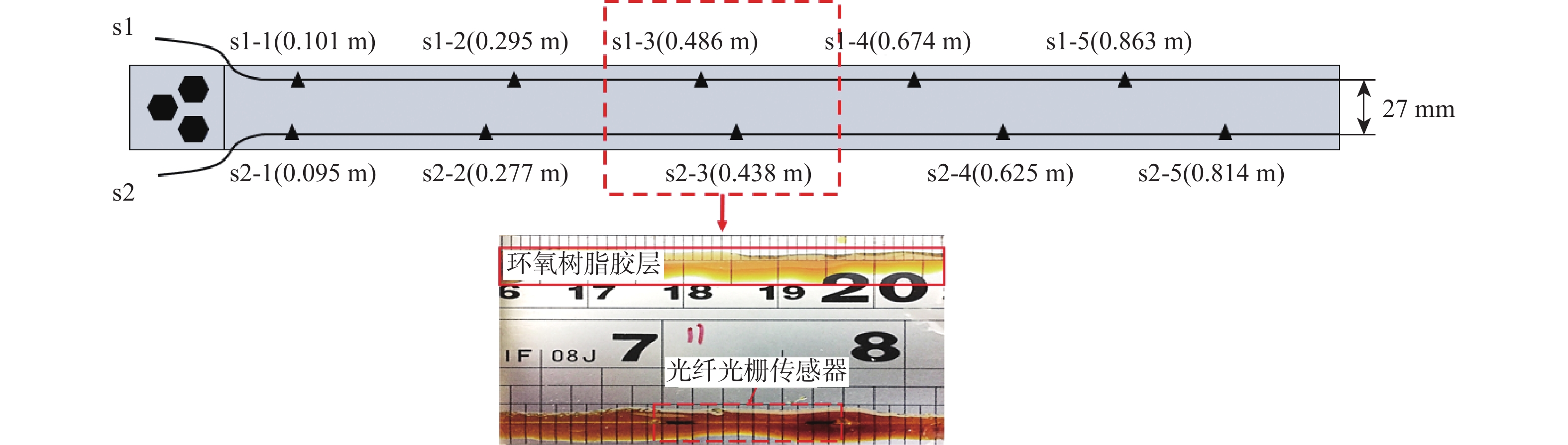
机翼展长/m 机翼弦长/m 机翼总质量/kg 1.0 0.01 0.672 表 6 不同展向位置机翼垂向变形
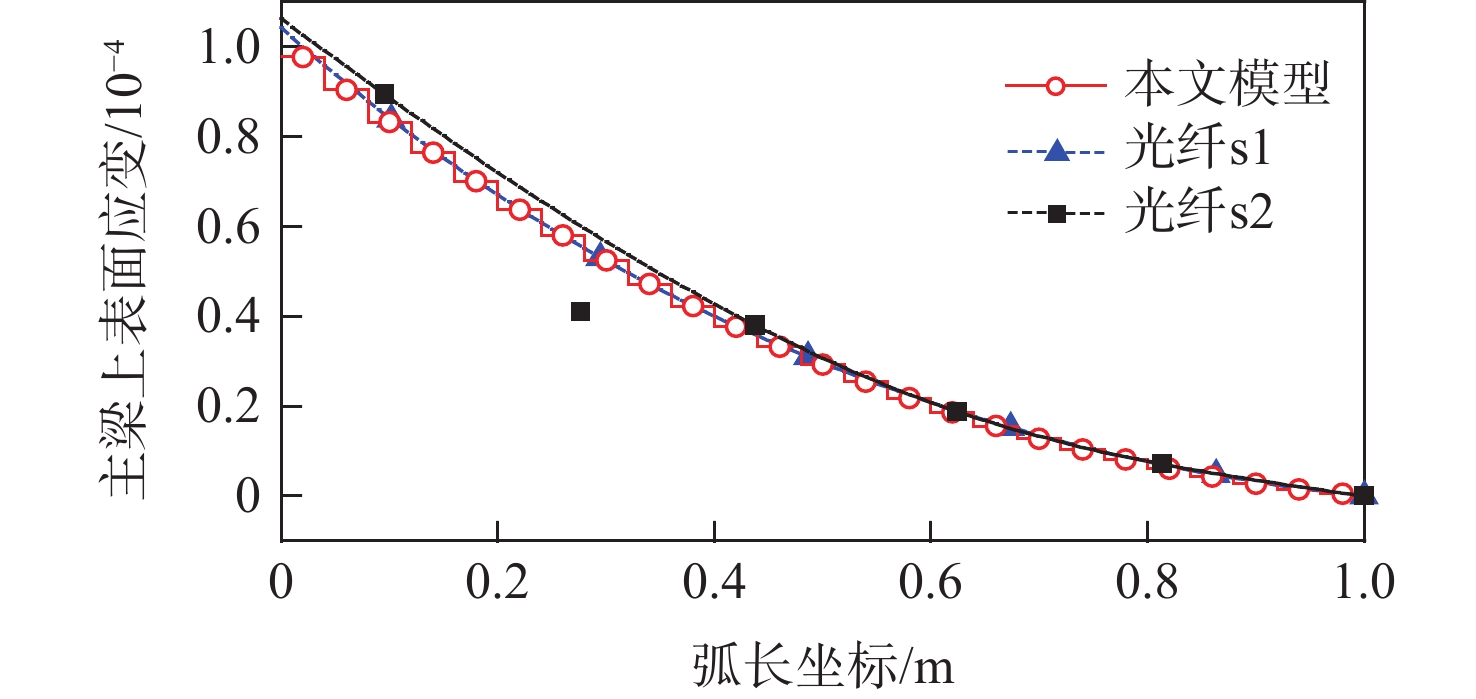
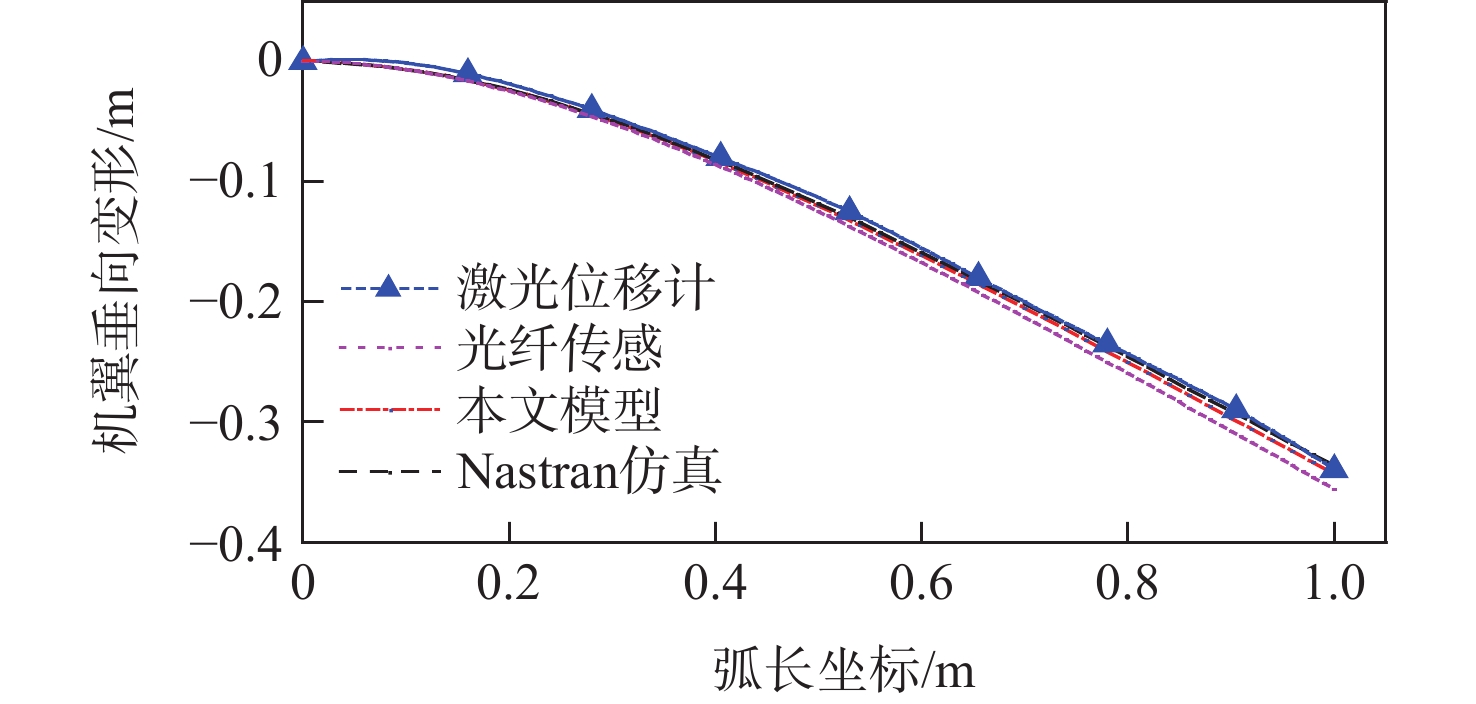
Table 6. Vertical displacements at different positions
展向位置/m 试验值/m 垂向变形z2/m z2−z1的
相对误差/%激光
位移计光纤
传感平均值z1 0.2 −0.01851 −0.02457 −0.02154 −0.02364 −0.21 0.4 −0.07833 −0.08559 −0.08196 −0.08243 −0.05 0.6 −0.15509 −0.16753 −0.16131 −0.16149 −0.02 0.8 −0.24355 −0.25978 −0.25167 −0.25058 0.11 1.0 −0.34 −0.35587 −0.34794 −0.34323 0.47 -
[1] 谢长川, 吴志刚, 杨超. 大展弦比柔性机翼的气动弹性分析[J]. 北京航空航天大学学报, 2003, 29(12): 1087-1090. doi: 10.3969/j.issn.1001-5965.2003.12.007XIE C C, WU Z G, YANG C. Aeroelastic analysis of flexible large aspect ratio wing[J]. Journal of Beijing University of Aeronautics and Astronautics, 2003, 29(12): 1087-1090(in Chinese). doi: 10.3969/j.issn.1001-5965.2003.12.007 [2] FUNG Y C, TONG P. Classical and computational solid mechanics[M]. Singapore: World Scientific, 2001. [3] 朱菊芬, 周承芳, 吕和祥. 一般杆系结构的非线性数值分析[J]. 应用数学和力学, 1987, 8(12): 1099-1109.ZHU J F, ZHOU C F, LYU H X. The nonlinear numerical analysis method for frames[J]. Applied Mathematics and Mechanics, 1987, 8(12): 1099-1109(in Chinese). [4] LOVE A E H. A treatise on the mathematical theory of elasticity[M]. 4th ed. New York: Dover, 1944. [5] REISSNER E. On one-dimensional large-displacement finite-strain beam theory[J]. Studies in Applied Mathematics, 1973, 52(2): 87-95. doi: 10.1002/sapm197352287 [6] SIMO J C. A finite strain beam formulation. The three-dimensional dynamic problem. Part I[J]. Computer Methods in Applied Mechanics and Engineering, 1985, 49(1): 55-70. doi: 10.1016/0045-7825(85)90050-7 [7] SIMO J C, VU-QUOC L. A three-dimensional finite-strain rod model. part II: Computational aspects[J]. Computer Methods in Applied Mechanics and Engineering, 1986, 58(1): 79-116. doi: 10.1016/0045-7825(86)90079-4 [8] IURA M, ATLURI S N. On a consistent theory, and variational formulation of finitely stretched and rotated 3-D space-curved beams[J]. Computational Mechanics, 1988, 4(2): 73-88. doi: 10.1007/BF00282411 [9] DANIELSON D A, HODGES D H. Nonlinear beam kinematics by decomposition of the rotation tensor[J]. Journal of Applied Mechanics, 1987, 54(2): 258-262. doi: 10.1115/1.3173004 [10] CRISFIELD M A, JELENIĆ G. Objectivity of strain measures in the geometrically exact three-dimensional beam theory and its finite-element implementation[J]. Proceedings of the Royal Society of London Series A:Mathematical, Physical and Engineering Sciences, 1999, 455(1983): 1125-1147. doi: 10.1098/rspa.1999.0352 [11] ZHONG P N, HUANG G J, YANG G W. Frame-invariance in finite element formulations of geometrically exact rods[J]. Applied Mathematics and Mechanics, 2016, 37(12): 1669-1688. doi: 10.1007/s10483-016-2147-8 [12] SIMO J C, TARNOW N, DOBLARE M. Non-linear dynamics of three-dimensional rods: Exact energy and momentum conserving algorithms[J]. International Journal for Numerical Methods in Engineering, 1995, 38(9): 1431-1473. doi: 10.1002/nme.1620380903 [13] ZUPAN D, SAJE M. Finite-element formulation of geometrically exact three-dimensional beam theories based on interpolation of strain measures[J]. Computer Methods in Applied Mechanics and Engineering, 2003, 192(49-50): 5209-5248. doi: 10.1016/j.cma.2003.07.008 [14] ČEŠAREK P, SAJE M, ZUPAN D. Kinematically exact curved and twisted strain-based beam[J]. International Journal of Solids and Structures, 2012, 49(13): 1802-1817. doi: 10.1016/j.ijsolstr.2012.03.033 [15] SU W H, CESNIK C E S. Strain-based geometrically nonlinear beam formulation for modeling very flexible aircraft[J]. International Journal of Solids and Structures, 2011, 48(16-17): 2349-2360. doi: 10.1016/j.ijsolstr.2011.04.012 [16] MENG Y, WAN Z Q, XIE C C, et al. Time-domain nonlinear aeroelastic analysis and wind tunnel test of a flexible wing using strain-based beam formulation[J]. Chinese Journal of Aeronautics, 2021, 34(1): 380-396. doi: 10.1016/j.cja.2020.07.027 [17] MENG Y, XIE C C, WAN Z Q. Strain-based shape prediction for flexible beam-like structures[C]//Proceedings of the AIAA Scitech 2019 Forum. Reston: AIAA, 2019. [18] 肖业伦. 航空航天器运动的建模 飞行动力学的理论基础[M]. 北京: 北京航空航天大学出版社, 2003: 6-17.XIAO Y L. Modeling of aerospace vehicle motion: Theoretical basis of flight dynamics[M]. Beijing: Beihang University Press, 2003: 6-17 (in Chinese). [19] MENG Y, XIE C C, WAN Z Q. Deformed wing shape prediction using fiber optic strain data[C]//International Forum on Aeroelasticity and Structural Dynamics 2017. Como: IFASD, 2017. 期刊类型引用(1)
1. 祖洪飞,曹贤浩,陈旭雯. 基于大变形理论的机械手四维柔性位移感器研究及标定. 传感技术学报. 2022(10): 1326-1334 .  百度学术
百度学术其他类型引用(2)
-







 下载:
下载:

 百度学术
百度学术

