-
摘要:
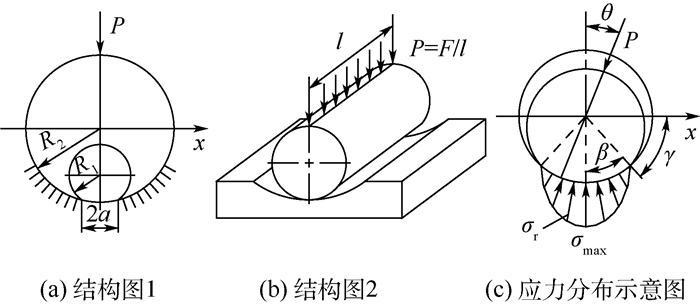
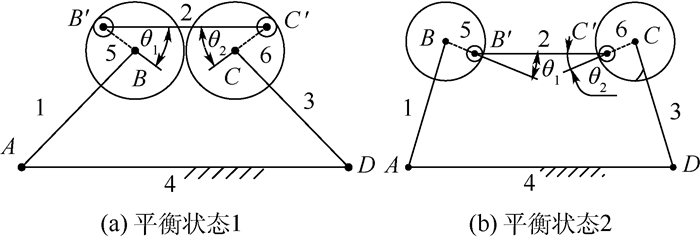
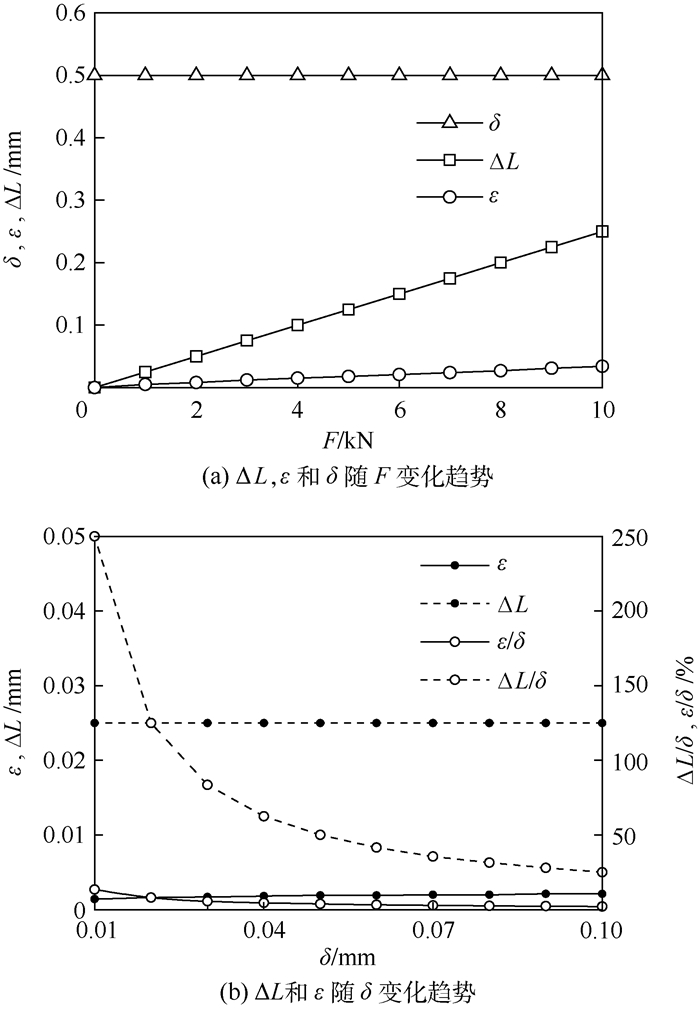
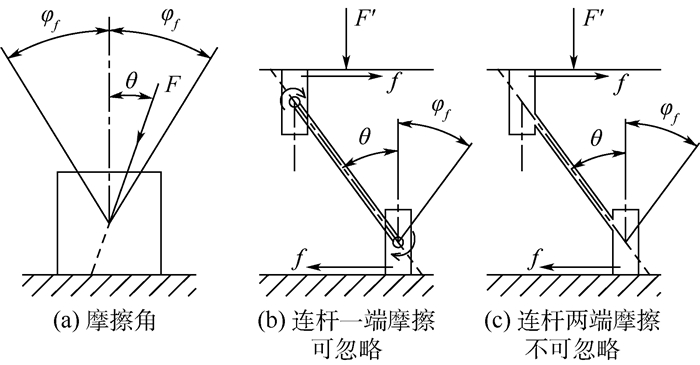
滑动配合条件下，转动副（R副）通过接触面间的相对滑动提供一个转动自由度。由于接触面间的摩擦和间隙共同作用，R副会在一个小的摆角范围内发生自锁，这一摆角将在机构平衡时带来连杆长度误差和连杆偏角误差。本文指出了R副中临界角的存在，讨论了使得R副实现自锁的临界角范围，在考虑接触变形和不考虑接触变形2种条件下对临界角进行了计算，并以一平面四连杆机构作为应用算例，综合对比了铰链间隙、接触变形和摩擦因素对所在连杆机构输出精度的影响。算例结果表明，在一般的精度计算中铰链间隙及连杆弹性变形起主要影响作用，铰链本身的接触变形量可忽略。
Abstract:Revolute pin joint (revolute pair) possesses one degree of freedom, i.e. rotation movement, through relative sliding between contact pairs. Existence of friction and clearance in revolute joints has crucial effects on the overall precision of mechanism. Using the concept of critical angle, the marginal value in which revolute joints could keep in balance state is discussed in this study. Firstly, frictional angle and self-lock caused by friction and clearance in revolute pin joints are investigated, which contribute to not only length error but also angle error in mechanism with such joints. Then, critical angles are calculated with and without contact deformation respectively. Moreover, a four-bar mechanism with one revolute joint studied above is chosen as a case study to evaluate the impact of clearance of joint, contact deformation and friction on the overall precision. Analytical results indicate that the clearance in revolute joint and elastic deformation of mechanism play the most important roles in the system, and the contact deformation of joint could be neglected in the overall precision analysis.
-
图 3 不考虑接触变形的R副临界角分析示意图
Figure 3. Schematic diagrams of critical angle for revolute pair without contact deformation
图 4 两圆柱体接触应力分布示意图
Figure 4. Schematic diagram of distribution of contact stress between two cylinders
图 5 引入一个虚拟连杆的四连杆机构示意图
Figure 5. Schematic diagram of a four-bar mechanism with one virtual link
图 6 有2个非理想铰链的四连杆机构的2种典型平衡状态
Figure 6. Two typically balanced states of four-bar mechanism with two non-ideal links
图 8 ΔL、ε和δ随F及ΔL和ε随δ的变化示意图
Figure 8. Variation of ΔL, ε and δ with F and variation of ΔL, ε with δ
表 1 算例用四连杆机构参数
Table 1. Parameters of four-bar mechanism in case study
参数类型 数值 四连杆机构
参数条件LAB=LB′C′=LCD=50 mm
LAD=100 mm
A杆=6.25π mm2圆柱铰链
参数条件RB=RC，RB′=RC′(θmax1=θmax2=θmax, L5=L6)
l柱销=10 mm变量 铰链间隙δ
连杆压力F
接触表面滑动摩擦系数μ
下载: 导出CSV
-
[1] 邹慧君, 高峰.现代机构学进展[M].北京:高等教育出版社, 2007:93-94.ZOU H J, GAO F.The progress of modern organizations[M].Beijing:Higher Education Press, 2007:93-94(in Chinese). [2] 郭卫东.机械原理[M].北京:科学出版社, 2010:168.GUO W D.Mechanical principle[M].Beijing:Science Press, 2010:168(in Chinese). [3] 黄昔光, 廖启征.空间6R串联机器人机构位置逆解新算法[J].北京航空航天大学学报, 2010, 36(3):295-298. http://bhxb.buaa.edu.cn/CN/abstract/abstract8500.shtmlHUANG X G, LIAO Q Z.A new algorithm position inverse solution of 6-R serial robot mechanism[J].Journal of Beijing University of Aeronautics and Astronautics, 2010, 36(3):295-298(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract8500.shtml [4] 尚国强, 陈五一, 韩先国, 等.并联机床的球铰链设计与分析[J].机械技术史及机械设计, 2008:286-290. http://www.cnki.com.cn/Article/CJFDTOTAL-JXJS200800050.htmSHANG G Q, CHEN W Y, HAN X G, et al.Spherical hinge design and analysis of parallel machine tool[J].Mechanical Technology and Mechanical Design, 2008:286-290(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-JXJS200800050.htm [5] REIS V L, DANIEL G B, CAVALCA K L.Dynamic analysis of a lubricated planar slider-crank mechanism considering friction and Hertz contact effects[J].Mechanism and Machine Theory, 2014, 74:257-273. doi: 10.1016/j.mechmachtheory.2013.11.009 [6] ZHANG X C, ZHANG X M, CHEN Z.Dynamic analysis of a 3-RRR parallel mechanism with multiple clearance joints[J].Mechanism and Machine Theory, 2014, 78:105-115. doi: 10.1016/j.mechmachtheory.2014.03.005 [7] BAI Z F, ZHAO Y.A hybrid contact force model of revolute joint with clearance for planar mechanical systems[J].International Journal of Non-Linear Mechanics, 2013, 48:15-36. doi: 10.1016/j.ijnonlinmec.2012.07.003 [8] JUNG K.Dynamic response of a revolute joint with clearance[J].Mechanism and Machine Theory, 1995, 31(1):121-134. https://www.researchgate.net/publication/222782375_Dynamic_response_of_a_revolute_joint_with_clearance [9] PAYANDEH S, FARAZ A.Towards approximate models of coulomb frictional moments in:(I) revolute pin joints and (Ⅱ) spherical-socket ball joints[J].Journal of Engineering Mathematics, 2001, 35(403):283-296. [10] 李新友, 陈五一, 韩先国.基于正交设计的3-RPS并联机构精度分析与综合[J].北京航空航天大学学报, 2011, 37(8):979-984. http://bhxb.buaa.edu.cn/CN/abstract/abstract12045.shtmlLI X Y, CHEN W Y, HAN X G.3-RPS parallel mechanism based on orthogonal design precision analysis and synthesis[J].Journal of Beijing University of Aeronautics and Astronautics, 2011, 37(8):979-984(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract12045.shtml [11] KHAN A W, CHEN W Y.Systematic geometric error modeling for workspace volumetric calibration of a 5-axis turbine blade grinding machine[J].Chinese Journal of Aeronautics, 2010, 23(5):604-615. doi: 10.1016/S1000-9361(09)60261-2 [12] 瓦伦丁·L·波波夫.接触力学与摩擦学的原理及其应用[M].李强, 等, 译.北京:清华大学出版社, 2011:169-170.VALENTIN L P.Contact mechanics and tribology principle application[M].LI Q, et al, translated.Beijing:Tsinghua University Press, 2011:169-170(in Chinese). [13] 王元淳, 沃国纬.弹性力学[M].上海:上海交通大学出版社, 1998:133-135.WANG Y C, WO G W.Elastic mechanics[M].Shanghai:Shanghai Jiao Tong University Press, 1998:133-135(in Chinese). [14] 刘鸿文.材料力学[M].北京:高等教育出版社, 2005:34.LIU H W.Material mechanics[M].Beijing:Higher Education Press, 2005:34(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 839
- HTML全文浏览量: 55
- PDF下载量: 589
- 被引次数: 0
