链式平滑切换变体飞行器LPV鲁棒跟踪控制

贾臻; 董朝阳; 王青

doi:10.13700/j.bh.1001-5965.2016.0246

链式平滑切换变体飞行器LPV鲁棒跟踪控制

doi: 10.13700/j.bh.1001-5965.2016.0246

贾臻¹,
董朝阳^1, ,,
王青²

1.
北京航空航天大学航空科学与工程学院, 北京 100083
2.
北京航空航天大学自动化科学与电气工程学院, 北京 100083

基金项目:

国家自然科学基金 61273083

国家自然科学基金 61403028

详细信息

作者简介:
贾臻, 男, 硕士研究生。主要研究方向:切换系统、鲁棒控制、飞行器控制、飞行力学建模

董朝阳, 男, 博士, 教授, 博士生导师。主要研究方向:切换系统、飞行器控制、飞行力学建模

通讯作者:
董朝阳, E-mail:dongchaoyang@buaa.edu.cn

中图分类号: V249.1
计量
- 文章访问数: 906
- HTML全文浏览量: 156
- PDF下载量: 10
- 被引次数: 0
出版历程
- 收稿日期: 2016-03-29
- 录用日期: 2016-07-01
- 网络出版日期: 2017-04-20

LPV robust tracking control for chain smooth switched morphing aircraft

1.
School of Aeronautic Science and Engineering, Beijing University of Aeronautics and Astronautics, Beijing 100083, China
2.
School of Automation Science and Electrical Engineering, Beijing University of Aeronautics and Astronautics, Beijing 100083, China

Funds:

National Natural Science Foundation of China 61273083

National Natural Science Foundation of China 61403028

More Information

Corresponding author: DONG Chaoyang, E-mail: dongchaoyang@buaa.edu.cn

摘要

摘要:
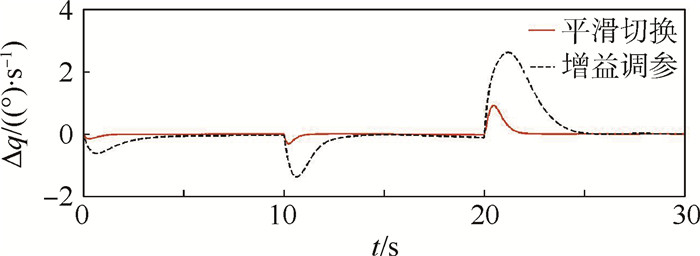
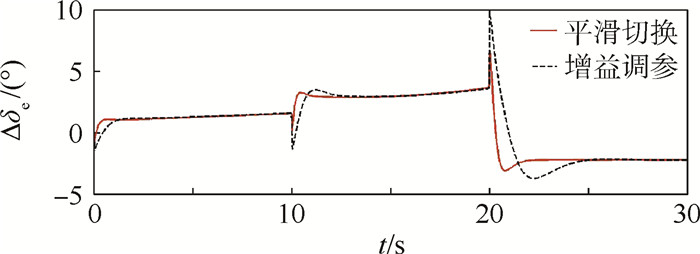
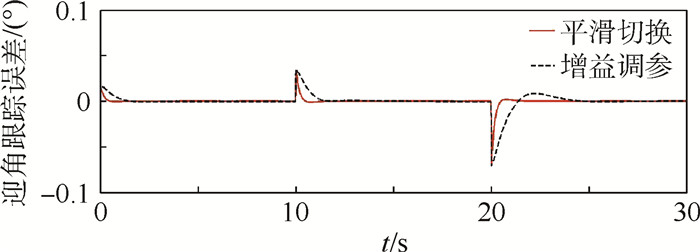
针对后掠角可变的变体飞行器，研究了一类变体飞行器的建模与控制问题。拟合气动参数与后掠角变化的关系，利用Jacobian线性化的方法得到线性变参数（LPV）模型。进一步建立一类平滑切换系统，同时引入切换序列受限的链式切换，推导了链式平滑切换系统在有限时间有界且具有鲁棒性能指标的充分条件。设计了一种平滑切换镇定控制器的求解算法，并给出控制增益的求解步骤。基于广义系统理论提出了保证变体飞行器姿态跟踪系统鲁棒稳定的充分条件，并通过算例验证所提方法有效性。
- 鲁棒控制 /
- 平滑切换 /
- 切换系统 /
- 变体飞行器 /
- 链式切换
Abstract:
Aimed at morphing aircraft with variable sweepback, this paper studies the issue of modeling and control for a class of morphing aircrafts. Fitting the relationship between aerodynamic parameters and sweepback, we developed linear parameter varying (LPV) model by Jacobian linearization approach. Then a smooth switching system approach with limited switching sequence, chain switching, is modeled, and the sufficient conditions are provided to ensure the finite-time boundedness and robust performance index of the chain smooth switched system. A solving algorithm of stabilizer for smooth switching controller is proposed, and the solving steps for gain control are presented. Based on the generalized system theory, the sufficient conditions for robust stability of the attitude tracking system are proposed. The numerical example simulation results are given to illustrate the validity of the devised approach.
- robust control /
- smooth switching /
- switching system /
- morphing aircrafts /
- chain switching

HTML全文

图 1 迎角响应曲线

Figure 1. Angle of attack response curves

下载: 全尺寸图片幻灯片

图 2 俯仰角速率响应曲线

Figure 2. Pitch angle rate response curves

下载: 全尺寸图片幻灯片

图 3 油门开度响应曲线

Figure 3. Throttle position response curves

下载: 全尺寸图片幻灯片

图 4 升降舵偏角响应曲线

Figure 4. Elevator angle response curves

下载: 全尺寸图片幻灯片

图 5 迎角跟踪误差曲线

Figure 5. Angle of attack tracking error curves

下载: 全尺寸图片幻灯片

表 1 变体飞行器构型参数

Table 1. Configuration parameters of morphing aircraft

形态	ν/(°)	J_yf/(kg·m²)	m_T/kg	m_w/kg	m_a/kg	x_w/m	x_a/m	B/m	S/m²	c_A/m
巡航	15	3 107.5	907.8	272	26.36	0	-3.2	6.803 3	4.362 1	0.710 1
高速	60	3 107.5	907.8	272	26.36	-0.607 2	3.065 6	3.84	6.079 2	1.911 7
注: ν—后掠角; J_yf—俯仰转动惯量; m_T—总重; m_a—配重；x_a—配重质心位置; B—翼展。

下载: 导出CSV

表 2 切换子系统控制增益矩阵

Table 2. Gain control matrix of switched subsystem

构型	反馈控制增益
构型	K_{x, i}	K_{β, i}
M₁₅
M₂₀
M₂₅
M₃₀
M₃₅
M₄₀
M₄₅
M₅₀
M₅₅
M₆₀

下载: 导出CSV

参考文献(19)

[1]	WEISSHAAR T A.Morphing aircraft systems:Historical perspectives and future challenges[J].Journal of Aircraft, 2013, 50(2):337-353. doi: 10.2514/1.C031456
[2]	CROSSLEY W A, SKILLEN M D, FROMMER J B.Morphing aircraft sizing using design optimization[J].Journal of Aircraft, 2011, 48(2):612-622. doi: 10.2514/1.C031180
[3]	SOFLA A Y N, MEGUID S A, TAN K T, et al.Shape morphing of aircraft wing:Status and challenges[J].Materials and Design, 2010, 31(3):1284-1292. doi: 10.1016/j.matdes.2009.09.011
[4]	HUANG R, QIU Z P.Transient aeroelastic responses and flutter analysis of a variable-span wing during the morphing process[J].Chinese Journal of Aeronautics, 2013, 26(6):1430-1438. doi: 10.1016/j.cja.2013.07.047
[5]	POPOV A V, GRIGORIE L T, BOTEZ R.Closed-loop control validation of a morphing wing using wind tunnel tests[J].Journal of Aircraft, 2010, 47(4):1309-1317. doi: 10.2514/1.47281
[6]	BALDELL D H, LEE D H, PEÑR S S.et al.Modeling and control of an aeroelastic morphing vehicle[J].Journal of Guidance, Control, and Dynamics, 2008, 31(6):1687-1699. doi: 10.2514/1.35445
[7]	RUBAGOTTI M, ZACCARIAN L, BEMPORAD A.A Lyapunovmethod for stability analysis of piecewise-affine systems over non-invariant domains[J].International Journal of Control, 2016, 89(5):950-959. doi: 10.1080/00207179.2015.1108456
[8]	CHUMALEE S, WHIDBORNE J F.Gain-scheduled H_∞ control via parameter-dependent Lyapunov functions[J].International Journal of Systems Science, 2015, 46(1):125-138. doi: 10.1080/00207721.2013.775386
[9]	ROMDLONY M Z, JAYAWARDHANA B.Stabilization with guaranteed safety using control Lyapunov-Barrier function[J].Automatica, 2016, 66:39-47. doi: 10.1016/j.automatica.2015.12.011
[10]	ANIMESH C, DANIEL T G, RICK L.Time-varying dynamics of a micro air vehicle with variable-sweep morphing[J].Journal of Guidance, Control, and Dynamics, 2012, 35(3):890-903. doi: 10.2514/1.55078
[11]	乐挺, 王立新, 艾俊强.Z型翼变体飞机的纵向多体动力学特性[J].航空学报, 2010, 31(4):679-686. http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201004006.htm YUE T, WANG L X, AI J Q.Longitudinal multibody dynamic characteristics of Z-wing morphing aircraft[J].Acta Aeronautica et Astronautica Sinica, 2010, 31(4):679-686(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201004006.htm
[12]	YUE T, WANG L X, AI J Q.Longitudinal linear parameter varying modeling and simulation of morphing aircraft[J].Journal of Aircraft, 2013, 50(6):1673-1681. doi: 10.2514/1.C031316
[13]	薛静, 杨亚洁, 刘宇, 等.基于L1自适应控制的无人机横侧向控制[J].西北工业大学学报, 2015, 33(1):40-44. http://www.cnki.com.cn/Article/CJFDTOTAL-XBGD201501008.htm XUE J, YANG Y J, LIU Y, et al. Lateral roll angle control of UAV based on L1 adaptive control method[J].Journal of Northwestern Polytechnical University, 2015, 33(1):40-44(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-XBGD201501008.htm
[14]	SEIGLER T M, NEAL D A.Analysis of transition stability for morphing aircraft[J].Journal of Guidance, Control, and Dynamics, 2009, 32(6):1947-1953. doi: 10.2514/1.44108
[15]	CUI L, CHEN L, DUAN D P.Gain-scheduling model predictive control for unmanned airship with LPV system description[J].Journal of Systems Engineering and Electronics, 2015, 26(5):1043-1051. doi: 10.1109/JSEE.2015.00113
[16]	YUE T, WANG L X, AI J Q.Gain self-scheduled H_∞ control for morphing aircraft in the wing transition process based on an LPV model[J].Chinese Journal of Aeronautics, 2013, 26(4):909-917. doi: 10.1016/j.cja.2013.06.004
[17]	段广仁, 王好谦.多模型切换控制及其在BTT导弹设计中的应用[J].航空学报, 2005, 26(2):144-147. http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB200502003.htm DUAN G R, WANG H Q.Multi-model switching control and its application to BTT missile design[J].Acta Aeronautica et Astronautica Sinica, 2005, 26(2):144-147(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB200502003.htm
[18]	HOU Y Z, WANG Q, DONG C Y.Gain scheduled control:Switched polytopic system approach[J].Journal of Guidance, Control, and Dynamics, 2011, 34(2):623-629. doi: 10.2514/1.51699
[19]	HOU Y Z, DONG C Y, WANG Q.Stability analysis of switched linear systems with locally overlapped switching law[J].Journal of Guidance, Control, and Dynamics, 2010, 33(2):396-403. doi: 10.2514/1.45795