-
摘要:
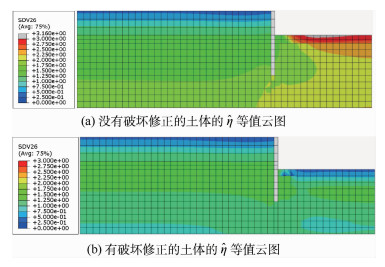
在采用UH模型的用户材料子程序（UMAT）进行有限元计算过程中时常会出现局部单元破坏的情况，主要包括拉裂和剪坏，这种破坏应力状态的存在不仅会使得计算结果不合理，也会降低有限元计算的稳定性。针对采用UH模型UMAT进行有限元计算时遇到的局部单元破坏问题，根据一定的假设条件，并结合不同坐标系下的应力变换关系，推导得到适用于UH模型的三维问题单元破坏修正公式；采用FORTRAN语言编写出相应的子程序，将其嵌入UH模型UMAT中，以消除采用UH模型进行有限元计算时出现的不合理的破坏应力状态，提高UH模型UMAT有限元计算的稳定性，并通过有限元模拟基坑开挖的例子验证本文所提的单元破坏修正方法的有效性和合理性。
Abstract:The problem of some elements' damage often appears when finite element calculation of using UH model UMAT is conducted, including tension failure and shear failure. The failure stress state not only makes the results unreasonable, but also reduces the stability of calculation. To solve the problem generated by unreasonable failure stress state when the UH model's UMAT is used to conduct finite element analysis, based on certain assumptions and combined with stress transformation relationship under different coordinates, three-dimensional element failure correction formulas for UH model can be elicited. Then FORTRAN language is used to write the subroutine of element failure correction, and it is embedded in the UH model's UMAT to eliminate the unreasonable failure stress state and improve the stability of finite element calculation. Finally, an example of foundation pit excavation is used to verify the validity and rationality of this method.
-
Key words:
- UH model /
- UMAT /
- finite element /
- tension failure /
- shear failure /
- failure correction
-
图 1 UH模型的当前屈服面与参考屈服面
Figure 1. Current yield surface and referential yield surface of UH model
图 12 有无破坏修正的土体的竖向位移等值云图
Figure 12. Vertical displacement contour of soils with and without failure correction
表 1 新旧坐标轴之间的方向余弦
Table 1. Direction cosine between new and old coordinate
坐标轴 x y z x′ l1 m1 n1 y′ l2 m2 n2 z′ l3 m3 n3 
下载: 导出CSV
表 2 UH模型参数取值
Table 2. Parameter value of UH model
参数 M ν κ λ e0 N c/kPa 数值 0.984 0.3 0.016 0.09 0.6 1.15 10
下载: 导出CSV
表 3 破坏修正前后计算结果对比
Table 3. Comparison of calculation results before and after failure correction
变量 1 244号单元第1积分点 18号单元第4积分点 修正前 修正后 修正前 修正后 
32.53 32.53 42.11 42.11 
7.39 23.97 5.07 23.93 
-14.16 21.44 -7.47 21.44 σx/kPa -11.16 3.51 -15.61 2.86 σy/kPa -35.600 0.007 -28.910 0 σz/kPa 8.19 10.10 19.92 20.30 τxz/kPa -8.03 -2.74 -5.24 -2.57 
4.714 0.387 3.373 0.670 
1.908 1.908 1.801 1.801 
-0.223 29.290 -0.444 10.830
下载: 导出CSV
-
[1] YAO Y P, HOU W, ZHOU A N.UH model:Three-dimensional unified hardening model for overconsolidated clays[J].Géotech-nique, 2009, 59(5):451-469. doi: 10.1680/geot.2007.00029 [2] 姚仰平, 侯伟, 罗汀.土的统一硬化模型[J].岩石力学与工程学报, 2009, 28(10):2135-2151. doi: 10.3321/j.issn:1000-6915.2009.10.023YAO Y P, HOU W, LUO T.Unified hardening model for soils[J].Chinese Journal of Rock Mechanics and Engineering, 2009, 28(10):2135-2151(in Chinese). doi: 10.3321/j.issn:1000-6915.2009.10.023 [3] 姚仰平, 李自强, 侯伟, 等.基于改进伏斯列夫线的超固结土本构模型[J].水利学报, 2008, 39(11):1244-1250. doi: 10.3321/j.issn:0559-9350.2008.11.013YAOY P, LI Z Q, HOU W, et al.Constitutive model of over-consolidated clay based on improved Hvorslev envelope[J].Journal of Hydraulic Engineering, 2008, 39(11):1244-1250(in Chinese). doi: 10.3321/j.issn:0559-9350.2008.11.013 [4] 甄文战. 岩土材料变形局部化问题理论及数值分析研究[D]. 上海: 上海大学, 2013: 24-31.ZHEN W Z.Theoretical and numberical simulations of deformation localization of geomaterials[D].Shanghai:Shanghai University, 2013:24-31(in Chinese). [5] 甄文战, 孙德安. 统一硬化参数模型在有限元中的应用及验证[C]//岩土力学与工程新进展. 上海: 同济大学出版社, 2007: 70-73.ZHEN W Z, SUN D A.Application and verification of unified hardening parameter model in finite element analysis[C]//New Development of Rock and Soil Mechanics and Engineering. Shanghai:Tongji University Press, 2007:70-73(in Chinese). [6] 姚仰平, 冯兴, 黄祥, 等. UH模型在有限元分析中的应用[J].岩土力学, 2010, 31(1):237-245. http://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201001042.htmYAO Y P, FENG X, HUANG X, et al.Application of UH model to finite element analysis[J].Rock and Soil Mechanics, 2010, 31(1):237-245(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-YTLX201001042.htm [7] 冯兴. UH模型的有限元实现及其在地基变形分析中的应用[D]. 北京: 北京航空航天大学, 2013: 38-80.FENG X.Finite element realization of UH model and its application to the analysis of subgrade deformation[D].Beijing:Beijing University of Aeronautics and Astronautics, 2013:38-80(in Chinese). [8] 罗汀, 秦振华, 姚仰平, 等.UH模型切线刚度矩阵对称化及其应用[J].力学学报, 2011, 43(6):1186-1190. doi: 10.6052/0459-1879-2011-6-lxxb2010-538LUO T, QIN Z H, YAO Y P, et al.Symmetrization and applications of tangent stiffness matrix for UH model[J].Chinese Journal of Theoretical and Applied Mechanics, 2011, 43(6):1186-1190(in Chinese). doi: 10.6052/0459-1879-2011-6-lxxb2010-538 [9] 熊文林.非关联塑性切线刚度矩阵的对称表示[J].应用数学和力学, 1986, 7(11):983-991. http://www.cnki.com.cn/Article/CJFDTOTAL-YYSX198611003.htmXIONG W L.Symmetric formulation of tangential stiffness for non-associated plasticity[J].Applied Mathematics and Mechanics, 1986, 7(11):983-991(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-YYSX198611003.htm [10] 罗汀, 姚仰平, 侯伟.土的本构关系[M].北京:人民交通出版社, 2010:170-179.LUO T, YAO Y P, HOU W.Soil constitutive models[M].Beijing:China Communication Press, 2010:170-179(in Chinese). [11] 王乃东, 姚仰平.基于变换应力方法的各向异性模型三维化[J].岩土工程学报, 2011, 33(1):50-56. http://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201101011.htmWANG N D, YAO Y P.Generalization of anisotropic constitutive models using transformedstress method[J].Chinese Journal of Geotechnical Engineering, 2011, 33(1):50-56(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-YTGC201101011.htm [12] MATSUOKA H, YAO Y P, SUN D A.The Cam-claymodels revised by the SMP criterion[J].Journal of the Japanese Geotechnical Society Soils & Foundation, 1999, 39(1):81-95. [13] 黄祥. 超固结土模型与ABAQUS软件结合与应用[D]. 北京: 北京航空航天大学, 2010: 17-24.HUANG X.The application of combining over-consolidated clay model of ABAQUS[D].Beijing:Beijing University of Aeronautics and Astronautics, 2010:17-24(in Chinese). [14] 罗汀, 张盼盼, 姚仰平, 等.考虑膨胀效应的UH模型及其有限元实现[J].北京航空航天大学学报, 2016, 42(1):13-20. http://bhxb.buaa.edu.cn/CN/abstract/abstract13667.shtmlLUO T, ZHANG P P, YAO Y P, et al.UH model considering the expansion of the soils and its finite element implementation[J].Journal of Beijing University of Aeronautics and Astronautics, 2016, 42(1):13-20(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13667.shtml [15] 钱家欢, 殷宗泽. 土工原理与计算[M]. 2版. 北京: 中国水利水电出版社, 1996: 91-92.QIAN J H, YIN Z Z.Principle and calculation of geotechnical engineering[M].2nd ed.Beijing:China Water & Power Press, 1996:91-92(in Chinese). [16] 杨光华, 李广信, 介玉新.土的本构模型的广义位势理论及其应用[M].北京:中国水利水电出版社, 2007:41-43.YANG G H, LI G X, JIE Y X.The generalized potential theory and its application of the constitutive model of soils[M].Beijing:China Water & Power Press, 2007:41-43(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 649
- HTML全文浏览量: 60
- PDF下载量: 520
- 被引次数: 0
