-
摘要:
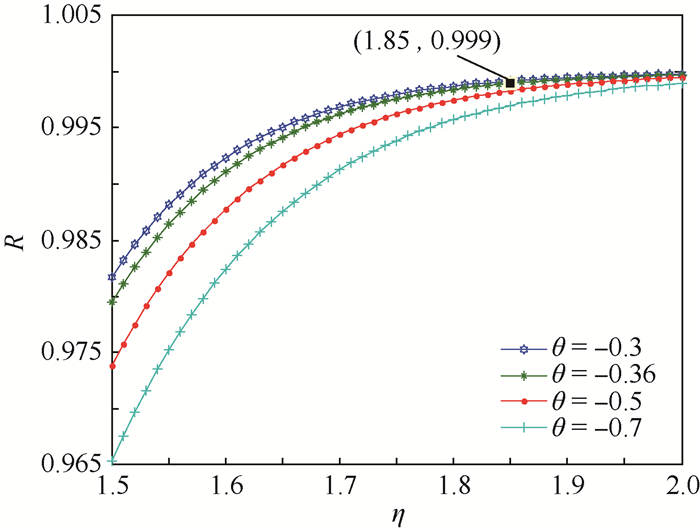
针对传统结构可靠性试验的验证多是基于载荷应力和结构强度相互独立的假设问题，从应力和强度数据的相关性分析与度量出发，在二者均为正态随机变量的前提下，建立了一种基于Copula函数相关应力-强度干涉模型的结构可靠性加严试验验证方案设计方法。该方法结合Copula函数和应力-强度干涉模型实现相关条件下原可靠性指标与加严条件下可靠性指标的转化，适用于小样本情况下基于传统成败型试验方法评估其可靠性。研究结果表明：相比独立假设，应力和强度呈负相关时，会增加试验样本量且样本量随负相关程度减弱而减少；呈正相关时，会减少试验样本量且样本量随正相关程度增强而减少。
Abstract:The traditional test verification of structural reliability is generally based on the independence assumption between loading stress and structural strength. Stating with the correlation analysis of stress and strength, we propose a hardened test method of structural reliability verification based on the stress-strength interference model with Copula correlation when loading and strength both follow the normal distribution. The method combines Copula functions with stress-strength interference model to achieve the transformation of the original reliability index and reliability index under the hardened condition, and the reliability is estimated by the traditional binomial distribution test under the condition of small sample. Compared with the independence assumption, the results show that the negative correlation between stress and strength leads to the increase of sample size and the sample size decreases with the decrease of negative correlation; the positive correlation leads to the decrease of sample size and the sample size decreases with the increase of positive correlation.
-
图 1 不同θ下可靠度-生存系数变化
Figure 1. Variation of reliability-survival coefficient with different θ
图 2 试验样本量与相关程度参数的关系
Figure 2. Relationship between test sample size and correlation coefficient
表 1 独立与相关情况下试验样本量的比较
Table 1. Comparison of test sample size between independent and correlative conditions
k RL=0.998 RL=0.996 RL=0.994 相关 独立 相关 独立 相关 独立 1.2 97 64 57 39 41 29 1.3 39 24 24 16 18 13 1.4 19 11 13 8 10 7 1.5 10 6 7 5 6 4 
下载: 导出CSV
-
[1] 温玉全, 洪东跑, 王玮.基于试验熵的火工品可靠性评估理论与方法研究[J].爆炸与冲击, 2007, 27(6):553-556. doi: 10.11883/1001-1455(2007)06-0553-04WEN Y Q, HONG D P, WANG W.Study on theory and method of reliability assessment of explosive initiator based on testing entropy[J].Explosion and Shock Waves, 2007, 27(6):553-556(in Chinese). doi: 10.11883/1001-1455(2007)06-0553-04 [2] 荣吉利, 白美, 刘志权.加严条件下火工机构可靠性评估方法[J].北京理工大学学报, 2004, 24(2):117-120. http://www.cnki.com.cn/Article/CJFDTOTAL-BJLG200402006.htmRONG J L, BAI M, LIU Z Q.Reliability assessment of pyrotechnical devices under rigorous conditions[J].Transactions of Beijing Institute of Technology, 2004, 24(2):117-120(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-BJLG200402006.htm [3] 刘智洋, 刘鲁, 黄敏.可靠性增长的单调约束模型[J].北京航空航天大学学报, 2009, 35(9):1104-1107. http://bhxb.buaa.edu.cn/CN/abstract/abstract8676.shtmlLIU Z Y, LIU L, HUANG M.Monotone restriction model of reliability growth evaluation[J].Journal of Beijing University of Aeronautics and Astronautics, 2009, 35(9):1104-1107(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract8676.shtml [4] SCHICK G J, DRNAS T M.Bayesian reliability dmonstration[M]//HENKE M, JAEGER A, WARTMANN R, et al.Proceedings in operations research.Heidelberg:Physica-Verlag HD, 1972:92-102. [5] 卓洛托夫А А, 季托夫М И. 空间运载器的可靠性保证[M]. 王廼斌, 译. 北京: 宇航出版社, 1996: 17-24.3ОЛОТОВ А А, ТИТОВ М И.Reliability ensures for the space vehicles[M].WANG N B, translated.Beijing:China Astronautic Publishing House, 1996:17-24(in Chinese). [6] 荣吉利, 张涛.航天火工机构可靠性的强化试验验证方法[J].宇航学报, 2013, 30(6):2426-2430. http://www.cnki.com.cn/Article/CJFDTOTAL-YHXB200906063.htmRONG J L, ZHANG T.Reliability validation on spacecraft pyrotechnical devices using the hardened test method[J].Journal of Astronautics, 2013, 30(6):2426-2430(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-YHXB200906063.htm [7] 马斌捷, 张俊华.已知强度和载荷变差系数的结构可靠性分析[J].机械强度, 1994, 16(1):1-6. http://www.cnki.com.cn/Article/CJFDTOTAL-JXQD401.000.htmMA B J, ZHANG J H.The structural reliability analysis for known variation coefficients of strength and load[J].Journal of Mechanical Strength, 1994, 16(1):1-6(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-JXQD401.000.htm [8] 荣吉利, 宋乾强.正态应力-正态强度下可靠度精确置信下限[J].兵工学报, 2015, 36(2):332-336. http://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201502021.htmRONG J L, SONG Q Q.The exact lower confidence limit of reliability for normal stress and normal strength[J].Acta Armamentarii, 2015, 36(2):332-336(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-BIGO201502021.htm [9] SUN Y, MA L, MORRIS J.A practical approach for reliability prediction of pipeline systems[J].European Journal of Operational Research, 2009, 198(1):210-214. doi: 10.1016/j.ejor.2008.07.040 [10] 唐家银, 何平, 陈崇双.相关性失效机械系统的可靠性分析方法[M].北京:国防工业出版社, 2014:15-48.TANG J Y, HE P, CHEN C S.The reliability analysis method for mechanical system with relational failure[M].Beijing:National Defence Industry Press, 2014:15-48(in Chinese). [11] 唐小松, 李典庆, 周创兵, 等.联合分布函数构造的Copula函数方法及结构可靠度分析[J].工程力学, 2013, 30(12):8-17. http://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201312004.htmTANG X S, LI D Q, ZHOU C B, et al.Modeling bivariate distribution using Copulas and its application to component reliability analysis[J].Engineering Mechanics, 2013, 30(12):8-17 (in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201312004.htm [12] WU X Z.Modelling dependence structures of soil shear strength data with bivariate copulas and applications to geotechnical reliability analysis[J].Soils and Foundations, 2015, 55(5):1243-1258. doi: 10.1016/j.sandf.2015.09.023 [13] MICHIELS F, DE SCHEPPER A.A new graphical tool for copula selection[J].Journal of Computational and Graphical Statistics, 2013, 22(2):471-493. doi: 10.1080/10618600.2012.672080 [14] NELSEN R B.An introduction to copulas[M].Berlin:Springer, 2010:157-222. [15] 王鹏, 杜志明.变加严系数的加严试验方法[J].质量与可靠性, 2007, 130(4):24-28. http://www.cnki.com.cn/Article/CJFDTOTAL-ZNYZ200704012.htmWANG P, DU Z M.The hardened test method considering the changing hardened cofficient[J].Quality and Reliability, 2007, 130(4):24-28(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-ZNYZ200704012.htm -
下载:
点击查看大图
计量
- 文章访问数: 922
- HTML全文浏览量: 104
- PDF下载量: 475
- 被引次数: 0
