-
摘要:
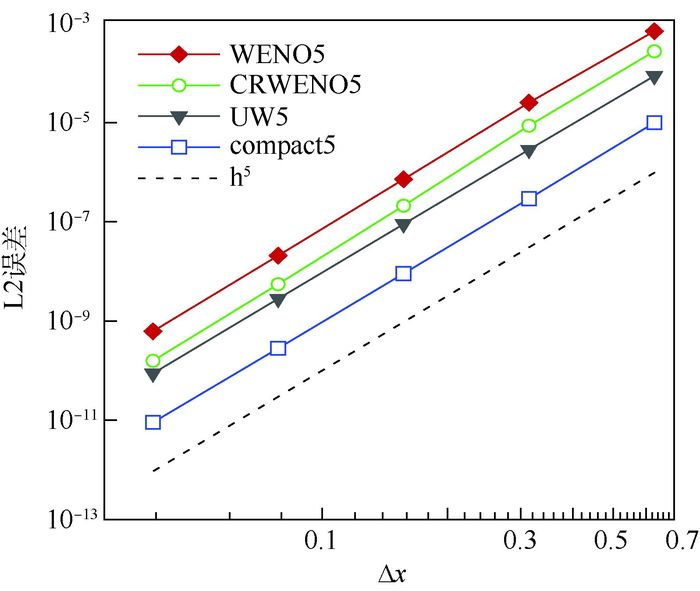
针对可压缩多尺度流动数值模拟特点，研究一种五阶高分辨率紧致型激波捕捉格式——紧致重构加权基本无振荡（CRWENO）格式。该格式利用非线性权系数将低阶紧致格式加权组合以达到高阶精度。在光滑区域蜕化成具有高分辨率的五阶线性紧致格式，在间断附近则能保持计算稳定无振荡。对CRWENO格式、目前广泛使用的加权基本无振荡（WENO）格式及两格式对应的线性格式（即五阶线性迎风格式和五阶紧致格式）进行数值性能研究，评估非线性权系数对格式耗散及频谱特性的影响。使用一维、二维、三维典型算例进行数值试验，探讨线性/非线性、紧致/非紧致格式在可压缩多尺度流动模拟中的优势和不足。结果表明，CRWENO格式在强压缩性流场模拟中能够稳定地捕捉激波，其紧致特性则改善了非线性格式普遍存在的耗散过大、分辨率较差的问题，使其能够清晰捕捉多尺度流动结构。因此，该格式在可压缩多尺度流动模拟中具有较大优势。
Abstract:Aimed at compressible multiscale flow simulations, a fifth-order high-resolution compact shock capturing scheme, compact-reconstruction weighted essentially non-oscillatory (CRWENO), is studied. Nonlinear weights are used to combine lower-order compact schemes to approximate a higher-order compact scheme. The scheme becomes the fifth-order linear compact scheme in smooth regions, while preserves computational stability across discontinuities. Numerical properties were analyzed for CRWENO and weighted essentially non-oscillatory (WENO) which is widely used these days, as well as the linear schemes that they correspond to, i.e. the fifth-order upwind linear scheme and the fifth-order compact scheme. The impacts of nonlinear weights on dissipation and spectral properties are evaluated. The advancements and drawbacks of linear/nonlinear and compact/explicit schemes in compressible multiscale flow simulations are discussed by performing 1D, 2D and 3D typical numerical tests. It can be concluded that CRWENO scheme can obtain non-oscillatory results near strong discontinuous regions. Its compact characteristic improves the problems of over-dissipation and low resolution exiting in nonlinear schemes and makes it clearly resolve multiscale flow structures. In a word, CRWENO is a proper candidate for compressible multiscale flow simulations.
-
图 3 Sod激波管不同格式的密度分布曲线
Figure 3. Density distribution curves of Sod shock tube with different schemes
图 4 Δx=1/320时，前向台阶密度等值线图
Figure 4. Density contours at forward facing step at Δx=1/320
图 5 泰勒-格林涡平均动能及其动能耗散率随时间变化曲线
Figure 5. Variation of kinetic energy and kinetic energy dissipation rate of Taylor-Green vortex with time
图 6 t=9时泰勒-格林涡能谱分布曲线
Figure 6. Energy spectrum distribution curves of Taylor-Green vortex at t=9
图 7 不同时刻动能与初始动能之比及拟涡能与初始拟涡能之比随时间变化曲线
Figure 7. Variation curves of ratio of kinetic energy to initial kinetic energy and ratio of enstrophy to initial enstrophy at different moments with time
图 8 2种格式在t/τ=4时刻的能谱分布曲线
Figure 8. Energy spectrum distribution curves of two schemes at t/τ=4
-
[1] 屈峰, 阎超, 于剑, 等.高精度激波捕捉格式的性能分析[J].北京航空航天大学学报, 2014, 40(8):1085-1089. http://bhxb.buaa.edu.cn/CN/abstract/abstract13000.shtmlQU F, YAN C, YU J, et al.Assessment of shock capturing methods for numerical simulations of compressible turbulence with shock waves[J].Journal of Beingjing University of Aeronautics and Astronautics, 2014, 40(8):1085-1089(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13000.shtml [2] LELE S K.Compact finite difference schemes with spectral-like resolution[J].Journal of Computational Physics, 1992, 103(1):16-42. doi: 10.1016/0021-9991(92)90324-R [3] VAN LEER B.Towards the ultimate conservation difference scheme Ⅴ:A second-order sequal to Godunov's method[J].Journal of Computational Physics, 1979, 32(1):101-136. doi: 10.1016/0021-9991(79)90145-1 [4] HARTEN A.High resolution schemes for hyperbolic conservation laws[J].Journal of Computational Physics, 1983, 49(3):357-393. doi: 10.1016/0021-9991(83)90136-5 [5] JIANG G S, SHU C W.Efficient Implementation of weighted ENO schemes[J].Journal of Computational Physics, 1996, 126(1):202-228. doi: 10.1006/jcph.1996.0130 [6] GEROLYMOS G A, SÉNÉCHAL D, VALLET I.Very-high-order WENO schemes[J].Journal of Computational Physics, 2009, 228(23):8481-8524. doi: 10.1016/j.jcp.2009.07.039 [7] MARTIN M P, TAYLOR E M, WU M, et al.A bandwidth-optimized WENO scheme for the effective direct numerical simulation of compressible turbulence[J].Journal of Computational Physics, 2006, 220(1):270-289. doi: 10.1016/j.jcp.2006.05.009 [8] HENRICK A K, ASLAM T D, POWERS J M.Mapped weighted essentially non-oscillatory schemes:Achieving optimal order near critical points[J].Journal of Computational Physics, 2005, 207(2):542-567. doi: 10.1016/j.jcp.2005.01.023 [9] ACKER F, BORGES R B D R, COSTA B.An improved WENO-Z scheme[J].Journal of Computational Physics, 2016, 313:726-753. doi: 10.1016/j.jcp.2016.01.038 [10] GHOSH D, BAEDER J.Compact reconstruction schemes with weighted ENO limiting for hyperbolic conservation laws[J].SIAM Journal on Scientific Computing, 2012, 34(3):A1678-A1706. doi: 10.1137/110857659 [11] GHOSH D, MEDIDA S, BAEDER J D.Application of compact-reconstruction weighted essentially nonoscillatory schemes to compressible aerodynamic flows[J].AIAA Journal, 2014, 52(9):1858-1870. doi: 10.2514/1.J052654 [12] GHOSH D, CONSTANTINESCU E M, BROWN J.Efficient implementation of nonlinear compact schemes on massively parallel platforms[J].SIAM Journal on Scientific Computing, 2015, 37(3):C354-C383. doi: 10.1137/140989261 [13] PENG J, SHEN Y.Improvement of weighted compact scheme with multi-step strategy for supersonic compressible flow[J].Computers & Fluids, 2015, 115:243-255. http://dspace.imech.ac.cn/bitstream/311007/58426/1/J2015-065.pdf [14] ROE P L.Approximate Riemann solvers, parameter vectors and difference schemes[J].Journal of Computational Physics, 1981, 43(2):357-372. doi: 10.1016/0021-9991(81)90128-5 [15] GOTTLIEB S, SHU C.Total variation diminishing Runge-Kutta schemes[J].Mathematics of computation of the American Mathematical Society, 1998, 67(221):73-85. doi: 10.1090/mcom/1998-67-221 [16] 傅德薰, 马延文, 李新亮, 等.可压缩湍流直接数值模拟[M].北京:科学出版社, 2010:34-36.FU D X, MA Y W, LI X L, et al.Direct numerical simulation of the compressible turbulences[M].Beijing:Science Press, 2010:34-36(in Chinese). [17] FAUCONNIER D, DICK E.On the spectral and conservation properties of nonlinear discretization operators[J].Journal of Computational Physics, 2011, 230(12):4488-4518. doi: 10.1016/j.jcp.2011.02.025 [18] SOD G A.A survey of several finite difference methods for systems of nonlinear hyperbolic conservation laws[J].Journal of Computational Physics, 1978, 27(1):1-31. doi: 10.1016/0021-9991(78)90023-2 [19] WOODWARD P, COLELLA P.The numerical simulation of two-dimensional fluid flow with strong shocks[J].Journal of Computational Physics, 1984, 54(1):115-173. doi: 10.1016/0021-9991(84)90142-6 [20] DEBONIS J R.Solutions of the Taylor-Green vortex problem using high resolution explicit finite difference methods:AIAA-2013-0382[R].Reston:AIAA, 2013. [21] BULL J R, JAMESON A.Simulation of the compressible Taylor-Green vortex using high-order flux reconstruction schemes[J].AIAA Journal, 2015, 53(9):2750-2761. doi: 10.2514/1.J053766 [22] DE WIART C C, HILLEWAERT K, DUPONCHEEL M, et al.Assessment of a discontinuous Galerkin method for the simulation of vortical flows at high Reynolds number[J].International Journal for Numerical Methods in Fluids, 2014, 74(7):469-493. doi: 10.1002/fld.v74.7 [23] SAMTANEY R, PULLIN D I, KOSOVIC B.Direct numerical simulation of decaying compressible turbulence and shocklet statistics[J].Physics of Fluids, 2001, 13(5):1415-1430. doi: 10.1063/1.1355682 -
下载:
点击查看大图
计量
- 文章访问数: 801
- HTML全文浏览量: 189
- PDF下载量: 556
- 被引次数: 0
