-
摘要:
针对磁性目标跟踪问题，以磁偶极子等效场源模型为基础，建立磁性目标跟踪的离散状态空间模型，将磁偶极子目标实时跟踪问题转化为状态空间模型的滤波估值问题。针对磁性目标初始条件难以获得且现有卡尔曼类滤波算法在大初始误差条件下容易出现发散的问题，提出一种递推观测更新的卡尔曼滤波算法，将现有的一步观测更新描述为递推更新过程，等效降低大初始误差带来的大非线性误差。仿真与实测数据测试结果表明，本文算法具有良好的精度和收敛性，能够有效抑制磁偶极子跟踪中由于大初始误差导致的滤波发散，适于实际应用。
Abstract:The magnetic target tracking problem is addressed in this paper by establishing the discrete state-space model on the basis of the equivalent magnetic dipole model in order to formulate the real-time magnetic dipole target tracking problem as filtering estimation problem of state-space model. Then a novel filtering approach with the recursive update process is proposed to address the divergence problem of magnetic target tracking under large prior error condition when using present Kalman-type filters. The one-step measurement update is replaced by recursive update process; hence the large nonlinearized error caused by large prior error is reduced in each recursive step. The proposed algorithm is tested by simulation and real-world magnetic data. Both results validate the superior performance in comparison with present filters in terms of accuracy and convergence, and the capacity to suppress the divergence problem caused by large prior error in magnetic dipole target tracking.
-
Key words:
- magnetic dipole /
- tracking /
- nonlinear filtering /
- linearization /
- Kalman filter
-
图 1 Ψ=π/3时位置和磁矩分量RMSE
Figure 1. RMSE of position and magnetic moment component at Ψ=π/3
图 2 Ψ=π/8时位置和磁矩分量RMSE
Figure 2. RMSE of position and magnetic moment component at Ψ=π/8
图 3 Ψ=π/16时位置和磁矩分量RMSE
Figure 3. RMSE of position and magnetic moment component at Ψ=π/16
图 6 不同初值条件下各算法对实测数据的跟踪处理结果
Figure 6. Real-world data tracking and processing results by each algorithm under different initializing conditions
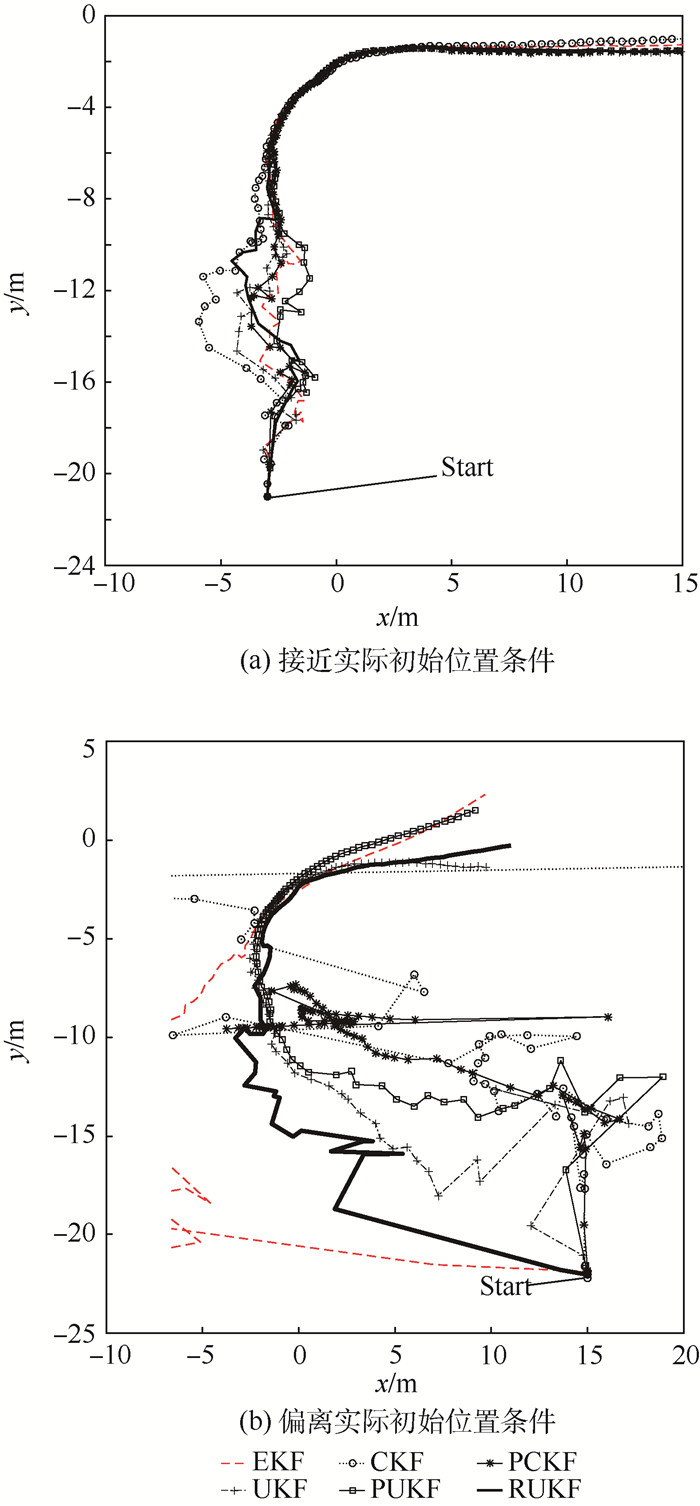
表 1 各算法在不同初值条件下的归一化后验残差平方和
Table 1. Normalized posterior residual square sum for each algorithm under different initializing conditions
算法 r* 偏离实际初始位置 接近实际初始位置 EKF 9.363×104 9.285×104 UKF 1.179×105 9.635×104 CKF 9.453×105 1.623×107 PUKF 4.722×105 3.725×103 PCKF 1.504×105 2.341×106 RUKF 8.356×104 1.538×103 
下载: 导出CSV
-
[1] MCAULAY A.Computerized model demonstrating magnetic submarine localization[J].IEEE Transaction on Aerospace and Electronic System, 1977, 13(3):246-254. http://ieeexplore.ieee.org/xpls/abs_all.jsp?arnumber=4101801 [2] MCFEE E, DAS Y, ELLINGSON O.Locating and identifying compact ferrous objects[J].IEEE Transactions on Geosciences and Remote Sensing, 1990, 28(2):182-193. doi: 10.1109/36.46697 [3] PHAM D, AZIZ S.A real-time localization system for an endoscopic capsule using magnetic sensors[J].Sensors, 2014, 14(1):20910-20929. http://ieeexplore.ieee.org/xpls/abs_all.jsp?arnumber=6827653 [4] NARA T, SUZUKI S, ANDO S, et al.A closed-form formula for magnetic dipole localization by measurement of its magnetic field and spatial gradients[J].IEEE Transaction on Magnetic, 2006, 42(10):3291-3293. doi: 10.1109/TMAG.2006.879151 [5] 王金根, 龚沈光.基于运动标量磁强计的磁性目标定位问题研究[J].电子学报, 2002, 30(7):1057-1060. http://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200207032.htmWANG J G, GONG S G.Research on the problem of localizing magnetic target based on motion scalar magnetometer[J].Acta Electronica Sinica, 2002, 30(7):1057-1060(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-DZXU200207032.htm [6] BIRSAN M.Recursive Bayesian method for magnetic dipole tracking with a tensor gradiometer[J].IEEE Transactions on Magnetics, 2011, 47(2):409-415. doi: 10.1109/TMAG.2010.2091964 [7] WAHLSTROM N.Target tracking using Maxwell's equations[D].Linkoping:Linkoping University, 2010. [8] BIRSAN M.Recursive Bayesian method for magnetic dipole tracking with a tensor gradiometer[J].IEEE Transactions on Magnetic, 2011, 47(2):409-415. doi: 10.1109/TMAG.2010.2091964 [9] DOUCET A, GODSILL S, ANDRIEU S, et al.On sequential Monte Carlo sampling methods for Bayesian filtering[J].Statistics and Computing, 2000, 10(3):197-208. doi: 10.1023/A:1008935410038 [10] KOTECHA J H, DJURIC P M.Gaussian particle filtering[J].IEEE Transactions on Signal Processing, 2003, 51(10):259-260. [11] RUDOLPH M, DOUCET A, NANDO F.The unscented particle filter[R].Cambridge:Cambridge University Engineering Department, 2000. [12] JAZWINSKI A.Stochastic process and filtering theory[M].New York:Academic Press, 1970. [13] SIMON J, JEFFERY U, HUGH D.A new method for the nonlinear transformation of means and covariances in filters and estimators[J].IEEE Transactions on Automatic Control, 2000, 45(3):477-481. doi: 10.1109/9.847726 [14] ARASARATNAM I, HAYKIN S.Cubature Kalman filter[J].IEEE Transactions on Automatic Control, 2009, 56(6):1254-1269. [15] MORELANDE M R, GARCIA-FERNANDEZ A F.Analysis of Kalman filter approximations for nonlinear measurements[J].IEEE Transacions on Signal Process, 2013, 61(12):5477-5484. http://adsabs.harvard.edu/abs/2013ITSP...61.5477M [16] HUANG Y L, ZHANG Y G, LI N, et al.Gaussian approximate filter with progressive measurement update[C]//Proceedings of 54th IEEE Conference on Decision and Control.Piscataway, NJ:IEEE Press, 2015:4344-4349. -
下载:
点击查看大图
计量
- 文章访问数: 756
- HTML全文浏览量: 110
- PDF下载量: 424
- 被引次数: 0
