-
摘要:
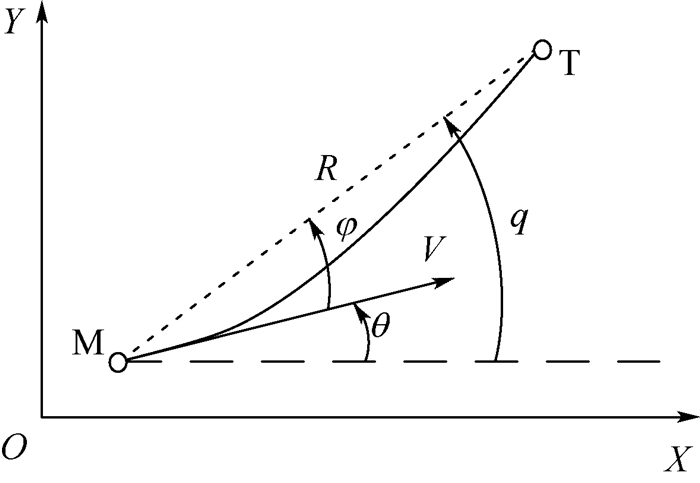
将分段线性逼近与迭代求解的思想扩展到对导弹的时变速度进行分段预测,给出了适用于反舰导弹速度时变情况的大前置角下比例导引律和偏置比例导引律的剩余时间估计算法。该算法在现有分段迭代算法的基础上,依据闭环形式的反舰导弹速度微分方程,分转弯平飞段和近似直线飞行段2种情况,对导弹未来速度的大小进行分段迭代预测并对剩余时间估计进行修正。算法中还给出了偏置比例导引律作用下近似直线飞行段剩余飞行航程的估计公式。仿真结果验证了本文算法的有效性。
Abstract:The idea of piecewise linear approximation and piecewise iterations is extended to the anti-ship missile's piecewise velocity prediction. Time-to-go estimation algorithms suitable for anti-ship missiles with time varying velocity are designed for proportional navigation guidance law and a biased proportional navigation guidance law with impact angle control both in the case of large lead angle. The proposed time-to-go estimation algorithms, which are based on the anti-ship missiles' differential equation of velocity in closed form and the current piecewise-iterative time-to-go estimation algorithms for the above mentioned guidance laws, perform piecewise-iterative prediction to the future velocity of anti-ship missiles for two flight cases: one for turning flight on level, the other for nearly straight flight on level, and then make corrections to the current time-to-go estimation algorithms. A range-to-go estimation formula is also given for the biased proportional navigation guidance law with impact angle control in the case of nearly straight flight on level. Numerical simulations are provided to illustrate the effectiveness of the proposed algorithm.
-
[1] JEON I S, LEE J I, TAHK M J.Impact-time-control guidance law for anti-ship missiles[J].IEEE Transactions on Control Systems Technology, 2006, 14(2):260-266. doi: 10.1109/TCST.2005.863655 [2] JEON I S, LEE J I, TAHK M J.Homing guidance law for cooperative attack of multiple missiles[J].Journal of Guidance, Control, and Dynamics, 2010, 33(1):275-280. doi: 10.2514/1.40136 [3] 李新三, 汪立新, 王明建, 等.基于MPSC和CPN制导方法的协同制导律[J].北京航空航天大学学报, 2016, 42(9):1857-1863. http://bhxb.buaa.edu.cn/CN/abstract/abstract13731.shtmlLI X S, WANG L X, WANG M J, et al.Cooperative guidance law based on MPSC and CPN guidance method[J]. Journal of Beijing University of Aeronautics and Astronautics, 2016, 42(9):1857-1863(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13731.shtml [4] 孙雪娇, 周锐, 吴江, 等.多导弹分布式协同制导与控制方法[J].北京航空航天大学学报, 2014, 40(1):120-124. http://bhxb.buaa.edu.cn/CN/abstract/abstract12834.shtmlSUN X J, ZHOU R, WU J, et al. Distributed cooperative guidance and control for multiple missiles[J]. Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(1):120-124(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract12834.shtml [5] 赵启伦, 陈建, 董希旺, 等.拦截高超声速目标的异类导弹协同制导律[J].航空学报, 2016, 37(3):936-948. http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201603020.htmZHAO Q L, CHEN J, DONG X W, et al. Cooperative guidance law for heterogeneous missiles intercepting hypersonic weapon[J]. Acta Aeronautica et Astronautica Sinica, 2016, 37(3):936-948(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-HKXB201603020.htm [6] TAHK M J, RYOO C K, CHO H J. Recursive time-to-go estimation for homing guidance missiles[J].IEEE Transactions on Aerospace and Electronic Systems, 2002, 38(1):13-24. doi: 10.1109/7.993225 [7] LAM V C. Time-to-go estimate for missile guidance:AIAA-2005-6459[R].Reston:AIAA, 2005. [8] RYOO C K, CHO H J, TAHK M J.Time-to-go weighted optimal guidance with impact angle constraints[J]. IEEE Transactions on Control Systems Technology, 2006, 14(3):483-492. doi: 10.1109/TCST.2006.872525 [9] WHANG I H, RA W S.Time-to-go estimation filter for anti-ship missile application[C]//SICE Annual Conference. Piscataway, NJ:IEEE Press, 2008:247-250. [10] WHANG I H, RA W S.Time-to-go estimator for missiles guided by BPNG[C]//International Conference on Control, Automation and Systems.Piscataway, NJ:IEEE Press, 2008:463-467. [11] SHIN H S, CHO H S, TSOURDOS A.Time-to-go estimation using guidance command history[C]//Proceedings of the 18th IFAC World Congress.Laxenburg:IFAC Secretariat, 2011:5531-5536. [12] CHO H J, RYOO C K.Implementation of optimal guidance laws using predicted missile velocity profiles[J].Journal of Guidance, Control, and Dynamics, 1999, 22(4):579-588. doi: 10.2514/2.4420 [13] 李辕, 赵继广, 白国玉, 等.基于预测碰撞点的剩余飞行时间估计方法[J].北京航空航天大学学报, 2016, 42(8):1667-1674. http://bhxb.buaa.edu.cn/CN/abstract/abstract13404.shtmlLI Y, ZHAO J G, BAI G Y, et al. Method of time-to-go estimation based on predicted crack point[J]. Journal of Beijing University of Aeronautics and Astronautics, 2016, 42(8):1667-1674(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13404.shtml [14] 张友安, 马国欣.大前置角下比例导引律的剩余时间估计算法[J].哈尔滨工程大学学报, 2013, 34(11):1409-1414. http://youxian.cnki.com.cn/yxdetail.aspx?filename=BJHK20170111001&dbname=CAPJ2015ZHANG Y A, MA G X. Time-to-go estimation algorithm for the proportional navigation guidance law with a large lead angle[J].Journal of Harbin Engineering University, 2013, 34(11):1409-1414(in Chinese). http://youxian.cnki.com.cn/yxdetail.aspx?filename=BJHK20170111001&dbname=CAPJ2015 [15] ZHANG Y A, MA G X, WU H L. A biased proportional navigation guidance law with large impact angle constraint and the time-to-go estimation[J]. Proceedings of the Institution of Mechanical Engineers, Part G:Journal of Aerospace Engineering, 2014, 228(10):1725-1734. doi: 10.1177/0954410013513754 -







 下载:
下载: