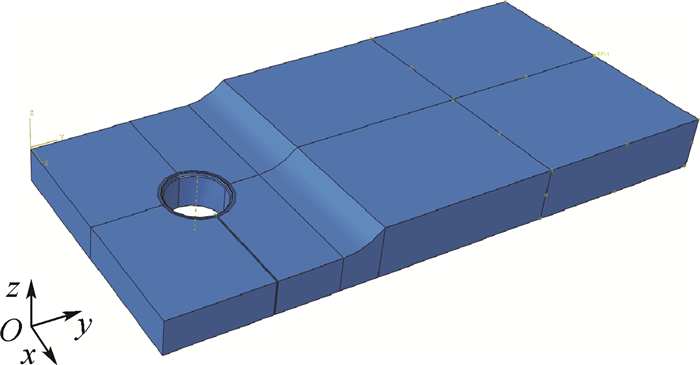
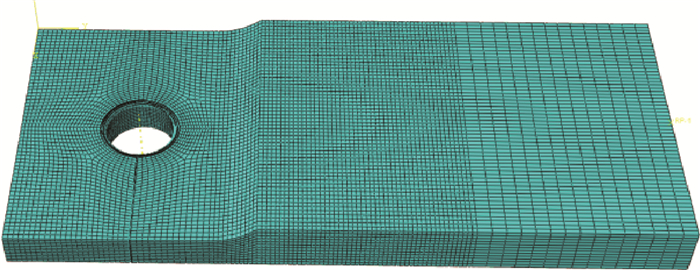
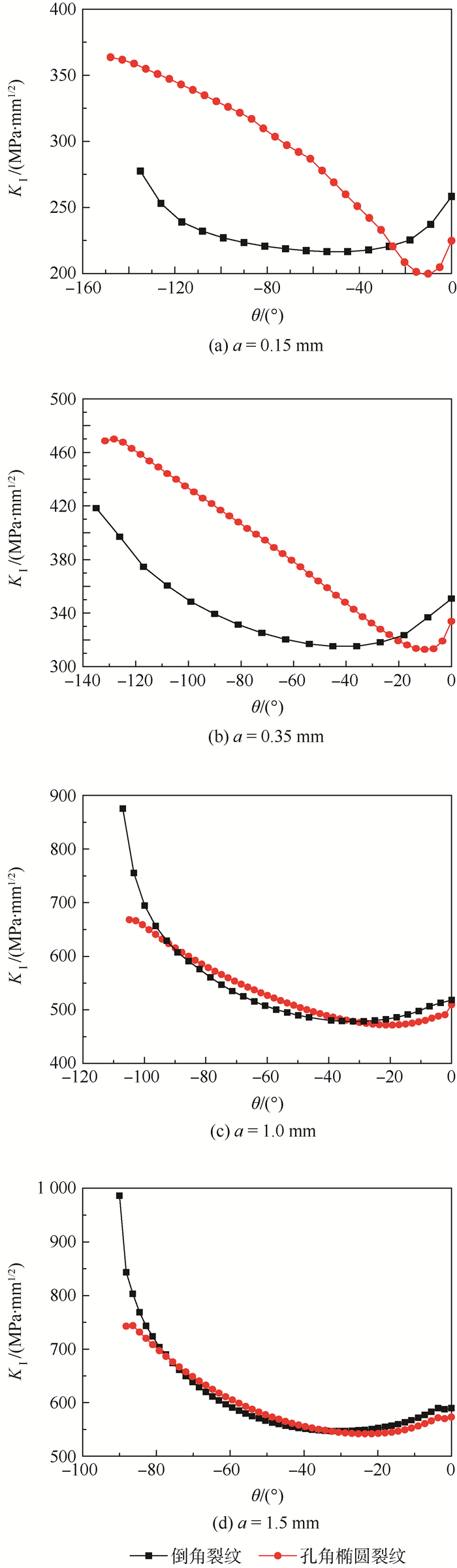
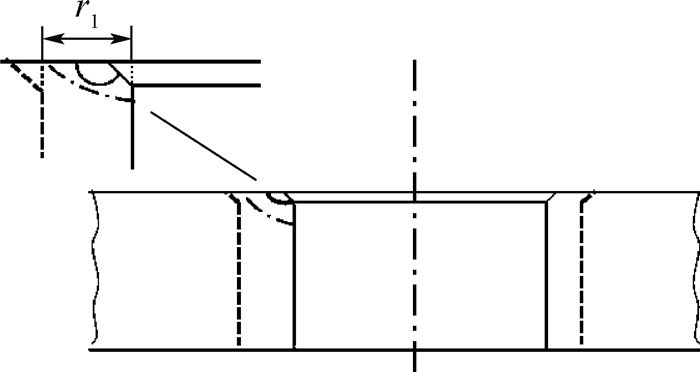
Determination method of equivalent initial flaw size for crack initiated at hole chamfering
-
摘要:
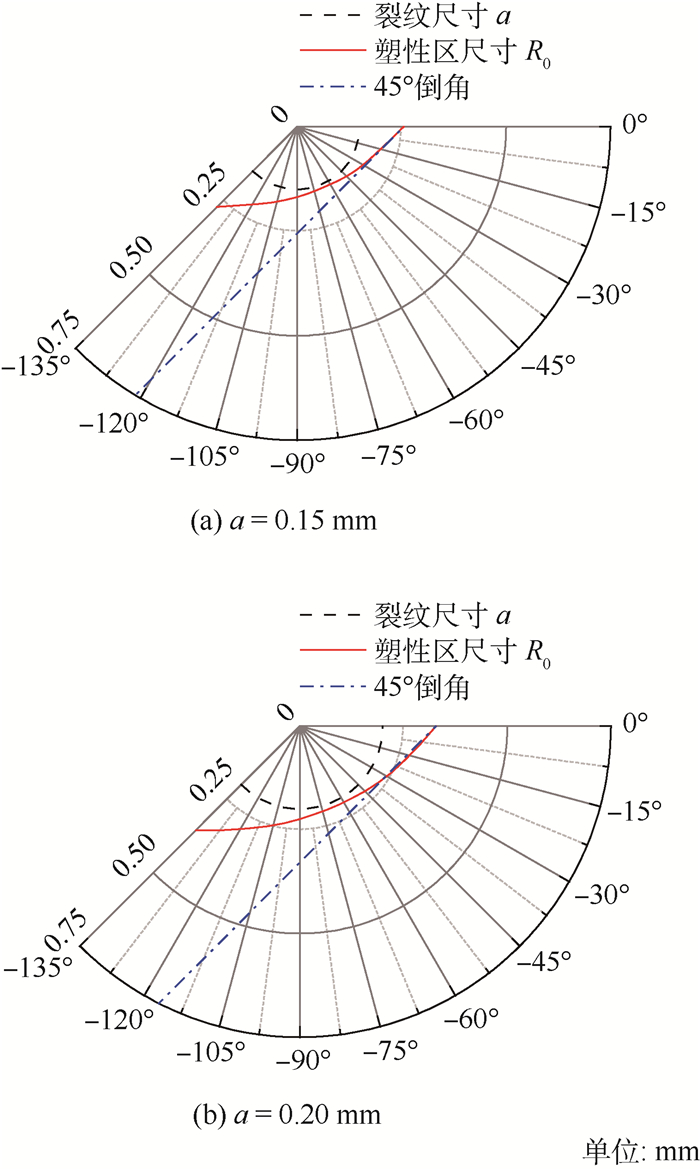
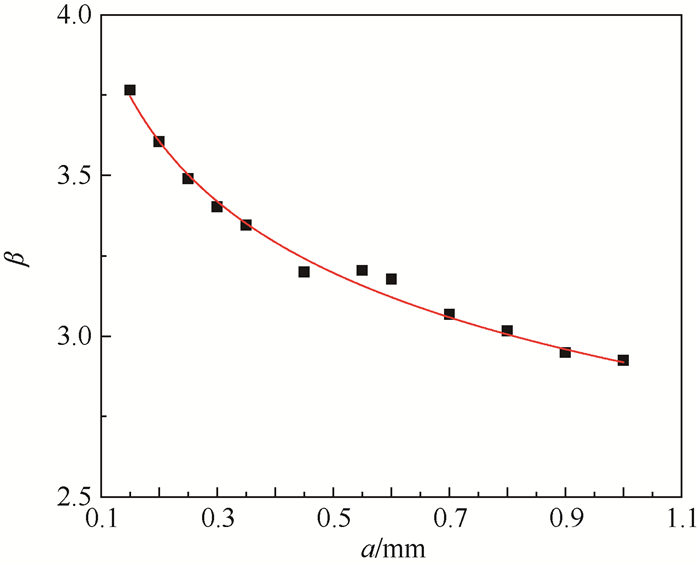
孔边倒角裂纹是含孔下陷细节的常见裂纹形态，为进行含孔下陷细节的经济寿命评定，需要确定倒角裂纹的原始疲劳质量（IFQ）。首先，为探究倒角对裂纹前缘应力强度因子的影响，进行了有、无倒角2种情况下应力强度因子的有限元分析。计算表明倒角对相对小裂纹的应力强度因子影响显著。其次，为合理表征该种裂纹的IFQ，将初始缺陷当量为萌生于倒角和试件表面交点，前缘为圆弧的初始裂纹，以萌生点到裂纹前缘沿孔径向的距离作为裂纹特征尺寸。最后，采用相对小裂纹扩展方程描述倒角裂纹的扩展规律，反推得到倒角裂纹的当量初始缺陷尺寸（EIFS）分布。统计分析表明，采用本文定义的裂纹特征尺寸得到的EIFS分布参数与应力水平无关。
-
关键词:
- 原始疲劳质量(IFQ) /
- 应力强度因子 /
- 有限元 /
- 裂纹扩展方程 /
- 当量初始缺陷尺寸(EIFS)
Abstract:Cracks often initiate at the chamfering for sagging holes. The initial fatigue quality (IFQ) of this type of crack needs to be determined prior to economic life assessment. Firstly, finite element models with and without chamfering are developed so as to investigate the effect of chamfering on the stress intensity factors at the crack front. The results show that the chamfering imposes great influence on the stress intensity factors of relatively small crack. Secondly, in order to characterize the IFQ of sagging holes, a circular-front crack initiated at the intersection of the chamfering and specimen surface is taken as the initial flaw, with the radial distance of crack front from the initiation site as the crack size. Finally, the crack growth equation for relatively small cracks is employed to characterize the crack growth behavior, and the equivalent initial flaw size (EIFS) distribution is obtained through back-extrapolation. Statistical analyses show that the EIFS distribution parameters obtained by using the proposed crack size definition are independent of stress levels.
-
图 3 有无倒角下的2种裂纹形态
Figure 3. Shape of two types of cracks initiated at holes with and without chamfering
图 7 2种裂纹形态下应力强度因子的对比
Figure 7. Comparison of stress intensity factors of two types of cracks
图 13 无量纲化应力强度因子随裂纹尺寸变化曲线
Figure 13. Changing curve of non-dimensional stress intensity factor with crack size
表 1 7B04-T74铝合金力学性能
Table 1. Mechanical properties of 7B04-T74 aluminum alloy
参数 弹性模量
E/GPa泊松比
ν屈服强度
σs/MPa抗拉强度
σb/MPa数值 70 0.33 450 518 
下载: 导出CSV
表 2 2种应力水平下Z分布参数估计
Table 2. Z distribution parameter estimation for two stress levels
应力水平 
应力水平1 0.806 0.250 应力水平2 1.043 0.443
下载: 导出CSV
-
[1] TONG Y C.Literature review on aircraft structural risk and reliability analysis:DSTO-TR-1110[R].Melboure:DSTO Aeronautical and Maritime Research Labratory, 2001. [2] RUDD J L, GRAY T D.Quantification of fastener-hole quality[J].Journal of Aircraft, 1978, 15(3):143-147. doi: 10.2514/3.58332 [3] RUDD J L.Applications of the equivalent initial quality method:AFFDL-TM-76-83-FBE[R].Dayton:Wright-Patterson AFB, 1977. [4] MANNING S D, YANG J N, SHINOZUKA M.USAF durability design handbook:Guidelines for the analysis and design of durable aircraft structures:AFFDL-TR84-3027[R].Dayton:Wright-Patterson AFB, 1984. [5] YANG J N.Statistical estimation of economic life for aircraft structures[J].Journal of Aircraft, 1980, 17(7):528-535. doi: 10.2514/3.57935 [6] BARTER S A, MOLENT L.Fatigue cracking from a corrosion pit in an aircraft bulkhead[J].Engineering Failure Analysis, 2014, 39(4):155-163. https://www.sciencedirect.com/science/article/pii/S1350630714000326 [7] MOLENT L.A review of equivalent pre-crack sizes in aluminium alloy 7050-T7451[J].Fatigue & Fracture of Engineering Materials & Structures, 2014, 37(10):1055-1074. https://www.sciencedirect.com/science/article/pii/S001379441400280X [8] MOLENT L, SUN Q, GREEEN A J.Characterisation of equivalent initial flaw sizes in 7050 aluminium alloy[J].Fatigue & Fracture of Engineering Materials & Structures, 2006, 29(11):916-937. https://www.researchgate.net/publication/229546175_Characterisation_of_equivalent_initial_flaw_sizes_in_7050_aluminium_alloy [9] MATTRAND C, BOURINET J M, THÉRET D.Analysis of fatigue crack growth under random load sequences derived from military in-flight load data[C]//ICAF 2011 Structural Integrity:Inuence of Eciency and Green Imper-atives.Berlin:Springer-Verlag, 2011:399-413. [10] MATTRAND C, BOURINET J M.Random load sequences and stochastic crack growth based on measured load data[J].Engineering Fracture Mechanics, 2011, 78(17):3030-3048. doi: 10.1016/j.engfracmech.2011.08.022 [11] XIANG Y, LIU Y. EIFS-based crack growth fatigue life prediction of pitting-corroded test specimens[J].Engineering Fracture Mechanics, 2010, 77(8):1314-1324. doi: 10.1016/j.engfracmech.2010.03.018 [12] FAWAZ S A.Equivalent initial flaw size testing and analysis of transport aircraft skin splices[J].Fatigue & Fracture of Engineering Materials & Structures, 2003, 26(3):279-290. https://www.researchgate.net/publication/293333775_Equivalent_initial_flaw_size_testing_and_analysis_of_transport_aircraft_skin_splices [13] WANG D Y.An investigation of initial fatigue quality:STPZ8860S[R].West Conshohocken:ASTM Special Technical Publication, 1982. [14] 航空航天部AFFD系统办公室. 美国空军耐久性手册背景材料: 第Ⅷ卷[M]西安: 航空航天部AFFD系统办公室, 1989.AFFD System Engineering Office of the Department of Aeronautics and Astronautics. The background information of USAF durability design handbook:Vol.Ⅷ[M].Xi'an:AFFD System Engineering Office of the Department of Aeronautics and Astronautics, 1989(in Chinese). [15] YANG J, MANNING S, RUDD J, et al.Effect of specimen size and notch geometry on equivalent initial flaw size distribution[C]//AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference and Exhibit.Reston:AIAA, 1996:385-397. [16] 曹淑森, 贺小帆, 杨博霄, 等.夹持边界条件下表面裂纹应力强度因子求解[J].北京航空航天大学学报, 2014, 40(11):1637-1642. http://bhxb.buaa.edu.cn/CN/abstract/abstract13088.shtmlCAO S S, HE X F, YANG B X, et al.Solution of stress intensity factor of surface cracked geometry with clamped ends[J].Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(11):1637-1642(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13088.shtml [17] 张行.断裂力学[M].北京:中国宇航出版社, 1990:72-169.ZHANG X.Fracture mechanics[M].Beijing:China Aerospace Press, 1990:72-169(in Chinese). [18] 刘文珽.概率断裂力学与概率损伤容限/耐久性[M].北京:北京航空航天大学出版社, 1999:144-181.LIU W T.Probabilistic fracture mechanics and probability damage tolerance/durability[M]. Beijing:Beihang University Press, 1999:144-181(in Chinese). [19] PROVAN J W.Probabilistic fracture mechanics and reliability[M]. Leiden:Martinus Nijhoff Publishers, 1987:70-72. [20] 高镇同.疲劳应用统计学[M].北京:国防工业出版社, 1986:277-295.GAO Z T. Fatigue application statistics[M]. Beijing:National Defence of Industry Press, 1986:277-295(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 914
- HTML全文浏览量: 95
- PDF下载量: 434
- 被引次数: 0
