Inertia mass of force transducers based on a modified Monte Carlo calibration method
-
摘要:
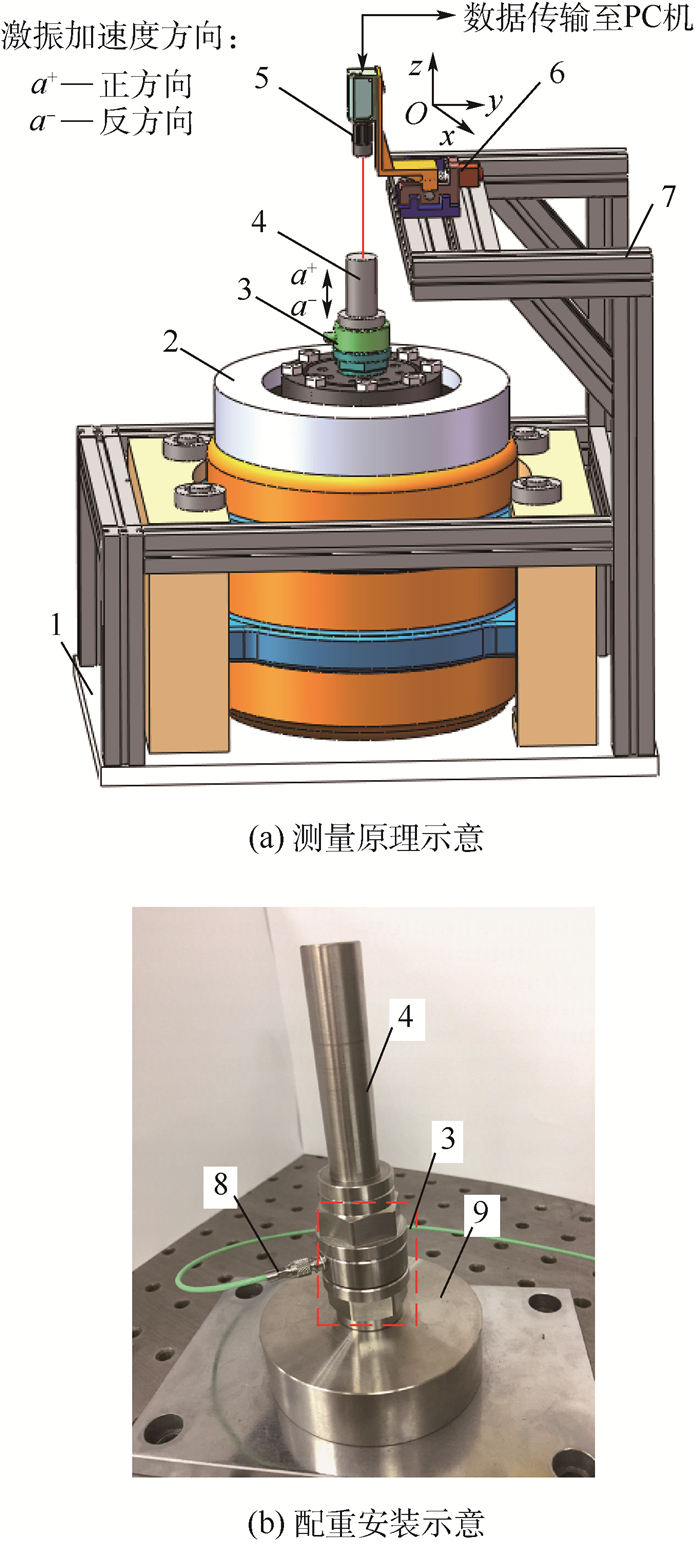
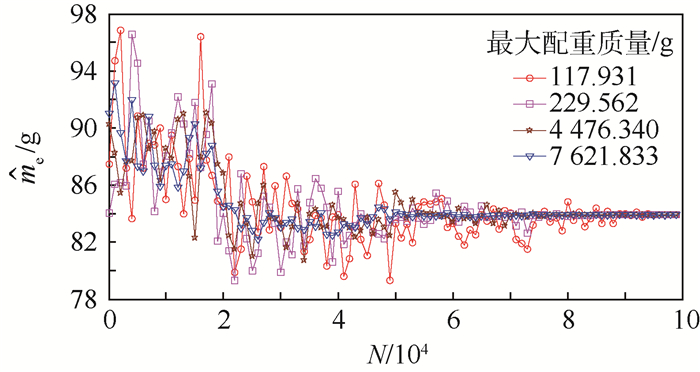
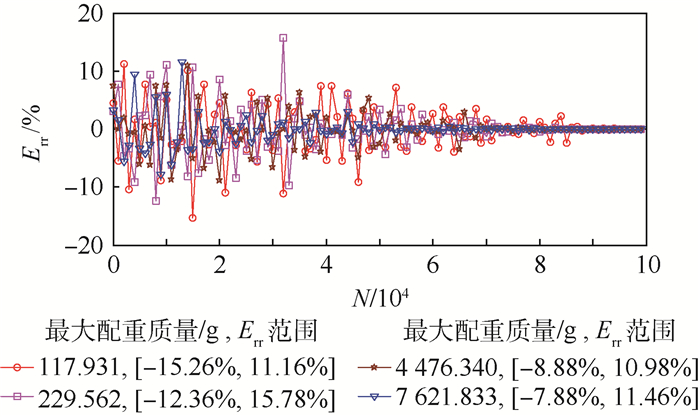
惯性质量是力传感器模型的重要校准参数，也是影响动态力测量精度的关键因素之一。为了消除参数误差对惯性质量校准模型引起的病态，提出一种改进Monte Carlo校准（MMCC）方法。首先，建立力传感器惯性质量、配重质量与测量响应之间的模型；其次，利用伪随机数生成技术，分别对该模型中的配重质量、加速度和电压进行样本空间的全域模拟；然后，根据区间判断准则筛选出满足预设精度的有效样本；最后，结合有效样本的概率，估计出力传感器的惯性质量，并实现动态校准。为了验证本文方法的准确性，利用正弦激振台对Kistler 9331B型力传感器进行动态校准。实验结果表明，惯性质量的估计值为83.91 g，估计误差为0.67%，标准差为0.74 g；动态力的校准误差范围为[-7.88%，11.46%]。校准误差明显低于传统的二次及多次配重法。
-
关键词:
- 力传感器 /
- 估计 /
- 模型病态 /
- Monte Carlo模拟 /
- 动态校准
Abstract:As an important parameter of a force transducer, the inertia mass can reduce the measurement accuracy of a dynamic force unless it has been accurately estimated. To eliminate the ill-posedness of an calibration model of the inertia mass caused by parameter errors, a modified Monte Carlo calibration (MMCC) method is proposed. Firstly, the mathematical model among the inertia mass, the additional mass, and the measurement response of the force transducer is built. Secondly, the parameter samples of this model including additional mass, acceleration, and voltage are simulated by pseudo-random number generation globally. Thirdly, the valid samples of these parameters are selected by interval screening technique. Finally, the inertia mass of the force transducer is estimated by solving the probability of these valid samples as well as the calibration of the force transducer. The accuracy of the MMCC method is verified by dynamic calibrating a Kistler 9331B force transducer with a sinusoidal vibration exciter. The experimental results show that the estimate of the inertia mass is 83.91g, the estimation error is 0.67%, the standard deviation is 0.74 g, and the calibration error range of the dynamic force is[-7.88%, 11.46%]. It indicates that the calibration error of MMCC method is less than the traditional secondary additional mass method and the multi-additional mass method.
-
Key words:
- force transducers /
- estimation /
- ill-posedness of model /
- Monte Carlo simulation /
- dynamic calibration
-
图 3 惯性质量的伪随机数模拟分布
Figure 3. Simulated distribution of pseudo-random numbers for inertia mass
图 5 力传感器动态校准装置
1—隔振基座；2—正弦激振台；3—被校准力传感器；4—配重；5—双频激光干涉仪；6—二维精密微动平台；7—测量支架；8—信号传输线；9—传感器基座。
Figure 5. Dynamic calibration device of a force transducer
图 6 不同配重质量的有效样本概率
Figure 6. Probability of valid samples for different additional mass
表 1 力传感器的激励与响应
Table 1. Excitations and responses of force transducer
No. 117.931 g配重 229.562 g配重 4 476.340 g配重 7 621.833 g配重 ai(t)/(m·s-2) Ui(t)/mV ai(t)/(m·s-2) Ui(t)/mV ai(t)/(m·s-2) Ui(t)/mV ai(t)/(m·s-2) Ui(t)/mV 1 151.92 623.69 158.78 650.83 165.65 679.46 178.68 732.70 2 152.61 623.46 159.47 654.34 166.33 682.31 179.37 736.02 3 153.29 629.81 160.16 656.49 167.02 685.99 180.05 739.03 4 153.98 633.01 160.84 659.73 167.70 688.37 180.74 739.89 5 154.67 634.48 161.53 662.12 168.39 691.56 181.43 745.46 6 155.35 636.03 162.21 665.26 169.08 694.24 182.11 747.95 7 156.04 636.83 162.90 668.72 169.76 696.18 182.79 750.59 8 156.73 642.98 163.59 669.61 170.45 699.95 183.48 753.29 9 157.41 645.64 164.27 670.38 171.13 702.01 184.17 755.78 10 158.09 648.50 164.96 673.33 165.65 679.46 184.86 758.45 
下载: 导出CSV
表 2 传统配重法的估计误差
Table 2. Estimation error of traditional additional mass method
方法 估计值/g 标准差/g 估计误差/% 二次配重法 85.70 2.24 2.13 多次配重法 84.98 1.34 1.28
下载: 导出CSV
-
[1] VLAJIC N, CHIJIOKE A.Traceable dynamic calibration of force transducers by primary means[J].Metrologia, 2016, 53(4):S136-S148. doi: 10.1088/0026-1394/53/4/S136 [2] REZAYAT A, NASSIRI V, PAUW D, et al.Identification of dynamic forces using group-sparsity in frequency domain[J].Mechanical Systems and Signal Processing, 2016, 70(71):756-768. [3] 姚建涛, 李立建, 许允斗, 等.超静定六维力传感器静定测量模型及标定方法[J].仪器仪表学报, 2013, 34(9):1927-1933.YAO J T, LI L J, XU Y D, et al.Statically determinate measurement model and calibration method of statically indeterminate six-axis force sensor[J].Chinese Journal of Scientific Instrument, 2013, 34(9):1927-1933(in Chinese). [4] SCHLEGEL C, KIECKENAP G, GLÖCKNER B, et al.Traceable periodic force calibration[J].Metrologia, 2012, 49(3):224-235. doi: 10.1088/0026-1394/49/3/224 [5] 高云凯, 冯海星, 方剑光, 等.基于质量线法的驾驶室惯性参数识别试验研究[J].振动与冲击, 2013, 32(16):193-197. doi: 10.3969/j.issn.1000-3835.2013.16.034GAO Y K, FENG H X, FANG J G, et al.Experimental study on identification of inertia parameters of truck cab based on mass line method[J].Journal of Vibration and Shock, 2013, 32(16):193-197(in Chinese). doi: 10.3969/j.issn.1000-3835.2013.16.034 [6] KUMME R.Investigation of the comparison method for the dynamic calibration of force transducers[J].Measurement, 1998, 23(4):239-245. doi: 10.1016/S0263-2241(98)00027-X [7] FUJⅡ Y. Proposal for a step response evaluation method for force transducers[J].Measurement Science and Technology, 2003, 14(10):1741-1746. doi: 10.1088/0957-0233/14/10/301 [8] FUJⅡ Y.Toward dynamic force calibration[J].Measurement, 2009, 42(7):1039-1044. doi: 10.1016/j.measurement.2009.03.006 [9] SHABANI R, TARIVERDILO S, SALARIEH H.Nonlinear identification of electro-magnetic force model[J].Journal of Zhejiang University:Science A, 2010, 11(3):165-174. doi: 10.1631/jzus.A0900203 [10] QIAO B, ZHANG X, WANG C, et al.Sparse regularization for force identification using dictionaries[J].Journal of Sound and Vibration, 2016, 368(1):71-86. [11] 汪凤泉, 许秀之.动态力传感器校准的两次配重消去法[J].计量学报, 1990, 11(4):304-310.WANG F Q, XU X Z.The elimination method by adding balance weight twice in calibration of dynamic force transducer[J].Acta Metrologica Sinica, 1990, 11(4):304-310(in Chinese). [12] 孙兴盛, 刘杰, 丁飞, 等.基于矩阵摄动的随机结构动态载荷识别技术[J].机械工程学报, 2014, 50(13):148-156.SUN X S, LIU J, DING F, et al.Identification method of dynamic loads for stochastic structures based on matrix perturbation theory[J].Journal of Mechanical Engineering, 2014, 50(13):148-156(in Chinese). [13] 杨子凯, 王建林, 于涛, 等.基于预测误差法的加速度传感器动态模型参数辨识[J].仪器仪表学报, 2015, 36(6):1244-1249. http://www.cnki.com.cn/Article/CJFDTotal-YQXB201506007.htmYANG Z K, WANG J L, YU T, et al.Dynamic model parameter identification of the acceleration sensor based on the prediction error method[J].Chinese Journal of Scientific Instrument, 2015, 36(6):1244-1249(in Chinese). http://www.cnki.com.cn/Article/CJFDTotal-YQXB201506007.htm [14] THOMAS B, SARA S, POSSOLO A.Force calibration using errors in variables regression and Monte Carlo uncertainty evaluation[J].Metrologia, 2016, 53(3):965-971. doi: 10.1088/0026-1394/53/3/965 [15] 王中宇, 刘智敏, 夏新涛, 等.测量误差与不确定度评定[M].北京:科学出版社, 2008:17-21.WANG Z Y, LIU Z M, XIA X T, et al.Measurement error and uncertainty evaluation[M].Beijing:Science Press, 2008:17-21(in Chinese). [16] JIANG W S, WANG Z Y, MOURELATOS Z P.Application of non-equidistant fractional order accumulation model on trajectory prediction of space manipulator[J].IEEE/ASME Transactions on Mechatronics, 2016, 21(3):1420-1427. doi: 10.1109/TMECH.2016.2517183 期刊类型引用(5)
1. 景凤，郭婧娟. 基于BIM的高铁工程量清单EBS＼WBS研究. 铁道标准设计. 2020(02): 68-74 . 
百度学术2. 周泽鑫，孙志强，徐冰，洪扬. 空间光学遥感器真空热试验工装模块化设计. 北京航空航天大学学报. 2019(08): 1544-1551 . 
本站查看3. 张秋雁，张俊玮，丛中笑，宋锡强，曾招辉，王忠义. 符合智能制造硬件要求的积木式单相智能电能表设计研究. 自动化与仪器仪表. 2018(06): 37-40 . 百度学术4. 田启华，梅月媛，杜义贤，周祥曼. 基于聚类分析的大容量耦合设计任务规划的研究. 中国机械工程. 2018(05): 544-551 . 百度学术5. 刘航，何铭鑫，聂仕麟. 基于模块化技术的复杂产品融合设计方案研究——以斯特林发动机为例. 管理工程师. 2017(06): 28-31 . 百度学术其他类型引用(11)
-
下载:
百度学术
点击查看大图
计量
- 文章访问数: 711
- HTML全文浏览量: 57
- PDF下载量: 340
- 被引次数: 16
