-
摘要:
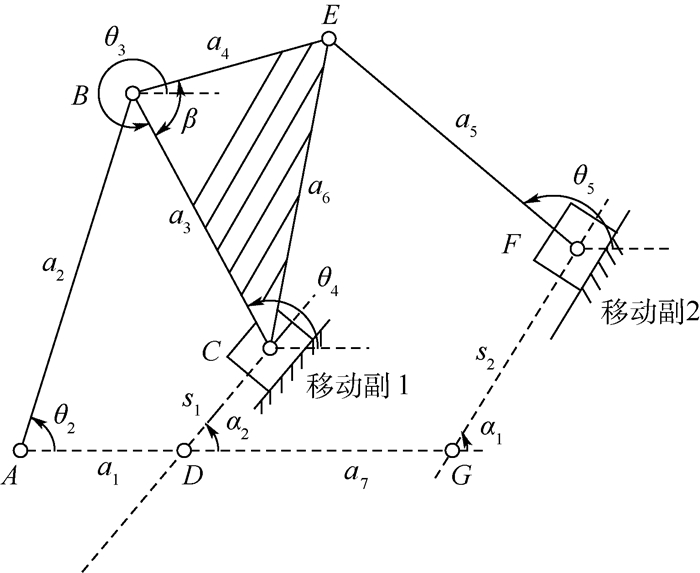
带移动副平面六连杆机构的分支、运动缺陷、运动范围等的判别是机构运动特性研究的重要指标。首先，将带2个移动副或3个移动副的平面六连杆机构分为四环链及五环链，引入欧拉公式、三角换元等方法，得到带移动副机构在极限位置（包括死点、分支点）的具体构型、具体关节输入输出关系以及关节旋转空间。其次，将2个链路结合，提出带2个移动副及3个移动副平面六连杆机构的分支识别方法。最后，通过实例验证，得到机构的分支点、死点及关节在所有极限位置的具体角度，为带移动副机构分支的自动识别提供了一种简便有效的方法。
Abstract:The identification of branch, motion defect and range of motion of the planar six-bar linkages with sliding pairs is an important indicator for motion characteristics of linkages. Firstly, this paper divides the planar six-bar linkages with two sliding pairs or three sliding pairs into four-bar chain or five-bar chain. B-ring in Euler's formula and trigonometric substitution method, the specific configurations (including branch points and dead points) of the planar linkages with sliding pairs in their limit positions, input and output relationship and joint rotation space are obtained. Secondly, this paper combines two chains and proposes a method for the branch identification of planar six-bar linkages with two or three sliding pairs. Finally, this paper verifies the proposed method with examples of planar six-bar linkages with two and three sliding pairs. The branches, dead points and the specific angles of joints in limit positions of the two mechanisms are calculated. The proposed method gives a convenient and effective way for the automatic branch identification of planar linkages with sliding pairs.
-
Key words:
- sliding pairs /
- planar six-bar linkages /
- limit position /
- branch /
- joint rotation space
-
图 5 带2个移动副平面六连杆机构分支识别流程
Figure 5. Flowchart of branch identification of planar six-bar linkage with two sliding pairs
图 6 带2个移动副平面六连杆机构分支曲线
Figure 6. Curve of branches of planar six-bar linkage with two sliding pairs
图 7 带2个移动副平面六连杆机构实例的分支曲线
Figure 7. Curve of branches of planar six-bar linkage with two sliding pairs
图 8 带2个移动副平面六连杆机构Δ4随θ2变化曲线
Figure 8. Change of Δ4 with θ2 for planar six-bar linkage with two sliding pairs
表 1 带2个移动副平面六连杆机构参数
Table 1. Parameters of planar six-bar linkage with two sliding pairs
参数 数值 a1 2.4 a2 5.5 a3 4.8 a4 4.2 a5 2.5 a7 2.4 β/(°) 45 α1/(°) 30 α2/(°) 50 
下载: 导出CSV
表 2 带2个移动副平面六连杆机构的分支点
Table 2. Branch points of planar six-bar linkage with two sliding pairs
分支点 θ2/(°) θ3/(°) θ4/(°) θ5/(°) 1 -161.4 -28.6 16.4 120 2 -141.6 -92.2 -47.2 -60 3 -105.5 55.3 100.3 120 4 -15.6 91.3 136.3 120 5 7.9 -152.7 -107.7 -60 6 72.6 -74.6 -29.6 120
下载: 导出CSV
表 3 带2个移动副平面六连杆机构的死点
Table 3. Dead points of planar six-bar linkage with two sliding pairs
下载: 导出CSV
表 4 带3个移动副平面六连杆机构参数
Table 4. Parameters of planar six-bar linkage with three sliding pairs
参数 数值 a1 3 a2 6 a3 5.2 a4 5 a7 4 β/(°) 50 γ/(°) 30 α1，α2/(°) 30
下载: 导出CSV
表 5 带3个移动副平面六连杆机构的死点
Table 5. Dead points of planar six-bar linkage with three sliding pairs
死点 θ2/(°) θ3/(°) θ4/(°) a -78.9 -40.0 10.0 b -158.9 -40.0 10.0
下载: 导出CSV
表 6 带2个移动副平面六连杆机构的2个位置参数
Table 6. Parameters of planar six-bar linkage with two sliding pairs for 2 positions
参数 位置1 位置2 θ2/(°) -50 10 B (3.54, -4.21) (5.42, 0.96) E (2.94, -0.06) (1.75, -1.10) F (1.32, 1.85) (-0.12, -2.97) Δ4 0.25 -0.34
下载: 导出CSV
-
[1] CHASE T R, MIRTH J A.Circuits and branches of single degree-of-freedom planar linkages[J].Journal of Mechanical Design, 1993, 115(2):223-230. doi: 10.1115/1.2919181 [2] TING K L, WANG J, XUE C, et al.Full rotatability and singularity of six-bar and geared five-bar linkages[J].Journal of Mechanisms and Robotics, 2010, 2(1):298-300. [3] TING K L, DOU X.Classification and branch identification of Stephenson six-bar chains[J].Mechanism & Machine Theory, 1996, 31(31):283-295. https://dialnet.unirioja.es/servlet/articulo?codigo=410136 [4] WANG J, TING K L, XUE C.Discriminant method for the mo-bility identification of single degree-of-freedom double-loop linkages[J].Mechanism & Machine Theory, 2010, 4(5):740-755. https://www.sciencedirect.com/science/article/pii/S0094114X09002274 [5] 杨通, 韩建友, 崔光珍, 等.给定两连杆运动面的Watt-Ⅰ六连杆机构空间解域综合方法[J].农业机械学报, 2014, 45(10):307-312. doi: 10.6041/j.issn.1000-1298.2014.10.048YANG T, HAN J Y, CUI G Z, et al.Synthesis of Watt-Ⅰ six-bar linkage based on spatial solution region for given two coupler plans[J].Transactions of the Chinese Society of Agricultural, 2014, 45(10):307-312(in Chinese). doi: 10.6041/j.issn.1000-1298.2014.10.048 [6] 韩建友, 崔光珍, 杨通, 等.六连杆机构四位置运动生成的解域综合理论与方法[J].北京航空航天大学学报, 2014, 40(9):1170-1175. http://bhxb.buaa.edu.cn/CN/abstract/abstract13015.shtmlHAN J Y, CUI G Z, YANG T, et al.The solution region synthesis theory and method of six-bar linkages with 4-position[J].Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(9):1170-1175(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13015.shtml [7] 崔光珍, 韩建友, 杨通.给定4R开链的八杆机构解域综合理论与方法[J].农业机械学报, 2015, 46(4):331-337. doi: 10.6041/j.issn.1000-1298.2015.04.049CUI G Z, HAN J Y, YANG T.Solution region synthesis method of eight-bar linkages for 4R open chain[J].Transactions of the Chinese Society of Agricultural Machinery, 2015, 46(4):331-337(in Chinese). doi: 10.6041/j.issn.1000-1298.2015.04.049 [8] 宋黎, 陈磊.含复铰有移动副平面运动链的结构类型综合方法[J].机械科学与技术, 2009, 28(3):295-300. https://www.cnki.com.cn/qikan-JXKX200903005.htmlSONG L, CHEN L.A method for structural type synthesis of planar kinematic chains with multiple joints and sliding joints[J]. Mechanical Science and Technology for Aerospace, 2009, 28(3):295-300(in Chinese). https://www.cnki.com.cn/qikan-JXKX200903005.html [9] 宋黎, 廖爱红, 聂松辉.含复铰有移动副平面机构运动分析数学模型的自动建立方法[J].机械科技与技术, 2012, 31(2):47-52.SONG L, LIAO A H, NIE S H.A method for automatically establishing model for kinematic analysis of planar mechanism with multiple joints and prismatic pair[J].Mechanical Science and Technology for Aerospace, 2012, 31(2):47-52(in Chinese). [10] PLECNIK M M, MCCARTHY J M.Kinematic synthesis of Stephenson Ⅲ six-bar function generators[J].Mechanism & Machine Theory, 2016, 97(9):112-126. https://www.sciencedirect.com/science/article/pii/S0094114X15002384 [11] PARRISH B E, MCCARTHY J M, EPPSTEIN D.Automated generation of linkage loop equations for planar one degree-of-freedom linkages, demonstrated up to 8-bar[J].Journal of Mechanisms and Robotics, 2015, 7(1):14-22. [12] 祖义祯, 邓华.基于弧长法的平面连杆机构运动分析[J].浙江大学学报(工学版), 2011, 45(12):2159-2168. doi: 10.3785/j.issn.1008-973X.2011.12.014ZU Y Z, DENG H.Kinematic analysis of planar pin-bar linkages by arc-length method[J].Journal of Zhejiang University(Engineering Science), 2011, 45(12):2159-2168(in Chinese). doi: 10.3785/j.issn.1008-973X.2011.12.014 [13] 李佳, 孔建益, 廖汉元, 等.全移动副机构的型综合[J].中国机械工程, 2012, 23(18):2165-2168. doi: 10.3969/j.issn.1004-132X.2012.18.005LI J, KONG J Y, LIAO H Y, et al.Type synthesis of mechanism with all prismatic pairs[J].China Mechanical Engineering, 2012, 23(18):2165-2168(in Chinese). doi: 10.3969/j.issn.1004-132X.2012.18.005 [14] 李占贤, 杨志杰, 于江.移动副驱动的2-DOF球面并联机构运动学分析[J].机械设计与制造, 2011(11):216-218. doi: 10.3969/j.issn.1001-3997.2011.11.084LI Z X, YANG Z J, YU J.Kinematic analysis of a 2-DOF spherical parallel mechanism driven by sliding pair[J].Machinery Design & Manufacture, 2011(11):216-218(in Chinese). doi: 10.3969/j.issn.1001-3997.2011.11.084 [15] SULTAN A, KALIM A.On the kinematics and synthesis of a geared five-bar slider-crank mechanism[J].Proceedings of the Institution of Mechanical Engineers Part C-Journal of Mechanical Engineering Science, 2011, 225(5):1253-1261. doi: 10.1177/2041298310392650 [16] SOH G S, YING F.Motion generation of planar six-and eight-bar slider mechanisms as constrained robotic systems[J].Journal of Mechanisms and Robotics, 2015, 7(3):975-978. [17] SALEH M A.Singularity traces of single degree-of-freedom planar linkages that include prismatic and revolute joints[J].Journal of Mechanisms and Robotics, 2015, 8(5):15-18. [18] WANG J, TING K L, ZHAO D X.Equivalent linkages and dead center positions of planar single-degree-of-freedom complex linkages[J].Journal of Mechanisms and Robotics, 2015, 7(4):1-6. -
下载:
点击查看大图
计量
- 文章访问数: 796
- HTML全文浏览量: 110
- PDF下载量: 474
- 被引次数: 0
