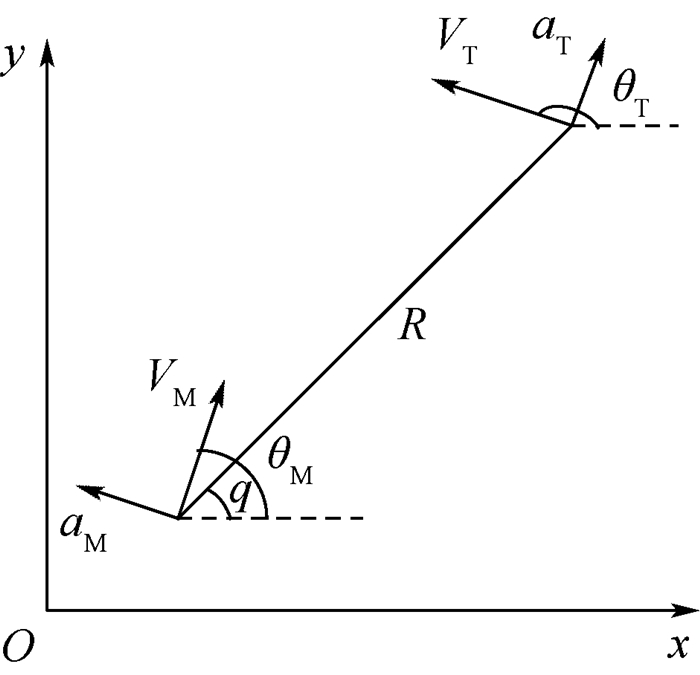
-
摘要:
为了实现大前置角拦截下的时间一致性饱和攻击，利用非线性导引方程，采取基于预测命中点（PIP）的剩余时间估计方法，结合等效滑模控制理论和Lyapunov稳定性定理，设计了一种大前置角拦截攻击时间控制导引律（ITCG）。针对固定目标和非机动运动目标，在弹目接近速度为负的情况下也能保证准确命中，实现了任意初始前置角下的指定时间到达，拓宽了导弹的制导初始条件，并给出了严格的理论证明。不同初始条件下的仿真结果验证了导引律的有效性。
Abstract:In order to achieve salvo attack for large heading errors with integrated time, an impact time control guidance (ITCG) law for large heading errors is proposed based on equivalent sliding mode control theory and Lyapunov stability theorem by adopting the nonlinear guidance equation and the time-to-go approximation algorithm according to predicting interception point (PIP). For stationary and non-maneuvering constant velocity targets, the proposed guidance law achieves impact time successfully with any designated initial heading error even when the closing speed is negative. Initial conditions of the missile guidance are widely broadened. Rigorous theoretical proof demonstrates the validity of the guidance law. The simulation results under different initial conditions verify the effectiveness of the proposed guidance law.
-
Key words:
- large heading errors /
- impact time control /
- salvo attack /
- equivalent sliding mode /
- guidance law
-
图 2 固定目标拦截时不同攻击时间仿真
Figure 2. Simulation of different impact time for intercepting stationary target
图 3 固定目标拦截时不同前置角仿真
Figure 3. Simulation of different heading errors for intercepting stationary target
图 4 运动目标拦截时不同攻击时间仿真
Figure 4. Simulation of different impact time for intercepting moving target
图 5 运动目标拦截时不同前置角仿真
Figure 5. Simulation of different heading errors for intercepting moving target
表 1 仿真参数值
Table 1. Value of simulation parameters
初始参数 数值 导弹初始位置/m (0，0) 目标初始位置/m (10 000，0) 导弹弹道倾角θM0/(°) 60 目标弹道倾角θT0/(°) 180 导弹速度VM/(m·s-1) 300 目标速度VT/(m·s-1) 0、120 导弹最大法向加速度aMmax/(m·s-2) 250 
下载: 导出CSV
-
[1] KIM B S, LEE J G, HAN H S.Biased PNG law for impact with angular constraint[J].IEEE Transactions on Aerospace and Electronic Systems, 1998, 34(1):277-288. doi: 10.1109/7.640285 [2] 张友安, 马国欣.攻击时间控制的动态逆三维制导律[J].哈尔滨工程大学学报, 2010, 31(2):215-219. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=hebgcdxxb201002014ZHANG Y A, MA G X.Dynamic inversion three-dimensional guidance law for impact time control[J].Journal of Harbin Engineering University, 2010, 31(2):215-219(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=hebgcdxxb201002014 [3] 张友安, 张友根.多导弹攻击时间与攻击角度两阶段制导[J].吉林大学学报(工学版), 2010, 40(5):1442-1447. http://or.nsfc.gov.cn/bitstream/00001903-5/101415/1/1000000735946.pdfZHANG Y A, ZHANG Y G.Two stages guidance to control impact time and impact angle for multi-missiles[J].Journal of Jilin University(Engineering and Technology Editiion), 2010, 40(5):1442-1447(in Chinese). http://or.nsfc.gov.cn/bitstream/00001903-5/101415/1/1000000735946.pdf [4] HARL N, BALAKRISHNAN S.Impact time and angle guidance with sliding mode control[J]. IEEE Transactions on Control Systems Technology, 2012, 20(6):1436-1449. doi: 10.1109/TCST.2011.2169795 [5] LEE J I, JEON I S, TAHK M J.Guidance law to control impact time and angle[J].IEEE Transactions on Aerospace and Electronic Systems, 2007, 43(1):301-310. doi: 10.1109/TAES.2007.357135 [6] ARITA S, UENO S. Optimal feedback guidance for nonlinear missile model with impact time and angle constraints[C]//AIAA Guidance, Navigation, and Control(GNC)Conference. Reston: AIAA, 2013: 1-12. [7] ZHANG L M, SUN M W, CHEN Z Q, et al.Receding horizon trajectory optimization with terminal impact specifications[J].Mathematical Problems in Engineering, 2014, 28(6):1-8. https://www.hindawi.com/journals/mpe/2014/604705/tab2/ [8] YAN F, KEMAO M, YUQING C.Cooperative guidance laws with constraints on impact time and terminal angle[J].Journal of System Simulation, 2014, 26(10):2434-2441. https://www.researchgate.net/publication/289645580_Cooperative_guidance_laws_with_constraints_on_impact_time_and_terminal_angle [9] 马国欣, 张友安.带有导引头视场限制的攻击时间控制导引律[J].弹道学报, 2013, 25(2):6-11. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=ddxb201302002MA G X, ZHANG Y A.Impact time control guidance law with seeker field-of-view limit[J].Journal of Ballistics, 2013, 25(2):6-11(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=ddxb201302002 [10] ZHANG Y A, MA G X, LIU A L.Guidance law with impact time and impact angle constraints[J].Chinese Journal of Aeronautics, 2013, 26(4):960-966. doi: 10.1016/j.cja.2013.04.037 [11] GHOSH S, GHOSE D, RAHA S. Three dimensional retro-PN based impact time control for higher speed non-maneuvering targets[C]//2013 IEEE 52nd Annual Conference on Decision and Control(CDC). Piscataway, NJ: IEEE Press, 2013: 4865-4870. [12] KUMAR S R, GHOSE D. Sliding mode control based guidance law with impact time constraints[C]//2013 American Control Conference(ACC). Piscataway, NJ: IEEE Press, 2013: 5760-5765. [13] JEON I S, LEE J I, TAHK M J.Homing guidance law for cooperative attack of multiple missiles[J].Journal of Guidance, Control, and Navigation, 2010, 33(1):275-280. doi: 10.2514/1.40136 [14] 马国欣, 张友安, 李大鹏.基于虚拟领弹的攻击时间和攻击角度控制[J].飞行力学, 2009, 27(5):51-54. http://d.wanfangdata.com.cn/Periodical_fxlx200905014.aspxMA G X, ZHANG Y A, LI D P.Virtual-leader based impact time and impact angle control[J].Flight Dynamics, 2009, 27(5):51-54(in Chinese). http://d.wanfangdata.com.cn/Periodical_fxlx200905014.aspx [15] SHIYU Z, RUI Z, CHEN W, et al.Design of time-constrained guidance laws via virtual leader approach[J].Chinese Journal of Aeronautics, 2010, 23(1):103-108. doi: 10.1016/S1000-9361(09)60193-X [16] KIM T H, LEE C H, JEON I S, et al.Augmented polynomial guidance with impact time and angle constraints[J].IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(4):2806-2817. doi: 10.1109/TAES.2013.6621856 [17] 李辕, 赵继广, 白国玉, 等.基于预测碰撞点的剩余飞行时间估计方法[J].北京航空航天大学学报, 2016, 42(8):1667-1674. http://bhxb.buaa.edu.cn/CN/abstract/abstract13404.shtmlLI Y, ZHAO J G, BAI G Y, et al.The method of time-to-go estimation based on predicted crack point[J].Journal of Beijing University of Aeronautics and Astronautics, 2016, 42(8):1667-1674(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13404.shtml -
下载:
点击查看大图
计量
- 文章访问数: 784
- HTML全文浏览量: 75
- PDF下载量: 321
- 被引次数: 0
