-
摘要:
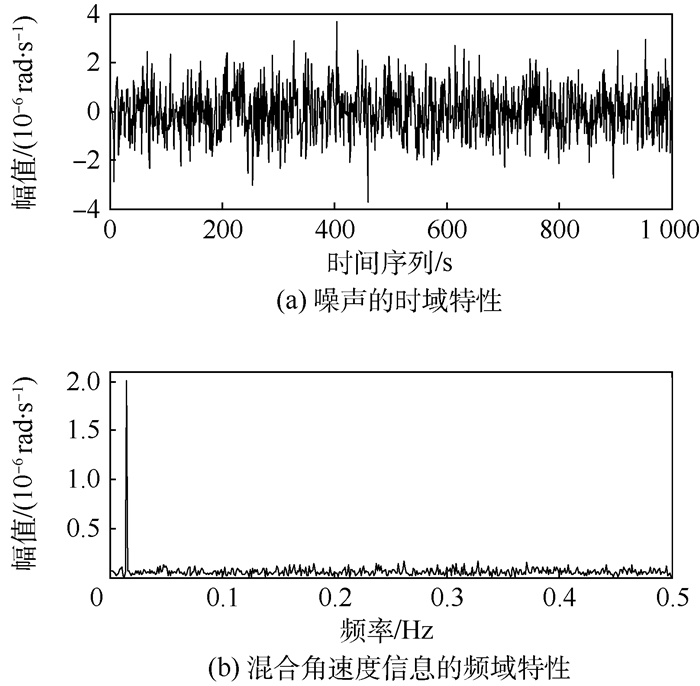
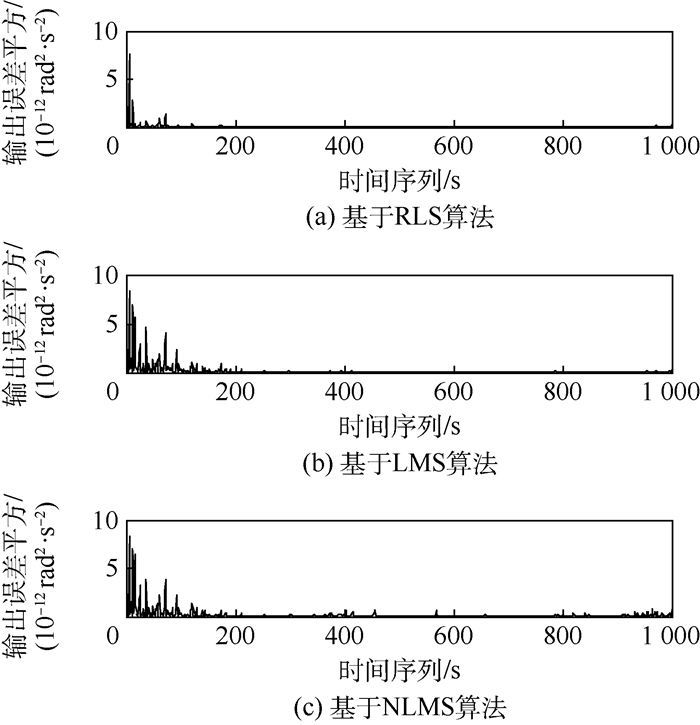
针对超流体陀螺相位波动噪声影响陀螺角速度检测精度的问题，提出了一种基于递推最小二乘（RLS）算法的陀螺自适应噪声抵消系统。首先，建立了超流体陀螺的相位检测模型，得到了陀螺输出薄膜幅值和相位的关系。其次，考虑热运动的影响，建立了相位波动噪声的等效输入角速度模型，探索了陀螺参数对角速度噪声的影响，得到了陀螺角速度噪声幅值范围。在此基础上，考虑该角速度噪声与输入角速度的互不相关性，将超流体陀螺薄膜幅值解算输出的混合角速度信息作为抵消系统的期望输入，将相位波动噪声引起的角速度噪声作为RLS自适应滤波器的参考输入，通过自适应调节参数使得RLS自适应滤波器的输出与混合角速度信息的噪声部分相抵消。通过与最小均方（LMS）算法仿真对比表明，在大角速度、大噪声情况下，该抵消系统能够有效消除陀螺混合角速度信息中的噪声成分，且具有较快的收敛速度和较好的稳定性。
-
关键词:
- 超流体陀螺 /
- 相位波动噪声 /
- 自适应滤波 /
- 递推最小二乘(RLS)算法 /
- 噪声抵消
Abstract:Aimed at the deterioration issue of gyroscope angular velocity measurement precision, which is caused by phase fluctuation noise of superfluid gyroscope, a gyroscope noise cancellation system based on recursive least square (RLS) algorithm was proposed. First, the phase detection model of superfluid gyroscope was established, and the relationship between thin film displacement and phase of gyroscope was obtained. Second, considering the influence of thermal motion, the noise equivalent input angular velocity model of gyroscope was established, the influence of gyroscope parameter on angular velocity noise was explored, and the amplitude range of angular velocity noise was obtained. Furthermore, considering the irrelevance between angular velocity noise and input angular velocity, the mixed angular velocity information which is calculated from the thin film amplitude of the gyroscope was taken as original input of the noise cancellation system, and angular velocity noise which is caused by phase noise as the reference input of RLS adaptive filter. The noise portion of the mixed angular velocity is offset by the output of RLS filter through adjusting the parameters. Finally, the comparison results between this method and least mean square (LMS) algorithm show that the noise portion in mixed angular velocity information can be effectively suppressed by this cancellation system with fast convergence speed and good stability in the case of large angular velocity and large noise.
-
图 5 背景信息的时域和频域特性
Figure 5. Time domain and frequency domain characteristics of background information
图 10 大角速度、大噪声时3种算法的均方误差
Figure 10. Mean square error of three algorithmswhen angular velocity and noise are large
表 1 超流体陀螺的主要参数
Table 1. Main parameters of superfluid gyroscope
参数 数值 柔性薄膜面积/cm2 0.5 微孔数目 4 225 超流体密度/(kg·m-3) 3 陀螺的感应面积/cm2 10 临界流量/(kg·s-1) 5.6×10-12 
下载: 导出CSV
表 2 输入信噪比为3 dB时的相关量化指标
Table 2. Relevant quantitative indicators when input signal to noise ratio is 3 dB
算法 均方误差/(10-12rad2·s-2) 输出信噪比/dB 100次迭代运算耗时/s RLS 1 16.43 5.124 LMS 1 12.03 2.552 NLMS 1 12.39 3.414
下载: 导出CSV
表 3 输入信噪比为-3 dB时的相关量化指标
Table 3. Relevant quantitative indicators when input signal to noise ratio is -3 dB
算法 均方误差/(10-12rad2·s-2) 输出信噪比/dB 100次迭代运算耗时/s RLS 4 16.31 5.281 LMS 4 9.29 2.474 NLMS 4 9.48 3.282
下载: 导出CSV
表 4 大角速度、大噪声时的相关量化指标
Table 4. Relevant quantitative indicators when angular velocity and noise are large
算法 均方误差/(10-12rad2·s-2) 输入信噪比/dB 输出信噪比/dB 100次迭代运算耗时/s RLS 16 3 16.44 5.304 LMS 16 3 4.52 2.492 NLMS 16 3 10.48 3.293
下载: 导出CSV
-
[1] DOLBIN A V.Physics of nanostructures at low temperatures[J].Low Temperature Physics, 2016, 42(2):75. doi: 10.1063/1.4941964 [2] PACKARD R.Principles of superfluid helium gyroscopes[J].Physical Review B, 1992, 46(6):3540-3549. doi: 10.1103/PhysRevB.46.3540 [3] SATO Y, ADITYA J, PACKARD R.Superfluid 4He quantum interference grating[J].Physical Review Letters, 2008, 101(8):085302-1-085302-4. doi: 10.1103/PhysRevLett.101.085302 [4] HOSKINSON E. Superfluid 4He weak links[D]. Berkeley: University of California, 2005: 45-58. http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.210.7628&rep=rep1&type=pdf [5] SATO Y.Sagnac-based rotation sensing with superfluid helium quantum interference devices[J].Comptes Rendus Physique, 2014, 15(10):898-906. doi: 10.1016/j.crhy.2014.10.004 [6] GOLOVASHKIN A I, ZHERIKHINA L N, TSKHOVREBOV A M, et al.Ordinary SQUID interferometers and superfluid helium matter wave interferometers:The role of quantum fluctuations[J].Journal of Experimental and Theoretical Physics, 2010, 111(2):332-339. doi: 10.1134/S1063776110080285 [7] SIMMOND R W, MARCHENKOV A, HOSKINSON E, et al.Quantum interference of superfluid 3He[J].Nature, 2001, 412(6842):55-58. doi: 10.1038/35083518 [8] SATO Y, PACKARD R.DC superconducting quantum interference device based neodymium magnet displacement sensor for superfluid experiments[J].Review of Scientific Instruments, 2009, 80(5):055102. doi: 10.1063/1.3129942 [9] CHUI T, HOLMES W, PENANEN K.Fluctuations of the phase difference across an array of Josephson junctions in superfluid 4He near the Lambda transition[J].Physical Review Letters, 2003, 90(8):085301. https://www.researchgate.net/profile/Warren_Holmes/publication/10860161_Fluctuations_of_the_Phase_Difference_across_an_Array_of_Josephson_Junctions_in_Superfluid_H_e_4_near_the_Lambda_Transition/links/004635277d654ca344000000/Fluctuations-of-the-Phase-Difference-across-an-Array-of-Josephson-Junctions-in-Superfluid-H-e-4-near-the-Lambda-Transition.pdf [10] NARAYANA S, SATO Y.Superfluid quantum interference in multiple-turn reciprocal geometry[J].Physical Review Letters, 2011, 106(25):255301. doi: 10.1103/PhysRevLett.106.255301 [11] MAO Y, DING F, ALSAEDI A, et al.Adaptive filtering parameter estimation algorithms for Hammerstein nonlinear systems[J].Signal Processing, 2016, 128:417-425. doi: 10.1016/j.sigpro.2016.05.009 [12] DU G, ZHANG P.A markerless human-robot interface using particle filter and Kalman filter for dual robots[J].IEEE Transactions on Industrial Electronics, 2015, 62(4):2257-2264. doi: 10.1109/TIE.2014.2362095 [13] AMIRYARAHMADI N, KROPP W, LARSSON K.Application of LMS algorithm to measure low-frequency transient forces from human walking[J].Acta Acustica United with Acustica, 2016, 102(1):23-34. doi: 10.3813/AAA.918921 [14] SATO Y, ADITYA J, PACKARD R.Flux locking a superfluid interferometer[J].Applied Physics Letters, 2007, 91(7):1-3. doi: 10.1063/1.2772659 [15] SATO Y. Experiments using 4He weak link[D]. Berkeley: University of California, 2007: 51-58. http://gradworks.umi.com/33/06/3306328.html [16] PACKARD R.The role of the Josephson-Anderson equation in superfluid helium[J].Review of Modern Physics, 1998, 70(2):641-651. doi: 10.1103/RevModPhys.70.641 [17] KOHLI A K, RAI A.Numeric variable forgetting factor RLS algorithm for second-order volterra filtering[J].Circuits, Systems, and Signal Processing, 2013, 32(1):223-232. doi: 10.1007/s00034-012-9445-7 -
下载:
点击查看大图
计量
- 文章访问数: 692
- HTML全文浏览量: 93
- PDF下载量: 399
- 被引次数: 0
