-
摘要:
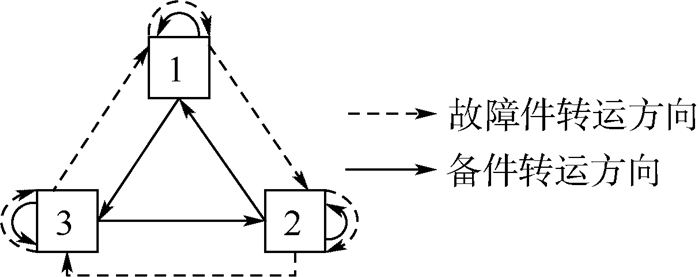
基于三站点库存组织研究了备件转运策略对于系统期望延期交货量（EBO）的影响。首先，依据三站点库存组织中备件和故障件的消耗与补给过程以及库存控制策略，给出了考虑保障站点供应优先级的手边现货、应得备件数和延期交货量之间的状态方程。其次，通过定义站点延期交货量与应得备件数之间的比例系数，将原非线性方程组转化成线性方程组，然后借助线性方程组解的判定法则对方程组解的唯一性进行判定，并结合不动点原理构造柯西列逼近原方程的解。最后，在算例分析中借助MATLAB对2种转运策略（按固定概率转运和按优先级约束转运）下系统EBO的数值大小进行对比。结果表明：按优先级约束进行备件转运能够在大部分情况下提高备件利用率同时降低系统EBO。此外，通过分析还可以发现，不同备件转运策略对系统EBO作用效果的显著性主要由转运策略的可行作用空间大小决定，而作用空间的可行变化范围主要由备件需求由本地满足的概率、库存配置方案以及需求率和维修速率决定。
-
关键词:
- 备件 /
- 三站点库存组织 /
- 期望延期交货量(EBO) /
- 转运策略 /
- 优先级约束
Abstract:In this paper, the effect of variant transshipment strategies on expected backorders (EBO) of a three-site inventory system is researched. Based on the predefined assumptions and inventory control strategy, as well as the consumption and replenishment process of the spares, the state equations of spares on hand, spares due in from repair and resupply, and backorders are presented with the consideration of the supply priority among sites. By defining the ratio between EBO and the number of spares due in, the original nonlinear equations can be transformed into linear equations. Since the coefficient matrix of the linear equations is invertible, it can be said that the linear equations has only one solution. As indicated above, a Cauchy sequence of the number of spares due in is constructed to approach the sole solution. Finally, the numerical values of the EBO under two transshipment strategies (fixed probability transshipment and priority preferred transshipment) are compared. The results show that the strategy of priority preferred transshipment can not only increase the utilization rate of spares but also reduce EBO of the system in most cases. In addition, it can be found that the effect of different transshipment strategies on the EBO mainly depends on the feasible space of transshipment strategies, which is mainly determined by the rate of demands filled by local site, the inventory allocation scheme, and the repairing and demanding rate of each site.
-
图 1 部件在三站点库存组织中的转运模型
Figure 1. Transshipment model of items in three-site inventory system
图 3 库存配置方案(s1+s2+s3=50)对系统EBO的影响曲线
Figure 3. Influence curves of inventory allocation schemes (s1+s2+s3=50) on system EBO
图 4 库存配置方案(s1=0, s2+s3=50)对系统EBO的影响曲线
Figure 4. Influence curves of inventory allocation schemes (s1=0, s2+s3=50) on system EBO
图 5 备件需求由本地满足的概率对系统EBO的影响曲线
Figure 5. Influence curves of probability of demands filled by local site on system EBO
图 6 需求率和运输时间对系统EBO的影响曲线
Figure 6. Influence curves of demanding rate and transport time on system EBO
-
[1] SHERBROOKE C C.METRIC:A multi-echelon technique for recoverable item control[J].Operations Research, 1968, 16(1):122-141. doi: 10.1287/opre.16.1.122 [2] MUCKSTADT J A. A model for a multi-item, multi-echelon, multi-indenture inventory system[J].Management Science, 1973, 20(4):472-481. [3] SLAY F M. VARI-METRIC: An approach to modeling multi-echelon resupply when the demand process is Poisson with a gamma prior: Report AF301-3[R]. Washington, D. C. : Logistics Management Institute, 1984. [4] SHERBROOKE C C.VARI-METRIC:Improved approximations for multi-indenture, multi-echelon availability models[J].Operations Research, 1986, 34(2):311-319. doi: 10.1287/opre.34.2.311 [5] AXSATER S.Modelling emergency lateral transshipments in inventory systems[J].Management Science, 1990, 36(11):1329-1338. doi: 10.1287/mnsc.36.11.1329 [6] KRANENBURG A A, HOUTUM G J V.A new partial pooling structure for spare parts networks[J].European Journal of Operational Research, 2009, 199(3):908-921. doi: 10.1016/j.ejor.2009.01.057 [7] LEE H L.A multi-echelon inventory model for repairable items with emergency lateral transshipments[J].Management Science, 1987, 33(10):1302-1316. doi: 10.1287/mnsc.33.10.1302 [8] TER S.Exact and approximate evaluation of batch-ordering policies for two-level inventory systems[J].Operations Research, 1993, 41(4):777-785. doi: 10.1287/opre.41.4.777 [9] 董琪, 徐延学, 丛林虎.基于Markov三级库存系统备件横向调拨配置模型[J].系统工程与电子技术, 2015, 37(11):2524-2530. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=xtgcydzjs201511016DONG Q, XU Y X, CONG L H.Allocation model of spare parts for three-echelon inventory system with lateral transshipment based on Markov[J].Systems Engineering and Electronics, 2015, 37(11):2524-2530(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=xtgcydzjs201511016 [10] 张光宇, 李庆民, 郭璇.基于横向转运策略的可修备件多点库存建模方法[J].系统工程与电子技术, 2012, 34(7):1424-1429. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=xtgcydzjs201207022ZHANG G Y, LI Q M, GUO X.Modeling for repairable spare parts in multi-location inventory system with lateral transshipments[J].Journal of Systems Engineering and Electronics, 2012, 34(7):1424-1429(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=xtgcydzjs201207022 [11] ALFREDSSON P, VERRIJDT J.Modeling emergency supply flexibility in a two-echelon inventory system[J].Management Science, 1999, 45(10):1416-1431. doi: 10.1287/mnsc.45.10.1416 [12] OLSSON F. An inventory model with unidirectional lateral transshipments[J].European Journal of Operational Research, 2010, 200(3):725-732. doi: 10.1016/j.ejor.2009.01.024 [13] AXSATER S.Evaluation of unidirectional lateral transshipments and substitutions in inventory systems[J].European Journal of Operational Research, 2003, 149(2):438-447. doi: 10.1016/S0377-2217(02)00447-2 [14] 阮旻智, 刘任洋.基于横向转运策略的多级库存配置建模与优化[J].中国工程科学, 2015, 17(5):106-112. http://industry.wanfangdata.com.cn/dl/Detail/Periodical?id=Periodical_zggckx201505018RUAN M Z, LIU R Y.Modeling and optimization for multi-echelon spares inventory configuration with lateral transshipments[J].Engineering Sciences, 2015, 17(5):106-112(in Chinese). http://industry.wanfangdata.com.cn/dl/Detail/Periodical?id=Periodical_zggckx201505018 [15] DIKS E B, KOK A G D.Controlling a divergent 2-echelon network with transshipments using the consistent appropriate share rationing policy[J].International Journal of Production Economics, 1996, 45(1-3):369-379. doi: 10.1016/0925-5273(95)00007-0 [16] OLSSON F.Optimal policies for inventory systems with lateral transshipments[J].International Journal of Production Economics, 2009, 118(1):175-184. doi: 10.1016/j.ijpe.2008.08.021 [17] JUNG B R, SUN B G, KIM J S, et al.Modeling lateral transshipments in multiechelon repairable-item inventory systems with finite repair channels[J].Computers & Operations Research, 2003, 30(9):1401-1417. https://www.sciencedirect.com/science/article/pii/S0305054802000783 [18] ALVAREZ E M, HEIJDEN M C V D, VLIEGEN I M H, et al.Service differentiation through selective lateral transshipments[J].European Journal of Operational Research, 2013, 237(3):824-835. https://www.sciencedirect.com/science/article/pii/S037722171400188X [19] KRANENBURG A A, HOUTUM G J V.A new partial pooling structure for spare parts networks[J].European Journal of Operational Research, 2009, 199(3):908-921. doi: 10.1016/j.ejor.2009.01.057 [20] KUKREJA A, SCHMIDT C P, MILLER D M.Stocking decisions for low-usage items in a multilocation inventory system[J].Management Science, 2001, 47(10):1371-1383. doi: 10.1287/mnsc.47.10.1371.10263 [21] 包记凯, 罗长远, 周道水, 等.维修备件横向转运库存优化模型[J].信息工程大学学报, 2014, 15(1):24-28. http://www.cnki.com.cn/Article/CJFDTOTAL-XXGC201401005.htmBAO J K, LUO C Y, ZHOU D S, et al.Optimization model for maintenance spare parts inventory system with lateral transshipments[J].Journal of Information Engineering University, 2014, 15(1):24-28(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-XXGC201401005.htm [22] 张颖, 王蕴, 薛佩.三站点备件供应保障关系建模与分析[J].北京航空航天大学学报, 2017, 43(8):1625-1631. http://bhxb.buaa.edu.cn/CN/abstract/abstract14114.shtmlZHANG Y, WANG Y, XUE P.Modeling and analysis of spares supply support relations among three stations[J].Journal of Beijing University of Aeronautics and Astronautics, 2017, 43(8):1625-1631(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract14114.shtml [23] NONAS L M, JORNSTEN K.Optimal solutions in the multi-location inventory system with transshipments[J].Journal of Mathematical Modelling and Algorithms in Operations Research, 2007, 6(1):47-75. doi: 10.1007/s10852-006-9049-y [24] TAGARAS G.Pooling in multi-location periodic inventory distribution systems[J].Omega, 1999, 27(1):39-59. doi: 10.1016/S0305-0483(98)00030-9 [25] 张勇, 杨宏伟, 杨学强, 等.基于复杂网络理论的装备保障网络模型研究[J].上海理工大学学报, 2012, 34(5):429-434. http://d.wanfangdata.com.cn/Periodical_shlgdxxb201205004.aspxZHANG Y, YANG H W, YANG X Q, et al.Model of equipment support network based on theory of complex networks[J].Journal of University of Shanghai for Science and Technology, 2012, 34(5):429-434(in Chinese). http://d.wanfangdata.com.cn/Periodical_shlgdxxb201205004.aspx [26] 张勇, 杨宏伟, 白勇, 等.基于复杂网络理论的装备保障网络实证研究[J].装备学院学报, 2014, 25(1):83-87. http://d.wanfangdata.com.cn/Periodical_zhjsxy201401023.aspxZHANG Y, YANG H W, BAI Y, et al.An empirical study on the equipment support network based on complex networks[J].Journal of Academy of Equipment, 2014, 25(1):83-87(in Chinese). http://d.wanfangdata.com.cn/Periodical_zhjsxy201401023.aspx [27] SHERBROOKE C C.Optimal inventory modeling of systems:Multi-echelon techniques[M].2nd ed.Boston:John Wiley & Sons, 2004:48-57. -
下载:
点击查看大图
计量
- 文章访问数: 1005
- HTML全文浏览量: 161
- PDF下载量: 316
- 被引次数: 0
