基于PCE的谐波减速器动态精度不确定性分析

张金洋; 张建国; 彭文胜; 刘育强; 汪龙

doi:10.13700/j.bh.1001-5965.2017.0305

基于PCE的谐波减速器动态精度不确定性分析

doi: 10.13700/j.bh.1001-5965.2017.0305

张金洋^{1, 2},
张建国^{1, 2, ,},
彭文胜^{1, 3},
刘育强⁴,
汪龙⁵

1.
北京航空航天大学可靠性与系统工程学院, 北京 100083
2.
北京航空航天大学可靠性与环境工程重点实验室, 北京 10008
3.
中国航空综合技术研究所, 北京 100028
4.
中国空间技术研究院总体部, 北京 100029
5.
北京卫星制造厂, 北京 100094

基金项目:

国家“973”计划 2013CB733000

详细信息

作者简介:
张金洋  男, 硕士研究生。主要研究方向:机械产品可靠性

张建国  男, 博士, 教授, 博士生导师。主要研究方向:机械产品可靠性、机电产品可靠性

彭文胜  男, 博士研究生。主要研究方向:机械设计、机电产品可靠性设计

通讯作者:
张建国, E-mail: zjg@buaa.edu.cn

中图分类号: V442
计量
- 文章访问数: 898
- HTML全文浏览量: 118
- PDF下载量: 541
- 被引次数: 0
出版历程
- 收稿日期: 2017-05-12
- 录用日期: 2017-06-16
- 网络出版日期: 2018-05-20

Dynamic accuracy uncertainty analysis of harmonic reducer based on PCE

ZHANG Jinyang^{1, 2},
ZHANG Jianguo^{1, 2
, ,},
PENG Wensheng^{1, 3},
LIU Yuqiang⁴,
WANG Long⁵

1.
School of Reliability and Systems Engineering, Beijing University of Aeronautics and Astronautics, Beijing 100083, China
2.
Science and Technology on Reliability and Engineering Laboratory, Beijing University of Aeronautics and Astronautics, Beijing 10008
3.
AVIC China Aero-poly Technology Establishment, Beijing 100028, China
4.
Institute of Spacecraft System Engineering CAST, Beijing 100029, China
5.
Beijing Satellite Manufacturer, Beijing 100094, China

Funds:

National Basic Research Program of China 2013CB733000

More Information

Corresponding author: ZHANG Jianguo, E-mail: zjg@buaa.edu.cn

摘要

摘要:
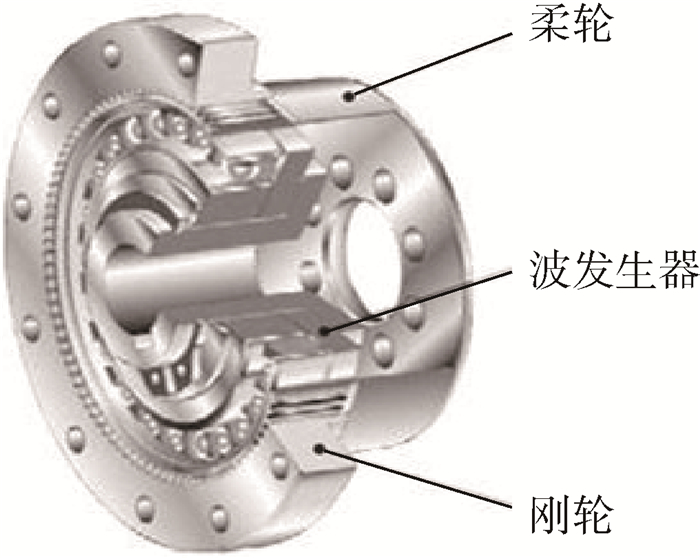
谐波减速器的动态精度不仅与其各个部件的制造公差和装配间隙有关，还必须考虑谐波减速器柔性和摩擦的影响。目前谐波减速器精度问题研究大多只考虑单一因素，在进行精度分析时没有考虑到模型参数的不确定性对精度的影响。本文研究了谐波减速器在静态因素（加工、装配）和动力学因素（柔性、摩擦）综合作用下的动态精度问题；建立了考虑静态误差、柔性的非线性动力学模型；利用多项式混沌展开（PCE）方法进行参数灵敏度分析和不确定性分析，并和Monte Carlo方法作了比较，结果表明PCE方法效率更高。并基于动态精度PCE进行可靠性分析，得到动态精度可靠度。
- 谐波减速器 /
- 动态精度 /
- 多项式混沌展开(PCE) /
- 灵敏度分析 /
- 可靠性分析
Abstract:
The dynamic accuracy of the harmonic reducer is related to the parts' tolerance and assembly clearance as well as the flexibility and friction of the harmonic reducer. Most of the published literatures consider only a single factor and do not take into account the influence of model parameter uncertainty. In this paper, the dynamic accuracy is researched considering static factors (machining and assembly) and dynamic characteristics (flexibility and friction). The nonlinear dynamic model is established, which contains static error and flexibility term. Polynomial chaos expansion (PCE) is used to handle the parameter sensitivity and uncertainty. By comparison, PCE is more efficient than Monte Carlo. Dynamic accuracy reliability is finally obtained through reliability analysis based on dynamic accuracy PCE.
- harmonic reducer /
- dynamic accuracy /
- polynomial chaos expansion (PCE) /
- sensitivity analysis /
- reliability analysis

HTML全文

图 1 谐波减速器结构简图

Figure 1. Structure diagram of harmonic reducer

下载: 全尺寸图片幻灯片

图 2 谐波减速器往复运动动态误差曲线

Figure 2. Dynamic error curve of harmonic reducer reciprocation

下载: 全尺寸图片幻灯片

图 3 谐波减速器物理简化模型

Figure 3. A physical simplified model of harmonic reducer

下载: 全尺寸图片幻灯片

图 4 谐波减速器Dymola仿真模型

Figure 4. Dymola simulation model of harmonic reducer

下载: 全尺寸图片幻灯片

图 5 谐波减速器精度测试平台

Figure 5. Precision test platform of harmonic reducer

下载: 全尺寸图片幻灯片

图 6 谐波减速器输入转速

Figure 6. Speed of harmonic reducer input

下载: 全尺寸图片幻灯片

图 7 谐波减速器输出转速

Figure 7. Speed of harmonic reducer output

下载: 全尺寸图片幻灯片

图 8 谐波减速器动态误差

Figure 8. Dynamic error of harmonic reducer

下载: 全尺寸图片幻灯片

图 9 静态误差和动态误差曲线

Figure 9. Static error and dynamic error curves

下载: 全尺寸图片幻灯片

图 10 不同扭转刚度k₁时动态误差变化曲线

Figure 10. Dynamic error curves at different torsional stiffness k₁

下载: 全尺寸图片幻灯片

图 11 不同输出轴转动惯量J_l时动态误差变化曲线

Figure 11. Dynamic error curves at different output shaft moment of inertia J_l

下载: 全尺寸图片幻灯片

图 12 不同柔轮切向相邻齿综合误差Δf′_f1时动态误差变化曲线

Figure 12. Dynamic error curves at different flexible wheel tangential adjacent gear comprehensive error Δf′_f1

下载: 全尺寸图片幻灯片

图 13 2种方法动态误差均值比较

Figure 13. Dynamic error mean comparison between two methods

下载: 全尺寸图片幻灯片

图 14 2种方法动态误差均方差比较

Figure 14. Dynamic error's mean square error comparison between two methods

下载: 全尺寸图片幻灯片

图 15 动态误差的Monte Carlo仿真曲线

Figure 15. Monte Carlo simulation curves of dynamic error

下载: 全尺寸图片幻灯片

表 1 谐波减速器动力学模型参数

Table 1. Parameters of harmonic reducer dynamic model

参数	数值
J_m/(kg·m²)	3.2×10^-4
J_l/(kg·m²)	8.5×10^-4
B_m/(N·m·s·rad^-1)	1.7×10^-4
B_l/(N·m·s·rad^-1)	5.0×10^-4
B_sp/(N·m·s·rad^-1)	2.8×10^-4
k₁/(N·m·rad^-1)	7 160
k₂/(N·m·rad^-3)	21 576
N	90

下载: 导出CSV

表 2 静态误差模型参数

Table 2. Parameters of static error model

参数	数值
Z₂	182
Z₁	180
α_n/(°)	20
E_f/m	3.1×10^-5
E_c/m	1.78×10^-4
E_b/m	2.05×10^-4
ΔF_p2/m	3.6×10^-5
Δf′_f2/m	1.14×10^-4
ΔF_p1/m	3.6×10^-5
Δf′_f1/m	1.14×10^-4

下载: 导出CSV

表 3 不同电机输入角下动态误差实验值与仿真值

Table 3. Experimental values and simulation values of dynamic errors under different motor input corners

电机输入角/(°)	动态误差实验值/(°)	动态误差仿真值/(°)	真实误差/ (°)	相对误差/%
50	0.023 473	0.021 908	0.001 565	4.7
100	0.018 379	0.017 472	0.000 907	4.9
200	0.016 727	0.015 486	0.001 241	7.4
300	0.018 503	0.017 256	0.001 247	6.7
400	0.016 205	0.017 369	0.001 164	7.1
500	0.019 181	0.018 688	0.000 493	2.5
700	0.017 800	0.019 320	0.002 820	8.5
800	0.019 794	0.017 913	0.001 881	9.5
1 000	0.020 950	0.022 243	0.001 293	4.1

下载: 导出CSV

表 4 谐波减速器不确定性参数及分布

Table 4. Uncertainty parameters and distribution of harmonic reducer

参数	分布	均值	标准差
B_m	正态分布	1.7×10^-4 N·m·s·rad^-1	0.000 03
J_l	正态分布	8.5×10^-4kg·m²	0.000 05
k₁	正态分布	7.16×10³ N·m·rad^-1	200
k₂	正态分布	2.157 6×10⁴ N·m·rad^-3	500
B_sp	正态分布	2.8 ×10^-4 N·m·s·rad^-1	0.000 04
E_f	正态分布	3.1 ×10^-5 m	0.000 005
E_c	正态分布	1.78 ×10^-4 m	0.000 04
E_b	正态分布	2.05 ×10^-4 m	0.000 05
Δf′_f1	正态分布	1.14×10^-4 m	0.000 04

下载: 导出CSV

表 5 动态精度多项式混沌展开式配点

Table 5. Collocation of dynamic accuracy polynomial chaos expansion

参数	多项式混沌展开配点
B_m	(0.000 187，0.000 17，0.000 152)
J_l	(0.000 879，0.000 85，0.000 821)
k₁	(0.000 721 8，0.000 716 0，0.000 710 2)
k₂	(0.020 999，0.021 576，0.022 153)
B_sp	(0.000 303，0.000 28，0.000 257)
E_f	(0.000 033 8，0.000 031，0.000 028 1)
E_c	(0.000 201，0.000 178，0.000 154)
E_b	(0.000 176 3，0.000 205，0.000 233 7)
Δf′_f1	(0.000 116 9，0.000 14，0.000 163 1)

下载: 导出CSV

表 6 动态精度多项式混沌展开式系数

Table 6. Polynomial chaotic expansion coefficient of dynamic accuracy

项	系数
1	0.012 5
ξ₁	0.032 8
ξ₂	-0.154 0
ξ₃	0.357 6
ξ₄	-0.009 3
ξ₅	0.000 9
ξ₆	0.014 0
ξ₇	0.074 9
ξ₈	0.007 2
ξ₉	0.102 3

下载: 导出CSV

表 7 动态误差多项式混沌展开式系数

Table 7. Polynomial chaos expansion coefficients of dynamic error

项	系数
1	0.024 151
ζ₁	-0.236 1
ζ₂	0.036 6
ζ₃	0.720 9
ζ₄	-0.023 6
ζ₅	-0.021 8
ζ₁-1	0.0365
ζ₂-1	-0.000 8
ζ₃-1	0.049 0
ζ₄-1	-0.000 2
ζ₅-1	-0.001 5
ζ₁ζ₂	0.003 7
ζ₁ζ₃	-0.036 5
ζ₁ζ₄	0.000 6
ζ₁ζ₅	-0.005 3
ζ₂ζ₃	-0.005 4
ζ₂ζ₄	0.000 1
ζ₂ζ₅	0.001 5
ζ₃ζ₄	0.003 9
ζ₃ζ₅	0.004 2
ζ₄ζ₅	-0.000 5

下载: 导出CSV

表 8 FORM法可靠度计算结果

Table 8. Reliability calculation results based on FORM method

迭代次数	β	可靠度
1	2.783	0.997 3
2	1.782	0.962 6
3	1.737	0.958 8
4	1.732	0.958 4
5	1.732	0.958 4

下载: 导出CSV

表 9 Monte Carlo仿真实验估算失效概率

Table 9. Failure probability estimation under Monte Carlo simulation experiment

n_t	n_f	P_f
500	14	0.028 0
2 000	61	0.030 5
4 000	126	0.031 5
6 000	205	0.034 2
7 000	254	0.036 3
8 000	305	0.038 1
10 000	381	0.038 1

下载: 导出CSV

参考文献(18)

[1]	NYE T, KRAML R. Harmonic drive gear error: Characterization and compensation for precision pointing and tracking[C]//Processing of the 25th Aerospace Mechanics, Symposium. Washington, D. C. : NASA, 1991: 237-252.
[2]	EMELYANOV A F.Calculation of the kinematic error of a harmonic gear transmission taking into account the compliance of elements[J].Soviet Engineering Research, 1983, 3(7):7-10.
[3]	GRAVAGNO F, MUCINO V H, PENNESSTRI E.Influence of wave generator profile on pure kinematic error and centrodes of harmonic drive[J].Mechanism and Machine Theory, 2016, 104:100-107. doi: 10.1016/j.mechmachtheory.2016.05.005
[4]	沙晓晨, 范元勋.谐波减速器传动误差的研究[J].机械制造, 2015, 44(5):50-54. http://www.cnki.com.cn/Article/CJFDTotal-ZZHD201505013.htm SHA X C, FAN Y X.Study of transmission error of harmonic drive reducer[J].Machine Building Automation, 2015, 44(5):50-54(in Chinese). http://www.cnki.com.cn/Article/CJFDTotal-ZZHD201505013.htm
[5]	HSIA L M. The analysis and design of harmonic gear drives[C]//Processing of the 1988 IEEE International Conference on Systems, Man, and Cybernetics. Piscataway, NJ: IEEE Press, 1988: 616-620.
[6]	TUTTLE T, SEERING W. Kinematic error, compliance, and friction in a harmonic drive gear transmission[C]//ASME Design Technical Conferences 19th Design Automation. New York: ASME, 1993: 319-324.
[7]	游斌弟, 赵阳.考虑非线性因素的谐波齿轮传动动态误差研究[J].宇航学报, 2010, 31(5):1297-1282. http://d.wanfangdata.com.cn/Periodical_yhxb201005004.aspx YOU B D, ZHAO Y.Study on dynamic error of harmonic drive with nonlinear factors[J].Journal of Astronautics, 2010, 31(5):1297-1282(in Chinese). http://d.wanfangdata.com.cn/Periodical_yhxb201005004.aspx
[8]	PREISSNER C, ROYSTON T J, SHU D.A high-fidelity harmonic drive model[J].Journal of Dynamic Systems, Measurement, and Control, 2012, 134(1):011002. doi: 10.1115/1.4005041
[9]	KIRCANSKI N, GOLDENBERG A, ANGELS J. Nonlinear modeling and parameter identification of harmonic drive gear transmissions[C]//Processing of the 32nd IEEE Conference on Robotics and Automatic. Piscataway, NJ: IEEE Press, 1995: 3027-3032.
[10]	王爱东. 机器人用谐波齿轮传动装置的运动精度分析[D]. 北京: 中国科学院, 2001: 11-15. WANG A D. Kinematic accuracy analysis of gear transmission for harmonic reducer of robot[D]. Beijing: Chinese Academy of Sciences, 2001: 11-15(in Chinese).
[11]	FATHI H, PRASANNA S, FRIEDHELM A.On the kinematic error in harmonic drive gears[J].Journal of Mechanical Design, 2001, 123(1):90-97. doi: 10.1115/1.1334379
[12]	WIENER N.The homogeneous chaos[J].American Journal of Mathematics, 1938, 60(4):897-936. doi: 10.2307/2371268
[13]	赵珂, 高正红, 黄江涛, 等.基于PCE方法的翼型不确定性分析及稳健设计[J].力学学报, 2014, 46(1):11-19. http://www.cqvip.com/QK/91029X/201401/48361837.html ZHAO K, GAO Z H, HUANG J T, et al.Uncertainty quantification and robust design of airfoil based on polynomial chaos technique[J].Chinese Journal of Theoretical and Applied Mechanics, 2014, 46(1):11-19(in Chinese). http://www.cqvip.com/QK/91029X/201401/48361837.html
[14]	LOEVETT T, PONCI F, MONTI A.A polynomial chaos approach to measurement uncertainty[J].IEEE Transations on Instrumentation and Measurement, 2006, 55(3):729-736. doi: 10.1109/TIM.2006.873807
[15]	BENJAMIN L, HOSAM K, JEFFREY L.Recursive maximum likelihood parameter estimation for state space systems using polynomial chaos theory[J].Automatica, 2011, 47(11):2420-2424. doi: 10.1016/j.automatica.2011.08.014
[16]	陶海川, 来新民.基于Dymola的无刷直流电机仿真模型[J].计算机仿真, 2005, 22(5):63-65. doi: 10.3969/j.issn.1006-9348.2005.05.018 TAO H C, LAI X M. Computer simulation of brushless DC motor system based on Dymola[J].Computer Simulation, 2005, 22 (5):63-65(in Chinese). doi: 10.3969/j.issn.1006-9348.2005.05.018
[17]	SUDRET B.Global sensitivity analysis using polynomial chaos expansion[J].Reliability Engineering and System Safety, 2008, 93(7):964-979. doi: 10.1016/j.ress.2007.04.002
[18]	PENG W S, ZHANG J G, ZHU D T.ABCLS methods for high-reliability aerospace mechanism with truncated random uncertainties[J].Chinese Journal of Aeronautics, 2015, 28(4):1066-1075. doi: 10.1016/j.cja.2015.06.012