-
摘要:
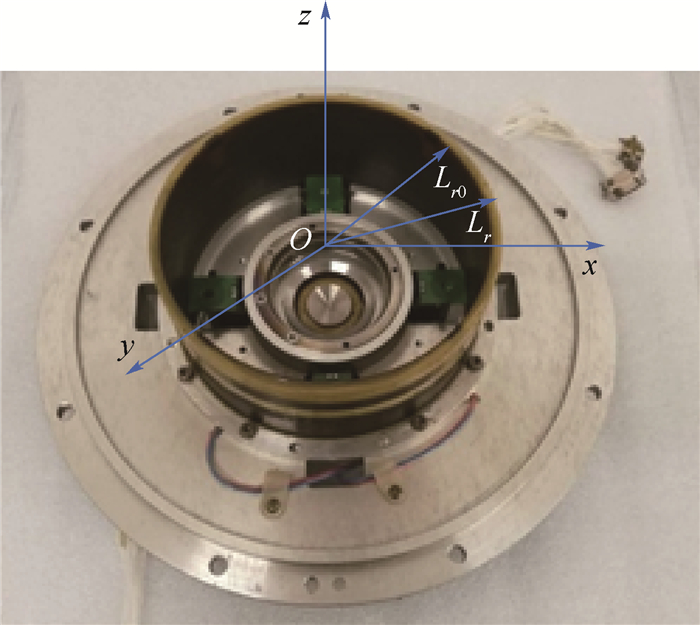
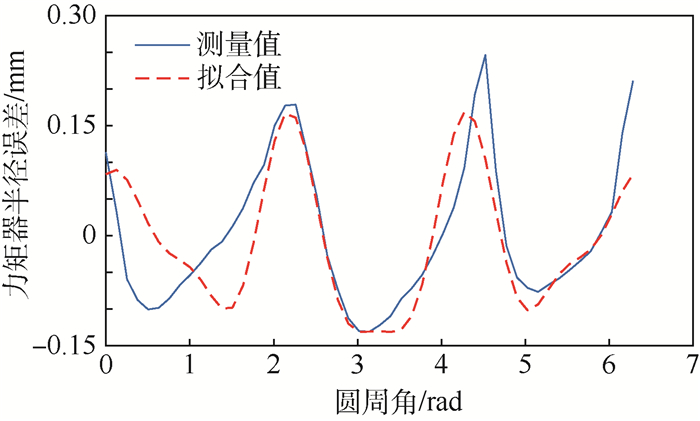
为提高磁悬浮控制敏感陀螺（MSCSG）对陀螺载体姿态的敏感精度，基于其洛伦兹力磁轴承（LFMB）的设计结构，提出了一种力矩器非圆性误差补偿方法。首先，针对一种新型双球形包络面转子MSCSG，介绍了MSCSG的结构特点与陀螺载体姿态角速度敏感原理，并分别建立了MSCSG力矩器半径误差模型、转子偏转干扰力矩模型与陀螺载体姿态角速度敏感误差模型。其次，通过实验测量了力矩器的圆度，通过MATLAB进行数据拟合得到了力矩器的非圆特性，采用勒让德多项式级数对力矩器非圆性进行了描述，并有效补偿了因力矩器非圆性误差导致的姿态角速度敏感误差。最后，对误差补偿效果进行了仿真验证，结果表明该补偿方法使陀螺载体姿态角速度敏感误差降低了83.5%。此外，本文方法还可以解决LFMB陀螺的相关共性问题。
-
关键词:
- 磁悬浮控制敏感陀螺(MSCSG) /
- 力矩器 /
- 洛伦兹力磁轴承(LFMB) /
- 姿态角速度敏感 /
- 非圆性误差补偿
Abstract:Based on the structural design of the Lorentz force magnetic bearing (LFMB), a kind of torquer's non-circular error compensation method is put forward to enhance the sensitivity accuracy of magnetically suspended control sensitive gyroscope (MSCSG) to gyro carrier attitude. First, for a new type MSCSG with double spherical envelope rotor, the structure features of MSCSG and the attitude angular velocity measurement principle of the gyro carrier are introduced and the radius error model of MSCSG torquer, the interference torque model of rotor's deflection and the measurement error model of the gyro carrier's attitude angular velocity are set up. Then, the roundness of torquer is measured through the experiment, and data fitting is conducted by MATLAB to obtain the non-circular characteristic of the torquer. The non-circular characteristic is described by Legendre polynomial series, and the measurement error of the gyro carrier's attitude angular velocity caused by the torquer's non-circular error is compensated effectively. Finally, the effect of error compensation is verified by the simulation and the results show that the compensation method makes the measurement error of the gyro carrier's attitude angular velocity reduce by 83.5%. In addition, the proposed method can solve the common problems related to LFMB gyro.
-
图 6 力矩器半径测量值随圆周角变化情况
Figure 6. Change of measured value of torquer radius with inscribed angle
图 7 干扰力矩测量值随圆周角变化
Figure 7. Change of measured value of disturbance torque with inscribed angle
图 9 MSCSG载体姿态角速度敏感系统框图
Figure 9. Block diagram of MSCSG carrier attitude angular velocity measurement system
表 1 仿真系统参数
Table 1. Parameters of simulation system
参数 取值 LFMB支架半径/mm 55.695 5 LFMB线圈匝数 200 LFMB线圈电流/A 1 LFMB磁场磁密/T 0.4 转子径向转动惯量/(kg·m2) 0.003 4 转子轴向转动惯量/(kg·m2) 0.005 2 LFMB线圈半张角/(°) 37 
下载: 导出CSV
-
[1] FANG J, WANG C, TANG J.Modeling and analysis of a novel conical magnetic bearing for vernier-gimballing magnetically suspended flywheel[J].ARCHIVE Proceedings of the Institution of Mechanical Engineers, Part C:Journal of Mechanical Engineering Science, 2013, 228(13):2416-2425. [2] HAN B, ZHENG S, WANG Z, et al.Design, modeling, fabrication, and test of a large-scale single-gimbal magnetically suspended control moment gyro[J].IEEE Transactions on Industrial Electronics, 2015, 62(12):7424-7435. doi: 10.1109/TIE.2015.2459052 [3] CUI P, CUI J, YANG Q, et al.The coupling characteristic investigation of double-gimbal magnetically suspended control moment gyro used on agile maneuver spacecraft[J].Mathematical Problems in Engineering, 2015, 2015:278145. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=Doaj000004222245 [4] REN Y, FANG J.Modified cross feedback control for a magnetically suspended flywheel rotor with significant gyroscopic effects[J].Mathematical Problems in Engineering, 2014, 2014:325913. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=Doaj000002936295 [5] BOLETIS A, BARROT F, MOSER R. Three axis active magnetic levitation for inertial sensing systems: US7252001[P]. 2007-08-07. [6] BOSGIRAUD T. Two degrees of freedom miniaturized gyroscope based on active magnetic bearings[D]. Lausanne: Ecole Polytechnique Federale de Lausanne, 2008: 1-15. [7] ZHANG H H.Measuring attitude rates through angular momentum gyros[J].Journal of Spacecraft & Rockets, 2012, 36(36):919-921. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ024240242/ [8] TYC G, STALEY D A, WHITEHEAD W R, et al. GyroWheel TM-An innovative new actuator/sensor for 3-axis spacecraft attitude control[C]//Proceedings of 13th Annual AIAA/USU Conference on Small Satellites. Reston: AIAA, 1999: 23-26. [9] WALKTY I, PETERSEN J, DOHERTY T, et al. SCISAT-1 ACE mission C&DH unit development[C]//Proceedings of 14th Annual AIAA/USU Conference on Small Satellites. Reston: AIAA, 2000: 1-13. [10] MARUYAMA Y, MIZUNO T, TAKASAKI M, et al.An application of active magnetic bearing to gyroscopic and inertial sensors[J].Journal of System Design & Dynamics, 2008, 2(1):155-164. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=Open J-Gate000000221330 [11] 刘彬, 房建成, 刘刚.一种磁悬浮陀螺飞轮方案设计与关键技术分析[J].航空学报, 2011, 32(8):1478-1487. http://d.old.wanfangdata.com.cn/Periodical/hkxb201108012LIU B, FANG J C, LIU G.Design of a magnetically suspended growheel and analysis of key technologies[J].Acta Aeronautica et Astronautica Sinica, 2011, 32(8):1478-1487(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/hkxb201108012 [12] 任元, 王卫杰, 刘强, 等. 一种磁悬浮控制敏感陀螺: ZL201510006597. 5[P]. 2017-04-28.REN Y, WANG W J, LIU Q, et al. A kind of magnetically suspended control sensitive gyroscope: ZL201510006597. 5[P]. 2017-04-28(in Chinese). [13] 刘强, 武登云, 樊亚洪, 等. 双定子三自由度解耦洛伦兹力磁轴承: CN204572783U[P]. 2015-06-24.LIU Q, WU D Y, FAN Y H, et al. Double stator three degrees of freedom decoupling Lorentz force magnetic bearing: CN204572783U[P]. 2015-06-24(in Chinese). [14] 刘强, 任元, 王卫杰, 等. 一种双永磁体内转子永磁偏置球面径向磁轴承:, CN204267528U[P]. 2015-02-25.LIU Q, REN Y, WANG W J, et al. A permanent magnet bias spherical radial magnetic bearing with double permanent magnets: CN204267528U[P]. 2015-02-25(in Chinese). [15] 辛朝军. 磁悬浮控制敏感陀螺误差分析与补偿方法研究[D]. 北京: 航天工程大学, 2017: 15.XIN C J. Error analysis and compensation method study of a magnetically suspended sensitive gyroscope[D]. Beijing: Space Engineering University, 2017: 15(in Chinese). [16] 许国锋, 蔡远文, 任元, 等.洛伦兹力磁轴承磁密均匀度设计与分析[J].北京航空航天大学学报, 2017, 43(3):559-566. http://bhxb.buaa.edu.cn/CN/abstract/abstract13947.shtmlXU G F, CAI Y W, REN Y, et al.Design and analysis on uniformity of magnetic flux density in Lorentz force-type magnetic bearing[J].Journal of Beijing University of Aeronautics and Astronautics, 2017, 43(3):559-566(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13947.shtml -
下载:
点击查看大图
计量
- 文章访问数: 678
- HTML全文浏览量: 82
- PDF下载量: 364
- 被引次数: 0
