一种电磁层析图像快速重建算法

刘泽; 肖君; 刘向龙; 赵鹏飞; 李勇; 霍继伟

doi:10.13700/j.bh.1001-5965.2017.0651

一种电磁层析图像快速重建算法

doi: 10.13700/j.bh.1001-5965.2017.0651

北京交通大学电子信息工程学院, 北京 100044

基金项目:

国家自然科学基金 61771041

详细信息

作者简介:
刘泽男, 博士, 教授。主要研究方向:过程参数检测、电磁层析成像

肖君女,硕士研究生。主要研究方向:电磁层析成像

通讯作者:
刘泽, E-mail: zliu@bjtu.edu.cn

中图分类号: TP23;TP212
计量
- 文章访问数: 701
- HTML全文浏览量: 101
- PDF下载量: 416
- 被引次数: 0
出版历程
- 收稿日期: 2017-10-23
- 录用日期: 2017-12-01
- 网络出版日期: 2018-08-20

An algorithm for fast reconstruction of electromagnetic tomography images

School of Electronic Information Engineering, Beijing Jiaotong University, Beijing 100044, China

Funds:

National Natural Science Foundation of China 61771041

More Information

Corresponding author: LIU Ze, E-mail: zliu@bjtu.edu.cn

摘要

摘要:
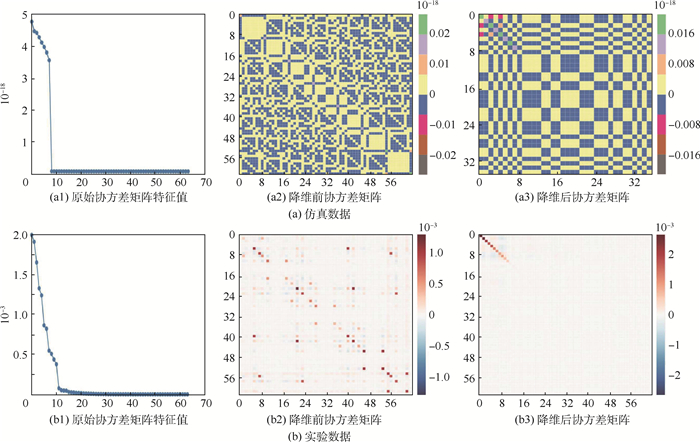
针对电磁层析成像（EMT）逆问题中，灵敏度矩阵的病态性、不适定性等问题，提出了一种新的电磁层析图像快速重建算法。利用主成分分析（PCA）对灵敏度矩阵做降维映射，再利用奇异值分解（SVD）求广义逆矩阵，重建图像。在选取灵敏度矩阵的协方差矩阵的特征值个数中，利用灵敏度矩阵特有的多样本特性，提出图像相关系数最大化算法，更加合理地去除灵敏度矩阵中的冗余信息，在尽可能不丢失成像特征信息的条件下，提高了解稳定性。实际采集数据成像时，该算法只需一次矩阵乘法运算，为快速实时成像提供了可能。与传统单步算法和迭代算法相比，该算法在成像质量和速度上都有较明显优势。
- 电磁层析成像(EMT) /
- 主成分分析(PCA) /
- 奇异值分解(SVD) /
- 图像相关系数最大化 /
- 降维
Abstract:
For the inverse problem of electromagnetic tomography (EMT), the pathological and ill posed problems of the sensitivity matrix are discussed. A new electromagnetic tomography image reconstruction algorithm is proposed for this situation. Firstly, the principal component analysis (PCA) is used to reduce the dimension of the sensitivity matrix, and then the singular value decomposition (SVD) is used to calculate the generalized inverse matrix to reconstruct the image. After the covariance matrix of the sensitivity matrix is obtained, we need to compute the number of eigenvalues that the covariance matrix should retain. Then the maximization of the image correlation coefficient algorithm is proposed to solve it by using the unique multi-sample characteristics of the sensitivity matrix. It is more reasonable for sensitivity matrix to remove redundant information. And it improves the stability of the solution as far as possible without losing imaging feature information. When the actual data is used for imaging, this algorithm needs only one matrix multiplication, which provides the possibility for fast real-time imaging. In conclusion, compared with the traditional single step algorithm and iterative algorithm, the proposed algorithm has obvious advantages in both imaging quality and speed.
- electromagnetic tomography (EMT) /
- principal component analysis (PCA) /
- singular value decomposition (SVD) /
- image correlation coefficient maximization /
- dimensionality reduction

HTML全文

图 1 仿真数据和实验数据的协方差矩阵及其特征值分布

Figure 1. Covariance matrix and eigenvalue distribution of simulation data and experimental data

下载: 全尺寸图片幻灯片

图 2 多样本的图像相关系数均值分布

Figure 2. Image correlation coefficient mean distribution with multi-sample

下载: 全尺寸图片幻灯片

图 3 电动平移台及其控制器

Figure 3. Electric moving stage and its controller

下载: 全尺寸图片幻灯片

图 4 8线圈传感器激励检测系统

Figure 4. 8-coil sensor excitation and detection system

下载: 全尺寸图片幻灯片

表 1 灵敏度矩阵降维前后多物体成像对比

Table 1. Multi-object imaging contrast before and after dimension reduction of sensitivity matrix

表 2 各算法多物体仿真成像对比

Table 2. Comparison of multi-algorithm and multi-object simulation imaging

表 3 仿真图像相关系数数据

Table 3. Image correlation coefficient data in simulation

样本类型	LBP法	Tikhonov 正则化法	Landweber 迭代算法	降维 SVD
1物体	-0.010 0	0.506 4	0.787 1	0.801 5
2物体	-0.007 0	0.595 4	0.761 9	0.688 5
3物体	-0.027 4	0.538 6	0.707 9	0.652 5
4物体	-0.040 6	0.424 5	0.644 9	0.606 1
5长物体	-0.026 5	0.279 6	0.319 4	0.326 4

下载: 导出CSV

表 4 仿真图像相对误差数据

Table 4. Image relative error data in simulation

样本类型	LBP法	Tikhonov 正则化法	Landweber 迭代算法	降维 SV
1物体	1.188 7	1.046 7	0.753 2	0.751 1
2物体	1.102 8	0.726 5	0.675 2	0.795 5
3物体	1.042 9	0.729 7	0.637 6	0.668 2
4物体	1.069 5	0.788 3	0.662 2	0.684 0
5长物体	3.422 0	0.886 5	0.868 8	0.875 2

下载: 导出CSV

表 5 各算法计算时间

Table 5. Calculation time of each algorithm

ms
样本类型	LBP法	Tikhonov 正则化法	Landweber 迭代算法	降维 SVD
1物体	1.1	0.4	200.1	0.2
2物体	1.2	0.4	306.4	0.2
3物体	1.1	0.4	440.4	0.2
4物体	1.2	0.3	280.8	0.2
5长物体	1.2	0.4	453.2	0.2

下载: 导出CSV

表 6 各算法实验成像对比

Table 6. Comparison of multi-algorithm experimental imaging

表 7 实验图像相关系数数据

Table 7. Image correlation coefficient data in experiment

样本类型	LBP法	Tikhonov 正则化法	Landweber 迭代算法	降维 SVD
1物体	0.531 6	0.612 2	0.652 3	0.647 5
2物体	0.453 4	0.543 3	0.608 4	0.599 0
3物体	0.467 1	0.519 1	0.587 9	0.590 4

下载: 导出CSV

表 8 实验图像相对误差数据

Table 8. Image relative error data in experiment

样本类型	LBP法	Tikhonov 正则化法	Landweber 迭代算法	降维 SVD
1物体	0.960 3	0.787 0	0.674 1	0.685 0
2物体	0.959 7	0.696 6	0.667 2	0.671 1
3物体	0.953 7	0.778 3	0.693 0	0.687 6

下载: 导出CSV

参考文献(19)

[1]	PEYTON A J, BACK M S, BORGES A R, et al. Development of electromagnetic tomography(EMT)for industrial applications. Part1: Sensor design and instrumentation[C]//1st World Congress on Industrial Process Tomography, 1999: 306-317.
[2]	RAMLI S, PEYTON A J. Feasibility study of planar-array electromagnetic inductance tomography(EMT)[C]//1st World Congress on Industrial Process Tomography, 1999: 502-510.
[3]	王化祥, 尹武良.用于多相界面检测系统的阵列式容栅电容传感器的优化设计[J].仪器仪表学报, 1996, 17(1):8-13. doi: 10.3321/j.issn:0254-3087.1996.01.002 WANG H X, YIN W L.Optimum design of an array of segmented capacitance sensor for multi-phase interface measuring system[J].Chinese Journal of Scientific Instrument, 1996, 17(1):8-13(in Chinese). doi: 10.3321/j.issn:0254-3087.1996.01.002
[4]	LIU C, XU L J, CHEN J L, et al.Development of a fan-beam TDLAS-based tomographic sensor for rapid imaging of temperature and gas concentration[J].Optics Express, 2015, 23(17):494-511. https://www.osapublishing.org/oe/abstract.cfm?URI=oe-23-17-22494
[5]	刘泽, 薛芳其, 杨国银, 等.电磁层析成像灵敏度矩阵实验测试方法[J].仪器仪表学报, 2013, 34(9):1982-1988. http://d.old.wanfangdata.com.cn/Periodical/yqyb201309010 LIU Z, XUE F Q, YANG G Y, et al.Experimental measurement method of sensitivity matrix for electromagnetic tomography[J].Chinese Journal of Scientific Instrument, 2013, 34(9):1982-1988(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/yqyb201309010
[6]	刘泽, 何敏, 徐苓安, 等.多激励模式的电磁层析成像系统[J].仪器仪表学报, 2001, 22(6):614-617. doi: 10.3321/j.issn:0254-3087.2001.06.019 LIU Z, HE M, XU L A, et al.Multi-mode excitation electromagnetic tomography (EMT) system[J].Chinese Journal of Scientific Instrument, 2001, 22(6):614-617(in Chinese). doi: 10.3321/j.issn:0254-3087.2001.06.019
[7]	彭黎辉, 陆耿, 杨五强.电容成像图像重建算法原理及评价[J].清华大学学报(自然科学版), 2004, 44(4):478-484. doi: 10.3321/j.issn:1000-0054.2004.04.013 PENG L H, LU G, YANG W Q.Image reconstruction algorithms for electrical capacitance tomography:State of the art[J].Journal of Tsinghua University(Science and Technology), 2004, 44(4):478-484(in Chinese). doi: 10.3321/j.issn:1000-0054.2004.04.013
[8]	吴杰, 李明峰, 余腾.测量数据处理中病态矩阵和正则化方法[J].大地测量和地球动力学, 2010, 30(4):102-105. http://d.old.wanfangdata.com.cn/Periodical/dkxbydz201004019 WU J, LI M F, YU T.Ill matrix and regularization method in surveying data processing[J].Journal of Geodesy and Geodynamics, 2010, 30(4):102-105(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/dkxbydz201004019
[9]	徐驰, 韩磊, 张书第, 等.最小二乘矩阵滤波器设计与性能分析[J].舰船科学技术, 2011, 33(4):72-76. doi: 10.3404/j.issn.1672-7649.2011.04.014 XU C, HAN L, ZHANG S D, et al.Design and performance analysis of least-square matrix filter[J].Ship Science and Technology, 2011, 33(4):72-76(in Chinese). doi: 10.3404/j.issn.1672-7649.2011.04.014
[10]	王友华.病态矩阵的本质及其解决方法[J].矿山测量, 2005(2):62-63. doi: 10.3969/j.issn.1001-358X.2005.02.024 WANG Y H.Essence of ill-conditioned matrix and corresponding solutions[J].Mine Surveying, 2005(2):62-63(in Chinese). doi: 10.3969/j.issn.1001-358X.2005.02.024
[11]	阮越, 陈汉武, 刘志昊, 等.量子主成分分析算法[J].计算机学报, 2014, 37(3):666-676. http://d.old.wanfangdata.com.cn/Periodical/jsjxb201403016 RUAN Y, CHEN H W, LIU Z H, et al.Quantum principal component analysis algorithm[J].Chinese Journal of Computers, 2014, 37(3):666-676(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/jsjxb201403016
[12]	周笋, 吉国力.基于改进BP算法的广义逆矩阵求解方法[J].厦门大学学报, 2003, 42(3):386-389. doi: 10.3321/j.issn:0438-0479.2003.03.027 ZHOU S, JI G L.Generalized inverse matrix method based on BP algorithm[J].Journal of Xiamen University, 2003, 42(3):386-389(in Chinese). doi: 10.3321/j.issn:0438-0479.2003.03.027
[13]	李高明, 李海鹏.求矩阵奇异值分解的一种新方法[J].数学实践与认识, 2011, 41(7):212-215. http://d.old.wanfangdata.com.cn/Periodical/sxdsjyrs201107028 LI G M, LI H P.A new methods of matrix singular values decomposition[J].Mathematics in Practice and Theory, 2011, 41(7):212-215(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/sxdsjyrs201107028
[14]	朱晓临, 李雪艳, 邢燕, 等.基于小波和奇异值分解的图像边缘检测[J].图学学报, 2014, 35(4):563-570. doi: 10.3969/j.issn.2095-302X.2014.04.012 ZHU X L, LI X Y, XING Y, et al.Image edge detection based on wavelet and singular value decomposition[J].Journal of Graphics, 2014, 35(4):563-570(in Chinese). doi: 10.3969/j.issn.2095-302X.2014.04.012
[15]	PENG L, YE J, LU G, et al.Evaluation of effect of number of electrodes in ECT sensors on image quality[J].IEEE Sensors Journal, 2012, 12(5):1554-1564. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=JJ0226557991
[16]	冯洁婷, 颜刚, 王涛, 等.Tikhonov正则化参数选择对高速率刺激听觉诱发电位重建的影响[J].航天医学与医学工程, 2012, 25(1):54-60. http://d.old.wanfangdata.com.cn/Periodical/htyxyyxgc201201012 FENG J T, YAN G, WANG T, et al.Effects of parameter selection of Tikhonov regularization on reconstruction of high-rate auditory evoked potentials[J].Space Medicine & Medical Engineering, 2012, 25(1):54-60(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/htyxyyxgc201201012
[17]	余瑞艳.基于混沌粒子群算法的Tikhonov正则化参数选取[J].数学研究, 2011, 44(1):101-106. http://d.old.wanfangdata.com.cn/Periodical/sxyj201101013 YU R Y.Optimal choosing of Tikhonov regularization parameter based on chaos particle swarm optimization algorithm[J].Journal of Mathematical Study, 2011, 44(1):101-106(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/sxyj201101013
[18]	唐利民, 朱建军.基于Landweber迭代的秩亏非线性最小二乘问题算法研究[J].大地测量与地球动力学, 2010, 30(1):95-98. http://d.old.wanfangdata.com.cn/Periodical/dkxbydz201001021 TANG L M, ZHU J J.Algorithm based on Landweber iteration for solving rank deficiency nonlinear least squares proble[J].Journal of Geodesy and Geodynamics, 2010, 30(1):95-98(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/dkxbydz201001021
[19]	王旭, 王静文, 王柯元.阈值Landweber在MIT图像重建中的应用[J].东北大学学报(自然科学版), 2016, 37(4):477-480. doi: 10.3969/j.issn.1005-3026.2016.04.005 WANG X, WANG J W, WANG K Y.Application of threshold-ing Landweber algorithm in MIT image reconstruction[J].Journal of Northeastern University (Natural Science), 2016, 37(4):477-480(in Chinese). doi: 10.3969/j.issn.1005-3026.2016.04.005