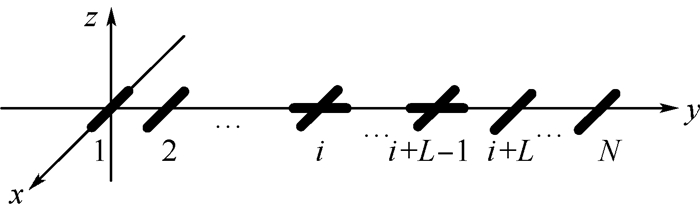Auxiliary polarization sensitive array beamforming based on non-circularity restoral
-
摘要:
为了降低极化敏感阵列（PSA）的应用成本，优化极化信息的利用效率，提高导向矢量失配条件下的滤波性能，提出了极化敏感辅助阵列（APSA）模型和基于二相编码信号非圆特征恢复的波束形成算法。在单极化线阵的基础上对部分阵元进行双极化改造，构成APSA；根据接收信号矢量的协方差阵和共轭协方差阵，按照非圆率最大准则，对构造的新协方差阵进行特征分解以确定权矢量，进而完成数字波束形成。详细分析了阵列模型的性能，并讨论了最小方差无失真响应（MVDR）算法和特征子空间投影（EP）算法。仿真结果表明，二相编码的非圆特征恢复算法不受导向矢量误差的影响，在阵列模型的基础上有主瓣干扰对抗能力，鲁棒性强。
-
关键词:
- 极化敏感阵列(PSA) /
- 非圆特征恢复 /
- 自适应波束形成 /
- 辅助阵列 /
- 二相编码
Abstract:In order to reduce the application cost of polarization sensitive array (PSA), optimize the utilization efficiency of polarization information and improve the filtering performance under the condition of steering vector mismatch, an auxiliary polarization sensitive array (APSA) model and a beamforming algorithm based on binary phase coded signal non-circularity restoral are proposed in this paper. In APSA, partial elements in single polarization linear array are turned into dual polarized elements; assuming that steering vector is unknown, the received signal covariance matrix and the conjugate covariance matrix are calculated, and a new covariance matrix is eigen-decomposed to determine the weight vector filtering in accordance with the non-circularity maximum criterion. This paper analyzes the performance of the array model, discusses the minimum variance distortionless response (MVDR) algorithm and the eigen-subspace projection (EP) algorithm, and makes a comparative analysis. The simulation results show that the non-circularity restoral algorithm of the binary phase coded signal will not be affected by the steering vector error, and it has excellent anti-jamming performance and strong robustness under the APSA model.
-
图 4 导向矢量空间角度误差对输出SINR的影响
Figure 4. Influence of steering vector angle error in space on output SINR
图 5 极化辅助阵元个数对输出SINR的影响
Figure 5. Influence of auxiliary polarization array element number on output SINR
图 6 干扰与目标极化差异对输出SINR的影响
Figure 6. Influence of interference polarization and target difference on output SINR
表 1 实验1详细参数设置
Table 1. Detailed parameter setting in Experiment 1
参数 信号 干扰1 干扰2 θ/(°) 15 14 17 γ/(°) 78 30 45 η/(°) 45 75 25 
下载: 导出CSV
-
[1] WEN D, MING D, GAO L, et al.A low-complexity DOA and polarization method of polarization-sensitive array[J].Sensors, 2017, 17(5):1170. doi: 10.3390/s17051170 [2] COMPTON R.On the performance of a polarization sensitive adaptive array[J].IEEE Transactions on Antennas and Propagation, 1981, 29(5):718-725. doi: 10.1109/TAP.1981.1142651 [3] WU D, XU Z, ZHANG L.Performance analysis of polarization-space-time three-domain joint processing for clutter suppression in airborne radar[J].Progress in Electromagnetics Research, 2012, 129:579-601. doi: 10.2528/PIER12052103 [4] LIU Z W, WANG Y X, ZHANG X R, et al.Spatially smoothed quaternion-Capon beamforming in the presence of coherent interferences[J].Transactions of Beijing Institute of Technology, 2016, 25(2):225-230. [5] LAN X, LIU W.Fully quaternion-valued adaptive beamforming based on crossed-dipole arrays[J].Electronics, 2017, 6(2):34. doi: 10.3390/electronics6020034 [6] JIANG M D, LIU W, LI Y.Adaptive beamforming for vector-sensor arrays based on a reweighted zero-attracting quaternion-valued LMS algorithm[J].IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2016, 63(3):274-278. doi: 10.1109/TCSII.2015.2482464 [7] DU L, LI J, STOICA P.Fully automatic computation of diagonal loading levels for robust adaptive beamforming[J].IEEE Transactions on Aerospace and Electronic Systems, 2010, 46(1):449-458. doi: 10.1109/TAES.2010.5417174 [8] LU L, LIAO Y.Improved algorithm of mainlobe interference suppression based on eigen-subspace[C]//International Conference on Communication and Signal Processing.Piscataway, NJ: IEEE Press, 2016: 133-137. [9] ZHANG X, LIU W, XU Y, et al.Quaternion-valued robust adaptive beamformer for electromagnetic vector-sensor arrays with worst-case constraint[J].Signal Processing, 2014, 104:274-283. doi: 10.1016/j.sigpro.2014.04.006 [10] 虞翔, 李旦, 张建秋.鲁棒成形极化敏感阵列波束的方法及极化估计[J].航空学报, 2017, 38(6):320752.YU X, LI D, ZHANG J Q, et al.A robust beamformer with a polarization sensitive array and polarization estimation[J].Acta Aeronautica et Astronautica Sinica, 2017, 38(6):320752(in Chinese). [11] SHERSON T, KLEIJN W B, HEUSDENS R.A distributed algorithm for robust LCMV beamforming[C]//IEEE International Conference on Acoustics, Speech and Signal Processing.Piscataway, NJ: IEEE Press, 2016: 101-105. [12] ZHANG X R, LIU Z W, XU Y G, et al.Adaptive tensorial beamformer based on electromagnetic vector-sensor arrays with coherent interferences[J].Multidimensional Systems and Signal Processing, 2015, 26(3):803-821. doi: 10.1007/s11045-014-0281-8 [13] 张贤达, 保铮.通信信号处理[M].北京:国防工业出版社, 2000:98-101.ZHANG X D, BAO Z.Communication signal processing[M].Beijing:National Defense Industry Press, 2000:98-101(in Chinese). [14] XU Y, MA J, LIU Z, et al.A class of diagonally loaded robust Capon beamformers for noncircular signals of interest[J].Signal Processing, 2014, 94:670-680. doi: 10.1016/j.sigpro.2013.07.013 [15] XU Y, YIN B, MA J, et al.High order noncircularity restoral diagonal loading robust adaptive beamforming: HNRDL[C]//Proceedings of 2015 International Radar Conference.Stevenage: IET, 2016: 3-8. [16] XIAO Y, YIN J, QI H, et al.MVDR algorithm based on estimated diagonal loading for beamforming[J].Mathematical Problems in Engineering, 2017, 2017:7904356. [17] HE Y, HONG Q, XIE H, et al.Energy error estimates of subspace method and multigrid algorithm for eigenvalue problems[J/OL].2017: 03038. [18] XU Y G, LIU T, LIU Z W.Output SINR of MV beamformer with one EM vector sensor of and magnetic noise power[C]//Proceedings of the International Conference on Signal Processing.Piscataway, NJ: IEEE Press, 2004: 419-422. [19] WANG W C, LIU C J, LIU F, et al.A new multi-beamforming method for large array[C]//2009 IEEE Radar Conference.Piscataway, NJ: IEEE Press, 2009: 1-4. [20] TAO J W.Performance analysis for interference and noise canceller based on hypercomplex and spatio-temporal-polarisation processes[J].IET Radar, Sonar and Navigation, 2013, 7(3):277-286. doi: 10.1049/iet-rsn.2012.0151 -
下载:
点击查看大图
计量
- 文章访问数: 699
- HTML全文浏览量: 129
- PDF下载量: 503
- 被引次数: 0
