基于代理模型的空间飞越发射窗口

向开恒; 李人杰; 陈杨

doi:10.13700/j.bh.1001-5965.2018.0264

基于代理模型的空间飞越发射窗口

doi: 10.13700/j.bh.1001-5965.2018.0264

1.
航天科工空间工程发展有限公司, 北京 100854
2.
北京电子工程总体研究所, 北京 100854

详细信息

作者简介:
向开恒  男, 博士, 研究员, 博士生导师。主要研究方向:航天器总体设计、航天器轨道动力学与控制

李人杰  男, 博士研究生。主要研究方向:航天器轨道动力学与控制

陈杨  男, 博士, 高级工程师。主要研究方向:航天器轨道动力学与控制

通讯作者:
向开恒, E-mail: xiangkaiheng@spacechina.com

中图分类号: V412.4
计量
- 文章访问数: 607
- HTML全文浏览量: 70
- PDF下载量: 291
- 被引次数: 0
出版历程
- 收稿日期: 2018-05-08
- 录用日期: 2018-07-28
- 网络出版日期: 2018-12-20

Launch window of space fly-by based on surrogate model

1.
CASIC Space Engineering Development Co., Ltd., Beijing 100854, China
2.
Beijing Institute of Electronic System Engineering, Beijing 100854, China

More Information

Corresponding author: XIANG Kaiheng, E-mail: xiangkaiheng@spacechina.com

摘要

摘要:
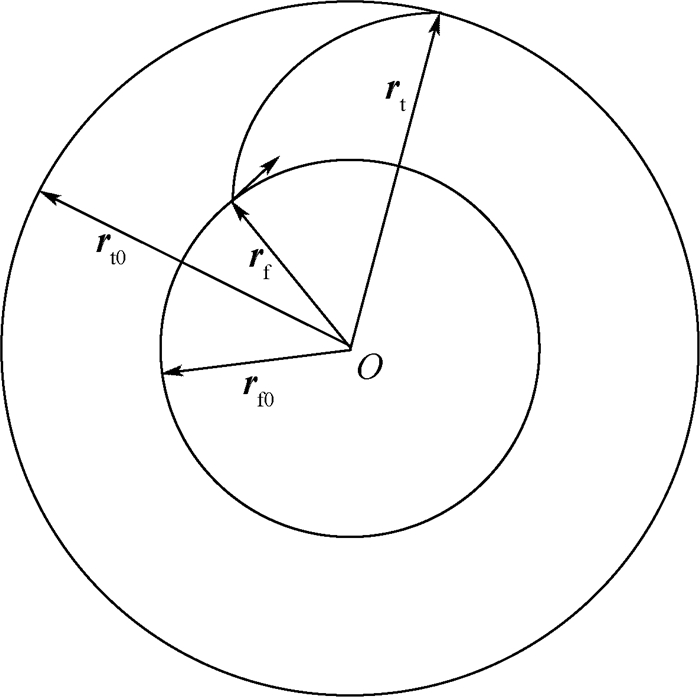
为了高效地分析空间飞越过程中航天器的初始位置对发射窗口的影响，研究了空间飞越任务流程，提出了不同初始条件下发射窗口的数值计算方法。在此基础上，为提高计算效率，研究了代理模型技术，包括样本点选取方法、代理模型构造方法和精度校验方法。对比分析了径向基函数（RBF）模型和Kriging模型，结果证明前者精度更高。使用RBF模型对不同初始条件下的发射窗口进行计算，耗时仅为使用真实模型时的0.29%，且精度校验满足要求，表明代理模型可以快速有效地分析初始条件对发射窗口的影响，为空间飞越轨道规划与设计提供理论依据和参考。
- 空间飞越 /
- 发射窗口 /
- 代理模型 /
- 试验设计 /
- 径向基函数(RBF)
Abstract:
In order to analyze the influence of initial positions on the launch window in space fly-by problem efficiently, the process of space fly-by was studied firstly in this paper. Then, a numerical method to calculate the launch window under different initial conditions was proposed. For improving calculation efficiency, surrogate model technology was studied, including sample points selection methods, surrogate models construction methods, and accuracy assessment methods. On the basis, two models, radial basis function (RBF) model and Kriging model, were compared. The results show that RBF model is more accurate for the problem in this paper. So it was applied to calculate launch windows under different conditions, which costs only 0.29% of the time that the true model costs, and the accuracy meets requirement. The results prove that using surrogate models can efficiently analyze the influence of initial conditions on the launch window, which will provide valuable theoretical foundation and reference for the orbital planning and design of space fly-by missions.
- space fly-by /
- launch window /
- surrogate model /
- design of tests /
- radial basis function (RBF)

HTML全文

图 1 空间飞越任务示意图

Figure 1. Schematic diagram of space fly-by mission

下载: 全尺寸图片幻灯片

图 2 空间飞越任务时序

Figure 2. Timing sequence of space fly-by mission

下载: 全尺寸图片幻灯片

图 3 发射窗口计算过程

Figure 3. Calculation process of launch window

下载: 全尺寸图片幻灯片

图 4 测试样本点分布

Figure 4. Distribution of test sample points

下载: 全尺寸图片幻灯片

图 5 真实模型和RBF模型的发射窗口长度等值线图

Figure 5. Contour map of launch window length of true model and RBF model

下载: 全尺寸图片幻灯片

图 6 平近点角相等时的发射窗口长度

Figure 6. Launch window length of equal mean anomalies

下载: 全尺寸图片幻灯片

图 7 可行的平近点角区间示意图

Figure 7. Schematic diagram of feasible region of mean anomaly

下载: 全尺寸图片幻灯片

图 8 任意一点的发射窗口分析

Figure 8. Analysis of launch window of one point

下载: 全尺寸图片幻灯片

图 9 发射窗口长度随时间的变化

Figure 9. Change of launch window length with time

下载: 全尺寸图片幻灯片

表 1 t₀时刻轨道根数

Table 1. Orbit elements at moment t₀

航天器 a/km e i/(°) Ω/(°) ω/(°)

飞越航天器 6 978 0.06 97.1 180 0

目标航天器 7 878 0.15 102.0 180 90

下载: 导出CSV

表 2 两种代理模型精度对比

Table 2. Accuracy comparison of two surrogate models

模型 R² RMSE

RBF模型 0.993 0.018

Kriging模型 0.952 0.049

下载: 导出CSV

表 3 代理模型和真实模型计算时间对比

Table 3. Comparison of calculation time between surrogate model and true model

s

模型
代理模型
构建时间发射窗口
计算时间总计算
时间

真实模型 57 688 57 688

RBF模型 3 473 168 3 641

下载: 导出CSV

参考文献(17)

[1]	田野.空间目标飞越式抵近观察制导方法研究[D].长沙: 国防科学技术大学, 2010: 1-2. TIAN Y.Study on guidance methods of fly-by approaching observation of space target[D].Changsha: National University of Defense Technology, 2010: 1-2(in Chinese).
[2]	黄江川, 王晓磊, 孟林智, 等.嫦娥二号卫星飞越4179小行星工程参数分析[J].中国科学:技术科学, 2013, 43(6):596-601. HUANG J C, WANG X L, MENG L Z, et al.Analysis of engineering parameters of CE-2 fly-by 4179 asteroid[J].Science China:Technology Science, 2013, 43(6):596-601(in Chinese).
[3]	李广华.近旁飞越航天器末制导方法研究[D].长沙: 国防科学技术大学, 2011: 1. LI G H.Study on terminal guidance of flyby spacecraft[D].Changsha: National University of Defense Technology, 2011: 1(in Chinese).
[4]	MATSUMOTO S, DUBOWSKY S, JACOBSEN S, et al.Fly-by approach and guidance for uncontrolled rotating satellite capture[C]//AIAA Guidance Navigation and Control Conference and Exhibit.Reston: AIAA, 2003: 1-10.
[5]	郝瑞, 孟云鹤, 郭胜鹏.多约束航天器飞越式接近的两级运动规划方法[J].上海航天, 2015, 32(3):16-21. doi: 10.3969/j.issn.1006-1630.2015.03.004 HAO R, MENG Y H, GUO S P.Two-stage motion planning method for fly-by approach of spacecraft with multiple constraints[J].Aerospace Shanghai, 2015, 32(3):16-21(in Chinese). doi: 10.3969/j.issn.1006-1630.2015.03.004
[6]	刘磊, 刘勇, 曹建峰, 等."嫦娥二号"探测小行星任务转移轨道设计[J].宇航学报, 2014, 35(3):262-268. doi: 10.3873/j.issn.1000-1328.2014.03.003 LIU L, LIU Y, CAO J F, et al.Mission design of the CHANG'E-2 asteroid exploration[J].Journal of Astronautics, 2014, 35(3):262-268(in Chinese). doi: 10.3873/j.issn.1000-1328.2014.03.003
[7]	闫循良, 廖守亿, 张金生, 等.天基发射方案与规划策略仿真研究[J].计算机仿真, 2013, 30(9):83-87. doi: 10.3969/j.issn.1006-9348.2013.09.019 YAN X L, LIAO S Y, ZHANG J S, et al.Space-based launch scheme and planning strategy simulation[J].Computer Simulation, 2013, 30(9):83-87(in Chinese). doi: 10.3969/j.issn.1006-9348.2013.09.019
[8]	王宇.基于不确定性的优化方法及其在飞机设计中的应用[D].南京: 南京航空航天大学, 2010: 18. WANG Y.Uncertainty-based optimization method and its application in aircraft design[D].Nanjing: Nanjing University of Aeronautics and Astronautics, 2010: 18(in Chinese).
[9]	王琦, 丁运亮, 陈昊.基于多级代理模型的优化算法[J].南京航空航天大学学报, 2008, 40(4):501-506. doi: 10.3969/j.issn.1005-2615.2008.04.017 WANG Q, DING Y L, CHEN H.Simulation optimization based on multilevel-surrogate models[J].Journal of Nanjing University of Aeronautics & Astronautics, 2008, 40(4):501-506(in Chinese). doi: 10.3969/j.issn.1005-2615.2008.04.017
[10]	周须峰, 唐硕, 高小五.空间目标可拦截区与可遭遇区确定[J].航天控制, 2006, 24(1):33-38. doi: 10.3969/j.issn.1006-3242.2006.01.008 ZHOU X F, TANG S, GAO X W.Determination of interceptable section and encounter section for space target[J].Aerospace Control, 2006, 24(1):33-38(in Chinese). doi: 10.3969/j.issn.1006-3242.2006.01.008
[11]	李人杰, 陈杨, 向开恒.考虑初始相位角影响的空间飞越发射窗口研究[J].现代防御技术, 2018, 46(3):171-176. LI R J, CHEN Y, XIANG K H.Study of launch window of space fly-by under influence of initial phase angle[J].Modern Defense Technology, 2018, 46(3):171-176(in Chinese).
[12]	王琦.MDO优化算法研究[D].南京: 南京航空航天大学, 2008: 39. WANG Q.Research on arithmetic of MDO[D].Nanjing: Nanjing University of Aeronautics and Astronautics, 2008: 39(in Chinese).
[13]	胡添元.飞翼布局飞机总体多学科设计优化研究[D].南京: 南京航空航天大学, 2010: 30. HU T Y.Multidisciplinary design optimization for flying wing aircraft preliminary design[D].Nanjing: Nanjing University of Aeronautics and Astronautics, 2010: 30(in Chinese).
[14]	龙腾, 刘建, WANG G G, 等.基于计算试验设计与代理模型的飞行器近似优化策略探讨[J].机械工程学报, 2016, 52(14):79-105. LONG T, LIU J, WANG G G, et al.Discuss on approximate optimization strategies using design of computer experiments and metamodels for flight vehicle design[J].Journal of Mechanical Engineering, 2016, 52(14):79-105(in Chineses).
[15]	姚雯.不确定性MDO理论及其在卫星总体设计中的应用[D].长沙: 国防科学技术大学, 2007: 27-28. YAO W.Research on uncertainty multidisciplinary design optimization theory and application to satellite system design[D].Changsha: National University of Defense Technology, 2007: 27-28(in Chinese).
[16]	LIANG B.Radial basis functions for solving PDEs[J].Mathematic Applicata, 2004, 17(2):227-233.
[17]	李靖.基于改进量子粒子群算法的运载火箭弹道优化[D].湘潭: 湘潭大学, 2014: 19-23. LI J.Launch vehicle trajectory optimization based on improved quantum particle swarm algorithm[D].Xiangtan: Xiangtan University, 2014: 19-23(in Chinese).