Influence of excitation and transition rates on ultraviolet radiation of thermal nonequilibrium nitrogen
-
摘要:
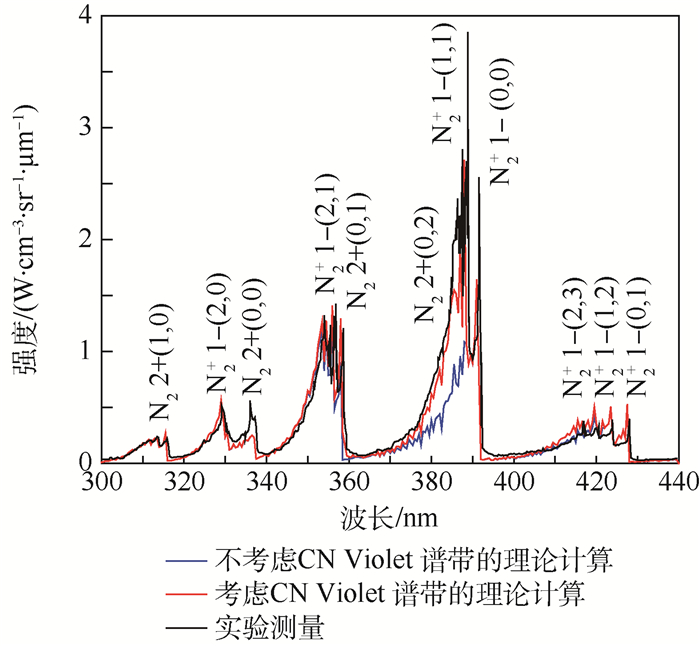
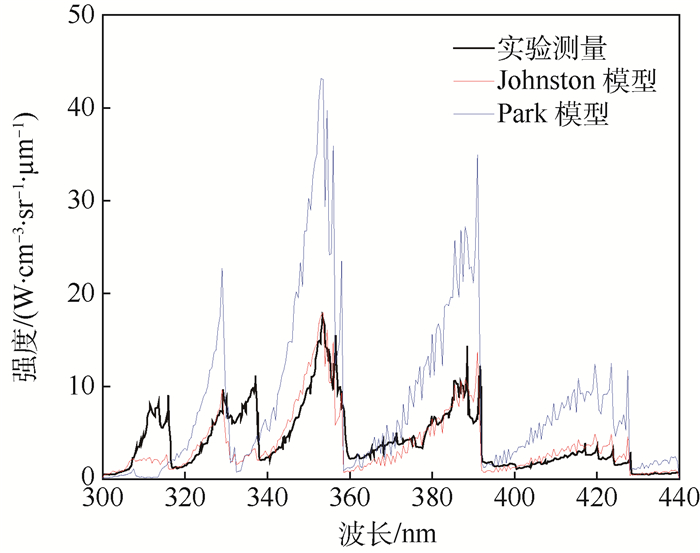
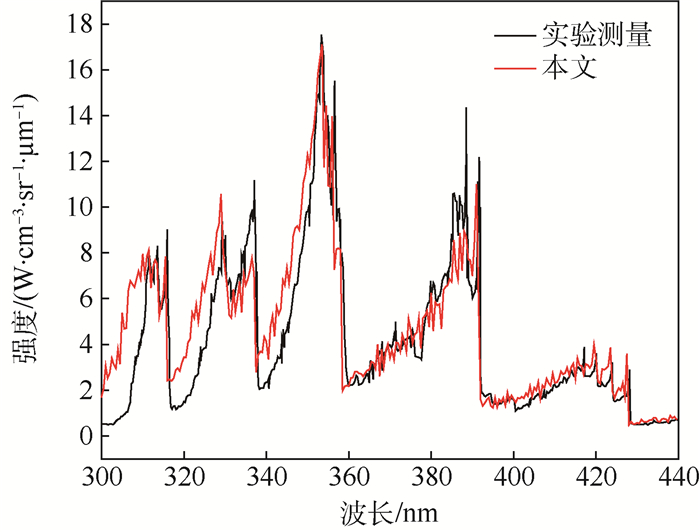
基于氮气的碰撞-辐射（CR）模型，计算了速度为6.2 km/s、初始压力为133 Pa的高超声速流动激波中N2和N2+分子电子能级的分布情况，分析了不同激发跃迁速率模型对电子能级分布及辐射光谱模拟的影响。针对流动中热力学非平衡区域和平衡区域，在300~440 nm的辐射光谱开展了逐线法的数值模拟，并与激波管实验测量光谱进行了对比。结果表明，目前的激发跃迁速率均存在偏差，综合Park模型的爱因斯坦系数和Johnston模型的碰撞激发速率可以得到与实验结果最为接近的辐射光谱。
-
关键词:
- 氮气 /
- 热力学非平衡 /
- 碰撞-辐射(CR)模型 /
- 紫外辐射 /
- 激发速率
Abstract:Based on the collision-radiation (CR) model of nitrogen, the distribution of the electronic energy levels of N2 and N2+ molecules in the hypersonic flow shock wave with velocity 6.2 km/s and initial pressure 133 Pa is calculated. The effects of different excitation and transition rate models on the electronic energy level distribution and the radiation spectrum simulation are analyzed. A line-by-line method is carried out to simulate the radiation spectrum of the thermal nonequilibrium region and the equilibrium region in the 300-440 nm, which are compared with the experimental measurement spectra of the shock tube. It is found that the current excitation and transition rates all have deviations, and the CR model that integrated the Einstein coefficient of Park model and the collision excitation rate of the Johnston model can gain the radiation spectrum which is the closest to the experimental results.
-
图 1 N2+(X-B)电子态的碰撞激发速率系数随温度的变化
Figure 1. Variation of electron collision excitation rate coefficient from N2+(X-B) state with temperature
图 2 平衡区测量与计算辐射光谱
Figure 2. Measured and calculated radiation spectra in equilibrium area
图 3 不同理论模型计算的非平衡区域辐射光谱与实验测量结果比较
Figure 3. Comparison of nonequilibrium radiation spectra calculated by different theoretical models with experimental measurements
图 4 采用修改模型计算的非平衡区域辐射光谱与实验测量结果比较
Figure 4. Comparison of radiation spectra of nonequilibrium area calculated by modified model with experimental measurement results
表 1 计算考虑电子能态
Table 1. Electronic states in calculation
能态 电子态 Ee/cm-1 简并度 N2 X1Σg+ 0 1 A3Σu+ 49754 3 B3Πu 59306 6 C3Πu 88977 6 N2+ X2Σg+ 0 2 A2Πu 9167 4 B2Σu+ 25461 4 C2Σu+ 64608 4 
下载: 导出CSV
表 2 不同理论模型计算的平衡区分子能级分布
Table 2. Molecular energy level distribution in equilibrium region calculated by different theoretical models
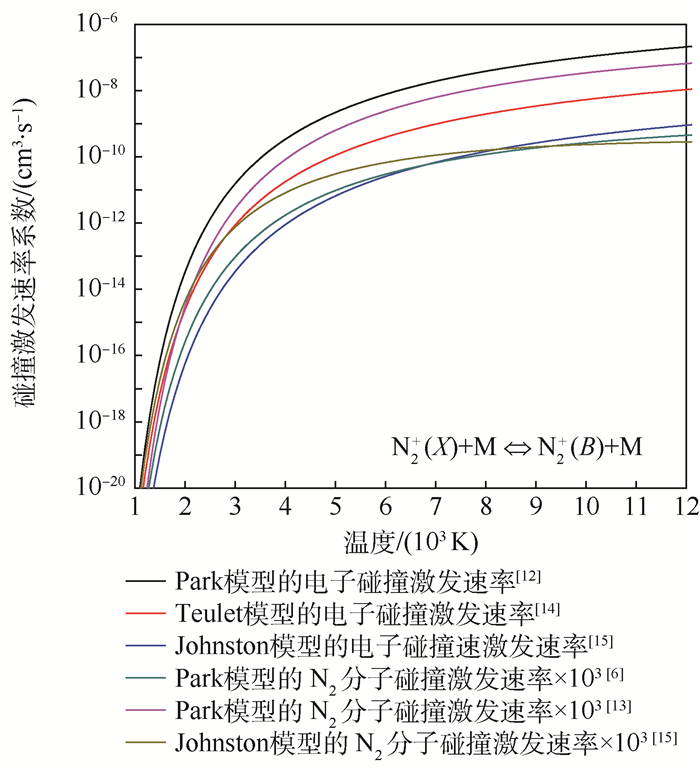
能态 Nie/cm-3 Park模型 Johnston模型 Ni/cm-3 Ni/Nie Ni/cm-3 Ni/Nie N2+(X) 1.36×1013 1.36×1013 1.00 1.36×1013 1.00 N2+(A) 4.68×1012 4.67×1012 1.00 4.68×1012 1.00 N2+(B) 4.03×1010 3.97×1010 0.99 2.35×1010 0.58 N2(X) 3.24×1017 3.24×1017 1.00 3.24×1017 1.00 N2(A) 3.64×1013 2.92×1013 0.80 3.66×1013 1.01 N2(B) 6.32×1012 5.11×1012 0.81 6.28×1012 0.99 N2(C) 2.55×1011 2.04×1011 0.80 2.52×1010 0.10
下载: 导出CSV
表 3 不同模型碰撞激发速率系数的计算结果
Table 3. Calculation results of collision excitation rate coefficient by different models
cm3·s-1 N2+反应过程 碰撞激发速率系数 N2反应过程 碰撞激发速率系数 Park模型 Johnston模型 Park模型 Johnston模型 N2+(X)+e－⇔N2+(A)+e－ 2.9×10-7 1.7×10-11 N2(X)+e－⇔N2(A)+e－ 9.1×10-13 1.4×10-12 N2+(X)+e－⇔N2+(B)+e－ 4.9×10-8 3.2×10-10 N2(X)+e－⇔N2(B)+e－ 3.3×10-13 4.7×10-13 N2+(X)+e－⇔N2+(C)+e－ 3.6×10-9 3.2×10-11 N2(X)+e－⇔N2(C)+e－ - 1.3×10-15 N2+(A)+e－⇔N2+(B)+e－ 8.8×10-7 8.1×10-11 N2(A)+e－⇔N2(B)+e－ 2.4×10-9 9.4×10-10 N2+(A)+e－⇔N2+(C)+e－ 2.7×10-8 8.3×10-11 N2(A)+e－⇔N2(C)+e－ - 1.1×10-10 N2+(B)+e－⇔N2+(C)+e－ 2.5×10-7 4.3×10-10 N2(B)+e－⇔N2(C)+e－ - 6.3×10-9 N2+(X)+e－⇔N+N++e－ 4.2×10-12 1.6×10-13 N2(X)+e－⇔N+N++e－ 1.2×10-16 4.1×10-15 N2+(A)+e－⇔N+N++e－ 1.5×10-11 1.7×10-12 N2(A)+e－⇔N+N++e－ 3.8×10-13 1.7×10-10 N2+(B)+e－⇔N+N++e－ 1.1×10-10 3.2×10-12 N2(B)+e－⇔N+N++e－ 1.6×10-12 1.8×10-11 N2+(C)+e－⇔N+N++e－ 3.9×10-9 1.0×10-10 N2(C)+e－⇔N+N++e－ - 6.3×10-9 N2+(X)+M⇔N2+(A)+M 1.1×10-12 3.0×10-12 N2(X)+M⇔N2(A)+M 5.6×10-14 2.5×10-17 N2+(X)+M⇔N2+(B)+M 4.1×10-13 1.9×10-13 N2(X)+M⇔N2(B)+M 1.7×10-14 0 N2+(X)+M⇔N2+(C)+M 3.2×10-14 0 N2(X)+M⇔N2(C)+M - 0 N2+(A)+M⇔N2+(B)+M 8.1×10-13 0 N2(A)+M⇔N2(B)+M 5.8×10-12 4.5×10-12 N2+(A)+M⇔N2+(C)+M 8.2×10-14 0 N2(A)+M⇔N2(C)+M - 0 N2+(B)+M⇔N2+(C)+M 3.8×10-13 0 N2(B)+M⇔N2(C)+M - 1.5×10-12 N2+(X)+M⇔N+N++M 8.8×10-14 6.9×10-18 N2(X)+M⇔N+N++M 4.6×10-15 0 N2+(A)+M⇔N+N++M 3.6×10-13 2.1×10-16 N2(A)+M⇔N+N++M 3.2×10-11 0 N2+(B)+M⇔N+N++M 4.4×10-12 5.3×10-16 N2(B)+M⇔N+N++M 1.7×10-10 0 N2+(C)+M⇔N+N++M 2.5×10-10 1.4×10-14 N2(C)+M⇔N+N++M - 0
下载: 导出CSV
表 4 不同理论模型计算的非平衡区分子电子能级数密度
Table 4. Molecular electron energy level density in nonequilibrium region calculated by different theoretical models
能态 Nie/cm-3 Park模型 Johnston模型 Ni/cm-3 Ni/Nie Ni/cm-3 Ni/Nie N2+(X) 1.22×1014 1.12×1014 0.92 1.23×1014 1.01 N2+(A) 6.31×1013 6.31×1013 1.00 6.23×1013 0.99 N2+(B) 1.13×1012 1.13×1012 1.00 3.96×1011 0.35 N2(X) 4.33×1017 4.44×1017 1.03 4.33×1017 1.00 N2(A) 4.56×1014 4.43×1013 0.10 3.99×1014 0.88 N2(B) 1.21×1014 3.64×1012 0.03 1.05×1014 0.87 N2(C) 7.47×1012 5.05×1010 0.01 1.37×1012 0.18
下载: 导出CSV
表 5 修改后的速率模型计算的分子电子能级数密度
Table 5. Molecular energy level density calculated by modified rate model
能态 平衡区 非平衡区 Nie/cm-3 Ni/cm-3 Ni/Nie Nie/cm-3 Ni/cm-3 Ni/Nie N2+(X) 1.36×1013 1.36×1013 1.00 1.22×1014 1.23×1014 1.01 N2+(A) 4.68×1012 4.68×1012 1.00 6.31×1013 6.23×1013 0.99 N2+(B) 4.03×1010 2.35×1010 0.58 1.13×1012 3.96×1011 0.35 N2(X) 3.24×1017 3.24×1017 1.00 4.33×1017 4.33×1017 1.00 N2(A) 3.64×1013 3.70×1013 1.02 4.56×1014 3.87×1014 0.85 N2(B) 6.32×1012 6.36×1012 1.01 1.21×1014 1.01×1014 0.83 N2(C) 2.55×1011 2.66×1011 1.04 7.47×1012 5.22×1012 0.70
下载: 导出CSV
-
[1] PARK C.Review of chemical kinetic problems for future NASA missions, Ⅰ.Earth entries[J].Journal of Thermophysics and Heat Transfer, 1993, 7(3):385-398. doi: 10.2514/3.431 [2] PARK C, HOWE J T, JAFFE R L, et al.Review of chemical-kinetic problems of future NASA missions, Ⅱ:Mars entries[J].Journal of Thermophysics and Heat Transfer, 1994, 8(1):9-23. doi: 10.2514-3.431/ [3] ANNALORO J, BULTEL A, OMALY P.Elaboration of a collisional-rediative model for CO2-N2-Ar plasma flows: Appliction to atmospheric Martian entries[C]//42nd AIAA Thermophysics Conference.Reston: AIAA, 2011: 3954. [4] CRUDEN B A, PRABHU D, MARTINEZ R, et al.Absolute radiation measurement in Venus and Mars entry conditions[C]//10th AIAA/ASME Joint Thermophysics and Heat Transfer Conference.Reston: AIAA, 2010: 092407. [5] LAUX C O, PIERROT L, GESSMAN R J.State-to-state modeling of a recombining nitrogen plasma experiment[J].Chemical Physics, 2012, 398(1):46-55. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=f67635d66f6d5f05229af6ef35fc3bb0 [6] PARK C.Calculation of nonequilibrium radiation in the flight regimes of aeroassisted orbital transfer vehicles[C]//AIAA 22nd Aerospace Sciences Meeting.Reston: AIAA, 1984: 1-13. [7] LEMAL A, JACOBS C M, PERRIN M Y, et al.Simulation of shock tube radiation measurements with a collisional-radiaitve model[C]//51st AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aeroespace Exposition.Reston: AIAA, 2013: 1-9. [8] LEE E S, PARK C, CHANG K S.Shock-tube determination of CN formation rate in a CO-N2 mixture[C]//45th AIAA Aerospace Sciences Meeting and Exhibit.Reston: AIAA, 2007: 1-13. [9] PANESI M, MAGIN T E, BOURDON A, et al.Analysis of the FIRE Ⅱ flight experiment by means of a collisional radiative model[C]//46th AIAA Aerospace Sciences Meeting and Exhibit.Reston: AIAA, 2008: 1-15. [10] SHARMA S P, GILLESPIE W.Nonequilibirum and equilibrium shock front radiation measurements[J].Journal of Thermophysics and Heat Transfer, 1991, 5(3):257-265. doi: 10.2514/3.259 [11] PARK C.Nonequilibrium hypersonic aerothermodynamics[M].New York:Wiley, 1989. [12] PARK C.Rate parameters for electronic excitation of diatomic Molecules Ⅰ.Electron-impact processes[C]//46th AIAA Aerospace Sciences Meeting and Exhibit, Aerospace Sciences Meetings.Reston: AIAA, 2008: 1206. [13] PARK C.Rate parameters for electronic excitation of diatomic Molecules Ⅱ.Heavy particle-impact processes[C]//46th AIAA Aerospace Sciences Meeting and Exhibit, Aerospace Sciences Meetings.Reston: AIAA, 2008: 1446. [14] TEULET P, SARRETTE J P, GOMES A M.Calculation of electron impact inelastic cross sections and rate coefficients for diatomic molecules.application to air molecules[J].Journal of Quantitative Spectroscopy and Radiative Transfer, 1999, 62(5):549-569. doi: 10.1016/S0022-4073(98)00129-0 [15] JOHNSTON C O.Nonequilibrium shock-layer radiative heating for earth and titan entry[D].Blacksburg: Virginia Polytechnic Institute and State University, 2006: 116-126. https://www.researchgate.net/publication/253796496_Nonequilibrium_shock-layer_radiative_heating_for_Earth_and_Titan_entry [16] 吴杰, 董雁冰.热力学非平衡分子辐射中的重粒子影响[J].红外与激光工程, 2012, 41(11):2981-2985. doi: 10.3969/j.issn.1007-2276.2012.11.023WU J, DONG Y B.Impact of heavy particles in radiation from thermally nonequilibrium molecules[J].Infrared and Laser Engineering, 2012, 41(11):2981-2985(in Chinese). doi: 10.3969/j.issn.1007-2276.2012.11.023 [17] ARNOLD J O, WHITING E E, LYLE G C.Line-by-line calculation of spectra from diatomic molecules and atoms assuming a voigt line profile[J].Journal of Quantitative Spectroscopy and Radiative Transfer, 1969, 9(6):775-798. doi: 10.1016/0022-4073(69)90075-2 -
下载:
点击查看大图
计量
- 文章访问数: 712
- HTML全文浏览量: 105
- PDF下载量: 424
- 被引次数: 0
