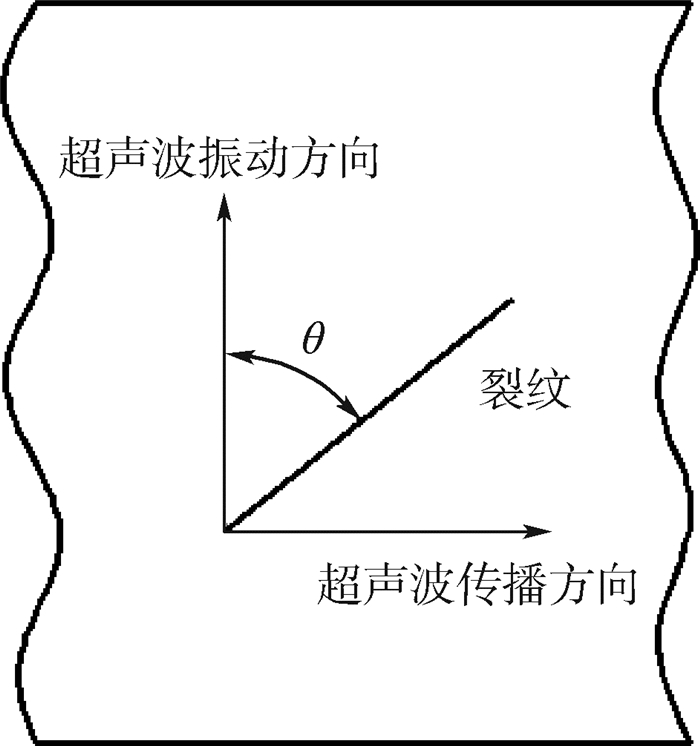
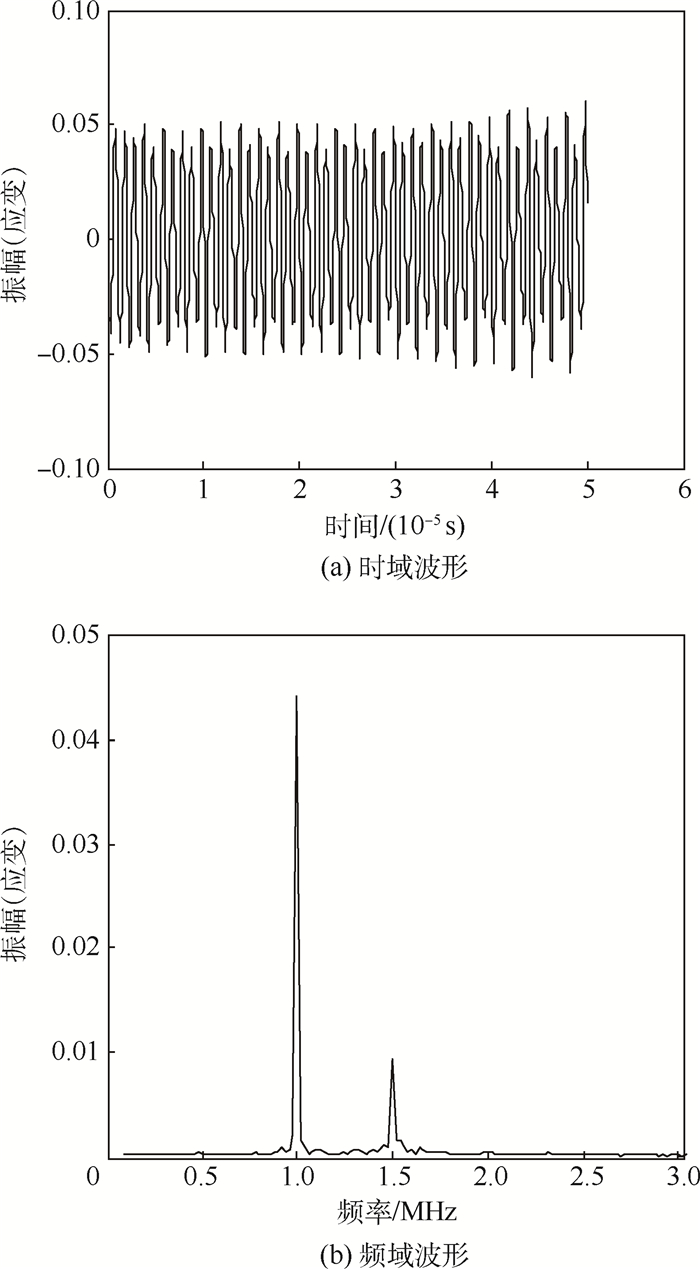
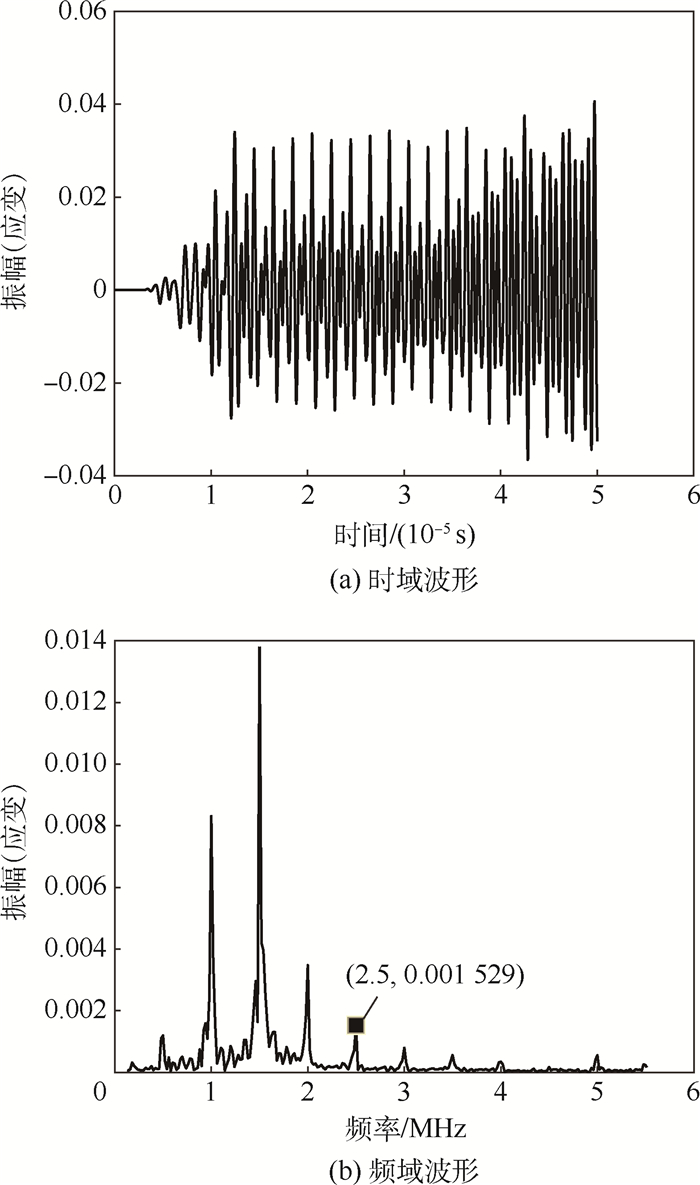
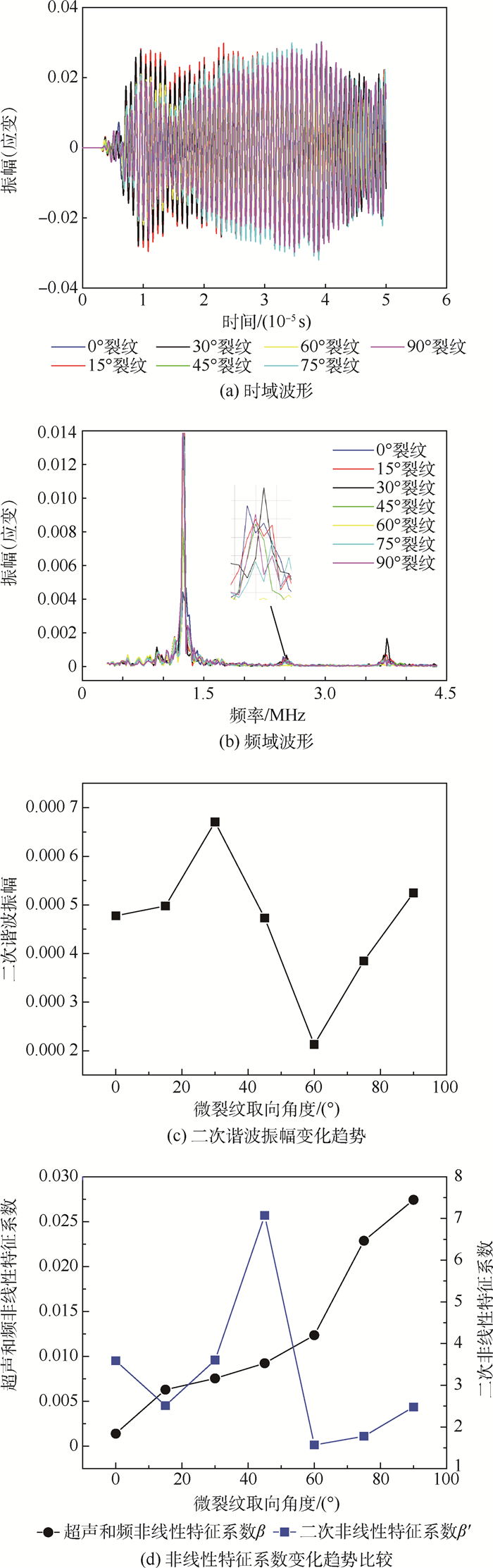
Sum frequency nonlinear effects of micro-crack orientation and ultrasound in metallic materials
-
摘要:
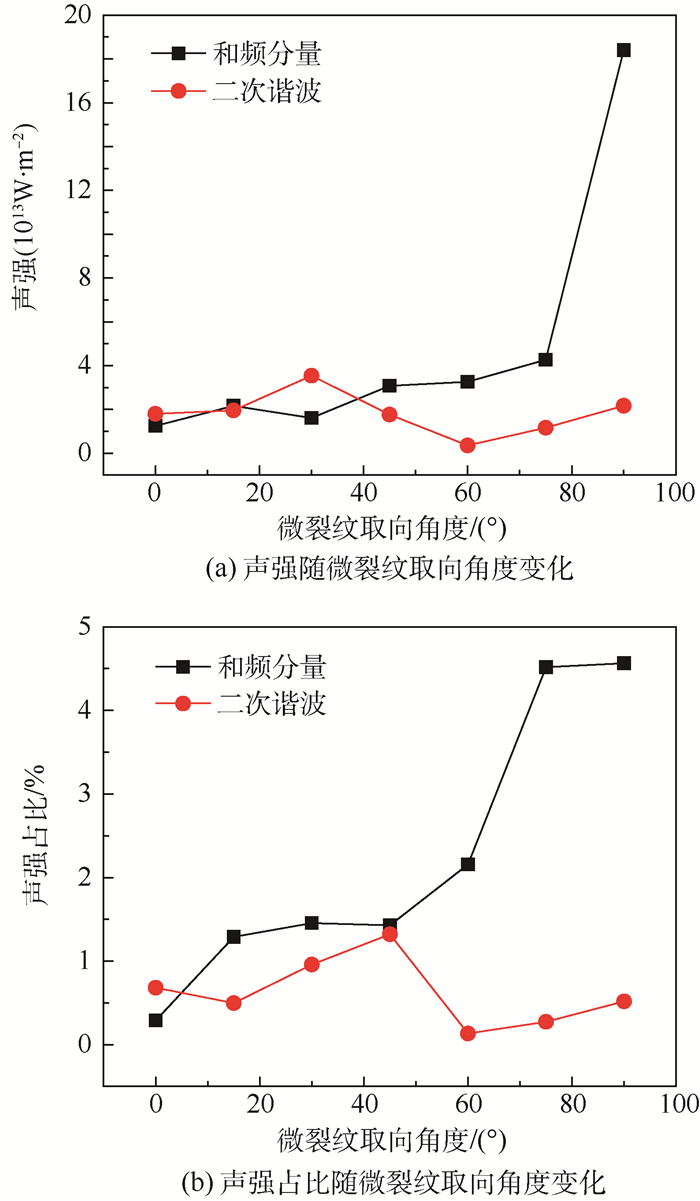
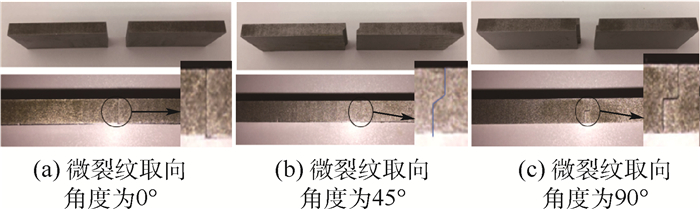
针对非线性超声无损检测金属材料微裂纹取向角度的问题，开展了微裂纹取向与超声波的和频非线性效应研究，建立了超声和频非线性特征系数与微裂纹取向角度的关系模型。理论和有限元仿真实验结果表明，随着微裂纹取向角度的逐渐增大，超声和频非线性特征系数与微裂纹取向角度之间呈现明显的正相关趋势，而且相比二次非线性特征系数，和频非线性特征系数对微裂纹取向检测更为敏感。同时，从超声波平均能流密度（即声强）的角度出发，计算可知和频分量声强会随着微裂纹取向角度的增大而增大，而二次谐波声强基本不会发生变化，同时和频分量声强占比相比于二次谐波声强占比也得到了明显提高。超声波声强计算结果与仿真计算结果趋势基本一致，证明了理论模型的正确性。通过实验验证了模型的有效性，为金属材料微裂纹取向的检测提供了一种有效的手段。
Abstract:In order to study the non-destructive testing of micro-crack orientation angle of metal materials, the research of the ultrasonic sum frequency nonlinear effect about the micro-crack orientation of metallic materials is carried out. In theory, the relationship between the ultrasonic nonlinear frequency characteristic coefficient and the orientation angle of micro-crack is established. Then, the results of finite element simulation and calculation show that with the gradual increase of the orientation angle of the micro-cracks, there is a clear positive correlation trend between the ultrasonic nonlinear frequency characteristic coefficient and the micro-crack orientation angle, and compared to the secondary nonlinear coefficient, the sum frequency nonlinear coefficient is more sensitive to micro-crack orientation detection. At the same time, from the perspective of the average ultrasonic wave energy density, for example, the sound intensity, the sound intensity of the sum frequency component will increase with the increase of the orientation angle of the micro-crack, and the sound intensity of the second harmonic component will not change substantially. The ratio of the sound intensity of the sum frequency component is also significantly higher than that of the second harmonic component. The calculation results of the ultrasonic intensity are basically consistent with the simulation results, which proves the correctness of the theoretical model. Finally, through the design experiments, the use of simulated cracks to verify the validity of the model provides an effective means for the detection of micro-crack orientation of metallic materials.
-
Key words:
- non-destructive testing /
- micro-crack /
- nonlinear ultrasound /
- sum frequency /
- micro-crack orientation
-
随着社会的发展进步及信息化时代的到来，人们的工作、生活等社会活动越来越依赖于位置信息，位置服务逐渐成为大家关注的焦点。目前室外定位导航技术大多依赖于卫星导航系统，经过几十年的发展，到现在已经比较成熟，如今在运载体导航、人员跟踪定位及紧急救助服务等方面发挥着巨大的作用[1]，并且实现了广域精准定位，广泛应用于工农业生产和军事等领域。
相比较于室外广域无线定位，室内定位有自己的特点，其范围一般较小，范围通常在几百米以内；另一方面由于室内环境的复杂，干扰因素较多，容易对定位信号产生影响。而卫星导航定位在室内由于受到建筑物、家具等的遮挡，卫星导航信号衰减很快，而且即使有一些信号，由于环境因素复杂，导致多径现象严重，使其无法在室内定位应用。
自20世纪90年代末起，室内无线定位技术开始受到人们的广泛关注。经过近20年的研究，室内定位技术已经取得了一些成果[2]，有一些比较成熟的技术得到了初步应用。室内定位技术分为局部室内定位和大范围室内定位，目前最常见的局域定位技术是WiFi室内定位，其基于信号能量的指纹匹配定位技术，定位精度能够达到3 m，但是信号受环境影响较大，而且需要前期采集大量的数据，定位时需要与数据库的数据进行比对。另一种室内定位技术UWB(Ultra Wideband)能够达到很高的定位精度，典型值为0.3 m，但缺点也很明显，即覆盖范围小。除此之外，还有一些新的局域室内定位技术，比如Zig Bee、蓝牙、射频标签等[3-4]，这些局域室内定位技术几乎都不是专门用作定位应用的，主要作用还是通信应用。
目前能够实现大范围室内定位的技术主要是架设地面伪卫星系统和利用通信基站的室内定位系统。北京邮电大学的时分&码分正交频分复用(TC-OFDM)[5]广域室内定位技术利用现有的通信基站，通过将定位信号承载到手机通信的信号上，能够实现大范围室内定位，系统精度虽然较高，但是大范围室内定位的技术复杂，而且需要较长的布设周期和较大的成本。地面伪卫星系统实现室内定位的代表是澳大利亚的Locata系统[6]，通过增大地面伪卫星发射信号的功率，在室内也可达到较高的定位精度，但其覆盖范围有限或需架设很多伪卫星基站。
目前，针对大型、复杂、多功能建筑，其内部结构复杂、信号环境恶劣、导航信号衰减幅度大，而且多径影响严重，无法直接利用卫星导航信号进行定位；并且在大型火灾、暴力恐怖袭击等灾害现场或事件发生地，电力供应常常被切断或无法使用，停电范围可能波及多个街区，导致目前已有的室内定位手段无法展开应用。针对突发事件现场，对定位系统的基本要求是独立、快速、易用及高精度，目前还缺乏成熟的技术或系统可以应用[7]。
当前室内定位方案难以成熟应用的问题，主要是覆盖范围与定位精度不可兼得[8]。如UWB的精度较高，但穿透能力有限；Locata和TC-OFDM系统为了保证覆盖，需要布设较多基站[9]；惯导模块或设备没有覆盖范围的限制，但存在积累误差[10]。
针对以上问题，本文提出了一种基于地面基站的大区域室内定位方案，不同于发射卫星信号的伪卫星，地面基站发送的是专门设计的导航信号，在频率、信号体制、定位方式等方面进行改进。考虑到频率与信号穿越性能的关系，选择了较低频段作为信号载波，以及采用码伪距和载波伪距联合定位的方式。本文利用所提出的新型定位方式，搭建了一个基本测试系统，通过信号的产生、发射，以及空间无线传播，进行信号的捕获、跟踪和伪距求差解算，初步验证了本文方法的可行性及有效性。
1. 系统模型
系统的设计采用覆盖与精度统筹考虑的策略，选用了76 MHz的载波频率，具有良好的信号穿透能力以及障碍翻越能力。采用伪随机码进行初步定位，获取大致的用户位置。采用载波进行精细测距，最终实现高精度的定位。
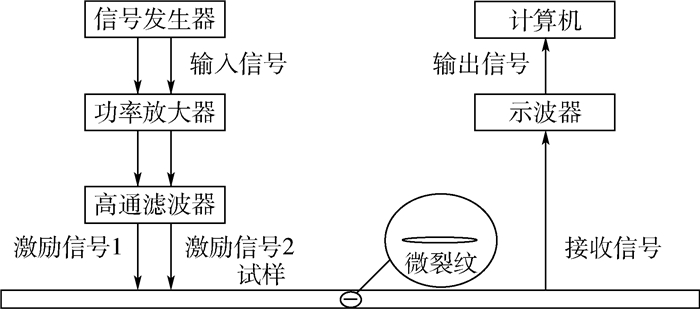
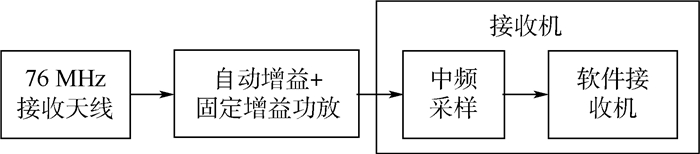
根据以上设计思路，专门研制了实际系统。系统包括多个发射基站及接收终端，下面分别进行介绍。发射端的组成框图如图 1所示。接收端的组成框图如图 2所示。
图中各部分的功能介绍如下：
现场可编程门阵列(FPGA)：完成数字基带信号的生成与调制，生成的数字信号包括导航电文、伪随机码和信号载波，采用低数字中频方式，将导航电文、伪随机码对数字载波进行调制。
AD9361射频：完成数字信号向射频信号的转换，数字基带信号输入给AD9361模块后，将进行滤波、数模转换、上变频等一系列处理，产生所需射频信号。对AD9361射频本振频率进行设置，产生中心频率为76 MHz的射频信号。
47 dB功放：将AD9361输出的模拟信号功率放大到1 W。
自动增益+固定增益功放：将天线接收后的信号进行功率放大，使信号的适应性增加，能够正常进行采样。
中频采样：将天线接收到的模拟信号使用62 MHz进行采样。采样后数据使用软件接收机进行捕获、跟踪，输出码相位和载波相位观测值。
2. 定位方案
系统研制完成后，通过在地面合适位置布设4~6个地面基站，使得在所服务的区域内都可以收到至少4个基站的定位信号，接收机对接收到的信号进行捕获跟踪，得到到达时间(TDOA)即距离的测量值，并进行定位解算，就可以获得用户的实际位置。定位过程如下：
1) 按布局设计搭建多个基站，使得服务区域内的GDOP值尽量小。
2) 通过地理测绘或全球导航卫星系统(GNSS)差分技术等，对基站的真实位置进行标定。
3) 通过光纤技术、时间双向比对技术等，实现对基站之间的高精度时间同步。
4) 各基站同步发射导航信号，利用低频载波增加覆盖范围。
5) 接收机接收4个质量较好、GDOP值较小的基站信号进行距离测量并实时定位，并且通过载波相位测量提高定位精度。
本系统的定位算法原理如下：利用载波波长较小，载波跟踪精度高和受多径影响小的特点，使用载波来进行高精度定位，缺点是载波整周是位置的。本定位方案使用码伪距来确定未知的载波整周，将载波伪距与码伪距确定的载波整周相结合，得到较精确的目标到信号源距离差，使用下面的TDOA方法完成定位结算。
基于TDOA的无线电定位是一种重要的目标三维定位方法，被广泛应用于导航、雷达等定位系统，定位方法主要分为2种：一是迭代算法[11]，二是解析算法[12-13]。迭代算法的优越性体现在能充分利用系统的冗余信息，计算精度高，但计算复杂，实时性差。解析算法精度较低，但实时性好。考虑到室内运动速度不高，本文采用迭代算法。
当已知信号源的位置坐标为Si(xi, yi, zi)T，目标位置坐标为Tr(xr, yr, zr)T，得到目标到信号源的距离差为
(1) 对目标到信号源的距离取平方：
(2) 通过对目标接收机码相位及载波相位观测值分别两两做差，可以得到距离差dij，在实际测试中，距离差含有各种因素引起的误差εij，假设距离差的理论值为dijt，所以距离差测量值可以表示为
(3) 将式(1)移项平方，得到ri2=(dij+rj)2，将式(2)代入，可得
(4) 式中：
由于测试误差的存在，式(4)的等号左边并不等于0，可用微小量δij代替。实际情况下，可以将式(4)表示成矩阵形式，通过最小二乘法就可以求解目标位置。
这种实现方案，优点是可以避免卫星导航中的主要误差因素，如星历、星钟、对流层、电离层等误差，导航信号的完好性也大大增强，并且利用低频载波可以增加覆盖范围和穿透能力，缺点是在地球表面的多径信号较多，需要通过抗多径技术减弱多径的影响。
与其他室内定位技术相比[14]，比如WiFi定位，本文方法利用伪随机码的相关性进行测距和定位，精度明显高于利用WiFi信号的功率测距方法，所以WiFi定位目前大多通过指纹匹配技术来提高精度；而UWB定位，其测距精度最高，但UWB工作频率也很高，受周围环境的影响很大，覆盖范围十分受限；Locata系统是一种地基伪卫星定位系统，目前有一定应用，但其在每个室内都需要布设多个伪卫星基站并实现同步，成本及布局问题限制了其进一步应用[15]。
3. 测试结果处理与分析
3.1 在室外空旷条件下测试
首先测试信号源间码相位和载波相位同步情况，接着测试76 MHz发射频率时中垂线处和偏移处接收码相位和载波相位能否体现距离差的变化。
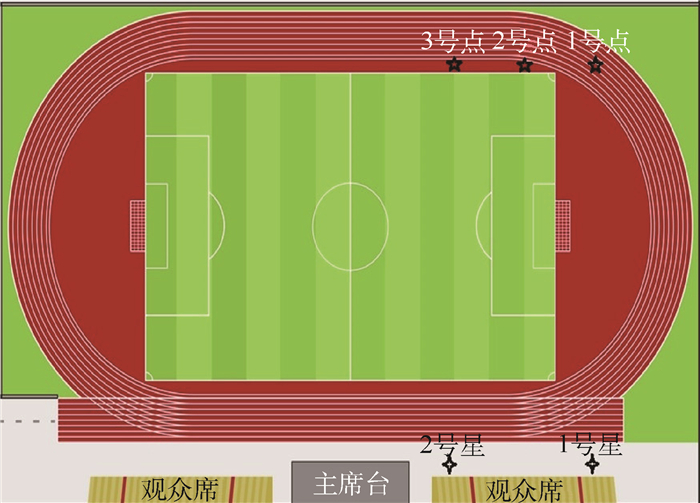
以操场为测试环境(见图 3)，在主席台右侧看台最高处台阶放置两台信号发射源，发射功率为30 dBm，信号源1、2号星间距为45 m。在对侧跑道上取3个等间隔的测试点(接收机位置)，分别为1号点、2号点、3号点。信号源与测试点构成长方形。2号点(取1号星、2号星中垂线与长方形的交点)距两信号源中点87 m。1号点、3号点与两信号源的距离差约为11 m。信号源与接收机的位置如图 3所示。
1) 首先进行2个信号源的同步测试，启动1号星与2号星，经过同步模块进行时钟校准后，使用电脑同时配置信号源，使两台信号源分别产生给定的定位信号。
将两台信号源通过导线同时连接接收机，通过接收机显示界面观察码相位，并采集码相位及载波相位观测量，其码相位差和载波相位差的测试曲线分别如图 4和图 5所示(测试2次)。其对应的测试数据如表 1所示。
表 1 测试数据(同步测试)Table 1. Test data (synchronous test)观测量 测试分组 均值/m 标准差/cm 码相位差 第1次 1.63 57.7 第2次 1.68 53.7 载波相位差 第1次 0.991 5 0.18 第2次 2.943 1 0.11 测试结果表明，系统工作正常，但不同组测试时可能会有半周差，可在后期处理时校正。可以看出，在导线连接条件下测试的精度较高，原因是由于没有引入空间传播方面的误差。
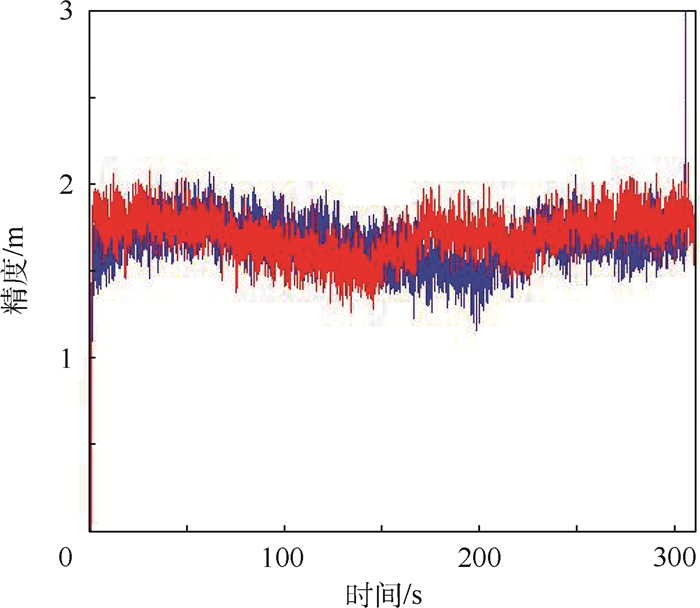
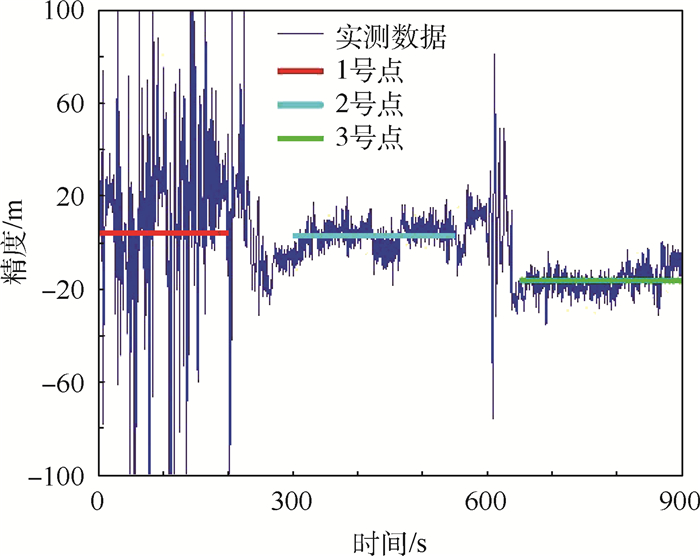
2) 下面进行运动状态下的测试，分别连接好发射、同步、接收设备，并启动其正常工作，在操场携带接收端设备首先从1号点沿直线走到2号点，再走到3号点，在该过程中在3个测试点各停留约200 s左右，实时采集并处理2个信号源发出的定位信号，其码相位差和载波相位差的测试曲线分别如图 6和图 7所示。其对应的测试数据如表 2所示。
图 6 码相位差值(运动状态下测试，1号点~3号点)Figure 6. Code phase difference value (test under motion, point 1 to 3)
图 7 载波相位差值(运动状态下测试，1号点~3号点)Figure 7. Carrier phase difference value (test under motion, point 1 to 3)表 2 测试数据(运动状态下测试，1号点~3号点)Table 2. Test data (test under motion, point 1 to 3)m 测试点 码相位差 载波相位差 均值 标准差 均值 标准差 1 3.66 66.8 13.2 8.397 2 2.83 5.6 31.1 0.19 3 -16.6 5.4 39.5 0.13 测试结果表明：码相位差值在1号点有较大的波动，在2号点、3号点比较平稳，标准差符合预期。同样，载波相位差在1号点时出现了几次跳跃，与静止状态不符，但2号点、3号点基本平稳，标准差也小，符合预期，并且比较理想地反映了从2号点到3号点的运动过程。
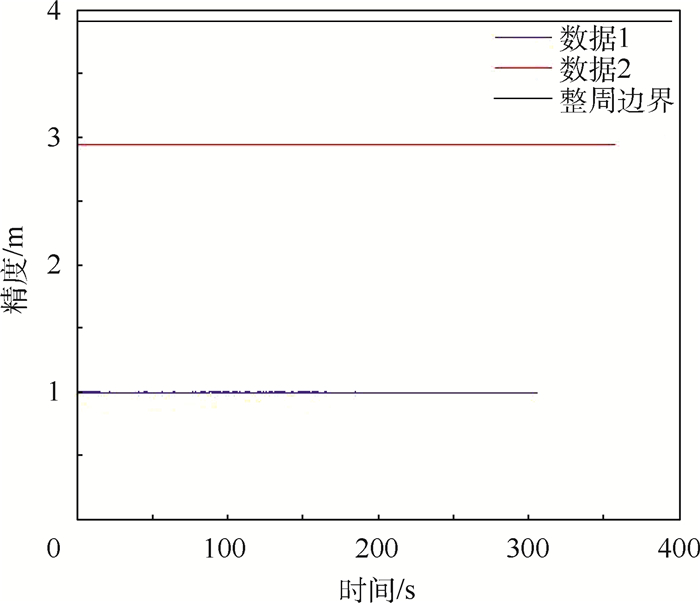
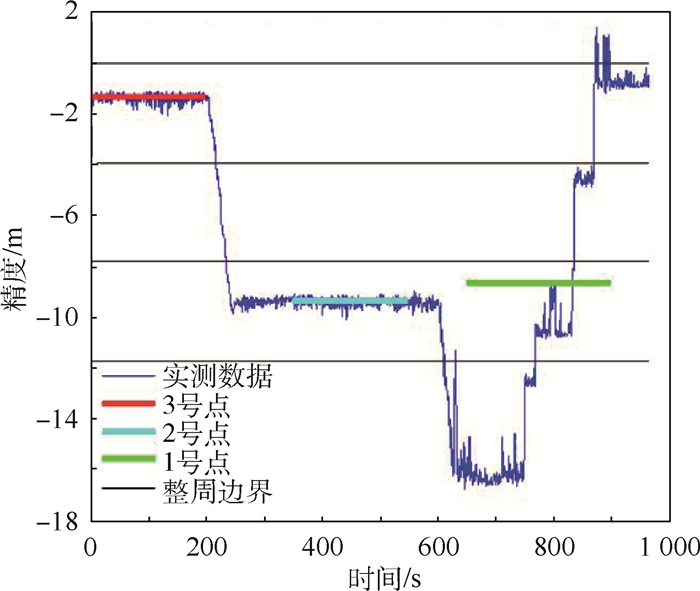
3) 最后再从3号点沿直线返回2号点，再返回1号点，同样在3个测试点各停留约200 s左右。实时采集并处理2个信号源发出的定位信号，其码相位差和载波相位差的测试曲线分别如图 8和图 9所示。其对应的测试数据如表 3所示。
图 8 码相位差值(运动状态下测试，3号点~1号点)Figure 8. Code phase difference value (test under motion, point 3 to 1)
图 9 载波相位差值(运动状态下测试，3号点~1号点)Figure 9. Carrier phase difference value (test under motion, point 3 to 1)表 3 测试数据(运动状态下测试，3号点~1号点)Table 3. Test data (test under motion, point 3 to 1)m 测试点 码相位差 载波相位差 均值 标准差 均值 标准差 3 -26.5 7.1 -1.38 0.138 2 4.4 4 -9.38 0.125 1 24.5 53.8 -8.67 6.4 测试结果表明：在2号点、3号点及其连接路径上的定位精度比较好，同样在1号点出现了大的误差和波动，从3号点走到2号点的路径刻画十分理想，说明了测试数据的可信性。虽然行走的方向与1号点~3号点相反，但2个方向的测试结果是一致的，2号点和3号点载波相位差对应的两次位置差值分别为8.4、8 m，比较接近，说明了测试的可重复性及有效性。
3.2 在复杂室内条件下测试
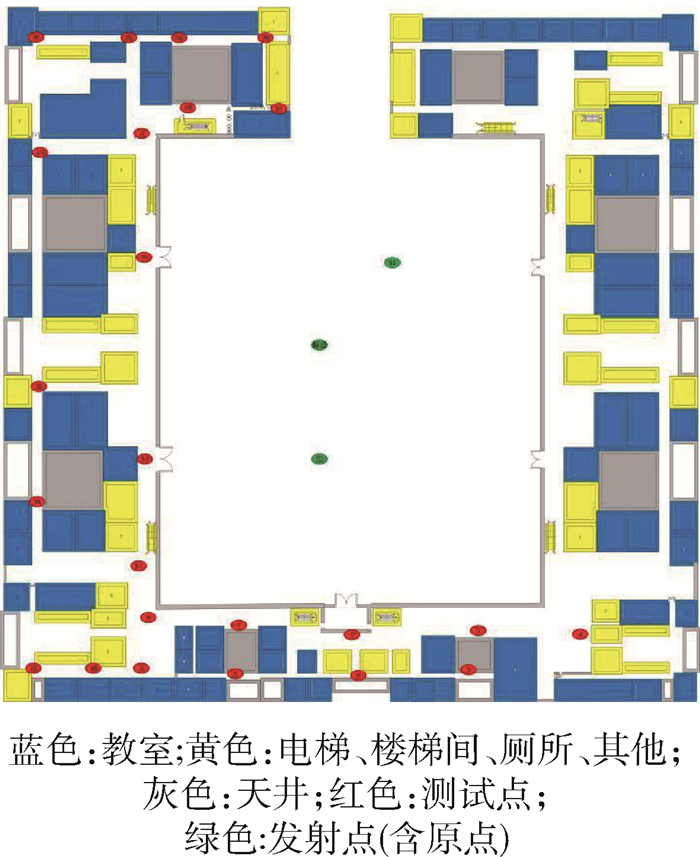
在前期室外测试验证了系统工作原理及测试结果正确性的基础上，开展了实际复杂室内条件下的测试，测试场景为北京航空航天大学新主楼，如图 10所示。
图中发射信号源放在位于大楼中间露天的地面绿点处，发射功率4 W，测试点为2楼室内的红点处，可以看到环境比较复杂，尤其外圈的一些红点，信号要穿过多重建筑实体才能够到达。
通过在红点处逐个测试，采集信号源穿过楼体的到达信号，通过事后的相应处理，得到以下结果：
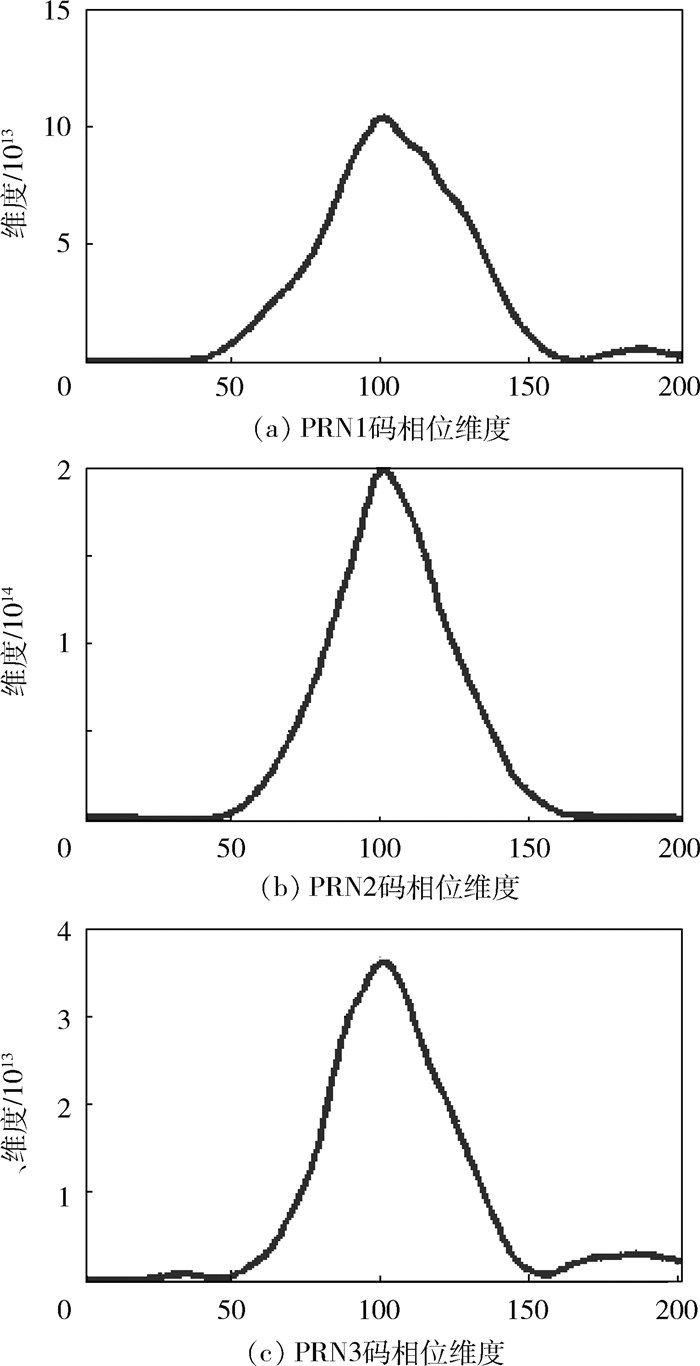
1) 在所有测试点(红点处)都可以接收、捕获到信号源发出的导航信号，说明本文的低频策略是有效的，该方法的覆盖能力较强。如在测试点2对3个基站信号的捕获情况如图 11所示。
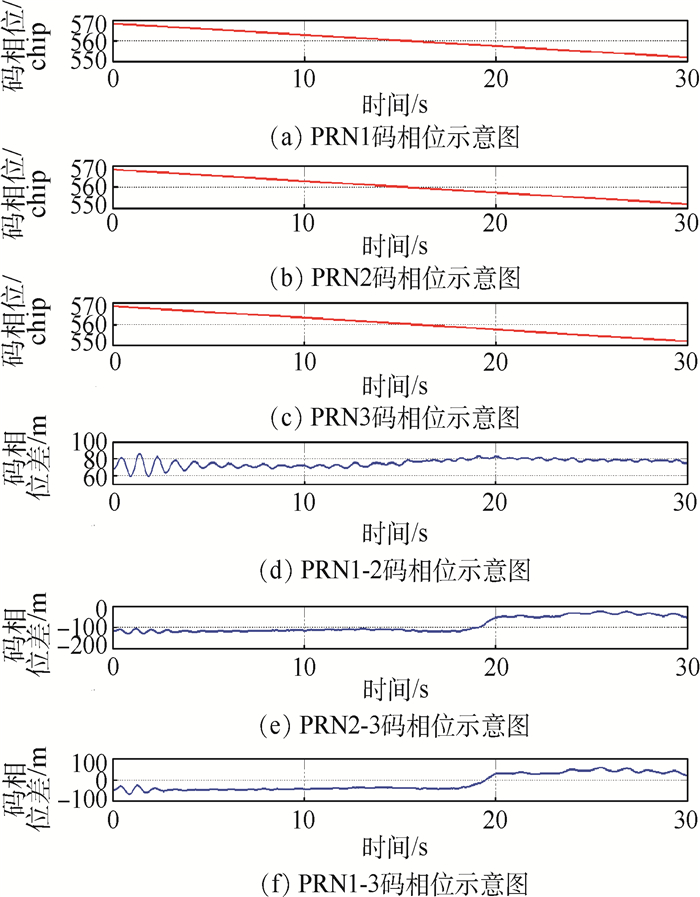
2) 由于环境复杂、多径严重等原因，测试数据的跟踪结果表明码相位和载波相位的误差都比较大，尤其信号路径长的测试点还出现了大的偏差，严重的已无法用于定位解算，需要进一步进行抗多径等处理。同样在测试点2，对3个基站的码跟踪曲线及基站间的码伪距差曲线如图 12所示。图 12(d)为PRN1-2码相位示意图，最大值和最小值分别为83.071 m和72.570 m，标准差为1.847 9 m，均值为78.605 4 m; 图 12(e)为PRN1-3码相位示意图，最大值和最小值分别为-21.904 7 m和-121.623 9 m，标准差为34.529 4 m，均值为-63.764 4 m; 图 12(f)为PRN1-3码相位示意图，最大值和最小值分别为57.223 8 m和-44.515 9 m，标准差为34.770 9 m，均值为14.840 9 m。从图中可以看出，各基站间的码伪距差波动较小，但其均值与事先标定的真值偏差较大，分析是由多径因素造成的固定偏差。
图 12 测试点2跟踪曲线及基站间距离差曲线Figure 12. Tracking curve and distance difference curves between base stations at test point 24. 结论
本文经过不同环境的测试并对结果进行分析，初步验证了所提方法的可行性，证明了系统定位的可行性和良好的覆盖能力，但也发现了系统进一步应用需解决的问题。综合分析本文测试结果，可以得出如下结论：
1) 在室外开阔环境(操场2号点、3号点)的测试，位置差的精度较高，动态测试符合预期，说明了方法的可行性和有效性。
2) 如果接收天线被行人遮挡(操场1号点，有跑步人员)，会出现码相位误差变大和载波相位跳变的情况，在使用中应该尽量避免。
3) 本文信号的穿透能力较强，对复杂室内的覆盖范围较大，但需要进一步解决多径严重，以及信号衰减大、信号质量下降等问题，才可以实现大区域的室内定位，这也是下一步的工作重点。
-
图 5 不同微裂纹取向角度仿真模型图
Figure 5. Simulation model illustration for different micro-crack orientation angles
图 7 微裂纹取向角度为90°时输出信号的波形与频谱
Figure 7. Waveform and spectrogram of output signal when micro-crack orientation angle is 90°
图 9 不同取向角度微裂纹的和频非线性效应
Figure 9. Sum frequency nonlinear effect of microcracks at different orientation angles
图 11 和频分量与二次谐波声强对比
Figure 11. Comparison of sound intensity of sum frequency component and second harmonic component
图 15 不同微裂纹取向角度输出信号频域波形
Figure 15. Frequency domain waveform of different orientation angle micro-crack output signal
图 16 超声和频非线性特征系数变化趋势
Figure 16. Tendency of ultrasound nonlinear characteristic coefficient of sum frequency
表 1 各分量声强计算结果及占比
Table 1. Computation results and proportions of sound intensity of each component
微裂纹取向角度/(°) I′1/(1013W·m－2) I′2/(1013W·m-2) η1/% η2/% 0 1.247 1.797 0.289 0.681 15 2.163 1.951 1.290 0.498 30 1.608 3.541 1.455 0.958 45 3.074 1.762 1.428 1.321 60 3.262 0.3572 2.157 0.134 75 4.264 1.166 4.518 0.273 90 18.41 2.166 4.566 0.518 
下载: 导出CSV
-
[1] MEYENDORF N G, RÖSNER H, KRAMB V, et al.Thermo-acoustic fatigue characterization[J].Ultrasonics, 2002, 40(1-8):427-434. doi: 10.1016/S0041-624X(02)00155-5 [2] 税国双, 汪越胜, 曲建民.材料力学性能退化的超声无损检测与评价[J].力学进展, 2005, 35(1):52-68. doi: 10.3321/j.issn:1000-0992.2005.01.006SHUI G S, WANG Y S, QU J M.Advances in nondestructive test and evaluation of material degradation using nonlinear ultrasound[J].Advances in Mechanics, 2005, 35(1):52-68(in Chinese). doi: 10.3321/j.issn:1000-0992.2005.01.006 [3] 高桂丽, 李大勇, 董静薇, 等.铝合金薄板疲劳裂纹的非线性声学特性[J].机械工程学报, 2010, 46(18):71-76.GAO G L, LI D Y, DONG J W, et al.Nonlinear acoustic characteristics of fatigue cracks in aluminum alloy sheet[J].Journal of Mechanical Engineering, 2010, 46(18):71-76(in Chinese). [4] JHANG K Y.Nonlinear ultrasonic techniques for non-destructive assessment of micro damage in material:A review[J].International Journal of Precision Engineering and Manufacturing, 2009, 10(1):123-135. doi: 10.1007/s12541-009-0019-y [5] 周正干, 刘斯明.非线性无损检测技术的研究、应用和发展[J].机械工程学报, 2011, 47(8):2-11.ZHOU Z G, LIU S M.Nonlinear ultrasonic techniques used in nondestructive testing:A review[J].Journal of Mechanical Engineering, 2011, 47(8):2-11(in Chinese). [6] 焦敬品, 樊仲祥, 吴斌, 等.闭合裂纹非共线混频超声检测试验研究[J].声学学报, 2017, 42(2):205-213.JIAO J P, FAN Z X, WU B, et al.Experiments of non-collinear mixed frequency ultrasonic for closed crack detection[J].Acta Acustica, 2017, 42(2):205-213(in Chinese). [7] 焦敬品, 孙俊俊, 吴斌, 等.结构微裂纹混频非线性超声检测方法研究[J].声学学报, 2013, 38(6):648-656.JIAO J P, SUN J J, WU B, et al.A frequency-mixing nonlinear ultrasonic technique for micro-crack detection[J].Acta Acustica, 2013, 38(6):648-656(in Chinese). [8] JIAO J, MENG X, HE C, et al.Nonlinear Lamb wave-mixing technique for micro-crack detection in plates[J].NDT & E International, 2016, 85:63-71. [9] CROXFORD A J, WILCOX P D, DRINKWATER B W, et al.The use of non-collinear mixing for nonlinear ultrasonic detection of plasticity and fatigue[J].Journal of the Acoustical Society of America, 2009, 126(5):117-122. doi: 10.1121/1.3231451 [10] SUN M, XIANG Y, DENG M, et al.Scanning non-collinear wave mixing for nonlinear ultrasonic detection and localization of plasticity[J].NDT & E International, 2018, 93:1-6. [11] MCGOGERN M, REIS H.Damage characterization in dimension limestone cladding using noncollinear ultrasonic wave mixing[J].Optical Engineering, 2015, 55(1):011012. [12] 冯侠, 邢修三.微裂纹取向对金属断裂过程的影响[J].北京理工大学学报, 1994, 14(3):234-239.FENG X, XING X S.On the effects of random orientation of microcracks upon the fracture process of metals[J].Journal of Beijing Institute of Technology, 1994, 14(3):234-239(in Chinese). [13] COURTNEY C R P, NEILD S A, WILCOX P D, et al.Application of the bispectrum for detection of small nonlinearities excited sinusoidally[J].Journal of Sound & Vibration, 2010, 329(20):4279-4293. [14] DONSKOY D, SUTIN A, EKIMOV A.Nonlinear acoustic interaction on contact interfaces and its use for nondestructive testing[J].NDT & E International, 2001, 34(4):231-238. [15] SOLODOV I Y, KROHN N, BUSSE G.An example of nonclassical acoustic nonlinearity in solids[J].Ultrasonics, 2002, 40(1-8):621-625. doi: 10.1016/S0041-624X(02)00186-5 [16] BREAZEALE M A, PHILIP J.Determination of third-order elastic constants from ultrasonic harmonic generation measurements[M].Pittsburg:Academic Press, 1987. [17] CROXFORD A J, DRINKWATER B W, WILCOX P D.Nonlinear ultrasonic characterization using the noncollinear method[C]//37th Annual Review of Progress in Quantitative Nondestructive Evaluation.New York: AIP, 2011: 330-337. doi: 10.1063/1.3591872 [18] 李海洋, 安志武, 廉国选, 等.粗糙接触界面超声非线性效应的概率模型[J].声学学报, 2015, 40(2):247-253.LI H Y, AN Z W, LIAN G X, et al.A probability model for ultrasonic nonlinear effects of rough contact interface[J].Acta Acustica, 2015, 40(2):247-253(in Chinese). 期刊类型引用(4)
1. 李昶，张佳佳，曹英卓. 基于跨域数据的位置能力研究与实践. 电信工程技术与标准化. 2022(05): 27-32 . 
百度学术2. 侯忠辉，陈国清，张琛. 基于大数据的手机报警精准定位技术研究. 电信快报. 2021(03): 16-19 . 百度学术3. 廖泽淇，王伟光，唐上钦，吴旭东. 基于MEMS传感器的手持电动工具运动状态三维定位监测系统. 工业仪表与自动化装置. 2020(05): 3-7 . 百度学术4. 罗伟华，许盛宏. 基于MR大数据的基站小区定位方案. 移动通信. 2020(10): 73-76 . 百度学术其他类型引用(4)
-
下载:
下载:
百度学术
点击查看大图
计量
- 文章访问数: 859
- HTML全文浏览量: 177
- PDF下载量: 752
- 被引次数: 8
