-
摘要:
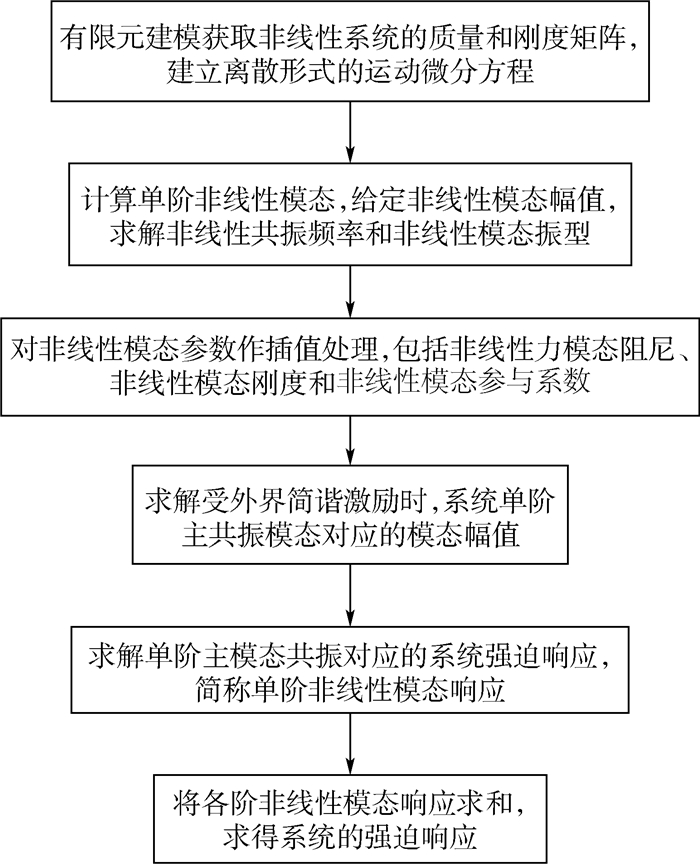
针对非线性问题计算方法复杂和计算时间冗长一直是动力学领域的难点问题，给出了一套简单、准确、高效的非线性模态分析方法，对于常见的非线性系统（如杜芬系统、干摩擦系统和非线性材料等）均适用，具有一般性。首先，给出所提方法的基本理论与分析流程；然后，以杜芬系统为例阐述了其在非线性实模态域的应用，以干摩擦系统为例描述了其在非线性复模态域的应用，以压电系统为例展示了其在多场耦合域的应用；最后，给出了基于该理论对大型复杂非线性系统求解时的减缩方法。所提方法的核心在于建立非线性模态参数关于模态幅值的变化规律，不仅将系统的稳态响应求解问题简化为一维代数问题，极大地简化了数值计算过程，而且有助于分析、理解系统的非线性动力学行为。将所提方法与模态综合法结合，可用于高效求解大型复杂非线性系统动力学特性。
Abstract:Nonlinear problem has always been an obstacle in dynamic analysis domain due to its complexity and high computational cost. This paper aims to present a simple, accurate and efficient nonlinear modal analysis method which can be applied to some common nonlinear systems, including Duffing system, dry friction, nonlinear material and so on. The kernel technique of this numerical method lies in establishing the variation law of the nonlinear modal parameters in function of modal amplitude:on the one hand, the steady-state problem is simplified into one-dimensional algebraic nonlinear problem, resulting in a significant simplification in numerical computation; on the other hand, the analysis of nonlinear modal parameters in function of modal amplitude provides a modal overview for the comprehension of system's nonlinear dynamic behavior. Following a description of the theoretical aspects and numerical simulation process of this method, it has been proven to be efficient in analyzing a Duffing system with real nonlinear mode, a dry friction system with complex nonlinear mode and a multi-physics system integrating piezoelectric material. A reduction method based on the proposed strategy is then presented, which is simple in mathematical form and efficient in numerical computations for analyzing large complex nonlinear systems. It has significant advantages in computational efficiency when combined with the mode synthesis method to solve the dynamic behavior of large complex nonlinear systems.
-
Key words:
- nonlinear modal analysis /
- mode synthesis /
- Duffing /
- dry friction /
- nonlinear material /
- reduced model
-
图 1 非线性模态分析求解稳态响应流程
Figure 1. Flowchart of steady-state response solved by nonlinear modal analysis
图 3 针对杜芬系统采用时域积分法和非线性模态分析方法计算m1的频响曲线
Figure 3. Frequency response curves of m1computed by time-domain integration method and nonlinear modal analysis method for Duffing system
图 4 针对杜芬系统采用时域积分法和非线性模态分析方法计算m2的频响曲线
Figure 4. Frequency response curves of m2 computed by time-domain integration method and nonlinear modal analysis method for Duffing system
图 5 杜芬系统的两阶非线性模态频率随模态幅值的变化
Figure 5. Variation of nonlinear modal frequency with modal amplitude for the two modes of Duffing system
图 6 杜芬系统的两阶模态参与系数随模态幅值的变化
Figure 6. Variation of modal participation factor with modal amplitude for the two modes of Duffing system
图 8 基于Masing法则建立的干摩擦迟滞环[17]
Figure 8. Hysteresis loop of dry friction based on Masing's rule[17]
图 9 针对干摩擦系统采用时域积分法和非线性模态分析方法计算m1的频响曲线
Figure 9. Frequency response curves of m1computed by time-domain integration method and nonlinear modal analysis method for dry friction system
图 10 针对干摩擦系统采用时域积分法和非线性模态分析方法计算m2的频响曲线
Figure 10. Frequency response curves of m2computed by time-domain integration method and nonlinear modal analysis method for dry friction system
图 11 不同正压力下m1的频响曲线
Figure 11. Frequency response curves of m1 under different normal pressure
图 12 不同正压力下m2的频响曲线
Figure 12. Frequency response curves of m2 under different normal pressure
图 13 第一阶共振频率附近，不同正压力下的非线性共振频率和干摩擦模态阻尼比随模态幅值的变化
Figure 13. Variation of nonlinear resonance frequency and dry friction modal damping with modal amplitude under different normal pressure for the first order mode
图 14 第二阶共振频率附近，不同正压力下的非线性共振频率和干摩擦模态阻尼比随模态幅值的变化
Figure 14. Variation of nonlinear resonance frequency and dry friction modal damping with modal amplitude under different normal pressure for the second order mode
图 16 谐波平衡法和非线性模态分析方法计算m1的频响曲线
Figure 16. Frequency response curves of m1 computed by harmonic balance method and nonlinear modal analysis method
图 17 谐波平衡法和非线性模态分析方法计算m2的频响曲线
Figure 17. Frequency response curves of m2 computed by harmonic balance method and nonlinear modal analysis method
图 18 第一阶共振频率附近，不同电容比下的非线性共振频率和模态阻尼比随模态幅值的变化
Figure 18. Variation of nonlinear resonance frequency and modal damping with modal amplitude under different capacitance ratios for the first order mode
图 19 第二阶共振频率附近，不同电容比下的非线性共振频率和模态阻尼随模态幅值的变化
Figure 19. Variation of nonlinear resonance frequency and modal damping with modal amplitude under different capacitance ratios for the second order mode
-
[1] JIANG D, PIERRE C, SHAW S W.Nonlinear normal modes for vibratory systems under harmonic excitation[J].Journal of Sound and Vibration, 2005, 288(4-5):791-812. doi: 10.1016/j.jsv.2005.01.009 [2] TOUZÉ C, AMABILI M.Nonlinear normal modes for damped geometrically nonlinear systems:Application to reduced-order modelling of harmonically forced structures[J].Journal of Sound and Vibration, 2006, 298(4-5):958-981. doi: 10.1016/j.jsv.2006.06.032 [3] RENSON L, KERSCHEN G, COCHELIN B.Numerical computation of nonlinear normal modes in mechanical engineering[J].Journal of Sound and Vibration, 2016, 364:177-206. doi: 10.1016/j.jsv.2015.09.033 [4] HUANG X R, JÉZÉQUEL L, BESSET S, et al.Nonlinear hybrid modal synthesis based on branch modes for dynamic analysis of assembled structure[J].Mechanical Systems and Signal Processing, 2018, 99:624-646. doi: 10.1016/j.ymssp.2017.07.002 [5] LIU J Z, LI L, HUANG X R, et al.Dynamic characteristics of the blisk with synchronized switch damping based on negative capacitor[J].Mechanical Systems and Signal Processing, 2017, 95:425-445. doi: 10.1016/j.ymssp.2017.03.049 [6] ROSENBERG R M.The normal modes of nonlinear n-degrees-of-freedom systems[J].Journal of Applied Mechanics, 1962, 29(1):595-611. [7] SZEMPLINSKA-STUPNICKA W. The resonant vibration of homogeneous non-linear systems[J].International Journal of Non-Linear Mechanics, 1980, 15(4-5):407-415. doi: 10.1016/0020-7462(80)90026-8 [8] JÉZÉQUEL L, LAMARQUE C.Analysis of non-linear dynamical systems by the normal form theory[J].Journal of Sound and Vibration, 1991, 149(3):429-459. doi: 10.1016/0022-460X(91)90446-Q [9] SETIO H D, SETIO S, JÉZÉQUEL L.A method of non-linear modal identification from frequency response tests[J].Journal of Sound and Vibration, 1992, 158(3):497-515. doi: 10.1016/0022-460X(92)90421-S [10] CHONG Y H, IMREGUN M.Development and application of a nonlinear modal analysis technique for mdof systems[J].Journal of Vibration and Control, 2001, 7(2):167-179. doi: 10.1177/107754630100700202 [11] GIBERT C.Fitting measured frequency response using non-linear modes[J].Mechanical Systems and Signal Processing, 2003, 17(1):211-218. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0210061484/ [12] 郑兆昌.关于线性和非线性系统内在的本质联系——多自由度非线性系统的定量和定性分析[J].振动与冲击, 2008, 27(1):4-8. doi: 10.3969/j.issn.1000-3835.2008.01.001ZHENG Z C.Intrinsic and simple connection of linear systems with non-linear ones:Quantitative and qualitative analysis of large scale multiple DOF nonlinear systems[J].Journal of Vibration and Shock, 2008, 27(1):4-8(in Chinese). doi: 10.3969/j.issn.1000-3835.2008.01.001 [13] HUANG X R, JÉZÉQUEL L, BESSET S, et al.Nonlinear modal synthesis for analyzing structures with a frictional interface using a generalized Masing model[J].Journal of Sound and Vibration, 2018, 434:166-191. doi: 10.1016/j.jsv.2018.07.027 [14] LIU J Z, LI L, FAN Y, et al.A modified nonlinear modal synthesis scheme for mistuned blisks with synchronized switch damping[J].International Journal of Aerospace Engineering, 2018, 2018:8517890. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=e32cdfd1859a4cb743ca24b85196e906 [15] DUFFING G.Elastizität und Reibung beim Riementrieb[J].Forschung Auf Dem Gebiet Des Ingenieurwesens A, 1931, 2(3):99-104. [16] 李琳, 刘久周, 李超.航空发动机中的干摩擦阻尼器及其设计技术研究进展[J].航空动力学报, 2016, 31(10):2305-2317. http://d.old.wanfangdata.com.cn/Periodical/hkdlxb201610001LI L, LIU J Z, LI C.Review of the dry friction dampers in aero-engine and their design technologies[J].Journal of Aerospace Power, 2016, 31(10):2305-2317(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/hkdlxb201610001 [17] CHIANG D Y.The generalized Masing models for deteriorating hysteresis and cyclic plasticity[J].Applied Mathematical Modelling, 1999, 23(11):847-863. doi: 10.1016/S0307-904X(99)00015-3 [18] BAMPTON M C C, CRAIG R R.Coupling of substructures for dynamic analyses[J].AIAA Journal, 1968, 6(7):1313-1319. doi: 10.2514/3.4741 [19] KRYLOV N M, BOGOLYUBOV N N.Introduction to non-linear mechanics[M].Princeton:Princeton University Press, 1947. -
下载:
点击查看大图
计量
- 文章访问数: 648
- HTML全文浏览量: 8
- PDF下载量: 658
- 被引次数: 0
