An improved car-following model considering effect of pedestrians of adjacent lane on traffic flow
-
摘要:
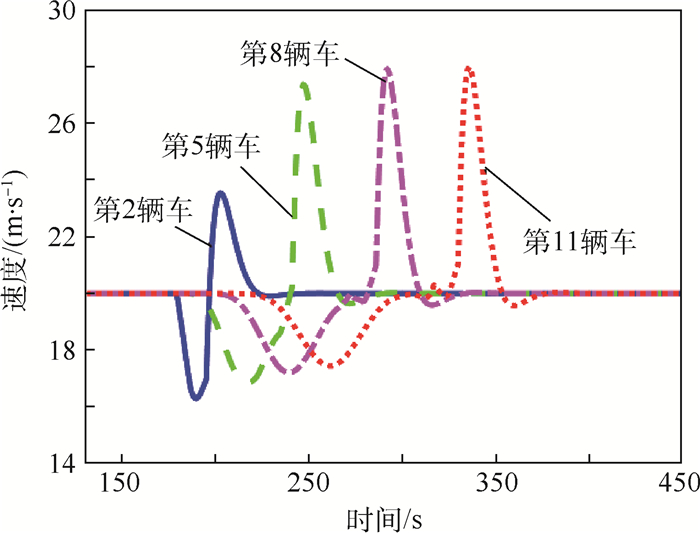
基于实际人车混合行驶的情形，提出了一个改进的车辆动力学模型。该模型考虑了邻车道或横向的行人、自行车等对主干道车辆的驾驶行为影响。基于经典的最优速度模型，将主干道车辆与行人之间的横向距离和纵向距离作为参数引入最优速度模型中。为了验证所提模型的稳定性和有效性，利用线性稳定性理论，推导出模型的稳定性条件和非稳定性条件，绘制中性稳定性曲线，直观描述了交通流稳定区域大小。理论结果表明：考虑横向行人干扰因素的改进跟驰模型比传统的只考虑单一车道车辆因素的跟驰模型更加的稳定，并且不同参数的变化，所引起的稳态区域也会发生变化。采用了更加真实的优化速度方程，通过仿真实验来描述车辆的驾驶行为；仿真实验列举了2种实际场景：行人稀少和行人较多时。分别绘制了车辆的速度-时间变化曲线以及车辆的时空图，实验结果表明：横向行人的确会干扰车辆的正常驾驶；不同场景下，行人数量的多寡也会对车辆的驾驶行为造成不同程度的影响。
Abstract:This paper proposes an improved vehicle dynamics model based on the current situation of mixed-pedestrian-vehicle driving in real life. The model considered the influence of pedestrians, bicycles, etc. on the adjacent lanes or in lateral directions on the driving behavior of the main road vehicles. Based on the classical optimal velocity model, this paper modelled the actual problem and introduced the lateral distance and longitudinal distance between the main road vehicle and the pedestrian as parameters into the optimal velocity model, and expanded to obtain the improved model. In order to verify the stability and effectiveness of the model, this paper used the linear stability theory to derive the stability and non-stable conditions of the model, draw a neutral stability curve, and visually describe the size of the traffic flow stability area. It is shown that the improved model considering the lateral pedestrian interference factor is more stable than the traditional car-following model considering only the single-lane vehicle factor, and the steady-state region caused by the change of different parameters also changes. This paper adopted a more realistic optimization of the velocity equation and described the driving behavior of the vehicle through simulation experiments. The simulation experiment lists two kinds of practical scenarios:when pedestrians are scarce and when pedestrians are more. The velocity-time curve of the vehicle and the space(distance)-time curve of the vehicle were plotted separately. The experimental results show that the lateral pedestrians do interfere with the normal driving of the vehicle; in different scenarios, the number of pedestrians will also affect the driving behavior of the vehicle to varying degrees.
-
图 2 不同参数组合(λ, p, q, γ)下的中性稳定性曲线
Figure 2. Neutral stability curves with different parameter sets of (λ, p, q, γ)
图 3 不同q和γ值的中性稳定性曲线(vmax=3 m/s, hc=4 m)
Figure 3. Neutral stability curves with different values of q and γ (vmax=3 m/s, hc=4 m)
图 4 当p=0.6, q=0.3, γ=0.1时，车辆速度随时间变化曲线
Figure 4. Variation curves of vehicle velocity with time for p=0.6, q=0.3, γ=0.1
图 5 当p=0.6, q=0.2, γ=0.2时，车辆速度随时间变化曲线
Figure 5. Variation curves of vehicle velocity with time for p=0.6, q=0.2, γ=0.2
图 6 场景2下的车辆速度随时间变化曲线
Figure 6. Variation curves of vehicle velocity with time in scene 2
图 7 通过行人人群时的车队时空图
Figure 7. Space-time plots for a motorcade passing by pedestrian crowd
表 1 车辆仿真参数
Table 1. Simulation parameters of vehicles
参数 数值 物理意义 ψ/m 25.0 车辆安全距离 ξ/m 23.3 距离参数 vmax/(m·s-1) 33.6 车辆最大速度 v/(m·s-1) 20 车辆初始化速度 αc/s-1 2.0 敏感度 ymin/m 7.02 最小车头时距 T/s 0.1 抽样时间 
下载: 导出CSV
表 2 行人仿真参数
Table 2. Simulation parameters of pedestrians
参数 数值 物理意义 vp/(m·s-1) 1.5 行人初始速度 vmaxp/(m·s-1) 3 行人最大速度 φ/m 7.6 行人安全距离
下载: 导出CSV
-
[1] NAGATANI T.The physics of traffic jams[J].Reports on Progress in Physics, 2002, 65(9):1331. doi: 10.1088/0034-4885/65/9/203 [2] TREIBER M, KESTING A, HELBING D.Delays, inaccuracies and anticipation in microscopic traffic models[J].Physica A:Statistical Mechanics and Its Applications, 2006, 360(1):71-88. doi: 10.1016/j.physa.2005.05.001 [3] NGODUY D.Analytical studies on the instabilities of heterogeneous intelligent traffic flow[J].Communications in Nonlinear Science and Numerical Simulation, 2013, 18(10):2699-2706. doi: 10.1016/j.cnsns.2013.02.018 [4] REUSCHEL A.Vehicle movements in a platoon with uniform acceleration or deceleration of the lead vehicle[J].Zeitschrift des Oesterreichischen Ingenieur-und Architekten-Vereines, 1950, 95:50-62. http://d.old.wanfangdata.com.cn/Conference/9100805 [5] PIPES L A.An operational analysis of traffic dynamics[J].Journal of Applied Physics, 1953, 24(3):274-281. doi: 10.1063/1.1721265 [6] CHANDLER R E, HERMAN R, MONTROLL E W.Traffic dynamics:Studies in car following[J].Operations Research, 1958, 6(2):165-184. http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_0805.3400 [7] NEWELL G F.Nonlinear effects in the dynamics of car following[J].Operations Research, 1961, 9(2):209-229. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=525133ec45fc2ee28473ad73850d115b [8] BANDO M, HASEBE K, NAKAYAMA A, et al.Dynamical model of traffic congestion and numerical simulation[J].Physical Review E, 1995, 51(2):1035-1045. doi: 10.1103/PhysRevE.51.1035 [9] JIANG R, WU Q, ZHU Z.Full velocity difference model for a car-following theory[J].Physical Review E, 2001, 64(1):017101. doi: 10.1103/PhysRevE.64.017101 [10] HELBING D, TILCH B.Generalized force model of traffic dynamics[J].Physical Review E, 1998, 58(1):133-138. doi: 10.1103/PhysRevE.58.133 [11] ZHOU J.An extended visual angle model for car-following theory[J].Nonlinear Dynamics, 2015, 81(1):549-560. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=cc496de659fcad2e8e9d9f5c27b67cce [12] YU S, SHI Z.An improved car-following model considering relative velocity fluctuation[J].Communications in Nonlinear Science and Numerical Simulation, 2016, 36:319-326. doi: 10.1016/j.cnsns.2015.11.011 [13] HELBING D, JIANG R, TREIBER M.Analytical investigation of oscillations in intersecting flows of pedestrian and vehicle traffic[J].Physical Review E, 2005, 72(4):046130. doi: 10.1103/PhysRevE.72.046130 [14] CHEN P, WU C, ZHU S.Interaction between vehicles and pedestrians at uncontrolled mid-block crosswalks[J].Safety Science, 2016, 82:68-76. doi: 10.1016/j.ssci.2015.09.016 [15] ZHANG X, CHANG G.A dynamic evacuation model for pedestrian-vehicle mixed-flow networks[J].Transportation Research Part C:Emerging Technologies, 2014, 40:75-92. doi: 10.1016/j.trc.2014.01.003 [16] ITO H, NISHINARI K.Totally asymmetric simple exclusion process with a time-dependent boundary:Interaction between vehicles and pedestrians at intersections[J].Physical Review E, 2014, 89(4):042813. doi: 10.1103/PhysRevE.89.042813 [17] JIANG R, WU Q.The moving behavior of a large object in the crowds in a narrow channel[J].Physica A:Statistical Mechanics and Its Applications, 2006, 364:457-463. doi: 10.1016/j.physa.2005.08.060 [18] JIANG R, WU Q.Interaction between vehicle and pedestrians in a narrow channel[J].Physica A:Statistical Mechanics and Its Applications, 2006, 368(1):239-246. doi: 10.1016/j.physa.2005.12.071 [19] WHITHAM G B.Exact solutions for a discrete system arising in traffic flow[C]//Proceedings of the Royal Society of London.Series A, Mathematical and Physical Sciences.London: The Royal Society of London, Mathematical, Physical and Engineering Sciences, 1990: 49-69. [20] GE H X, DAI S Q, DONG L Y, et al.Stabilization effect of traffic flow in an extended car-following model based on an intelligent transportation system application[J].Physical Review E, 2004, 70(6):066134. doi: 10.1103/PhysRevE.70.066134 [21] SAWADA S.Generalized optimal velocity model for traffic flow[J].International Journal of Modern Physics C, 2002, 13(1):1-13. doi: 10.1142/S0129183102002894 [22] LI Y, ZHANG L, ZHENG H, et al.Evaluating the energy consumption of electric vehicles based on car-following model under non-lane discipline[J].Nonlinear Dynamics, 2015, 82(1):629-641. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=7e0e18c866cda534db079396ed2e94f3 [23] PENG G H, SUN D H.A dynamical model of car-following with the consideration of the multiple information of preceding cars[J].Physics Letters A, 2010, 374:1694-1698. doi: 10.1016/j.physleta.2010.02.020 [24] TANG T, HUANG H, GAO Z.Stability of the car-following model on two lanes[J].Physical Review E, 2005, 72(6):066124. doi: 10.1103/PhysRevE.72.066124 [25] LI Z P, LIU Y C.Analysis of stability and density waves of traffic flow model in an its environment[J].The European Physical Journal B:Condensed Matter and Complex Systems, 2006, 53(3):367-374. doi: 10.1140/epjb/e2006-00382-7 [26] YU X.Analysis of the stability and density waves for traffic flow[J].Chinese Physics, 2002, 11(11):1128-1134. doi: 10.1088/1009-1963/11/11/307 [27] KONISHI K, KOKAME H, HIRATA K.Coupled map car-following model and its delayed-feedback control[J].Physical Review E, 1999, 60(4):4000-4007. doi: 10.1103/PhysRevE.60.4000 [28] BANDO M, HASEBE K, NAKANISHI K, et al.Analysis of optimal velocity model with explicit delay[J].Physical Review E, 1998, 58(5):5429-5450. doi: 10.1103/PhysRevE.58.5429 [29] BANDO M, HASEBE K, NAKANISHI K, et al.Phenomenological study of dynamical model of traffic flow[J].Journal de Physique I, 1995, 5(11):1389-1399. doi: 10.1051/jp1:1995206 [30] TADAKI S, KIKUCHI M, SUGIYAMA Y, et al.Coupled map traffic flow simulator based on optimal velocity functions[J].Journal of the Physical Society of Japan, 1998, 67(7):2270-2276. doi: 10.1143/JPSJ.67.2270 -
下载:
点击查看大图
计量
- 文章访问数: 614
- HTML全文浏览量: 58
- PDF下载量: 418
- 被引次数: 0
