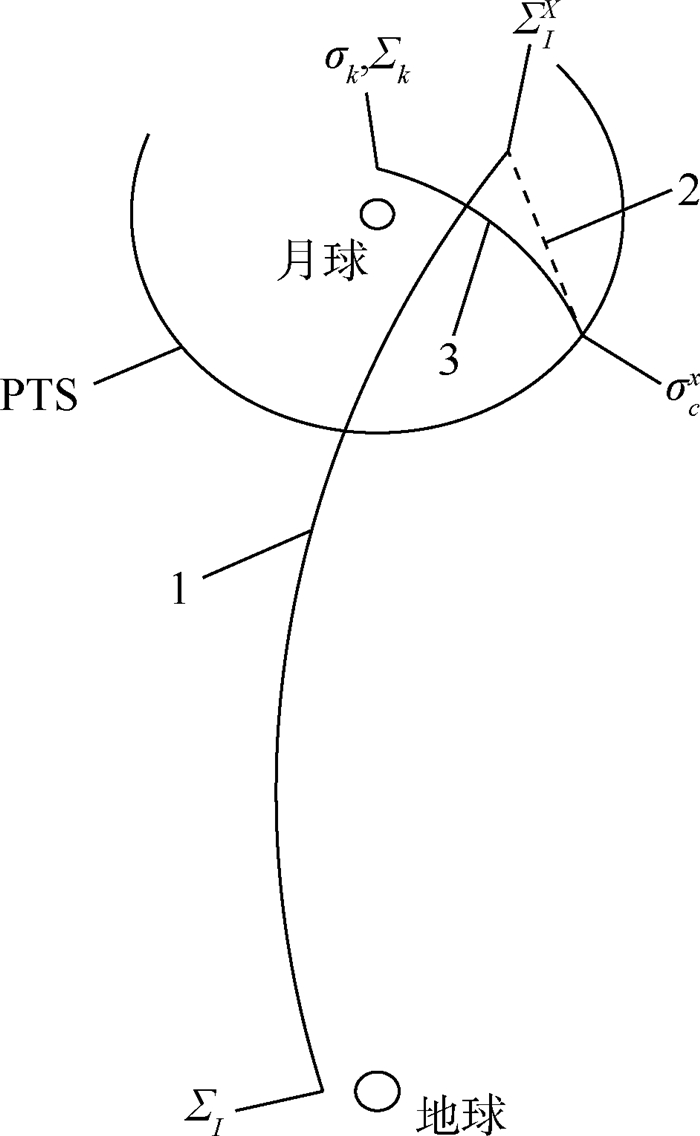
-
摘要:
针对从月球停泊轨道出发直接再入大气的月地转移轨道设计问题，提出了一种数值求解算法。该算法由初值设计和精确解求解两部分组成。首先，根据轨道设计的相应约束，采用伪状态理论，通过简单迭代求解高精度的初值。然后，考虑精确的动力学模型，通过数值积分计算真实轨道和状态转移矩阵，并利用微分修正方法搜索精确解。该算法通过设计高精度的初值，降低了月地转移轨道的设计难度。数值仿真表明：该算法求解效率高，具有良好的鲁棒性。
Abstract:A new solution algorithm for the design of Moon-to-Earth transfer orbit which leaves from the Moon parking orbit with direct atmospheric reentry and single impulse is presented in this paper. The algorithm is divided into two steps, designing the initial solution and searching the exact solution. First, according to the constraint conditions, the high-precision initial solution is generated by simple iteration using pseudostate theory. Then, the real orbit and state transition matrix are calculated by numerical integration in the real dynamic model, and the exact solution is found by the differential correction method. Because the initial solution with high accuracy is used, the difficulty of finding the solution for the design of Moon-to-Earth transfer orbit is greatly reduced. Numerical simulations indicate that the algorithm is of high efficiency and good robustness.
-
图 3 月地转移轨道3D示意图
Figure 3. Three-dimensional illustration of Moon-to- Earth transfer orbit
图 4 月地转移轨道2D示意图
Figure 4. Two-dimensional illustration of Moon-to- Earth transfer orbit
图 5 一个轨道周期内变轨速度增量变化趋势
Figure 5. Track change velocity increment versus time in one orbit period
表 1 初值设计时伪状态位置误差迭代过程
Table 1. Iteration process of pseudostate position error during initial solution design
迭代次数 Δx/m Δy/m Δz/m 1 7101140 -8735400 -3641240 2 453967 101658 104456 3 1622.07 279.576 393.42 4 102.868 13.296 21.020 5 5.799 0.804 1.249 6 0.350 0.0425 0.069 
下载: 导出CSV
表 2 精确解求解时再入点状态偏差迭代过程
Table 2. Iteration process of reentry point state error during exact solution search
迭代次数 ΔRe/m Δcos ie Δcos φe 1 413183 0.0112 0.0007 2 6385.410 0.0019 0.0015 3 321.208 0.00012 0 4 2.334 0 0
下载: 导出CSV
表 3 月地转移轨道特征参数
Table 3. Characteristic parameters of Moon-to-Earth transfer orbit
编号 再入
方式转移
时间/d再入点速度/
(m·s-1)再入
航程/km1 升轨 3.689 10.985 4969 2 升轨 3.871 10.984 10378 3 降轨 3.334 10.987 32748 4 降轨 3.516 10.985 38124
下载: 导出CSV
-
[1] 杨维廉, 周文艳.嫦娥一号月球探测卫星轨道设计[J].航天器工程, 2007, 16(6):16-24. http://d.old.wanfangdata.com.cn/Periodical/htqgc200706004YANG W L, ZHOU W Y.Orbit design for lunar exploration satellite CE1[J].Spacecraft Engineering, 2007, 16(6):16-24(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/htqgc200706004 [2] 李立涛, 杨涤, 崔祜涛.奔月轨道的一种求解方法[J].宇航学报, 2003, 24(2):150-155. http://d.old.wanfangdata.com.cn/Periodical/yhxb200302007LI L T, YANG D, CUI H T.An algorithm for the solution of trans-lunar trajectory[J].Journal of Astronautics, 2003, 24(2):150-155(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/yhxb200302007 [3] 周文艳, 杨维廉.月球探测器转移轨道的中途修正[J].宇航学报, 2004, 25(1):89-92. http://d.old.wanfangdata.com.cn/Periodical/yhxb200401015ZHOU W Y, YANG W L.Mid-correction of trans-lunar trajectory[J].Journal of Astronautics, 2004, 25(1):89-92(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/yhxb200401015 [4] OCAMPO C, SAUDEMONT R.Initial trajectory model for amulti-maneuver Moon-to-Earth abort sequence[J].Journal of Guidance, Control, and Dynamics, 2010, 33(4):1184-1194. doi: 10.2514/1.46955 [5] 高玉东, 郗晓宁, 白玉铸, 等.月球探测器返回轨道快速搜索设计[J].宇航学报, 2008, 29(3):765-771. http://d.old.wanfangdata.com.cn/Periodical/yhxb200803006GAO Y D, XI X N, BAI Y Z, et al.Fast searching design method for return transfer trajectory of lunar probe[J].Journal of Astronautics, 2008, 29(3):765-771(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/yhxb200803006 [6] 张磊, 于登云, 张熇.直接再入大气的月球返回轨道设计研究[J].航天器工程, 2010, 19(5):50-55. http://d.old.wanfangdata.com.cn/Periodical/htqgc201005009ZHANG L, YU D Y, ZHANG H.Design of moon return trajectory with direct atmospheric reentry[J].Spacecraft Engineering, 2010, 19(5):50-55(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/htqgc201005009 [7] 张磊, 于登云, 张熇.月地转移轨道快速设计与特性分析[J].中国空间科学技术, 2011, 31(3):62-70. http://d.old.wanfangdata.com.cn/Periodical/zgkjkxjs201103010ZHANG L, YU D Y, ZHANG H.Preliminary design and characteristic analysis of Moon-to-Earth transfer trajectories[J].Chinese Space Science and Technology, 2011, 31(3):62-70(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/zgkjkxjs201103010 [8] 郑爱武, 周建平, 刘勇.月地转移轨道快速设计方法[J].北京航空航天大学学报, 2014, 40(3):344-349. doi: 10.13700/j.bh.1001-5965.2013.0241ZHENG A W, ZHOU J P, LIU Y.Fast design method of Moon-to-Earth transfer trajectory[J].Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(3):344-349(in Chinese). doi: 10.13700/j.bh.1001-5965.2013.0241 [9] 郑爱武, 周建平.月地转移轨道精确轨道设计[J].飞行器测控学报, 2014, 33(1):52-58. http://d.old.wanfangdata.com.cn/Periodical/fxqckxb201401010ZHENG A W, ZHOU J P.Accurate design of Moon-to-Earth transfer orbit[J].Journal of Spacecraft TT&C Technology, 2014, 33(1):52-58(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/fxqckxb201401010 [10] 郑爱武, 周建平.直接再入大气的月地返回窗口搜索策略[J].航空学报, 2014, 35(8):2243-2250. http://d.old.wanfangdata.com.cn/Periodical/hkxb201408018ZHENG A W, ZHOU J P.A search strategy of back windows for Moon-to-Earth trajectories directly returning to the earth[J].Acta Aeronautica et Astronautica Sinica, 2014, 35(8):2243-2250(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/hkxb201408018 [11] 汪中生, 孟占峰, 高珊, 等.探测器月地转移入射变轨策略优化设计[J].航天器工程, 2019, 28(1):10-18. http://d.old.wanfangdata.com.cn/Periodical/htqgc201901002WANG Z S, MENG Z F, GAO S, et al.Optimization design of spacecraft trans-earth insertion strategy[J].Spacecraft Engineering, 2019, 28(1):10-18(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/htqgc201901002 [12] WILSON S W.A pseudostate theory for the approximation of three-body trajectories: AIAA-70-1061[R].Reston: AIAA, 1970. https://www.researchgate.net/publication/23621131_A_pseudostate_theory_for_the_approximation_of_three-body_trajectories [13] LUO Q, MENG Z, HAN C.Solution algorithm to a quasi-Lambert's problem with fixed flight-direction angle constraint[J].Celestial Mechanics and Dynamical Astronomy, 2011, 108(4):409-427. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=36bff6e83af1b406842b090577007c44 [14] ZIMMER S, OCAMPO C.Analytical gradients for gravity assist trajectories using constant specific impulse engines[J].Journal of Guidance, Control, and Dynamics, 2005, 28(4):753-760. http://cn.bing.com/academic/profile?id=3408f30422cb9dd1bef3058f2f0437cf&encoded=0&v=paper_preview&mkt=zh-cn [15] OCAMPO C, MUNOZ J P.Variational model for the optimization of constrained finite-burn escape sequences[J].Journal of Guidance, Control, and Dynamics, 2010, 33(5):1615-1621. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=CC0211535087 -
下载:
点击查看大图
计量
- 文章访问数: 756
- HTML全文浏览量: 213
- PDF下载量: 202
- 被引次数: 0
