3-PPP型柔性并联微定位平台的设计与分析

王保兴; 孟刚; 林苗; 李巍; 曹毅

doi:10.13700/j.bh.1001-5965.2019.0286

3-PPP型柔性并联微定位平台的设计与分析

doi: 10.13700/j.bh.1001-5965.2019.0286

王保兴¹,
孟刚¹,
林苗¹,
李巍²,
曹毅^{1, 3, ,}

1.
江南大学机械工程学院, 无锡 21412
2.
苏州工业职业技术学院, 苏州 215104
3.
江苏省食品先进制造装备技术重点实验室, 无锡 214122

基金项目:

江苏省“六大人才高峰”计划 ZBZZ-012

高等学校学科创新引智计划 B18027

江苏省研究生创新计划 SJCX18-0630

江苏省研究生创新计划 KYCX18-1846

详细信息

作者简介:
王保兴男, 硕士研究生。主要研究方向:柔性机构学

孟刚男, 硕士研究生。主要研究方向:柔性机构学

林苗男, 硕士研究生。主要研究方向:柔性机构学

李巍男, 博士。主要研究方向:软体机器人

曹毅男, 博士, 教授。主要研究方向:并联机器人、混联机器人、柔性机器人、软体机器人

通讯作者:
曹毅, E-mail: caoyi@jiangnan.edu.cn

中图分类号: V414.5;TH122
计量
- 文章访问数: 861
- HTML全文浏览量: 89
- PDF下载量: 444
- 被引次数: 0
出版历程
- 收稿日期: 2019-06-10
- 录用日期: 2019-08-30
- 网络出版日期: 2020-04-20

Design and analysis of a 3-PPP compliant parallel micro-positioning stage

WANG Baoxing¹,
MENG Gang¹,
LIN Miao¹,
LI Wei²,
CAO Yi^{1, 3
, ,}

1.
School of Mechanical Engineering, Jiangnan University, Wuxi 21412
2.
Suzhou Vocational Institute of Industrial Technology, Suzhou 215104, China
3.
Jiangsu Key Laboratory of Advanced Food Manufacturing Equipment and Technology, Wuxi 214122, China

Funds:

The Six Talent Peaks Project in Jiangsu Province ZBZZ-012

"111" Project B18027

Postgraduate Research and Practice Innovation Program of Jiangsu Provence SJCX18-0630

Postgraduate Research and Practice Innovation Program of Jiangsu Provence KYCX18-1846

More Information

Corresponding author: CAO Yi, E-mail: caoyi@jiangnan.edu.cn

摘要

摘要:
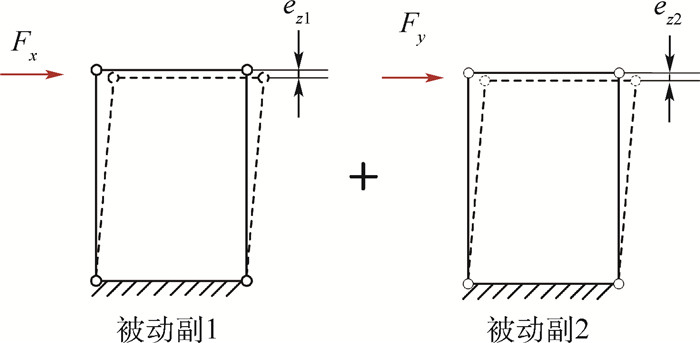
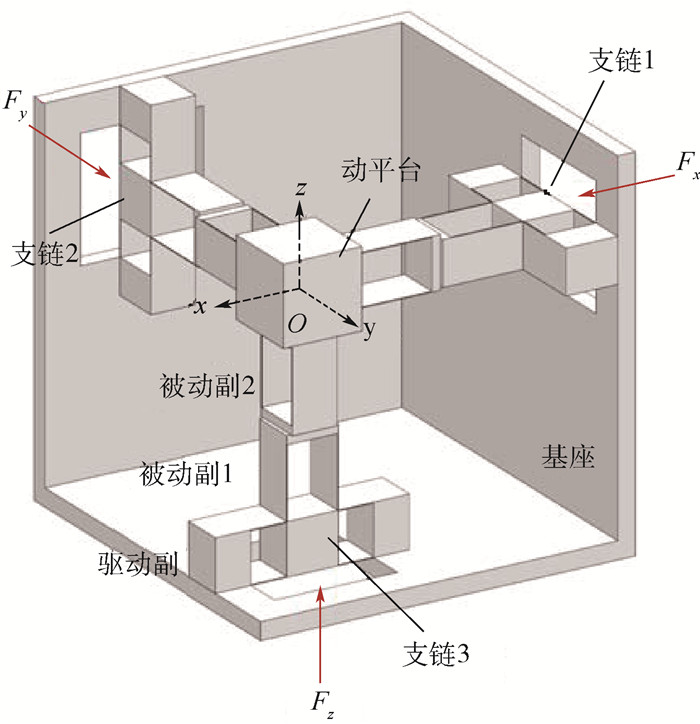
为解决现有空间平动柔性并联微定位平台（CPMS）结构布局不紧凑，且多轴驱动时各运动副的寄生运动相互累加，导致平台耦合误差增大的问题。首先，设计了一种基于柔性薄板的分布柔度式3-PPP型柔性并联微定位平台。其次，通过结构优化减小了平台的体积，并消除了支链中移动副寄生运动的累加现象。然后，基于柔度矩阵法建立了平台的输入刚度理论模型，并采用有限元仿真验证了理论模型的正确性；同时计算了平台的固有频率，并探究了其与柔性薄板尺寸参数之间的关系。最后，将结构优化前后的平台通过有限元仿真进行了对比分析。结果表明：结构优化后平台的体积减小了67%，且平台在单轴和多轴驱动时均具有更优的运动解耦特性和输入输出一致性。
- 柔性并联微定位平台(CPMS) /
- 柔度矩阵 /
- 耦合误差 /
- 固有频率 /
- 有限元仿真
Abstract:
The structure layouts of the existing spatial translational compliant parallel micro-positioning stages are not compact, and the parasitic motion of each kinematic joint accumulates during multi-axis actuation, which leads to the augment of cross-axis coupling error. In order to solve these problems, first, a distributed-compliance 3-PPP spatial translational compliant parallel micro-positioning stage (CPMS) based on compliant sheet was designed. Secondly, the stage volume was reduced, and the parasitic motion accumulation phenomenon of kinematic joints in each limb was eliminated by the way of structure optimization. Then, the theoretical model of input stiffness was deduced through compliance matrix method. The validity of the theoretical model was proved by finite element simulation. Besides, the natural frequency of the CPMS was calculated, and the relationship between natural frequency of the CPMS and size parameters of compliant sheet was explored. Finally, comparative analysis of the CPMS before and after structure optimization was conducted by finite element simulation. The results show that the volume of the CPMS is reduced by 67% after structure optimization, and the CPMS has better kinematic decoupling characteristic and input output consistency in both single-axis and multi-axis actuation.
- compliant parallel micro-positioning stage (CPMS) /
- compliance matrix /
- coupling error /
- natural frequency /
- finite element simulation

HTML全文

图 1 3-PPP型柔性并联微定位平台的初始结构

Figure 1. Original structure of a 3-PPP compliant parallel micro-positioning stage

下载: 全尺寸图片幻灯片

图 2 支链3中被动副的变形示意图

Figure 2. Schematic diagram of deformation of passive joints in limb 3

下载: 全尺寸图片幻灯片

图 3 反向串联方法示意图

Figure 3. Schematic diagram of inversion series method

下载: 全尺寸图片幻灯片

图 4 结构优化后的柔性支链

Figure 4. One compliant limb after structure optimization

下载: 全尺寸图片幻灯片

图 5 结构优化后的微定位平台

Figure 5. Micro-positioning stage after structure optimization

下载: 全尺寸图片幻灯片

图 6 一端固定的等截面梁

Figure 6. A constant section beam with one end fixed

下载: 全尺寸图片幻灯片

图 7 z轴方向的柔性支链

Figure 7. Compliant limb in z-axis direction

下载: 全尺寸图片幻灯片

图 8 驱动力为50 N时平台的仿真结果

Figure 8. Simulation result of stage with 50 N actuating force

下载: 全尺寸图片幻灯片

图 9 驱动力与参考点位移之间的关系

Figure 9. Relationship between actuating force and reference point displacement

下载: 全尺寸图片幻灯片

图 10 平台的1~6阶模态振型

Figure 10. 1-6 order mode shapes of stage

下载: 全尺寸图片幻灯片

图 11 平台的固有频率与柔性薄板尺寸参数之间的关系

Figure 11. Relationship between natural frequency of stage and compliant sheet size parameters

下载: 全尺寸图片幻灯片

图 12 不同输入条件下平台的仿真分析

Figure 12. Simulation analysis of stage under different input conditions

下载: 全尺寸图片幻灯片

图 13 单轴驱动条件下的仿真结果

Figure 13. Simulation results with single-axis actuation

下载: 全尺寸图片幻灯片

图 14 两轴驱动时平台在z轴方向的耦合位移

Figure 14. Coupling displacement of stage in z-axis direction with two-axis actuation

下载: 全尺寸图片幻灯片

图 15 三轴驱动时平台的丢失运动

Figure 15. Lost motion of stage with three-axis actuation

下载: 全尺寸图片幻灯片

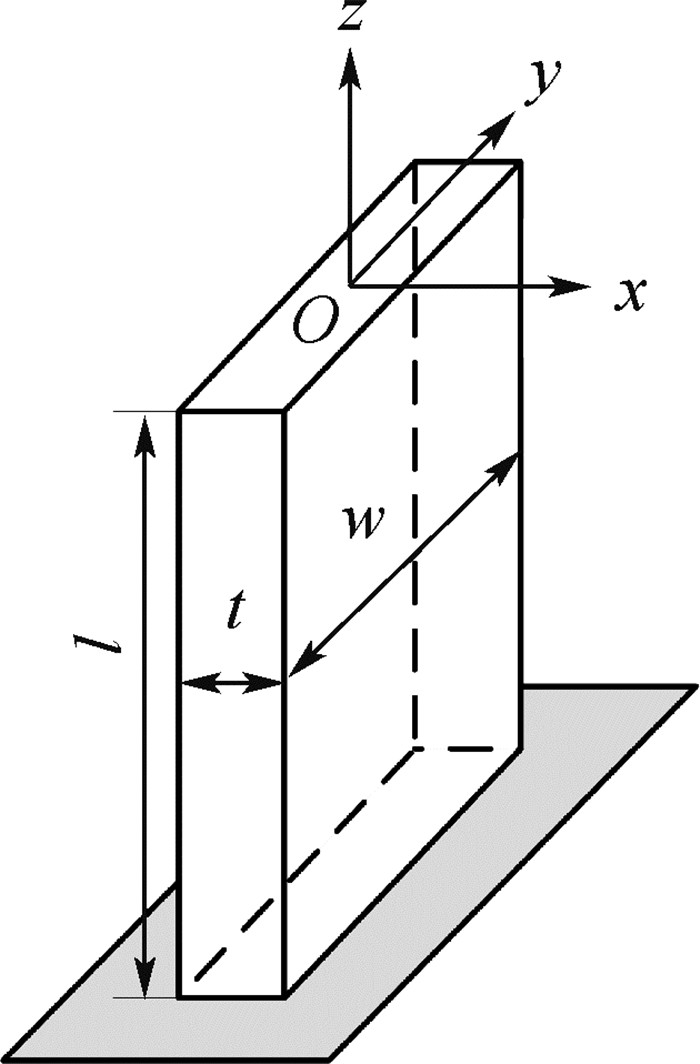
表 1 平台的尺寸参数

Table 1. Dimension parameters of stage

参数	数值/mm
t	0.5
w	25
l	40
H	59
u1	45.5
u2	28.5
s	44

下载: 导出CSV

表 2 参考点位移的矩阵法计算值、仿真值及相对误差

Table 2. Matrix method calculation values, simulation values and relative error of reference point displacement

驱动力/N	参考点位移/μm		相对误差/%
驱动力/N	理论计算值	仿真值	相对误差/%
10	70.74	73.15	3.41
20	141.47	143.36	1.33
30	212.21	208.78	1.62
40	282.94	268.73	5.02
50	353.68	323.36	8.57

下载: 导出CSV

表 3 参考点位移非线性法计算值、仿真值及相对误差

Table 3. Nonlinear method calculation values, simulation values and relative error of reference point displacement

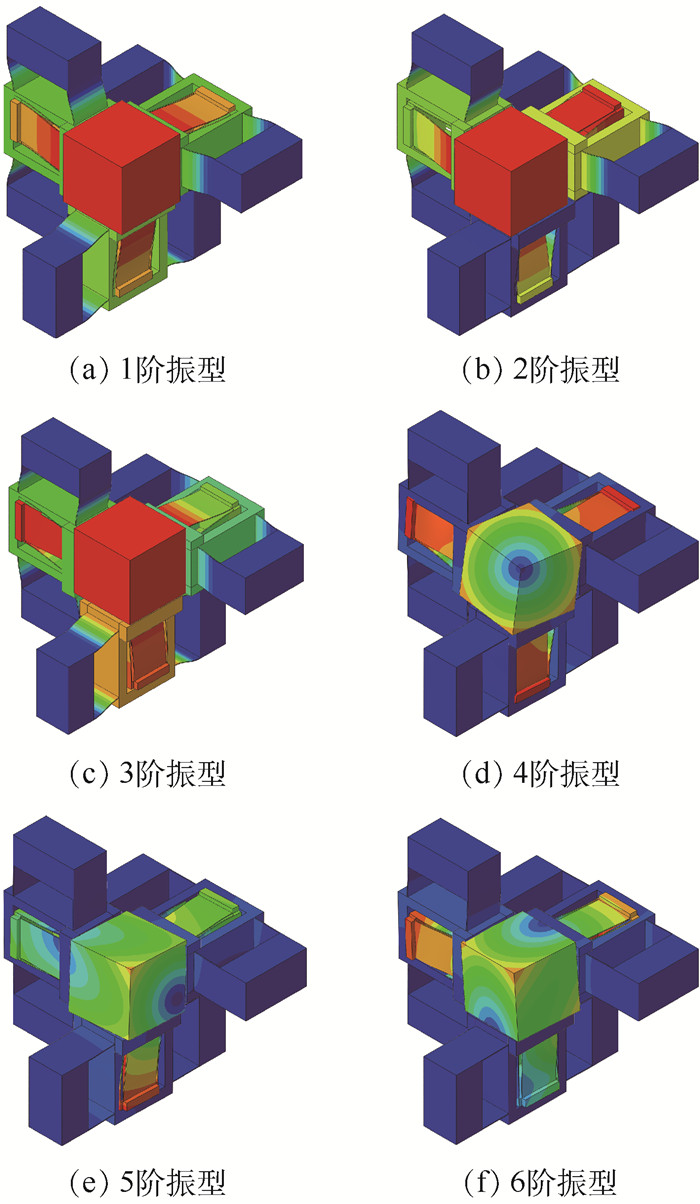
驱动力/N	参考点位移/μm		相对误差/%
驱动力/N	理论计算值	仿真值	相对误差/%
10	70.1	73.15	4.35
20	136.9	143.36	4.72
30	198.3	208.78	5.28
40	253.8	268.73	5.88
50	303.7	323.36	6.47

下载: 导出CSV

表 4 平台有限元分析的固有频率

Table 4. Natural frequency of stage obtained by finite element analysis

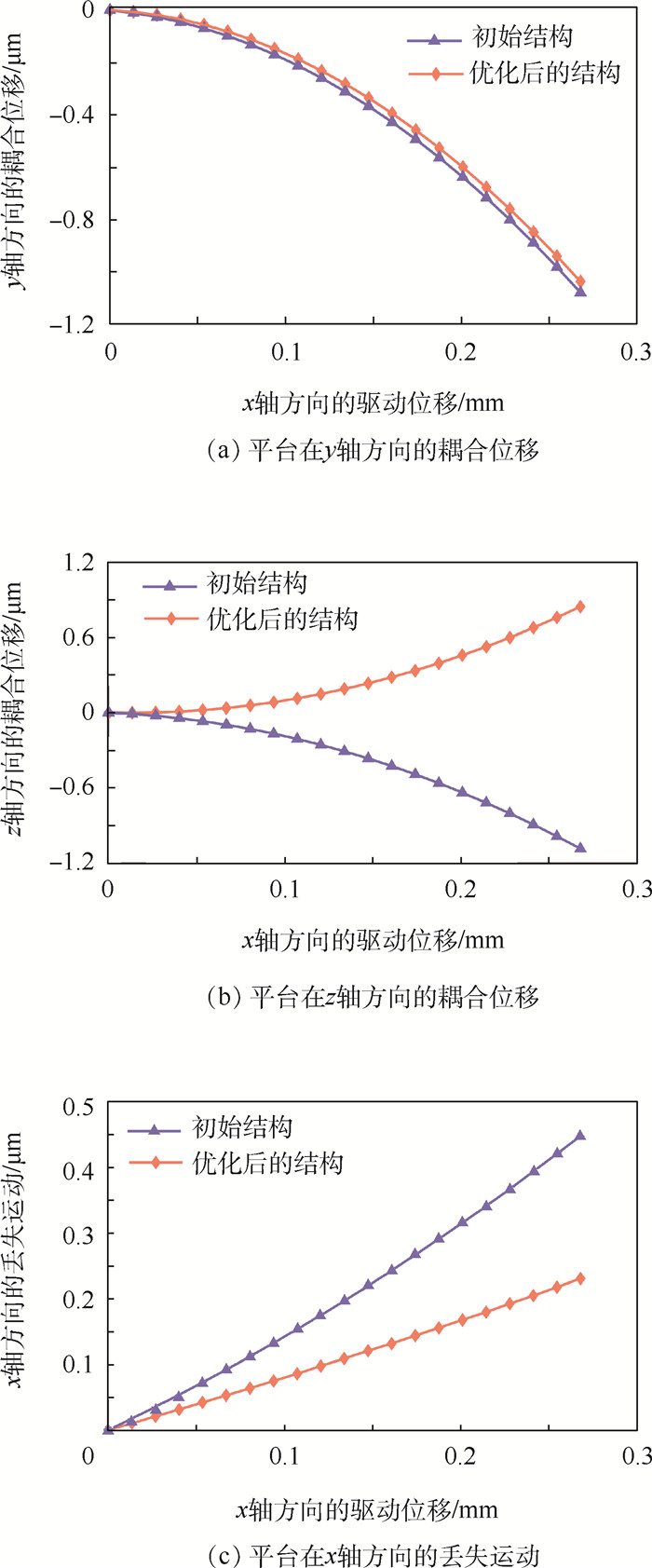
阶数	1阶	2阶	3阶	4阶	5阶	6阶
频率/Hz	94.49	94.49	94.49	694.13	700	700.13

下载: 导出CSV

表 5 单轴驱动时参考点的耦合位移与丢失运动

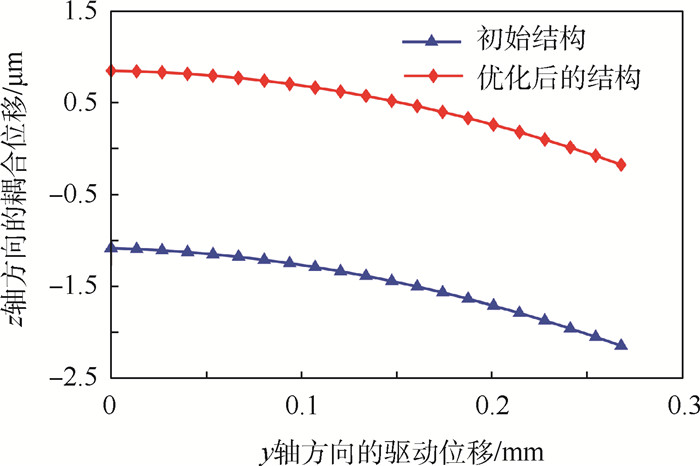
Table 5. Coupling displacement and lost motion of reference point with single-axis actuation μm

平台结构	y轴方向的最大耦合位移	z轴方向的最大耦合位移	x轴方向的最大丢失运动
初始结构	-1.08	-1.09	0.45
优化后的结构	-1.04	0.85	0.23

下载: 导出CSV

参考文献(31)

[1]	HOWELL L L.Compliant mechanisms[M].New York:John Wiley and Sons, 2001:2-14.
[2]	张宪民, 胡凯, 王念峰, 等.基于并行策略的多材料柔顺机构多目标拓扑优化[J].机械工程学报, 2016, 52(19):1-8. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201619001 ZHANG X M, HU K, WANG N F, etal.Multi-objectivetopology optimization of multiple materials compliant mechanisms based on parallel strategy[J].Journal of Mechanical Engineering, 2016, 52(19):1-8(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201619001
[3]	周睿, 周辉, 桂和利, 等.基于柔性铰链的二自由度微动平台分析及优化[J].北京航空航天大学学报, 2018, 44(9):199-207. doi: 10.13700/j.bh.1001-5965.2017.0706 ZHOU R, ZHOU H, GUI H L, et al.Analysis and optimization of 2-DoF micro-positioning stage based on flexible hinges[J].Journal of Beijing University of Aeronautics and Astronautics, 2018, 44(9):199-207(in Chinese). doi: 10.13700/j.bh.1001-5965.2017.0706
[4]	于靖军, 郝广波, 陈贵敏, 等.柔性机构及其应用研究进展[J].机械工程学报, 2015, 51(13):53-68. http://d.old.wanfangdata.com.cn/Periodical/jxgcxb201513006 YU J J, HAO G B, CHEN G M, et al.State-of-art of compliant mechanisms and their applications[J].Journal of Mechanical Engineering, 2015, 51(13):53-68(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/jxgcxb201513006
[5]	PAOLO D G, ALVISE B, PIERLUIGI B.New MEMS tweezers for the viscoelastic characterization of soft materials at the microscale[J].Micromachines, 2018, 9(1):15-37. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=MDPI000000179845
[6]	DSOUZA R D, NAVIN K P, THEODORIDIS T.Design fabrication and testing of a 2 DOF compliant flexural microgripper[J].Microsystem Technologies, 2018, 24(9):3867-3883. doi: 10.1007/s00542-018-3861-y
[7]	曹毅, 刘凯, 桂和利, 等.二自由度开口型空间夹持机构研究[J].机械工程学报, 2018, 54(11):94-101. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201811009 CAO Y, LIU K, GUI H L, et al.The research of one two-degree-of-freedom spatial gripper with opening[J].Journal of Mechanical Engineering, 2018, 54(11):94-101(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201811009
[8]	LIN C, WU Z H, REN Y H, et al.Characteristic analysis of unidirectional multi-driven and large stroke micro/nano-transmission platform[J].Microsystem Technologies, 2017, 23(8):3389-3400. doi: 10.1007/s00542-016-3130-x
[9]	ÖZKALE B, PARREIRA R, BEKDEMIR A, et al.Modular soft robotic microdevices for dexterous biomanipulation[J].Lab on a Chip, 2019, 19(5):778-788. doi: 10.1039/C8LC01200H
[10]	LI C, WANG J, CHEN S C.Flexure-based dynamic-tunable five-axis nanopositioner for parallel nanomanufacturing[J].Precision Engineering, 2016, 45:423-434. doi: 10.1016/j.precisioneng.2016.04.002
[11]	YONG Y K, MOHEIMANI S O R, KENTON B J, et al.Invited review article:High-speed flexure-guided nanopositioning:Mechanical design and control issues[J].Review of Scientific Instruments, 2012, 83(12):121101. doi: 10.1063/1.4765048
[12]	ZHU Z, TO S, ZHU W L, et al.Optimum design of a piezo-actuated triaxial compliant mechanism for nanocutting[J].IEEE Transactions on Industrial Electronics, 2018, 65(8):6362-6371. doi: 10.1109/TIE.2017.2787592
[13]	WATANABE S, ANDO T.High-speed XYZ-nanopositioner for scanning ion conductance microscopy[J].Applied Physics Letters, 2017, 111(11):113106. doi: 10.1063/1.4993296
[14]	HAO G B.Towards the design of monolithic decoupled XYZ compliant parallel mechanisms for multi-function applications[J].Mechanical Sciences, 2013, 4(2):291-302. doi: 10.5194/ms-4-291-2013
[15]	李海洋, 郝广波, 于靖军, 等.空间平动柔性并联机构的系统设计方法研究[J].机械工程学报, 2018, 54(13):57-65. http://d.old.wanfangdata.com.cn/Periodical/jxgcxb201813006 LI H Y, HAO G B, YU J J, et al.Systematic approach to the design of spatial translational compliant parallel mechanisms[J].Journal of Mechanical Engineering, 2018, 54(13):57-65(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/jxgcxb201813006
[16]	TANG X Y, CHEN I M, LI Q.Design and nonlinear modeling of a large-displacement XYZ flexure parallel mechanism with decoupled kinematic structure[J].Review of Scientific Instruments, 2006, 77(11):115101. doi: 10.1063/1.2364132
[17]	AWTAR S, USTICK J, SEN S.An XYZ parallel-kinematic flexure mechanism with geometrically decoupled degrees of freedom[J].Journal of Mechanisms and Robotics, 2012, 5(1):015001.
[18]	LI Y M, XU Q S.Design and optimization of an XYZ parallel micromanipulator with flexure hinges[J].Journal of Intelligent and Robotic Systems, 2009, 55(4-5):377-402. doi: 10.1007/s10846-008-9300-z
[19]	YUE Y, GAO F, ZHAO X, et al.Relationship among input-force payload stiffness and displacement of a 3-DOF perpendicular parallel micro-manipulator[J].Mechanism and Machine Theory, 2010, 45(5):756-771. doi: 10.1016/j.mechmachtheory.2009.12.006
[20]	WANG N, ZHANG Z Y, ZHANG X M, et al.Optimization of a 2-DOF micro-positioning stage using corrugated flexure units[J].Mechanism and Machine Theory, 2018, 121:683-696. doi: 10.1016/j.mechmachtheory.2017.11.021
[21]	CHAO D H, ZONG G H, LIU R.Design of a 6-DOF compliant manipulator based on serial-parallel architecture[C]//2005 IEEE/ASME International Conference on Advanced Intelligent Mechatronics.Piscataway, NJ: IEEE Press, 2005: 765-770.
[22]	KIM D, GWEON D G, SONG I, et al.Optimal design of a flexure hinge-based XYZ atomic force microscopy scanner for minimizing Abbe errors[J].Review of Scientific Instruments, 2005, 76(7):376-391. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=48cec0eac2a708eaa91eb54e93499f02
[23]	XU Q S, ZHU X B, DONG Z G, et al.Nonlinear modeling and analysis of compliant mechanisms with circular flexure hinges based on quadrature beam elements[J].Proceedings of the Institution of Mechanical Engineers, Part C:Journal of Mechanical Engineering Science, 2019, 233(9):3277-3285. doi: 10.1177/0954406218802945
[24]	LI Y M, WU Z G.Design analysis and simulation of a novel 3-DOF translational micromanipulator based on the PRB model[J].Mechanism and Machine Theory, 2016, 100:235-258. doi: 10.1016/j.mechmachtheory.2016.02.001
[25]	SHE Y, MENG D S, SU H J, et al.Introducing mass parameters to pseudo-rigid-body models for precisely predicting dynamics of compliant mechanisms[J].Mechanism and Machine Theory, 2018, 126:273-294. doi: 10.1016/j.mechmachtheory.2018.04.005
[26]	KOSEKI Y, TANIKAWA T, KOYACHI N, et al.Kinematic analysis of translational 3-DOF micro parallel mechanism using matrix method[J].IEEE/RSJ International Conference on Intelligent Robots and Systems, 2000, 1(3):786-792. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=CC026715134
[27]	TANG H, LI Y M.Design analysis and test of a novel 2-DOF nanopositioning system driven by dual mode[J].IEEE Transactions on Robotics, 2013, 29(3):650-662. doi: 10.1109/TRO.2013.2248536
[28]	余跃庆, 张亚涛, 张绪平, 等.柔顺微夹持机构理论分析与实验[J].农业机械学报, 2018, 49(11):393-398. http://d.old.wanfangdata.com.cn/Periodical/nyjxxb201811047 YU Y Q, ZHANG Y T, ZHANG X P, et al.Theoretical analysis and experiment on compliant microgripper mechanism[J].Transactions of the Chinese Society for Agricultural Machinery, 2018, 49(11):393-398(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/nyjxxb201811047
[29]	HAO G B, LI H Y, KEMALCAN S, et al.Understanding coupled factors that affect the modelling accuracy of typical planar compliant mechanisms[J].Frontiers of Mechanical Engineering, 2016, 11(2):129-134. doi: 10.1007/s11465-016-0392-z
[30]	杨志军, 白有盾, 陈新, 等.基于应力刚化效应的动态特性可调微动平台设计新方法[J].机械工程学报, 2015, 51(23):153-159. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201523019 YANG Z J, BAI Y D, CHEN X, et al.A new design method of dynamic characteristics adjustable micro motion stage based on tension stiffening[J].Journal of Mechanical Engineering, 2015, 51(23):153-159(in Chinese). http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jxgcxb201523019
[31]	XAVIER H, ROSS W, KONG X W.On a simplified nonlinear analytical model for the characterization and design optimization of a compliant XY micro-motion stage[J].Robotics and Computer-Integrated Manufacturing, 2018, 49:66-76. doi: 10.1016/j.rcim.2017.05.012