脉冲星方位误差估计的TSKF算法

许强; 范小虎; 徐利国; 王宏力; 冯磊

doi:10.13700/j.bh.1001-5965.2019.0288

脉冲星方位误差估计的TSKF算法

doi: 10.13700/j.bh.1001-5965.2019.0288

1.
青州高新技术研究所测试控制系, 潍坊 262500
2.
火箭军工程大学导弹工程学院, 西安 710025

基金项目:

国家自然科学基金 61503391

中国博士后科学基金 2017M613372

详细信息

作者简介:
许强, 男, 硕士, 助教。主要研究方向:导航、制导与仿真

范小虎, 男, 副教授。主要研究方向:导航、制导与仿真

冯磊, 男, 博士, 讲师。主要研究方向:导航、制导与仿真

通讯作者:
冯磊, E-mail: fengl1983@126.com

中图分类号: V324.2⁺1;P129
计量
- 文章访问数: 716
- HTML全文浏览量: 121
- PDF下载量: 268
- 被引次数: 0
出版历程
- 收稿日期: 2019-06-10
- 录用日期: 2019-09-12
- 网络出版日期: 2020-04-20

TSKF algorithm for pulsar position error estimation

1.
Department of Test and Control, Qingzhou Research Institute of High-technology, Weifang 262500, China
2.
College of Missile Engineering, Rocket Force University of Engineering, Xi'an 710025, China

Funds:

National Natural Science Foundation of China 61503391

China Postdoctoral Science Foundation 2017M613372

More Information

Corresponding author: FENG Lei, E-mail: fengl1983@126.com

摘要

摘要:
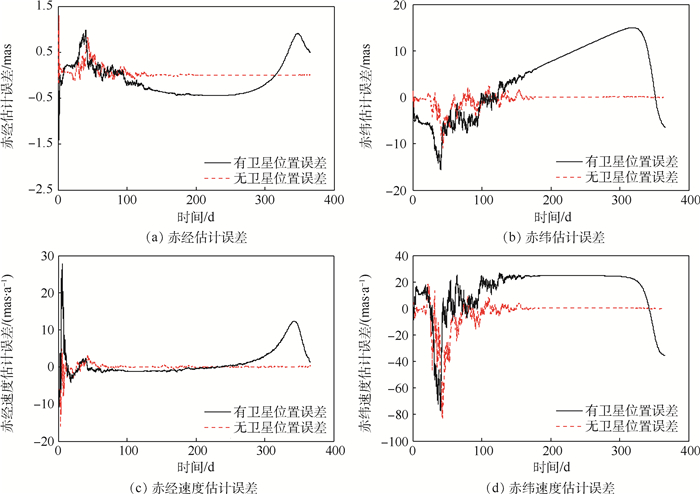
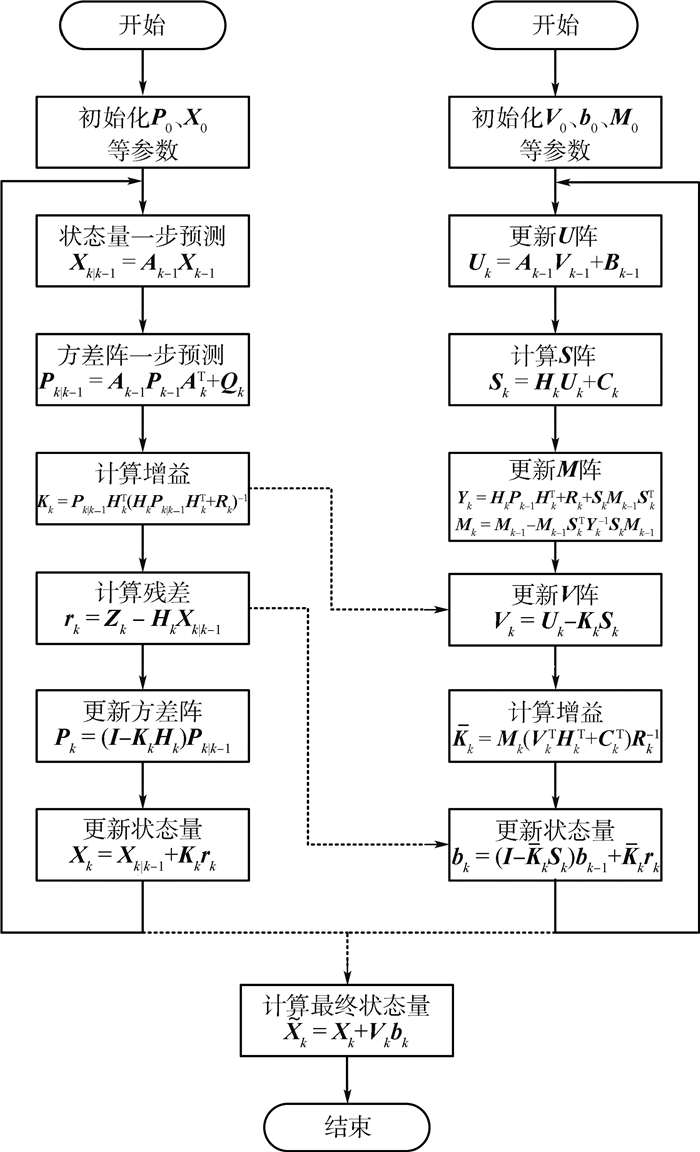
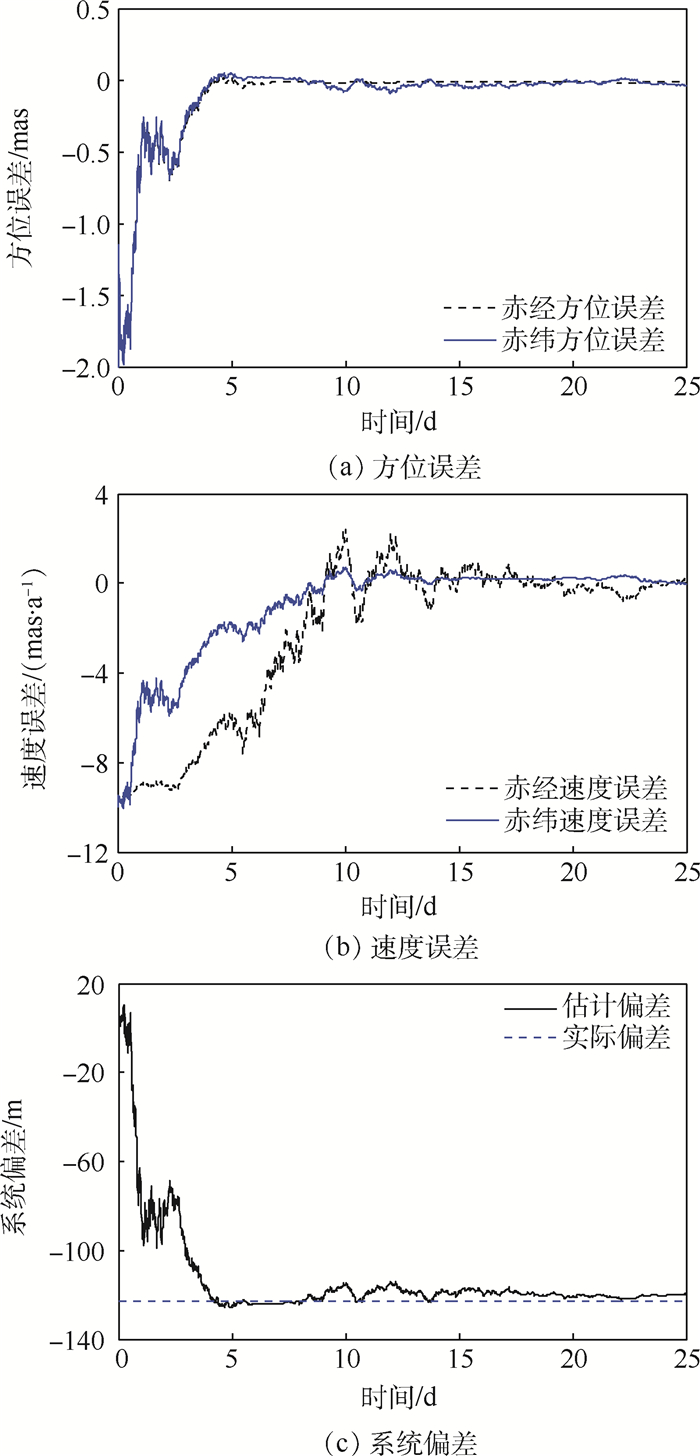
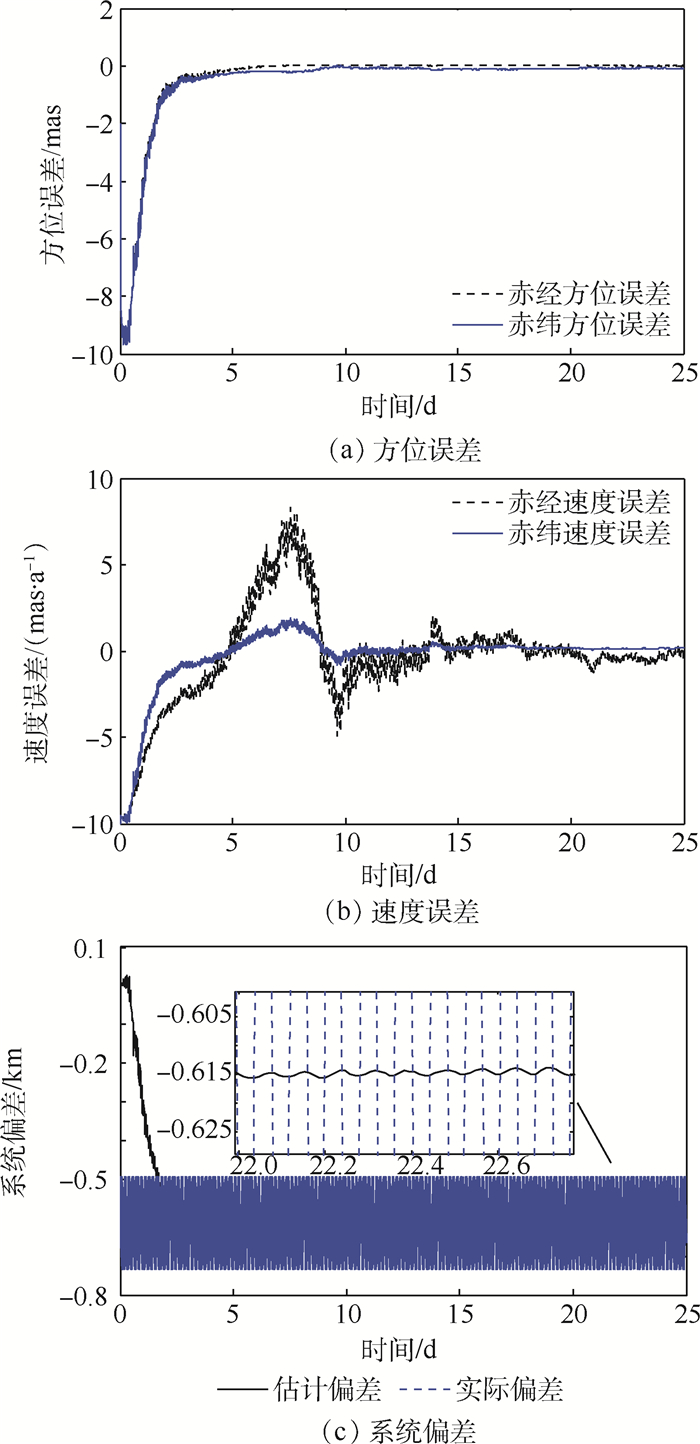
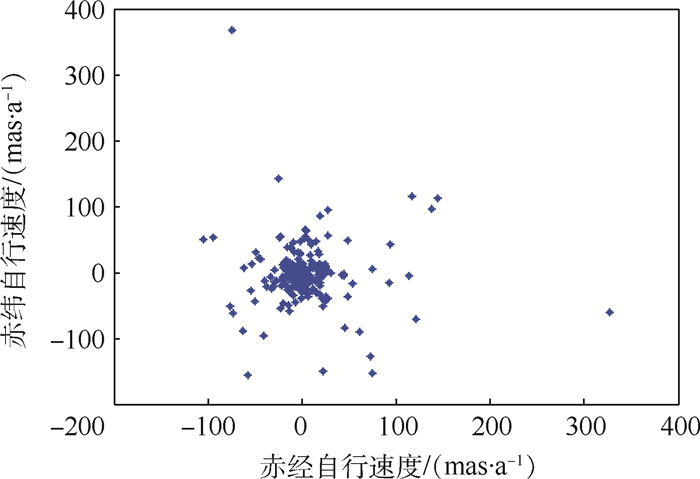
为提高脉冲星方位误差估计对方位自行速度及卫星位置误差的鲁棒性和整体运算的高效性，设计了两级卡尔曼滤波（TSKF）算法。首先，分析了方位自行速度及卫星位置误差对方位误差估计的影响，并分别结合相关算法进行了仿真验证。然后，结合方位误差估计的CV模型和两级卡尔量滤波的相关原理，写出了TSKF算法的更新方程，并分析了实现并行计算的基本流程。仿真实验的数据显示：在方位自行速度及卫星位置误差均存在的情况下，TSKF算法的方位估计精度约为0.1 mas，方位自行速度估计精度约为1.1 mas/a；与基于CV模型的估计算法相比，TSKF算法的浮点运算仅增加了0.048%。
- 方位误差 /
- 方位自行速度 /
- 卫星位置误差 /
- 卡尔曼滤波 /
- 两级滤波
Abstract:
In order to improve the robustness of the pulsar position error estimation to the proper motion and satellite position error and the efficiency of the overall algorithm, a two-stage Kalman filter (TSKF) algorithm is designed. Firstly, the influences of pulsar proper motion and satellite position error on pulsar error estimation are analyzed, and the simulation results are verified by combining relevant algorithms. Secondly, based on the CV model and the principle of two-stage Kalman filter, the update equations of TSKF algorithm are derived, and the basic flow of parallel computing is analyzed. The data of the simulation experiment show that position accuracy of the TSKF algorithm is about 0.1 mas and corresponding proper motion accuracy is about 1.1 mas/a in the case of both proper motion and satellite position error. Compared with the estimation algorithm based on CV model, the floating point operation of TSKF algorithm only increases by 0.048%.
- position error /
- proper motion /
- satellite position error /
- Kalman filter /
- two-stage filter

HTML全文

图 1 脉冲星方位自行速度分布

Figure 1. Proper motion distribution of pulsars

下载: 全尺寸图片幻灯片

图 2 脉冲星方位自行速度不确定度分布

Figure 2. Proper motion uncertainty distribution of pulsars

下载: 全尺寸图片幻灯片

图 3 不同条件下增广算法仿真结果

Figure 3. Simulation results of augmented algorithm under different conditions

下载: 全尺寸图片幻灯片

图 4 卫星位置误差对估计算法的影响原理

Figure 4. Principle of influence of satellite position error on estimation algorithm

下载: 全尺寸图片幻灯片

图 5 不同条件下基于CV模型的估计算法仿真结果

Figure 5. Simulation results of estimation algorithm based on CV model under different conditions

下载: 全尺寸图片幻灯片

图 6 并行计算流程图

Figure 6. Parallel computing flowchart

下载: 全尺寸图片幻灯片

图 7 TSKF算法仿真过程

Figure 7. Simulation process of TSKF algorithm

下载: 全尺寸图片幻灯片

图 8 卫星位置误差周期变化时TSKF算法仿真结果

Figure 8. Simulation results of TSKF algorithm when satellite position error period changes

下载: 全尺寸图片幻灯片

表 1 脉冲星B1821-24参数

Table 1. Parameters of pulsar B1821-24

参数	数值
赤经/(°)	276.13
赤纬/(°)	-24.87
距离/(10²⁰m)	1.694
脉冲周期P/(10^-3s)	3.045
脉冲宽度W/(10^-5s)	5.5
光子流量F_x/(10^-4ph·(cm²·s)^-1)	1.93
脉冲辐射流量与平均辐射流量之比P_f/%	98

下载: 导出CSV

表 2 仿真条件设置

Table 2. Simulation condition setup

实验编号	方位自行速度/ (mas·a^-1)	卫星位置误差/m
1	(0, 0)	(100, 100, 100)
2	(10, 10)	(0, 0, 0)
3	(-10, 10)	(100, 100, 100)
4	(20, 20)	(100, 100, 100)
5	(10, 10)	(100, -100, 100)
6	(10, 10)	(500, 500, 500)

下载: 导出CSV

表 3 仿真结果统计

Table 3. Simulation result statistics

实验编号	方位误差/mas		速度误差/(mas·a^-1)
实验编号	赤经	赤纬	赤经	赤纬
1	-0.003 9	-0.005 8	0.016 9	0.201 2
2	0.004 9	-0.024 6	-0.003 6	-0.542 9
3	0.024 1	-0.106 4	0.254 6	-1.013 5
4	0.004 9	0.014 3	-0.159 6	0.116 7
5	-0.005 6	0.092 9	-0.103 4	1.081 4
6	-0.001 6	-0.113 8	0.020 8	0.142 7

下载: 导出CSV

表 4 卫星位置误差周期变化时仿真结果统计

Table 4. Simulation result statistics when satellite position error period changes

实验条件/m			方位误差/mas		速度误差/(mas·a^-1)
L_x	L_y	L_z	赤经	赤纬	赤经	赤纬
100	100	100	0.015 1	-0.077 6	-0.085 6	0.153 0
200	200	200	-0.016 6	-0.121 9	-0.025 2	0.059 7
300	300	300	-0.020 5	-0.132 4	-0.047 3	0.103 7
100	200	300	-0.008 3	-0.138 5	-0.059 4	0.126 7
300	200	100	0.015 4	-0.075 5	-0.051 4	0.092 8

下载: 导出CSV

参考文献(16)

[1]	GRAVEN P, COLLINS J, SHEIKH S, et al.XNAV for deep space navigation[J].Advances in the Astronautical Sciences, 2008, 131:349-364.
[2]	SHEIKH S I, HELLINGS R W, MATZNER R A.High-order pulsar timing for navigation[C]//Proceedings of Annual Meeting of the Institute of Navigation.Manassas: Institute of Navigation, 2007: 432-443.
[3]	YOU S, WANG H, HE Y, et al.Pulsar profile construction based on double-redundant-dictionary and same-scale L₁-norm compressed sensing[J].Optik, 2018, 164:617-623. doi: 10.1016/j.ijleo.2018.03.066
[4]	TAO A, HONG X Y, ZHENG W M, et al.Space very long baseline interferometry in China[J].Advances in Space Research, 2020, 65(2):850-855. doi: 10.1016/j.asr.2019.03.030
[5]	WAJIMA K, HAGIWARA Y, TAO A, et al.The East-Asian VLBI network[J].Physics, 2015, 502:403-413. http://d.old.wanfangdata.com.cn/OAPaper/oai_arXiv.org_1311.3431
[6]	NING X, GUI M, FANG J, et al.Differential X-ray pulsar aided celestial navigation for Mars exploration[J].Aerospace Science & Technology, 2017, 62:36-45. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=f49a60e0267b8e11f7b03dcbac2bf3a0
[7]	XU Q, WANG H, FENG L, et al.An improved augmented X-ray pulsar navigation algorithm based on the norm of pulsar direction error[J].Advances in Space Research, 2018, 62(11):3187-3198. doi: 10.1016/j.asr.2018.08.026
[8]	孙守明, 郑伟, 汤国建.X射线脉冲星星表方位误差估计算法研究[J].飞行器测控学报, 2010, 29(2):57-60. http://d.old.wanfangdata.com.cn/Periodical/fxqckxb201002013 SUN S M, ZHENG W, TANG G J.A new estimation algorithm of the X-ray pulsar position error[J].Journal of Spacecraft TT & CC Technology, 2010, 29(2):57-60(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/fxqckxb201002013
[9]	王宏力, 许强, 由四海, 等.考虑卫星位置误差的增广脉冲星方位误差估计算法[J].国防科技大学学报, 2018, 40(5):177-182. http://d.old.wanfangdata.com.cn/Periodical/gfkjdxxb201805026 WANG H L, XU Q, YOU S H, et al.Augmented estimation algorithm for pulsar position error with satellite position error[J].Journal of National University of Defense Technology, 2018, 40(5):177-182(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/gfkjdxxb201805026
[10]	孙守明, 郑伟, 汤国建.基于CV模型的X射线脉冲星位置误差估计[J].系统仿真学报, 2010, 22(11):2712-2714. http://d.old.wanfangdata.com.cn/Periodical/xtfzxb201011058 SUN S M, ZHENG W, TANG G J.Position error estimation of X-ray pulsar based on CV model[J].Journal of System Simulation, 2010, 22(11):2712-2714(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/xtfzxb201011058
[11]	孙守明.基于X射线脉冲星的航天器自主导航方法研究[D].长沙: 国防科学技术大学, 2011. http://cdmd.cnki.com.cn/Article/CDMD-90002-2007140905.htm SUN S M.Study on autonomous navigation method of spacecraft based on X-ray pulsar[D].Changsha: National University of Defense Technology, 2011(in Chinese). http://cdmd.cnki.com.cn/Article/CDMD-90002-2007140905.htm
[12]	BOBOLTZ D A, FEY A L, JOHNSTON K J, et al.Astrometric positions and proper motions of 19 radio stars[J].Astronomical Journal, 2003, 126(1):484-493. doi: 10.1086/375462
[13]	MANCHESTER R N, HOBBS G B, TEOH A, et al.The australia telescope national facility pulsar catalogue[J].Astronomical Journal, 2005, 129(4):1993-2006. doi: 10.1086/428488
[14]	许强, 王宏力, 何贻洋, 等.基于截断误差的改进脉冲星导航观测方程[J].北京航空航天大学学报, 2018, 44(9):1974-1981. http://d.old.wanfangdata.com.cn/Periodical/bjhkhtdxxb201809021 XU Q, WANG H L, HE Y Y, et al.Improved pulsar navigation measurement equation based on truncation errors[J].Journal of Beijing University of Aeronautics and Astronautics, 2018, 44(9):1974-1981(in Chinese). http://d.old.wanfangdata.com.cn/Periodical/bjhkhtdxxb201809021
[15]	FRIEDLAND B.Treatment of bias in recursive filtering[J].IEEE Transactions on Automatic Control, 1969, AC-14(4):359-367. doi: 10.1109-TAC.1969.1099223/
[16]	HSIEH C S, CHEN F C.General two-stage Kalman filters[J].IEEE Transactions on Automatic Control, 2000, 45(4):819-824. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ026357299/