基于改进加权响应面的结构可靠度计算方法

吴洁; 张建国; 游令非; 叶楠

doi:10.13700/j.bh.1001-5965.2020.0251

基于改进加权响应面的结构可靠度计算方法

doi: 10.13700/j.bh.1001-5965.2020.0251

吴洁^{1, 2},
张建国^{1, 2, ,},
游令非^{1, 2},
叶楠^{1, 2}

1.
北京航空航天大学可靠性与系统工程学院, 北京 100083
2.
北京航空航天大学可靠性与环境工程技术国防科技重点实验室, 北京 100083

基金项目:

国家自然科学基金 51675026

详细信息

通讯作者:
张建国. E-mail: zjg@buaa.edu.cn

中图分类号: TB114.3
计量
- 文章访问数: 616
- HTML全文浏览量: 200
- PDF下载量: 87
- 被引次数: 0
出版历程
- 收稿日期: 2020-06-11
- 录用日期: 2020-08-07
- 网络出版日期: 2021-08-20

Structural reliability calculation method based on improved weighted response surface

WU Jie^{1, 2},
ZHANG Jianguo^{1, 2
, ,},
YOU Lingfei^{1, 2},
YE Nan^{1, 2}

1.
School of Reliability and System Engineering, Beihang University, Beijing 100083, China
2.
Science and Technology on Reliability and Engineering Laboratory, Beihang University, Beijing 100083, China

Funds:

National Natural Science Foundation of China 51675026

More Information

Corresponding author: ZHANG Jianguo. E-mail: zjg@buaa.edu.cn

摘要

摘要:
在结构可靠度分析中，响应面法由于具有良好的适用性和可操作性，是目前广泛使用的基于代理模型的分析方法。针对响应面法的计算效率和精度难以平衡兼顾等难点问题，提出一种基于改进加权响应面的结构可靠度计算方法。首先，在迭代过程中，同时考虑样本点与验算点距离、极限状态函数值、联合概率密度函数值3个权重因子对样本点进行赋权，采用加权回归并重复利用已有样本点更新不含交叉项的二次多项式响应面函数。其次，在迭代收敛后，选取已有样本点中权重较大的样本点加权拟合含有交叉项的二次多项式响应面函数。最后，结合数值算例和工程案例，通过与传统抽样方法和其他响应面法进行对比，验证了改进加权响应面法的可行性。结果表明所提方法具有较高效率的同时也保证了精度。
- 结构可靠度 /
- 响应面法 /
- 响应面函数 /
- 加权回归 /
- 样本重用
Abstract:
Response surface method is a widely used agent model analysis method for structural reliability analysis because of its good applicability and maneuverability. Aimed at the difficulties of the response surface method, such as balancing the efficiency and accuracy, a structural reliability calculation method based on improved weighted response surface is proposed. In the iterative process, three weighting factors, including the distance between the sample point and the design point, the absolute value of limit state function and the value of joint probability density function, are considered to weight the sample points. The quadratic polynomial response surface function without cross term is updated by weighted regression and reusing all known sample points. After the iterative convergence, the sample points with the larger weight among the existing sample points are selected to fit the quadratic polynomial response surface function with cross terms. Finally, with numerical examples and engineering cases, the feasibility of the proposed method is verified by comparing with traditional sampling methods and other response surface methods. The results show that the proposed method has higher efficiency and meanwhile guarantees accuracy.
- structural reliability /
- response surface method /
- response surface function /
- weighted regression /
- sample points reused

HTML全文

图 1 非线性加权响应面法流程^[22]

Figure 1. Flowchart of weighted nonlinear response surface method^[22]

下载: 全尺寸图片幻灯片

图 2 改进加权响应面法流程

Figure 2. Flowchart of improved weighted response surface method

下载: 全尺寸图片幻灯片

图 3 算例1求解过程

Figure 3. Solving process of Example 1

下载: 全尺寸图片幻灯片

图 4 带有集中力的悬臂梁

Figure 4. A cantilever beam with concentrated force

下载: 全尺寸图片幻灯片

图 5 算例2求解过程

Figure 5. Solving process of Example 2

下载: 全尺寸图片幻灯片

图 6 十杆桁架结构

Figure 6. Ten-bar truss structure

下载: 全尺寸图片幻灯片

图 7 十杆桁架ANSYS仿真结果

Figure 7. ANSYS simulation results of ten-bar truss

下载: 全尺寸图片幻灯片

图 8 算例3求解过程

Figure 8. Solving process of Example 3

下载: 全尺寸图片幻灯片

表 1 算例1计算结果比较

Table 1. Comparison of calculation results of Example 1

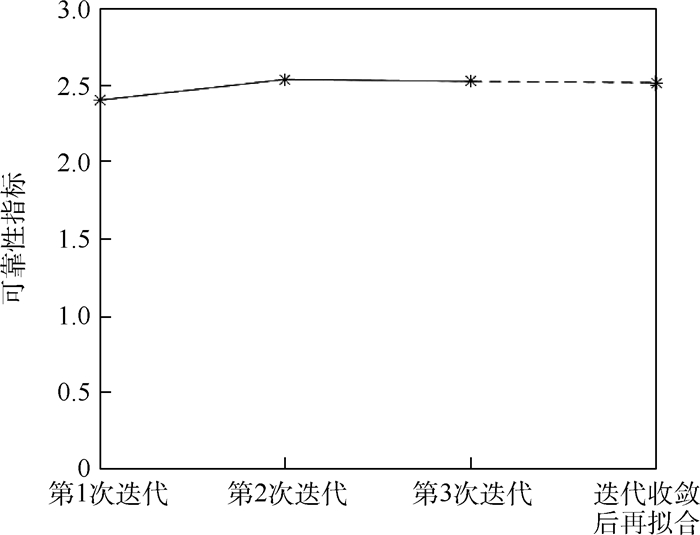
方法	失效概率	误差/%	结构分析次数	计算时间/s
蒙特卡罗法	0.003 622	0	10 000 000
经典响应面法	0.003 369	6.985 09	30	0.061
线性加权响应面法	0.003 362	7.178 36	20	0.023
单加权响应面法	0.003 366	7.067 92	20	0.026
双加权响应面法	0.003 393	6.322 37	20	0.034
改进加权响应面法	0.003 689	1.849 81	15	0.048

下载: 导出CSV

表 2 算例2计算结果比较

Table 2. Comparison of calculation results of Example 2

方法	失效概率	误差/%	结构分析次数	计算时间/s
蒙特卡罗法	0.005 959	0	10 000 000
经典响应面法	0.005 691	4.497 40	56	0.026
线性加权响应面法	0.005 691	4.497 40	28	0.021
单加权响应面法	0.005 689	4.530 96	49	0.031
双加权响应面法	0.005 861	1.644 57	21	0.030
改进加权响应面法	0.005 967	0.134 25	21	0.052

下载: 导出CSV

表 3 算例3计算结果比较

Table 3. Comparison of calculation results of Example 3

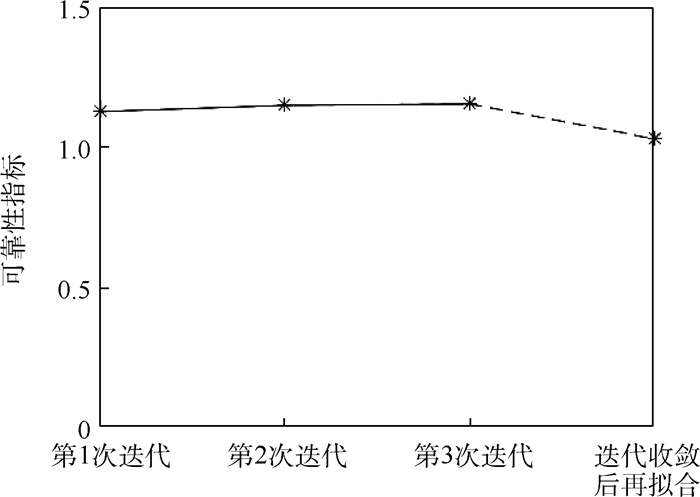
方法	失效概率	误差/%	结构分析次数
蒙特卡罗法^[23]	0.844 20	0	100 000
经典响应面法	0.836 41	0.922 77	196
线性加权响应面法	0.879 58	4.199 10	21
单加权响应面法	0.838 17	0.714 29	28
双加权响应面法	0.836 32	0.933 43	49
改进加权响应面法	0.848 89	0.555 56	21

下载: 导出CSV

参考文献(23)

[1]	徐铭阳, 刘林江. 可靠度理论及一次二阶矩法概述[J]. 山西建筑, 2016, 42(8): 67-68. doi: 10.3969/j.issn.1009-6825.2016.08.035 XU M Y, LIU L J. Illustration on reliability theory and first-order second-moment method[J]. Shanxi Architecture, 2016, 42(8): 67-68(in Chinese). doi: 10.3969/j.issn.1009-6825.2016.08.035
[2]	BREITUNG K. Asymptotic approximations for multinormal integrals[J]. Journal of Engineering Mechanics, 1984, 110(3): 357-366. doi: 10.1061/(ASCE)0733-9399(1984)110:3(357)
[3]	张明. 结构可靠度分析——方法与程序[M]. 北京: 科学出版社, 2009: 68-77. ZHANG M. Structural reliability analysis: Methods and procedures[M]. Beijing: Science Press, 2009: 68-77(in Chinese).
[4]	吕震宙, 宋淑芳, 李洪双, 等. 结构机构可靠性及可靠性灵敏度分析[M]. 北京: 科学出版社, 2009: 98-104. LV Z Z, SONG S F, LI H S, et al. Reliability and reliability sensitivity analysis of structural mechanism[M]. Beijing: Science Press, 2009: 98-104(in Chinese).
[5]	ROBERT C P, CASELLA G. Monte Carlo statistical methods[M]. 2nd ed. Berlin: Springer, 2004: 79-122.
[6]	赵国藩. 工程结构可靠性理论与应用[M]. 大连: 大连理工大学出版社, 1996: 145-158. ZHAO G F. Reliability theory and its applications for engineering structures[M]. Dalian: Dalian University of Technology Press, 1996: 145-158(in Chinese).
[7]	BUCHER C G, BOURGUND U. A Fast and efficient response surface approach for structural reliability problems[J]. Structural Safety, 1990, 7(1): 57-66. doi: 10.1016/0167-4730(90)90012-E
[8]	RAJASHEKHAR M R, ELLINGWOOD B R. A new look at the response surface approach for reliability analysis[J]. Structural Safety, 1993, 12(3): 205-220. doi: 10.1016/0167-4730(93)90003-J
[9]	KAYMAZ I, MCMAHON C A. A response surface method based on weighted regression for structural reliability analysis[J]. Probabilistic Engineering Mechanics, 2005, 20(1): 11-17. doi: 10.1016/j.probengmech.2004.05.005
[10]	赵洁, 吕震宙. 隐式极限状态方程可靠性分析的加权响应面法[J]. 机械强度, 2006, 28(4): 512-516. doi: 10.3321/j.issn:1001-9669.2006.04.009 ZHAO J, LV Z Z. Response surface method for reliability analysis of implicit limit state equation based on weighted regression[J]. Journal of Mechanical Strength, 2006, 28(4): 512-516(in Chinese). doi: 10.3321/j.issn:1001-9669.2006.04.009
[11]	NGUYEN X A, SELLIER A, DUPRAT F, et al. Adaptive response surface method based on a double weighted regression technique[J]. Probabilistic Engineering Mechanics, 2009, 24(2): 135-143. doi: 10.1016/j.probengmech.2008.04.001
[12]	李贵杰, 吕震宙, 赵新攀. 基于加权线性响应面的结构可靠性估计的鞍点逼近方法[J]. 机械强度, 2010, 32(6): 917-921. https://www.cnki.com.cn/Article/CJFDTOTAL-JXQD201006009.htm LI G J, LV Z Z, ZHAO X P. Saddlepoint approximation of structural reliability on weighted linear response surface method[J]. Journal of Mechanical Strength, 2010, 32(6): 917-921(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JXQD201006009.htm
[13]	钟宏林, 吴剑国, 王恒军. 可靠性分析的双加权响应面法[J]. 浙江工业大学学报, 2010, 38(2): 218-221. doi: 10.3969/j.issn.1006-4303.2010.02.021 ZHONG H L, WU J G, WANG H J. Reliability analysis of response surface method based on a double weighted regression technique[J]. Journal of Zhejiang University of Technology, 2010, 38(2): 218-221(in Chinese). doi: 10.3969/j.issn.1006-4303.2010.02.021
[14]	李广博. Fourier正交基神经网络加权响应面法的结构可靠性分析[D]. 长春: 吉林大学, 2014: 37-41. LI G B. Structural reliability analysis based on Fourier orthogonal neural network weighted response surface method[D]. Changchun: Jilin University, 2014: 37-41(in Chinese).
[15]	张吉宁, 郭书祥, 唐承, 等. 基于向量投影取样的改进加权响应面法[J]. 科学通报, 2017, 62(17): 1854-1860. https://www.cnki.com.cn/Article/CJFDTOTAL-KXTB201717009.htm ZHANG J N, GUO S X, TANG C, et al. An improved weighted response surface method based on vector projection sampling[J]. Chinese Science Bulletin, 2017, 62(17): 1854-1860(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-KXTB201717009.htm
[16]	张学刚. 一种改进响应面法结构可靠度计算方法[J]. 机械强度, 2018, 40(6): 1382-1388. https://www.cnki.com.cn/Article/CJFDTOTAL-JXQD201806044.htm ZHANG X G. Structural reliability analysis based on an improvement of the response surface method[J]. Journal of Mechanical Strength, 2018, 40(6): 1382-1388(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JXQD201806044.htm
[17]	贾长安. 基于随机变量相关和失效模式相关的结构可靠性算法研究[D]. 西安: 西安电子科技大学, 2018: 34-41. JIA C A. The study on structural reliability algorithm based on random variable correlation and failure mode correlation[D]. Xi'an: Xidian University, 2018: 34-41(in Chinese).
[18]	侯振兴. 基于响应面法的结构可靠度计算方法研究[D]. 秦皇岛: 燕山大学, 2019: 20-23. HOU Z X. Research on structural reliability calculation method based on response surface method[D]. Qinhuangdao: Yanshan University, 2019: 20-23(in Chinese).
[19]	LU C, FENG Y W, FEI C W. Weighted regression-based extremum response surface method for structural dynamic fuzzy reliability analysis[J]. Energies, 2019, 12(9): 1-16. http://www.researchgate.net/publication/332685745_Weighted_Regression-Based_Extremum_Response_Surface_Method_for_Structural_Dynamic_Fuzzy_Reliability_Analysis
[20]	洪林雄, 李华聪, 彭凯, 等. 基于高效搜索方法的可靠性分析改进响应面法[J]. 北京航空航天大学学报, 2020, 46(1): 95-102. doi: 10.13700/j.bh.1001-5965.2019.0169 HONG L X, LI H C, PENG K, et al. Improved response surface method of reliability analysis based on efficient search method[J]. Journal of Beijing University of Aeronautics and Astronautics, 2020, 46(1): 95-102(in Chinese). doi: 10.13700/j.bh.1001-5965.2019.0169
[21]	冯欢欢, 蒋向华. 样本重复使用失效响应曲线分析结构可靠度方法[J]. 航空动力学报, 2013, 28(10): 2228-2234. https://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201310011.htm FENG H H, JIANG X H. Sample points reused failure response curve method for structural reliability analysis[J]. Journal of Aerospace Power, 2013, 28(10): 2228-2234(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HKDI201310011.htm
[22]	赵洁. 机械可靠性分析的响应面法研究[D]. 西安: 西北工业大学, 2006: 23-27. ZHAO J. Research on response surface method of mechanical reliability analysis[D]. Xi'an: Northwestern Polytechnical University, 2006: 23-27(in Chinese).
[23]	CHOI S K, CANFIELD R A, GRANDHI R. Reliability-based structural design[M]. Berlin: Springer, 2007: 138-141.