厚胶层复合材料黏接结构中超声反射/透射特性的有限元仿真

何存富; 李永坤; 吕炎; 宋国荣

doi:10.13700/j.bh.1001-5965.2020.0305

厚胶层复合材料黏接结构中超声反射/透射特性的有限元仿真

doi: 10.13700/j.bh.1001-5965.2020.0305

北京工业大学材料与制造学部, 北京 100124

基金项目:

国家自然科学基金 11972052

国家自然科学基金 51875010

北京市教委科技一般项目 KM201710005012

详细信息

通讯作者:
吕炎, E-mail: lvyan@bjut.edu.cn

中图分类号: TB559
计量
- 文章访问数: 627
- HTML全文浏览量: 75
- PDF下载量: 182
- 被引次数: 0
出版历程
- 收稿日期: 2020-07-01
- 录用日期: 2020-08-28
- 网络出版日期: 2021-09-20

Finite element simulation of ultrasonic reflection/transmission characteristics for composite bonded joints with thick adhesive layers

Faculty of Materials and Manufacturing, Beijing University of Technology, Beijing 100124, China

Funds:

National Natural Science Foundation of China 11972052

National Natural Science Foundation of China 51875010

General Program of Science and Technology Development Project of Beijing Municipal Education Commission KM201710005012

More Information

Corresponding author: LYU Yan, E-mail: lvyan@bjut.edu.cn

摘要

摘要:
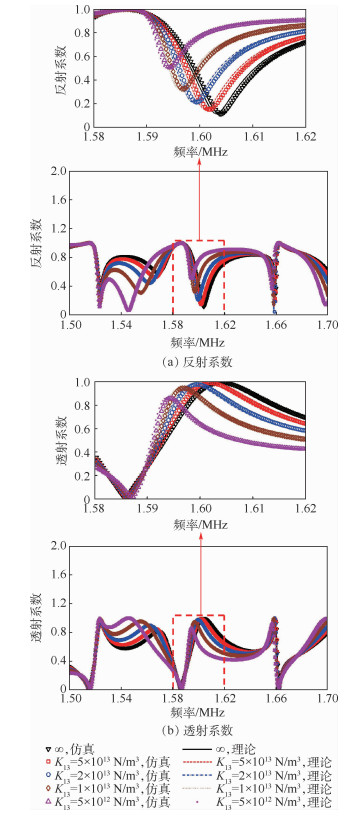
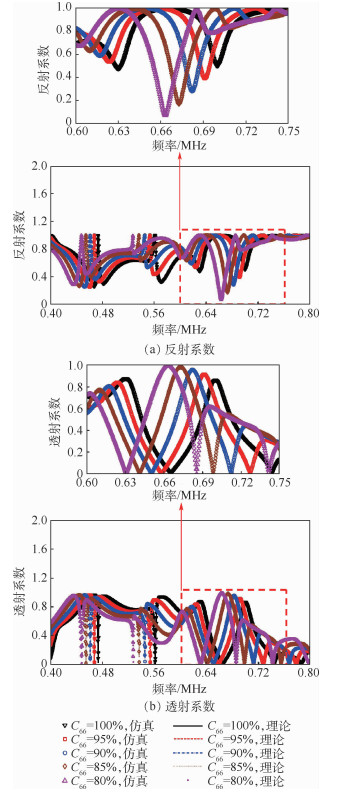
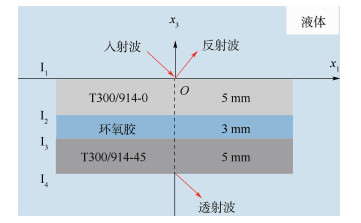
针对厚胶层复合材料黏接结构，采用有限元法对其中发生黏接界面弱化、胶层内聚弱化时的超声反射/透射系数进行了仿真计算。在仿真模型中，利用弹性薄层物理场边界模拟了常规理论推导中的弹簧模型边界条件，通过改变胶层材料的弹性常数实现了对胶层内聚状态变化的模拟。仿真计算结果表明：随着黏接界面弱化程度的加剧，超声反射/透射系数频谱曲线将向低频方向偏移，而超声反射/透射系数角谱曲线将向大角度方向偏移；随着黏接结构中胶层内聚弱化程度的加剧，对应超声反射/透射系数频谱、角谱曲线的偏移趋势与发生黏接界面弱化时的情况一致。研究成果解决了利用有限元法对厚胶层复合材料黏接结构中黏接界面、结构胶内聚层进行模拟处理的问题，且仿真结果和理论计算结果吻合良好。
- 黏接结构 /
- 复合材料 /
- 厚胶层 /
- 超声反射/透射 /
- 有限元
Abstract:
For composite bonded joints with thick adhesive layers, finite element method is applied to calculate the ultrasonic reflection/transmission coefficients when bonding interfaces and cohesion of adhesive layers are degraded. In the simulation model, the boundary conditions of the spring model used in conventional theoretical derivations are modeled by using the physical field boundary namely elastic thin layer, and the cohesive state of the adhesive layer is simulated by changing the elastic constants of it. The simulation results show that the frequency spectrum curve of reflection/transmission coefficients will shift to the low frequency side and the angular spectrum curve of reflection/transmission coefficients will shift to the large angle side with the increase of degradation degree of bonding interfaces. In addition, when the degradation of the cohesion of adhesive layers is getting worse, the shift tendency of the corresponding frequency and angular spectrum curve of reflection/transmission coefficients is consistent with that when degradation on bonding interfaces occurs. The problem of simulating bonding interfaces and structural adhesive's cohesion layers in composite bonded joints with thick adhesive layers by finite element method is solved, and the simulation results are in good agreement with theoretically calculated results.
- bonded joint /
- composite /
- thick adhesive layer /
- ultrasonic reflection/transmission /
- finite element

HTML全文

图 1 厚胶层复合材料黏接结构及声波传播示意图

Figure 1. Schematic of composite bonded joint with thick adhesive layer and acoustic wave travelling

下载: 全尺寸图片幻灯片

图 2 厚胶层复合材料黏接结构的仿真模型

Figure 2. Simulation model of composite bonded joint with thick adhesive layer

下载: 全尺寸图片幻灯片

图 3 厚胶层黏接界面分析模型

Figure 3. Analytical model of bonding interfaces in thick adhesive layer

下载: 全尺寸图片幻灯片

图 4 黏接界面弱化时的仿真与理论计算结果(频谱)

Figure 4. Finite element modeling and theoretical calculation results with degradation on bonding interface (frequency spectrum)

下载: 全尺寸图片幻灯片

图 5 黏接界面弱化时的仿真与理论计算结果(角谱)

Figure 5. Finite element modeling and theoretical calculation results with degradation on bonding interface (incident angle spectrum)

下载: 全尺寸图片幻灯片

图 6 胶层内聚弱化时的仿真与理论计算结果(频谱)

Figure 6. Finite element modeling and theoretical calculation results with degradation of cohesion in adhesive layer (frequency spectrum)

下载: 全尺寸图片幻灯片

图 7 胶层内聚弱化时的仿真与理论计算结果(角谱)

Figure 7. Finite element modeling and theoretical calculation results with degradation of cohesion in adhesive layer (incident angle spectrum)

下载: 全尺寸图片幻灯片

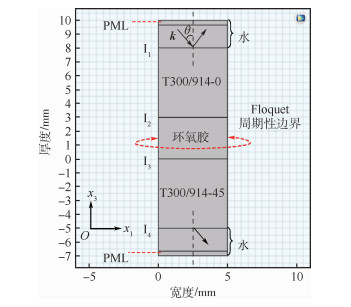
表 1 T300/914-0与环氧胶的材料参数

Table 1. Material properties of T300/914-0 and epoxy adhesive

材料	密度/(kg·m^-3)	C₁₁/GPa	C₁₂/GPa	C₂₃/GPa	C₄₄/GPa	C₂₂/GPa	C₅₅/GPa
T300/914-0	1 560	143.8	6.2	6.5	3.6	13.3	5.7
环氧胶	1 170	7.97	5.14	5.14	1.42	7.97	1.42

下载: 导出CSV

参考文献(18)

[1]	罗书舟, 陈超, 伍乾坤, 等. 复合材料单搭接胶接接头低速冲击数值模拟[J]. 振动与冲击, 2019, 38(1): 142-148. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201901022.htm LUO S Z, CHEN C, WU Q K, et al. Numerical simulation for low velocity impact performances of composite laminates single-lap adhesively bonded joints[J]. Journal of Vibration and Shock, 2019, 38(1): 142-148(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ201901022.htm
[2]	BARNES R H, MOROZOV E V. Structural optimization of composite wind turbine blade structures with variations of internal geometry configuration[J]. Composite Structures, 2016, 152: 158-167. doi: 10.1016/j.compstruct.2016.05.013
[3]	王小民, 李明轩, 毛捷, 等. 单层与衬底胶接结构超声反射波谱的低频特征[J]. 声学学报, 2005, 30(4): 337-342. doi: 10.3321/j.issn:0371-0025.2005.04.008 WANG X M, LI M X, MAO J, et al. Low-frequency features of the ultrasound echo from an adhesively bonded layer-substrate structure[J]. Acta Acustica, 2005, 30(4): 337-342(in Chinese). doi: 10.3321/j.issn:0371-0025.2005.04.008
[4]	梁祖典, 燕瑛, 张涛涛, 等. 复合材料单搭接胶接接头试验研究与数值模拟[J]. 北京航空航天大学学报, 2014, 40(12): 1786-1792. doi: 10.13700/j.bh.1001-5965.2014.0010 LIANG Z D, YAN Y, ZHANG T T, et al. Experimental investigation and numerical simulation of composite laminate adhesively bonded single-lap joints[J]. Journal of Beijing University of Aeronautics and Astronautics, 2014, 40(12): 1786-1792(in Chinese). doi: 10.13700/j.bh.1001-5965.2014.0010
[5]	许昶, 刘志明. CFRP平-折-平连接接头试验研究与数值模拟[J]. 北京航空航天大学学报, 2019, 45(11): 2207-2216. doi: 10.13700/j.bh.1001-5965.2019.0062 XU C, LIU Z M. Experimental study and numerical simulation on CFRP flat-joggle-flat joints[J]. Journal of Beijing University of Aeronautics and Astronautics, 2019, 45(11): 2207-2216(in Chinese). doi: 10.13700/j.bh.1001-5965.2019.0062
[6]	VIJAYA KUMAR R L, BHAT M R, MURTHY C R. Some studies on evaluation of degradation in composite adhesive joints using ultrasonic techniques[J]. Ultrasonics, 2013, 53(6): 1150-1162. doi: 10.1016/j.ultras.2013.01.014
[7]	LEIDERMAN R, JUNQUEIRA B F, CASTELLO D A, et al. Identifying the ultrasonic inspecting fields that most strongly interact with adhesive bonding defects[J]. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 2018, 40(4): 1-13. doi: 10.1007/s40430-018-1151-3
[8]	BAIK J M, THOMPSON R B. Ultrasonic scattering from imperfect interfaces: A quasi-static model[J]. Journal of Nondestructive Evaluation, 1984, 4(3-4): 177-196. doi: 10.1007/BF00566223
[9]	ROKHLIN S I, WANG Y J. Analysis of boundary conditions for elastic wave interaction with an interface between two solids[J]. Journal of the Acoustical Society of America, 1991, 89(2): 503-515. doi: 10.1121/1.400374
[10]	ROKHLIN S I, HUANG W. Ultrasonic wave interaction with a thin anisotropic layer between two anisotropic solids: Exact and asymptotic-boundary-condition methods[J]. Journal of the Acoustical Society of America, 1992, 92(3): 1729-1742. doi: 10.1121/1.403912
[11]	SIRYABE E, RÉNIER M, MEZIANE A, et al. Apparent anisotropy of adhesive bonds with weak adhesion and non-destructive evaluation of interfacial properties[J]. Ultrasonics, 2017, 79: 34-51. doi: 10.1016/j.ultras.2017.02.020
[12]	MORI N, MATSUDA N, KUSAKA T. Effect of interfacial adhesion on the ultrasonic interaction with adhesive joints: A theoretical study using spring-type interfaces[J]. Journal of the Acoustical Society of America, 2019, 145(6): 3541. doi: 10.1121/1.5111856
[13]	WU C Y, WANG H, ZHAO J N, et al. Prediction of viscoelastic pavement responses under moving load and nonuniform tire contact stresses using 2.5-D finite element method[J]. Mathematical Problems in Engineering, 2020, 2020: 1-16. http://www.researchgate.net/publication/338683704_Prediction_of_Viscoelastic_Pavement_Responses_under_Moving_Load_and_Nonuniform_Tire_Contact_Stresses_Using_25-D_Finite_Element_Method
[14]	NAYFEH A H, CHIMENTI D E. Ultrasonic wave reflection from liquid-coupled orthotropic plates with application to fibrous composites[J]. Journal of Applied Mechanics, 1988, 55(4): 863-870. doi: 10.1115/1.3173734
[15]	宋国荣, 刘明坤, 吕炎, 等. 正交各向异性板液/固界面的声反射与声透射[J]. 声学学报, 2020, 45(1): 77-86. https://www.cnki.com.cn/Article/CJFDTOTAL-XIBA202001009.htm SONG G R, LIU M K, LU Y, et al. Acoustic reflection and transmission at the interface between orthotropic plate and liquid media[J]. Acta Acustica, 2020, 45(1): 77-86(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XIBA202001009.htm
[16]	GAUTHIER C, GALY J, ECH-CHERIF-KETTANI M, et al. Evaluation of epoxy crosslinking using ultrasonic Lamb waves[J]. International Journal of Adhesion and Adhesives, 2018, 80: 1-6. doi: 10.1016/j.ijadhadh.2017.09.008
[17]	BROTHERHOOD C J, DRINKWATER B W, DIXON S. The detectability of kissing bonds in adhesive joints using ultrasonic techniques[J]. Ultrasonics, 2003, 41(7): 521-529. doi: 10.1016/S0041-624X(03)00156-2
[18]	STEWART I, CHAMBERS A, GORDON T. The cohesive mechanical properties of a toughened epoxy adhesive as a function of cure level[J]. International Journal of Adhesion and Adhesives, 2007, 27(4): 277-287. http://www.onacademic.com/detail/journal_1000034018854010_0611.html