一种自适应GNSS弱信号载噪比估计方法

孙文杰; 王兆瑞; 金声震; 艾国祥

doi:10.13700/j.bh.1001-5965.2020.0372

一种自适应GNSS弱信号载噪比估计方法

doi: 10.13700/j.bh.1001-5965.2020.0372

孙文杰^{1, 2},
王兆瑞^1, ,,
金声震¹,
艾国祥¹

1.
中国科学院国家天文台, 北京 100101
2.
中国科学院大学天文与空间科学学院, 北京 100049

基金项目:

国家自然科学基金 U1931125

国家自然科学基金 11603041

国家重点研发计划 2016YFB0501900

详细信息

通讯作者:
王兆瑞, E-mail: zhaorui_w@nao.cas.cn

中图分类号: V221⁺.3;TB553
计量
- 文章访问数: 1001
- HTML全文浏览量: 101
- PDF下载量: 119
- 被引次数: 0
出版历程
- 收稿日期: 2020-07-30
- 录用日期: 2020-12-11
- 网络出版日期: 2021-10-20

An adaptive carrier-to-noise ratio estimation method for GNSS weak signal

1.
National Astronomical Observatories, Chinese Academy of Sciences, Beijing 100101, China
2.
School of Astronomy and Space Science, University of Chinese Academy of Sciences, Beijing 100049, China

Funds:

National Natural Science Foundation of China U1931125

National Natural Science Foundation of China 11603041

National Key R & D Program of China 2016YFB0501900

More Information

Corresponding author: WANG Zhaorui, E-mail: zhaorui_w@nao.cas.cn

摘要

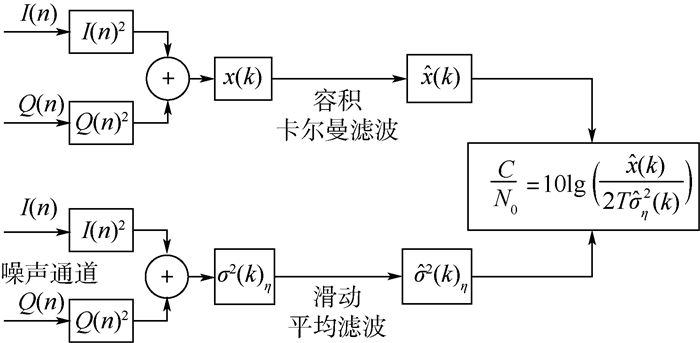
摘要:
全球导航卫星系统（GNSS）信号的载噪比（CNR）是衡量接收机工作性能的一个重要参数。为了准确得到载噪比估计值，推导并分析了2种常用的GNSS信号载噪比估计方法（方差求和法（VSM）、窄带宽带功率比值法（PRM）），并同时提出一种基于渐消因子容积卡尔曼滤波的自适应载噪比估计方法，比较了3种方法在通常的信号环境下和弱信号环境下的载噪比估计能力。结果显示：在信号较弱环境或信号受到遮挡产生突变等情况时，VSM方法与PRM方法均会产生较大的误差，而自适应载噪比估计方法能准确估计出信号的载噪比。
- 全球导航卫星系统(GNSS)信号 /
- GNSS接收机 /
- 载噪比(CNR)估计 /
- 容积卡尔曼滤波 /
- 渐消因子
Abstract:
The Carrier-to-Noise Ratio (CNR) of Global Navigation Satellite System (GNSS) signals is an important parameter to describe GNSS receiver's performance. In this paper, we derive and analyze two commonly used GNSS signal CNR estimation methods: Variance Summing Method (VSM) and Power Ratio Method (PRM). Meanwhile, we propose an adaptive CNR estimation method which is based on fading factor cubature Kalman filter. We compare the three methods to assess the CNR estimation ability in normal and weak signal environment. The results show that, when signal suddenly changes or signal is weak, the VSM and PRM will produce large estimation errors, while the adaptive CNR estimation methods can still accurately estimate the CNR of signal.
- Global Navigation Satellite System (GNSS) signal /
- GNSS receiver /
- Carrier-to-Noise Ratio (CNR) estimation /
- cubature Kalman filter /
- fade factor

HTML全文

图 1 自适应载噪比估计方法流程

Figure 1. Adaptive CNR estimation method's flowchart

下载: 全尺寸图片幻灯片

图 2 三种方法的估计误差

Figure 2. Three methods' estimation errors

下载: 全尺寸图片幻灯片

图 3 三种估计方法在载噪比突变环境中的估计误差

Figure 3. Three estimation methods' estimation errors in the environment of abrupt CNR change

下载: 全尺寸图片幻灯片

表 1 三种算法在不同载噪比环境下的估计误差

Table 1. Estimation errors of three method in different C/N₀ environments

载噪比/ (dB·Hz)	载噪比估计误差/(dB·Hz)
载噪比/ (dB·Hz)	VSM方法	PRM方法	自适应载噪比估计方法
45	0.54	0.55	0.40
35	0.77	1.03	0.47
25	0.81	1.66	0.52

下载: 导出CSV

参考文献(15)

[1]	鲁郁. 北斗/GPS双模软件接收机原理与实现技术[M]. 北京: 电子工业出版社, 2016: 197-203. LU Y. Principle and implementation technology of Beidou/GPS dual-mode software receiver[M]. Beijing: Publishing House of Electronics Industry, 2016: 197-203(in Chinese).
[2]	谢钢. GPS原理与接收机设计[M]. 北京: 电子工业出版社, 2017: 362-364. XIE G. Principles of GPS and receiver design[M]. Beijing: Publishing House of Electronics Industry, 2017: 362-364(in Chinese).
[3]	蒋君伟, 段晓辉, 林阳. 一种GPS信号载噪比估计的新算法[J]. 系统仿真学报, 2009, 21(24): 7786-7788. https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ200924022.htm JIANG J W, DUAN X H, LIN Y. New algorithm for GPS C/N₀ estimation[J]. Journal of System Simulation, 2009, 21(24): 7786-7788(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-XTFZ200924022.htm
[4]	GROVES P D. GPS signal-to-noise measurement in weak signal and high-interference environments[J]. Navigation, 2005, 52(2): 83-94. doi: 10.1002/j.2161-4296.2005.tb01734.x
[5]	SHARAWI M S, AKOS D M, ALOI D N. GPS C/N₀/estimation in the presence of interference and limited quantization levels[J]. IEEE Transactions on Aerospace and Electronic Systems, 2007, 43(1): 227-238. doi: 10.1109/TAES.2007.357129
[6]	FALLETTI E, PINI M, PRESTI L L, et al. Assessment on low complexity C/N₀ estimators based on M-PSK signal model for GNSS receivers[C]//2008 IEEE/ION Position, Location and Navigation Symposium. Piscataway: IEEE Press, 2008: 167-172.
[7]	LASHLEY M. Modeling and performance analysis of GPS vector tracking algorithms[M]. Auburn: Auburn University, 2009: 318-324.
[8]	巴晓辉, 刘海洋, 郑睿, 等. 一种有效的GNSS接收机载噪比估计方法[J]. 武汉大学学报(信息科学版), 2011, 36(4): 457-460. https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201104019.htm BA X H, LIU H Y, ZHENG R, et al. An effective carrier-to-noise ratio estimation method for GNSS receivers[J]. Geomatics and Information Science of Wuhan University, 2011, 36(4): 457-460(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-WHCH201104019.htm
[9]	徐昌元, 唐小妹, 倪少杰, 等. 电离层闪烁背景下不同载噪比估计算法的性能分析[J]. 全球定位系统, 2016, 41(5): 23-27. https://www.cnki.com.cn/Article/CJFDTOTAL-QUDW201605005.htm XU C Y, TANG X M, NI S J, et al. Ionospheric anomalies preceding kumamoto earthquake detected by GNSS[J]. GNSS World of China, 2016, 41(5): 23-27(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-QUDW201605005.htm
[10]	王姣, 姜苏洋, 狄世超, 等. GNSS系统中一种低复杂度的载噪比估计算法[J]. 哈尔滨工程大学学报, 2018, 39(6): 1087-1092. https://www.cnki.com.cn/Article/CJFDTOTAL-HEBG201806018.htm WANG J, JIANG S Y, DI S C, et al. A low complexity carrier-to-noise ratio estimation algorithm in GNSS systems[J]. Journal of Harbin Engineering University, 2018, 39(6): 1087-1092(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-HEBG201806018.htm
[11]	何文涛, 徐建华, 叶甜春. GPS弱信号的自适应载噪比估计算法[J]. 电子技术应用, 2010, 36(6): 111-114. https://www.cnki.com.cn/Article/CJFDTOTAL-DZJY201006049.htm HE W T, XU J H, YE T C. Adaptive C/N estimation method of GPS weak signal[J]. Application of Electronic Technology, 2010, 36(6): 111-114(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-DZJY201006049.htm
[12]	ARASARATNAM I, HAYKIN S. Cubature Kalman filters[J]. IEEE Transactions on Automatic Control, 2009, 54(6): 1254-1269. doi: 10.1109%2FTAC.2009.2019800
[13]	周东华, 席裕庚, 张钟俊. 非线性系统带次优渐消因子的扩展卡尔曼滤波[J]. 控制与决策, 1990, 5(5): 1-6. https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC199005000.htm ZHOU D H, XI Y G, ZHANG Z J. Extended Kalman filter with suboptimal fading factors for nonlinear systems[J]. Control and Decision, 1990, 5(5): 1-6(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC199005000.htm
[14]	鲍水达, 张安, 高飞. 一种新的多渐消因子容积卡尔曼滤波[J]. 计算机测量与控制, 2019, 27(2): 241-245. https://www.cnki.com.cn/Article/CJFDTOTAL-JZCK201902053.htm BAO S D, ZHANG A, GAO F. A new multi-decay factor volume Kalman filter[J]. Computer Measurement and Control, 2019, 27(2): 241-245(in Chinese). https://www.cnki.com.cn/Article/CJFDTOTAL-JZCK201902053.htm
[15]	朱珍珍. 卫星导航矢量跟踪关键技术研究[D]. 长沙: 国防科学技术大学, 2011: 84-88. ZHU Z Z. Research on key techniques of vector tracking for satellite navigation[D]. Changsha: National University of Defense Technology, 2011: 84-88(in Chinese).