基于飞行冲突网络最优支配集的冲突调配策略

吴明功; 毕可心; 温祥西; 孙继昆

doi:10.13700/j.bh.1001-5965.2021.0233

基于飞行冲突网络最优支配集的冲突调配策略

doi: 10.13700/j.bh.1001-5965.2021.0233

吴明功^{1, 2},
毕可心^{1, 2},
温祥西^{1, 2, ,},
孙继昆³

1.
空军工程大学空管领航学院，西安 710051
2.
国家空管防相撞技术重点实验室，西安 710051
3.
中国人民解放军93735部队，天津 301799

基金项目: 国家自然科学基金(71801221)；国家社会科学基金(19BGL297)

详细信息

作者简介:
毕可心,等：基于飞行冲突网络最优支配集的冲突调配策略 11

通讯作者:
E-mail：wxxajy@163.com

中图分类号: V355；O231.5
计量
- 文章访问数: 586
- HTML全文浏览量: 106
- PDF下载量: 81
- 被引次数: 0
出版历程
- 收稿日期: 2021-05-07
- 录用日期: 2021-06-11
- 网络出版日期: 2021-07-14
- 整期出版日期: 2023-02-28

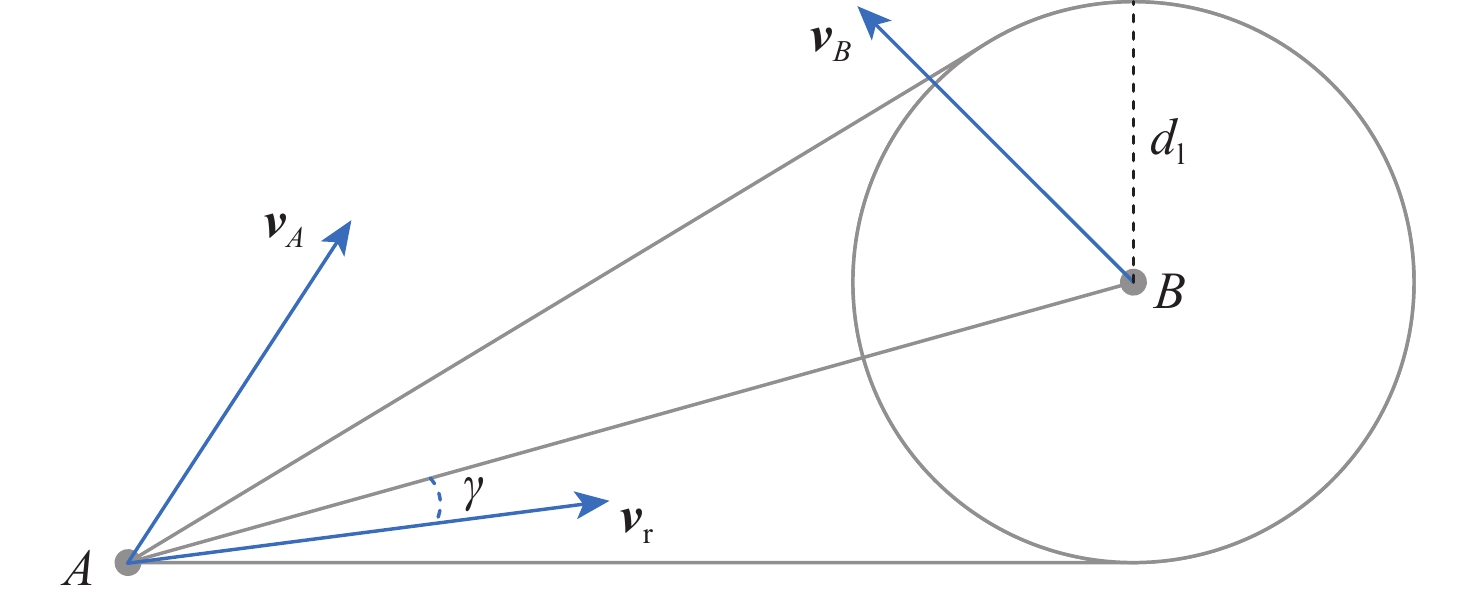
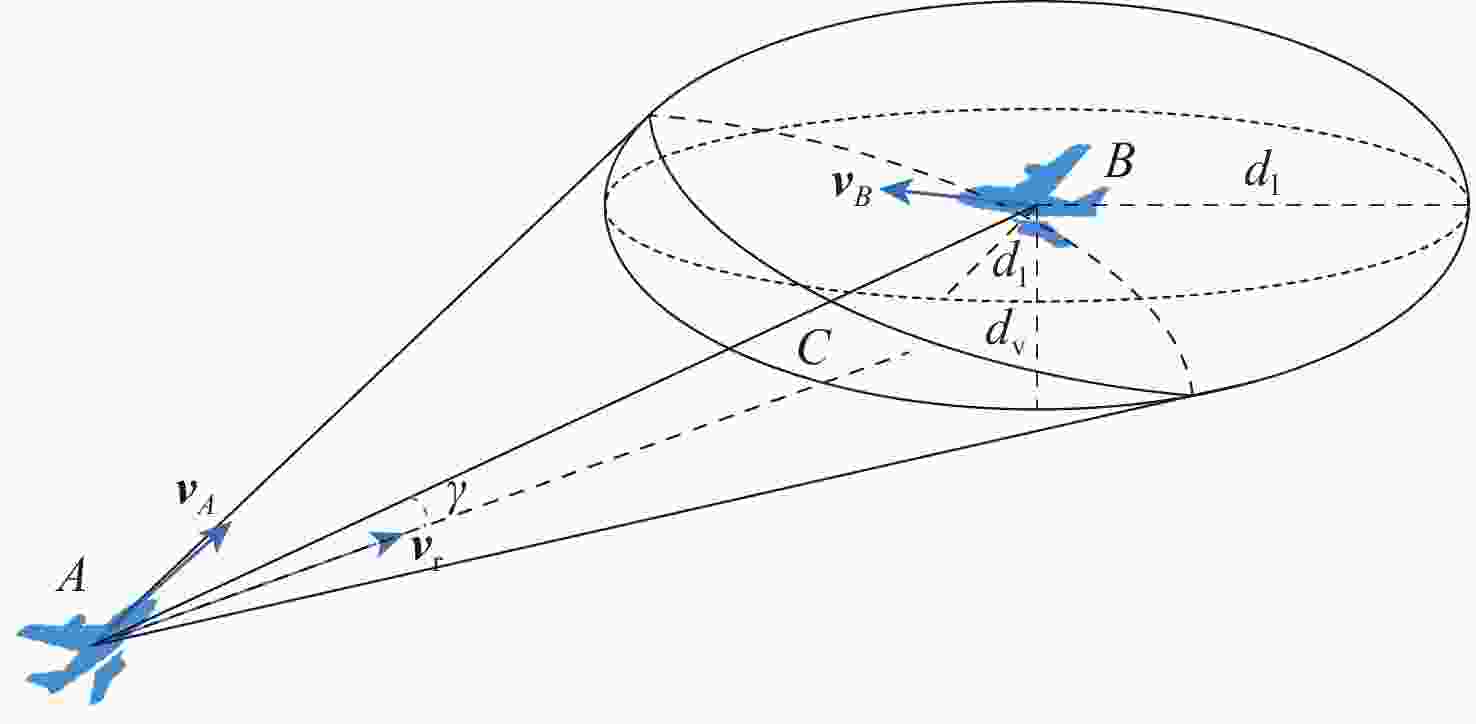
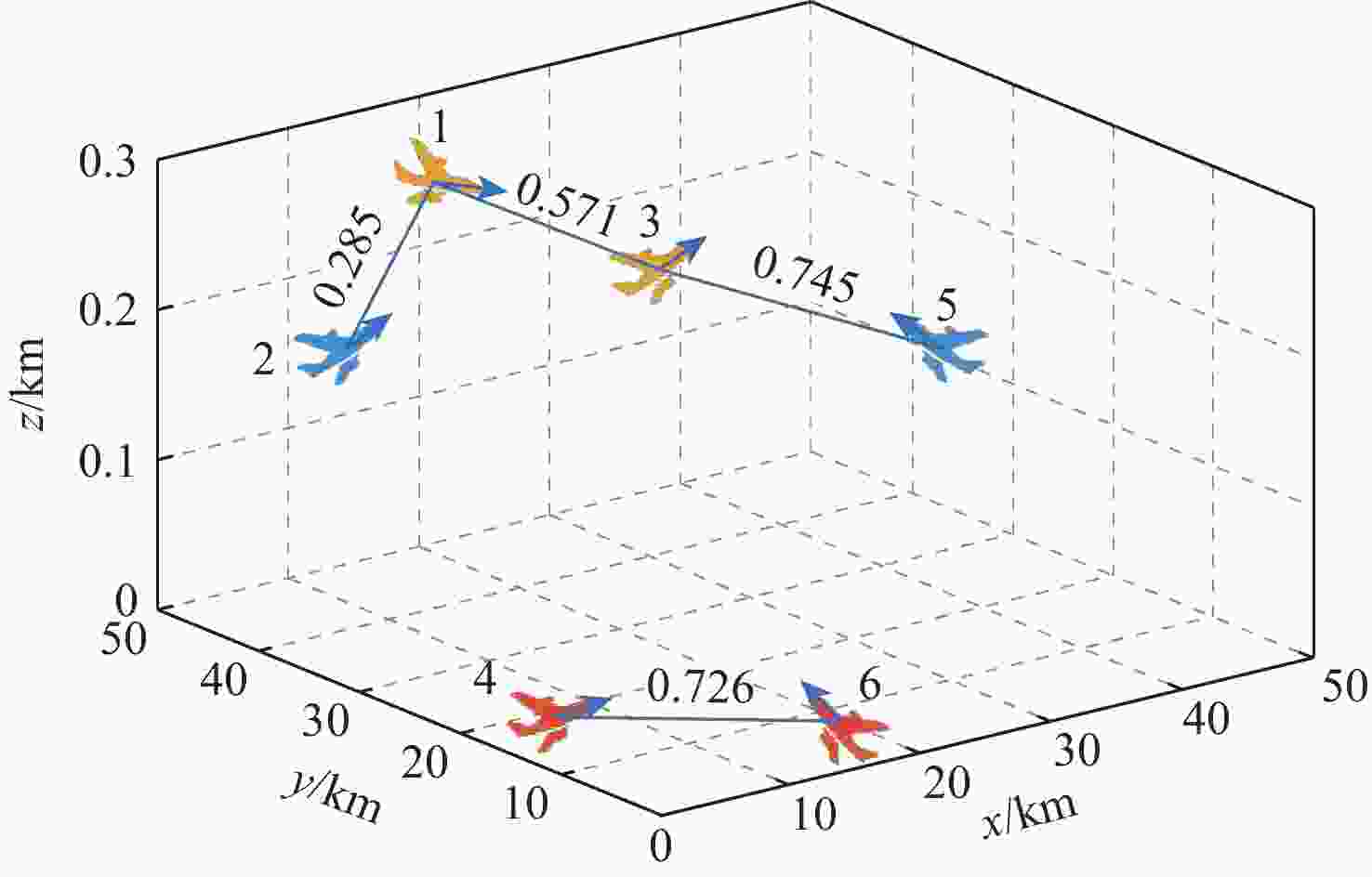
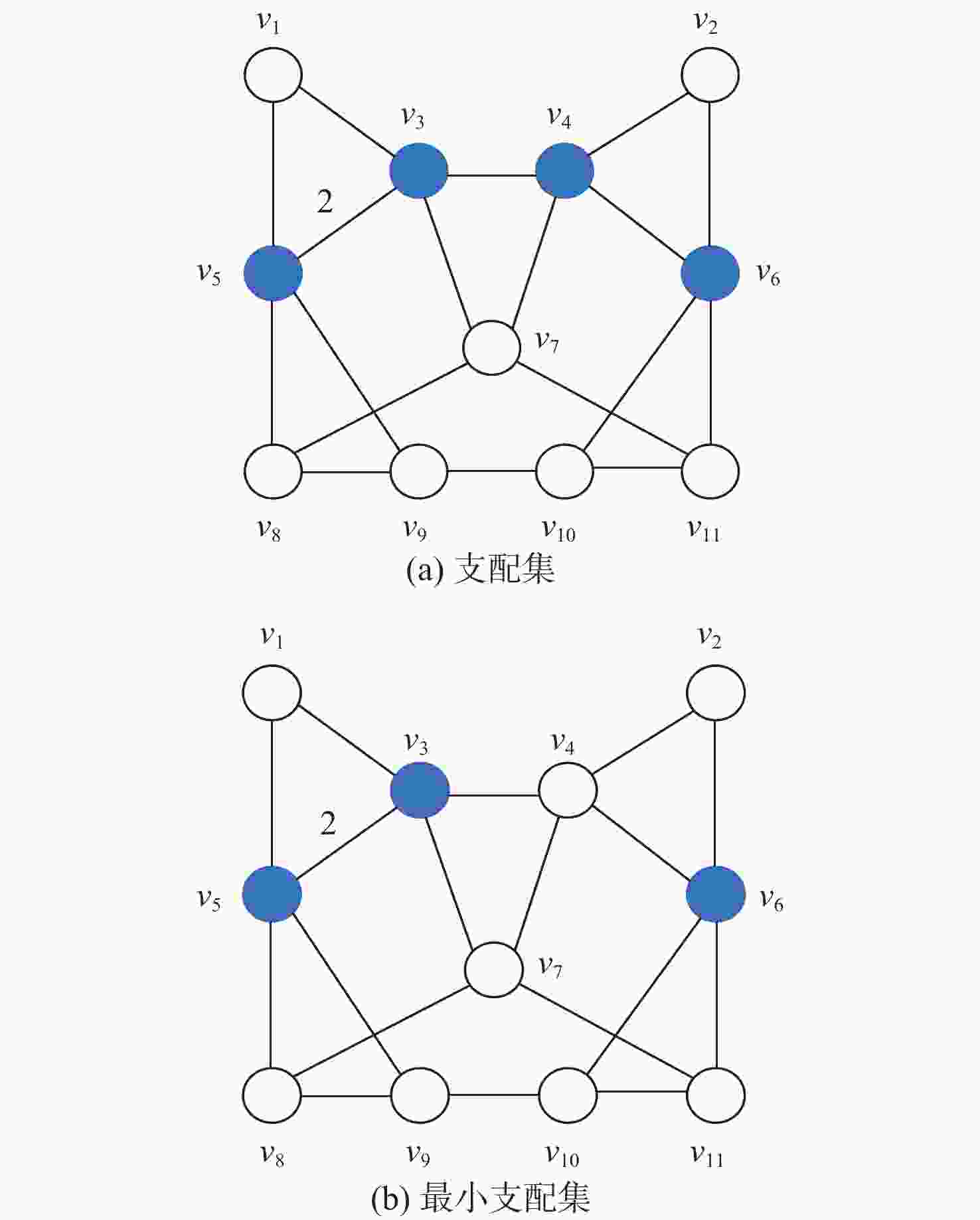
Conflict resolution strategy based on optimal dominating set of flight conflict networks

WU Minggong^{1, 2},
BI Kexin^{1, 2},
WEN Xiangxi^{1, 2
, ,},
SUN Jikun³

1.
ATC & GCI College，Air Force Engineering University，Xi’an 710051，China
2.
National Key Laboratory of Air Traffic Collision Prevention，Xi’an 710051，China
3.
Unit 93735 of the PLA，Tianjin 301799，China

Funds: National Natural Science Foundation of China (71801221); National Social Science Fund of China (19BGL297)

More Information

Corresponding author: E-mail：wxxajy@163.com

摘要

摘要:
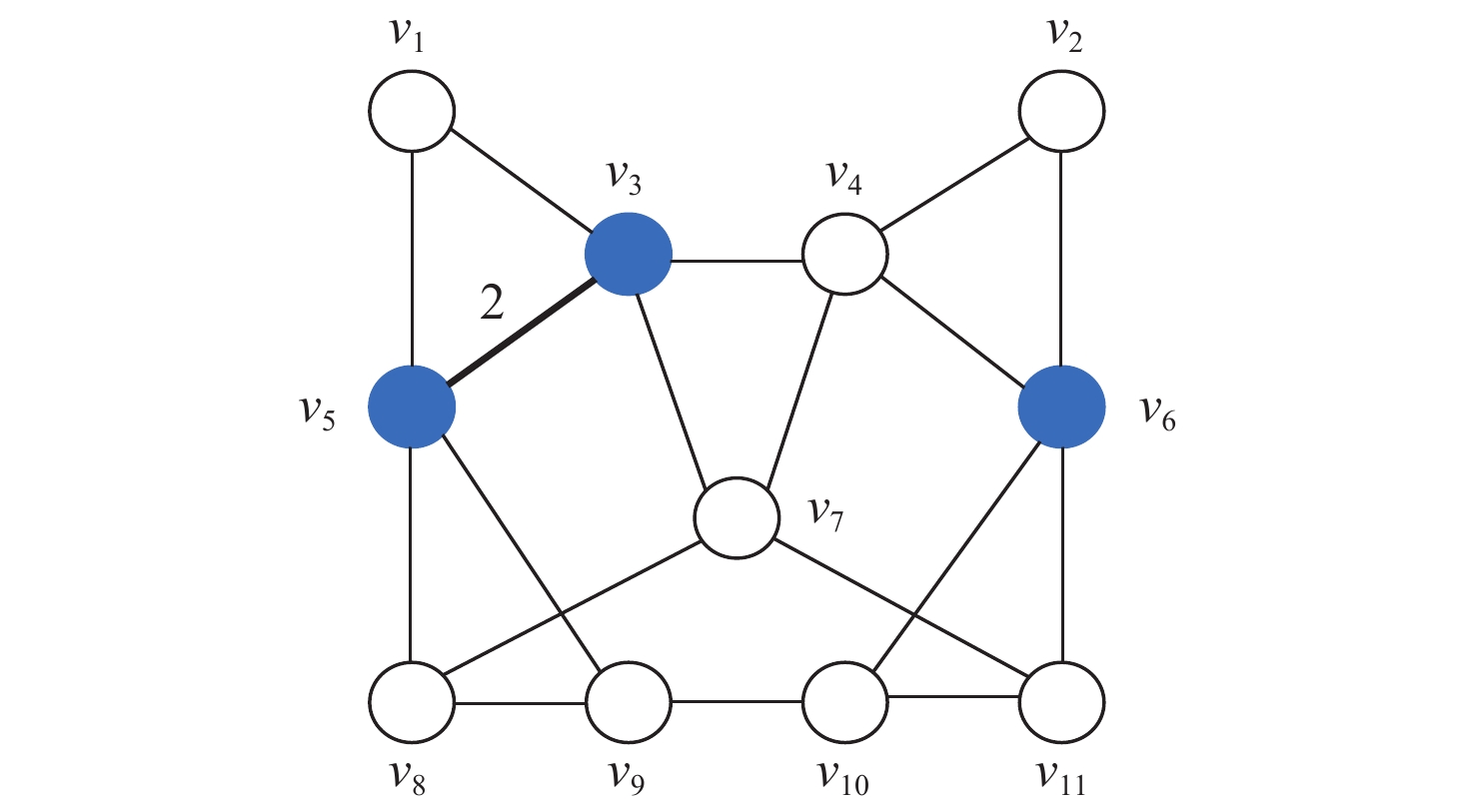
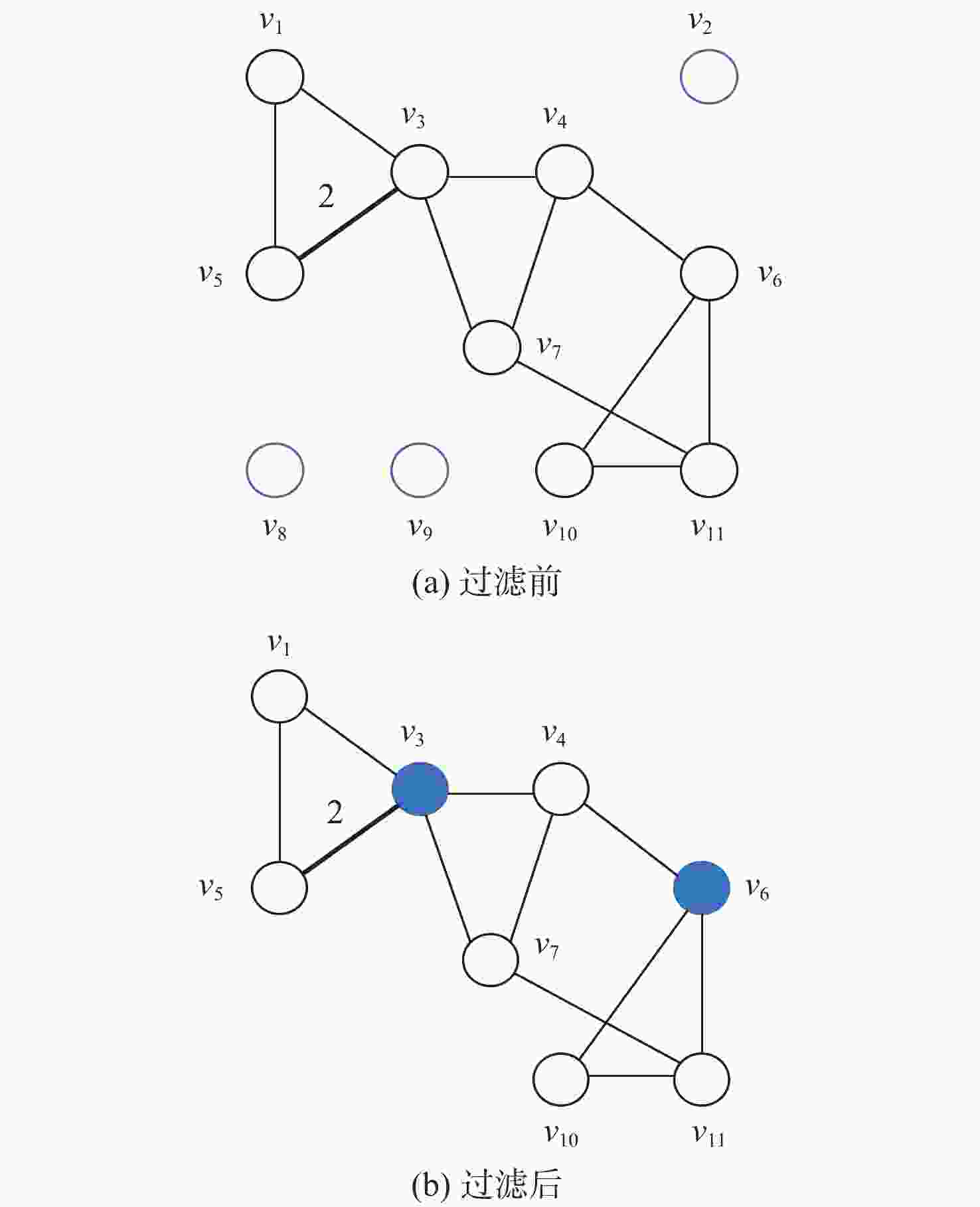
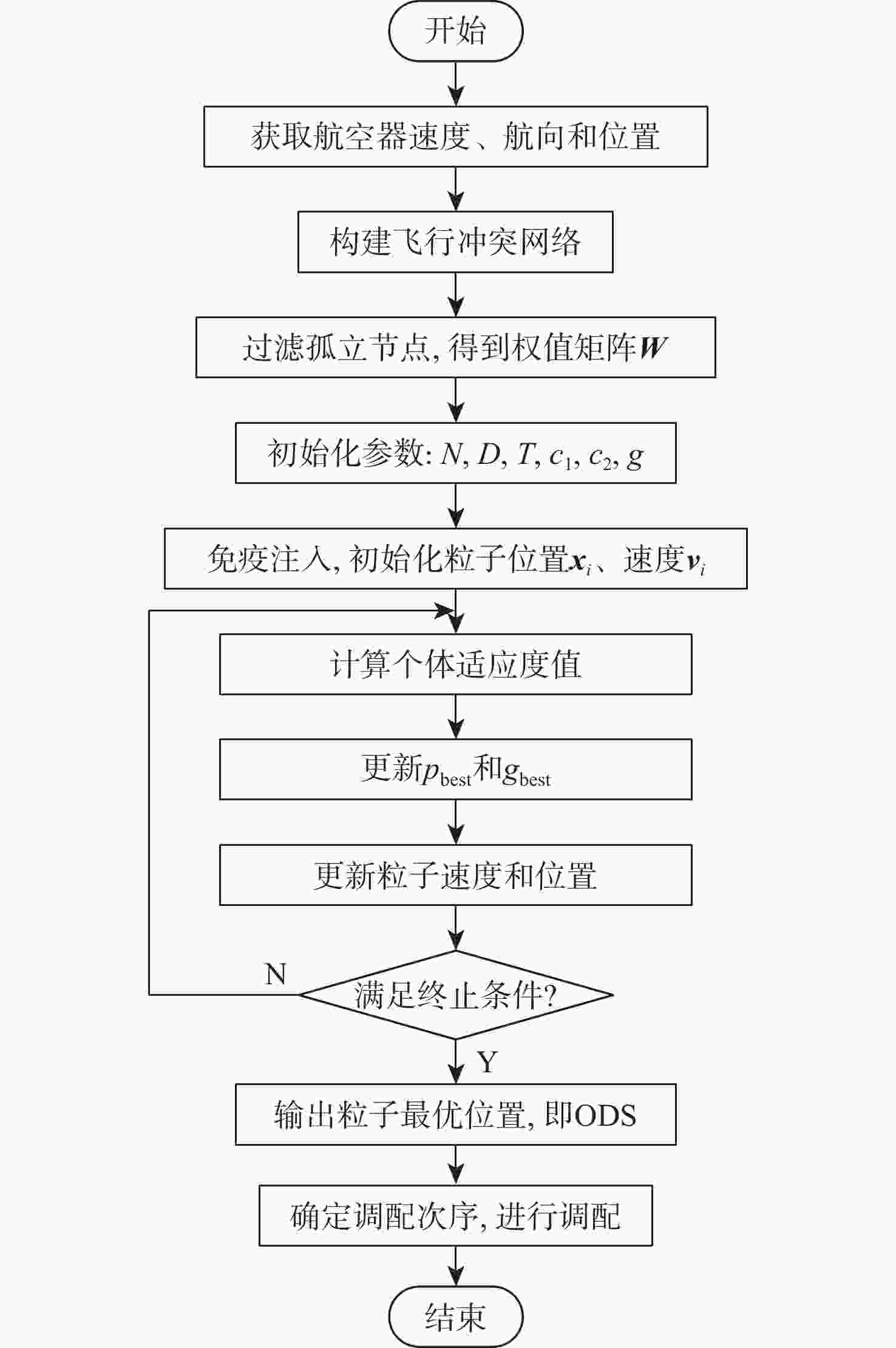
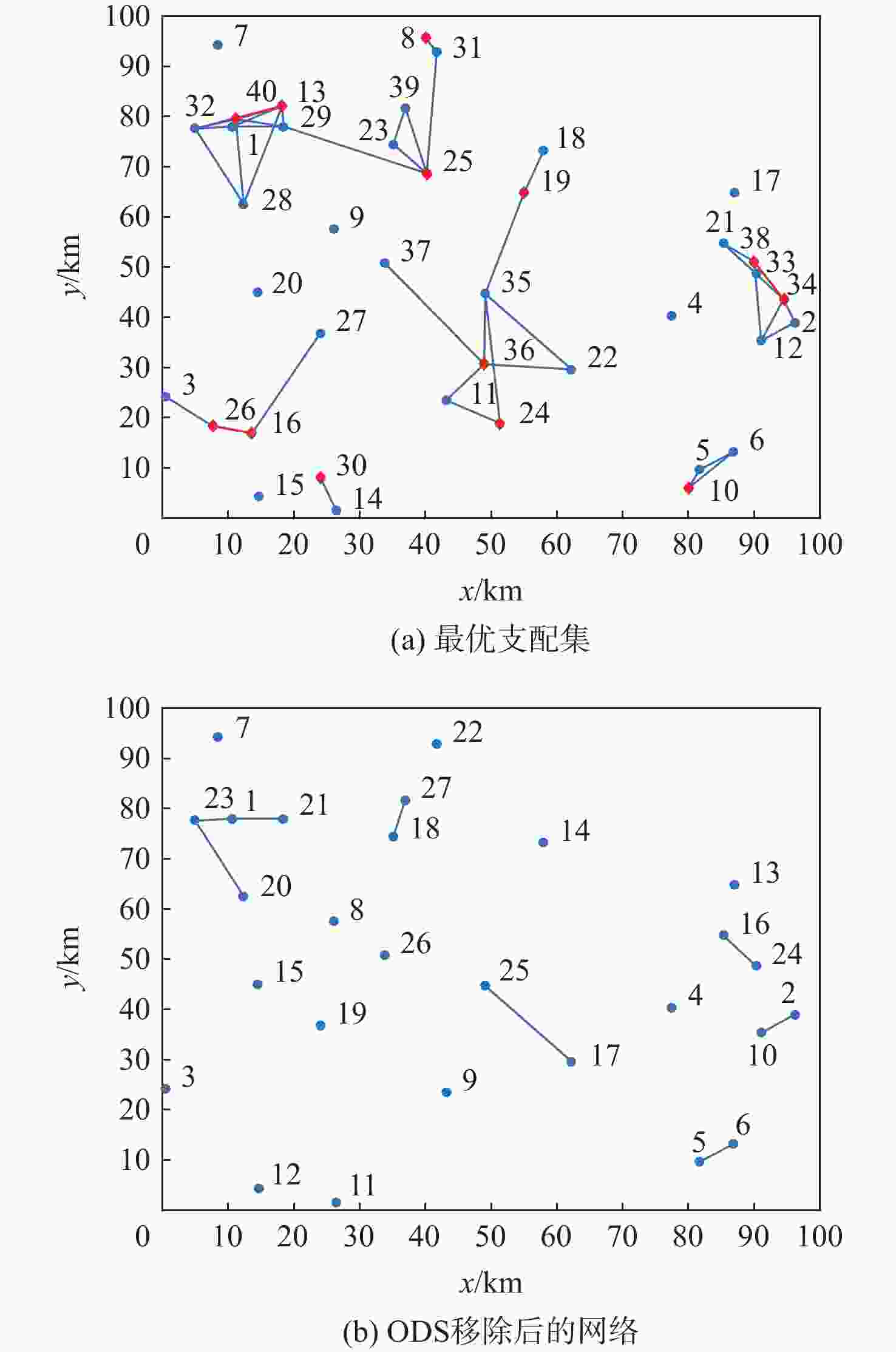
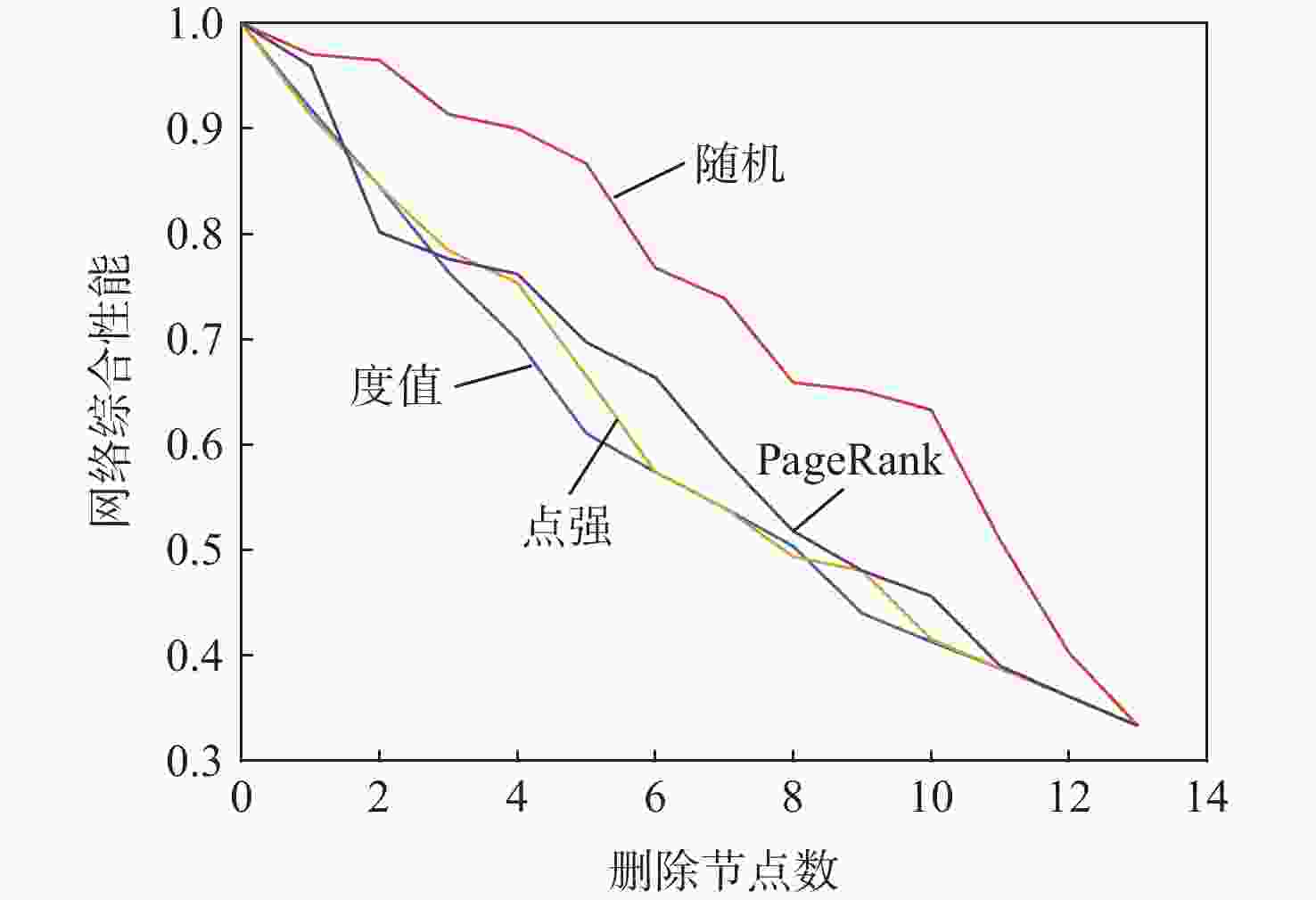
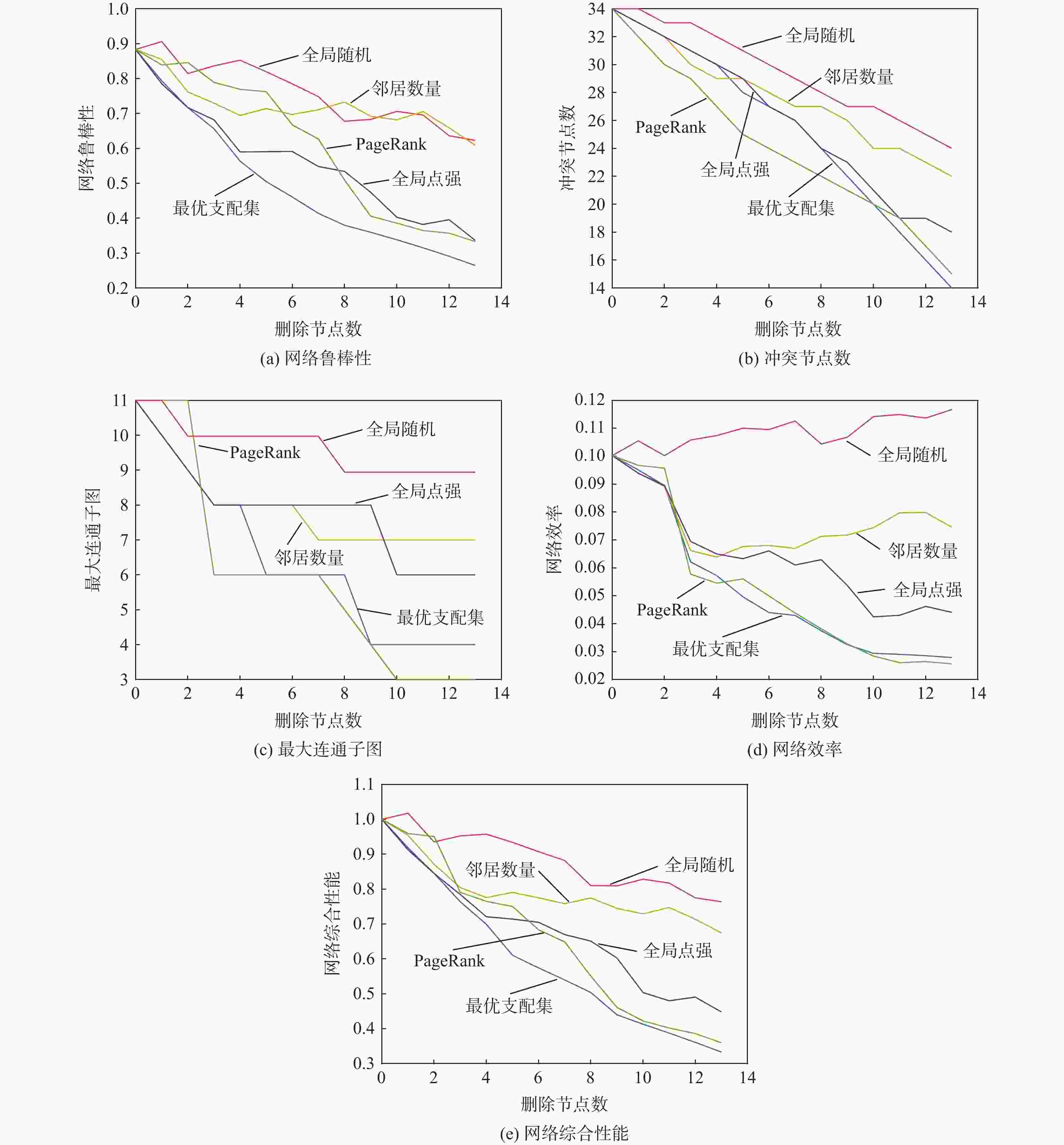
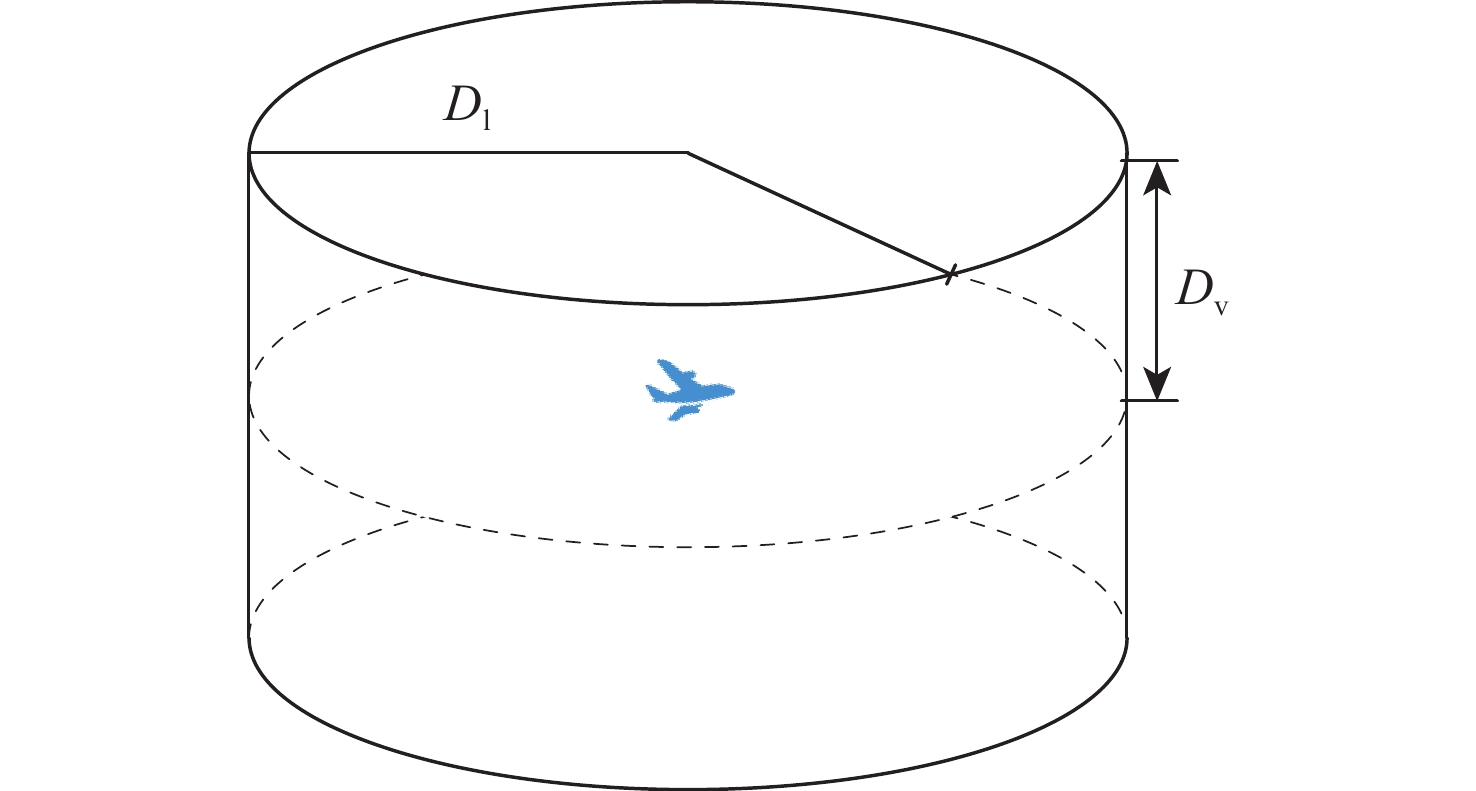
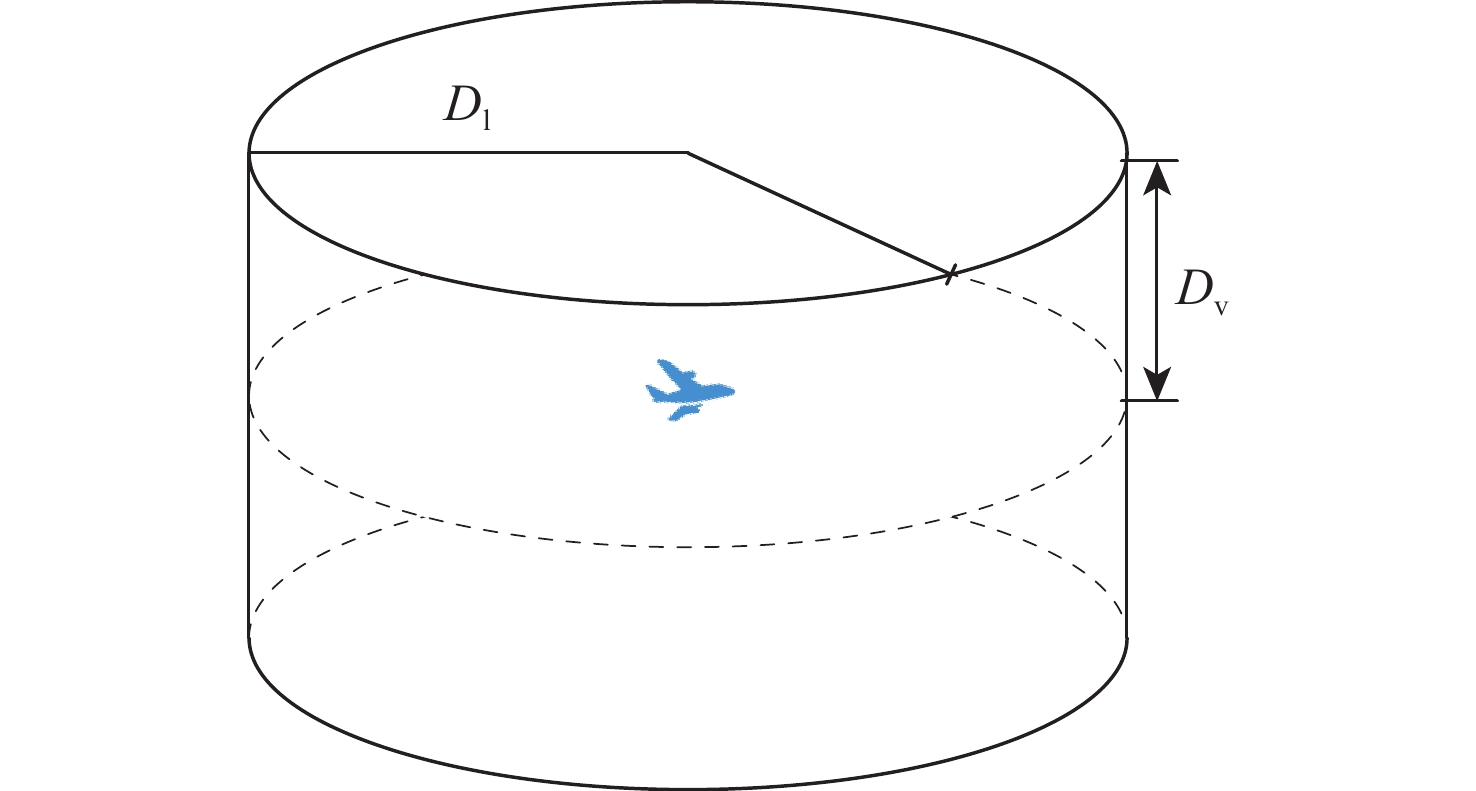
针对空中交通流量逐年上升、管制压力增大、飞行冲突难调配的问题，以航空器为节点，基于航空器之间的速度障碍关系建立飞行冲突网络。定义最优支配集的概念，通过移除飞行冲突网络的最优支配集节点，快速消解网络中的冲突，降低网络的复杂性。在使用粒子群（PSO）算法对网络最优支配集进行求解的过程中，引入免疫机制，设置节点和连边2种类型的抗原，保证对关键航空器和高风险冲突的优先调配。实验仿真表明：所提冲突调配策略相较于传统方法能够快速识别网络中的关键航空器节点，并对高风险的冲突连边具有较好的灵敏性，可为管制员和管制系统提供更加准确、可靠的信息和建议，在宏观上辅助进行飞行冲突的调配。
- 复杂网络 /
- 最优支配集 /
- 冲突调配 /
- 粒子群算法 /
- 速度障碍法
Abstract:
As air traffic flow grows year by year, control pressure keeps rising and to find a resolution to flight conflict is increasingly difficult. This paper takes aircrafts as the nodes and establishes a flight conflict network based on the velocity obstacle relationship between aircrafts. Then, the concept of optimal dominating set is defined. By eliminating the nodes in the optimal dominating set of the flight conflict network, the conflict could be resolved quickly, thus reducing the complexity of the network. While particle swarm optimization (PSO) algorithm is used in solving the network optimal dominating set, the immune mechanism is introduced, with two types of antigens, node and edge, being set to ensure the priority resolution of critical aircraft and high-risk conflicts. Compared with traditional method, the conflict resolution strategy presented in this paper can quickly identify key aircraft nodes in the network, and has good sensitivity to high-risk conflict edges, which can offer controllers and control system more accurate and reliable information to achieve flight conflict resolution.
- complex networks /
- optimal dominant set /
- conflict resolution /
- particle swarm optimization algorithm /
- velocity obstacle method

HTML全文

图 1 位置探测区

Figure 1. Position detection area

下载: 全尺寸图片幻灯片

图 2 椭球形飞行保护区

Figure 2. Ellipsoidal flight protection zone

下载: 全尺寸图片幻灯片

图 3 速度障碍法

Figure 3. Velocity obstacle method

下载: 全尺寸图片幻灯片

图 4 三维速度障碍模型

Figure 4. 3D velocity obstacle model

下载: 全尺寸图片幻灯片

图 5 飞行冲突网络示意图

Figure 5. Schematic of flight collision network

下载: 全尺寸图片幻灯片

图 6 最小支配集示意图

Figure 6. Schematic of minimum dominant set

下载: 全尺寸图片幻灯片

图 7 最优支配集

Figure 7. Optimal domination set

下载: 全尺寸图片幻灯片

图 8 过滤孤立节点

Figure 8. Filtration of isolated nodes

下载: 全尺寸图片幻灯片

图 9 冲突调配步骤

Figure 9. Conflict resolution process

下载: 全尺寸图片幻灯片

图 10 昆明长水国际机场空域雷达图像

Figure 10. Airspace radar image of Kunming Changshui International Airport

下载: 全尺寸图片幻灯片

图 11 网络对比

Figure 11. Network comparison

下载: 全尺寸图片幻灯片

图 12 两种网络节点度值的比较

Figure 12. Node degree comparison of two networks

下载: 全尺寸图片幻灯片

图 13 两种算法的对比

Figure 13. Comparison of two algorithms

下载: 全尺寸图片幻灯片

图 14 从网络中移除最优支配集

Figure 14. Removal of ODS from network

下载: 全尺寸图片幻灯片

图 15 ODS移除顺序对网络性能下降速度的影响

Figure 15. Effect of ODS removal order on network performance degradation rate

下载: 全尺寸图片幻灯片

图 16 不同删除方法对网络性能的影响

Figure 16. Impact of different deletion methods on network performance

下载: 全尺寸图片幻灯片

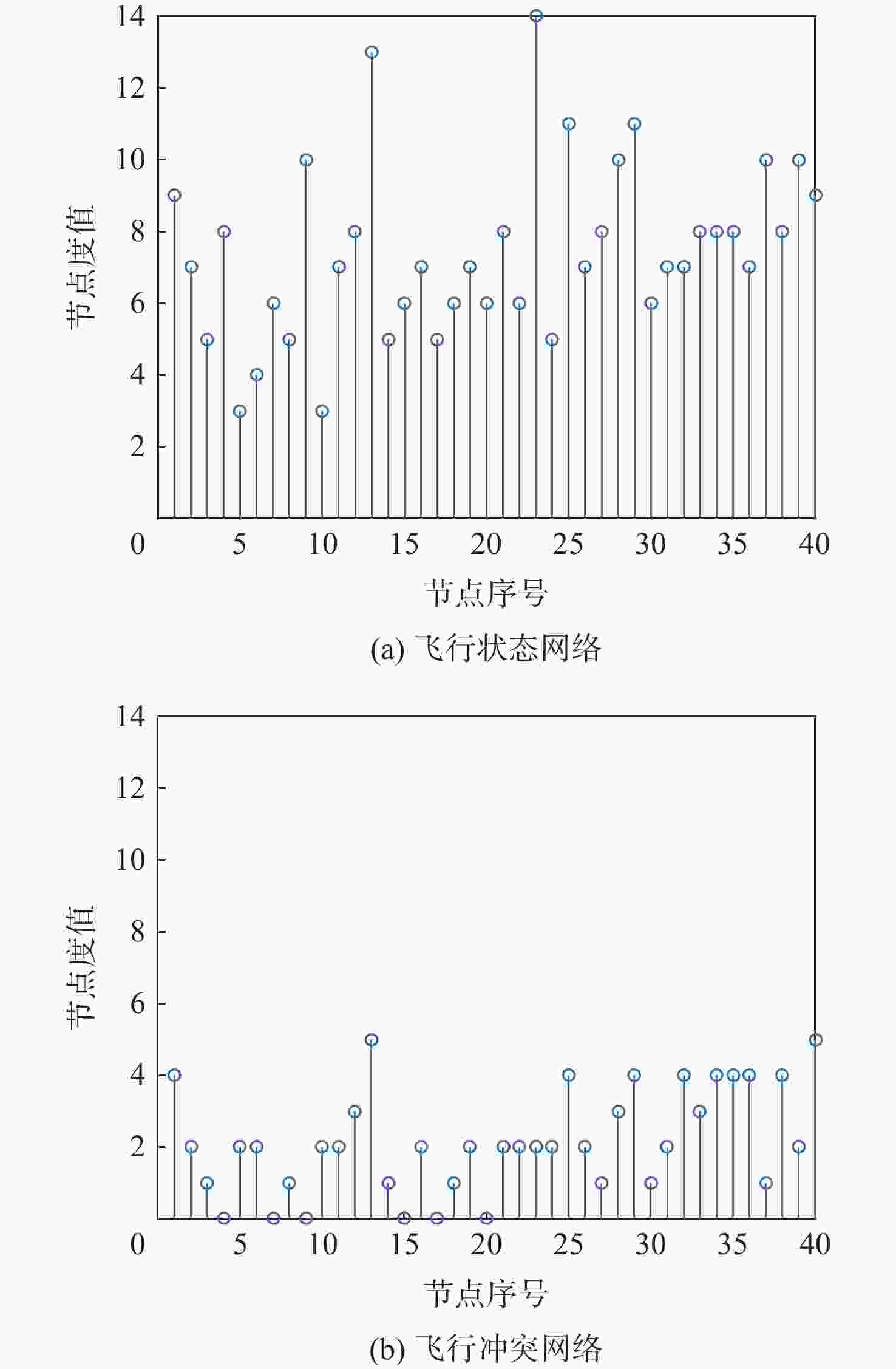
表 1 两种网络中节点的度值

Table 1. Node degrees in two networks

序号	节点度值		序号	节点度值
序号	飞行状态网络	飞行冲突网络	序号	飞行状态网络	飞行冲突网络
1	8	4	21	7	2
2	6	2	22	5	2
3	4	1	23	13	2
4	7	0	24	4	2
5	2	2	25	10	4
6	3	2	26	6	2
7	5	0	27	7	1
8	4	1	28	9	3
9	9	0	29	10	4
10	2	2	30	5	1
11	6	2	31	6	2
12	7	3	32	6	4
13	12	5	33	7	3
14	4	1	34	7	4
15	5	0	35	7	4
16	6	2	36	6	4
17	4	0	37	9	1
18	5	1	38	7	4
19	6	2	39	9	2
20	5	0	40	8	5

下载: 导出CSV

参考文献(19)

[1]	CHE J J. Report of civil aviation airspace development in China 2019: CACC-2020-09[R]. Beijing: CAAC, 2020.
[2]	张启钱, 王中叶, 张洪海, 等. 基于SMILO-VTAC模型的复杂低空多机冲突解脱方法[J]. 交通运输工程学报, 2019, 19(6): 125-136. ZHANG Q Q, WANG Z Y, ZHANG H H, et al. SMILO-VTAC model based multi-aircraft conflict resolution method in complex low-altitude airspace[J]. Journal of Traffic and Transportation Engineering, 2019, 19(6): 125-136(in Chinese).
[3]	王泽坤, 吴明功, 温祥西, 等. 基于速度障碍法的飞行冲突解脱与恢复策略[J]. 北京航空航天大学学报, 2019, 45(7): 1294-1302. WANG Z K, WU M G, WEN X X, et al. Flight collision resolution and recovery strategy based on velocity obstacle method[J]. Journal of Beijing University of Aeronautics and Astronautics, 2019, 45(7): 1294-1302(in Chinese).
[4]	蒋旭瑞, 吴明功, 温祥西, 等. 基于合作博弈的多机飞行冲突解脱策略[J]. 系统工程与电子技术, 2018, 40(11): 2482-2489. doi: 10.3969/j.issn.1001-506X.2018.11.14 JIANG X R, WU M G, WEN X X, et al. Conflict resolution of multi-aircraft based on the cooperative game[J]. Systems Engineering and Electronics, 2018, 40(11): 2482-2489(in Chinese). doi: 10.3969/j.issn.1001-506X.2018.11.14
[5]	陈伟锋, 邵之江. 基于析取关系直接变换的冲突解脱方法[J]. 航空学报, 2014, 35(4): 1122-1133. CHEN W F, SHAO Z J. Direct disjunction transcription based conflict resolution approach[J]. Acta Aeronautica et Astronautica Sinica, 2014, 35(4): 1122-1133(in Chinese).
[6]	HUANG S M, FERON E, REED G, et al. Compact configuration of aircraft flows at intersections[J]. IEEE Transactions on Intelligent Transportation Systems, 2014, 15(2): 771-783.
[7]	HONG Y, CHOI B, LEE K, et al. Conflict management considering a smooth transition of aircraft into adjacent airspace[J]. IEEE Transactions on Intelligent Transportation Systems, 2016, 17(9): 2490-2501.
[8]	VALENZUELA A, RIVAS D. Conflict resolution in converging air traffic using trajectory patterns[J]. Journal of Guidance, Control, and Dynamics, 2011, 34(4): 1172-1189.
[9]	CAFIERI S, REY D. Maximizing the number of conflict-free aircraft using mixed-integer nonlinear programming[J]. Computers & Operations Research, 2017, 80: 147-158.
[10]	WANG H Y, XU X H, ZHAO Y F. Empirical analysis of aircraft clusters in air traffic situation networks[J]. Proceedings of the Institution of Mechanical Engineers. Part G:Journal of Aerospace Engineering, 2017, 231(9): 1718-1731. doi: 10.1177/0954410016660870
[11]	JIANG X R, WEN X X, WU M G, et al. A complex network analysis approach for identifying air traffic congestion based on independent component analysis[J]. Physica A-Statistical Mechanics and Its Applications, 2019, 523: 364-381. doi: 10.1016/j.physa.2019.01.129
[12]	HUANG Y, TANG J, LAO S Y. Cooperative multi-UAV collision avoidance based on a complex network[J]. Applied Sciences-Basel, 2019, 9(19): 3943. doi: 10.3390/app9193943
[13]	JENIE Y I, KAMPEN E J, VISSER C C, et al. Three-dimensional velocity obstacle method for uncoordinated avoidance maneuvers of unmanned aerial vehicles[J]. Journal of Guidance, Control, and Dynamics, 2016, 39(10): 2312-2323. doi: 10.2514/1.G001715
[14]	王凯莉, 邬春学, 艾均, 等. 基于多阶邻居壳数的向量中心性度量方法[J]. 物理学报, 2019, 68(19): 235-245. WANK K L, WU C X, AI J, et al. Complex network centrality method based on multi-order K-shell vector[J]. Acta Physica Sinica, 2019, 68(19): 235-245(in Chinese).
[15]	何明, 许元云, 刘锦涛, 等基于k-shell分解的多智能体牵制控制算法[J]. 控制与决策, 2020, 35(10): 2556-2560. HE M, XU Y Y, LIU J T, et al. Multi-agent pinning control algorithm based on k-shell decomposition[J]. Control and Decision, 2020, 35(10): 2556-2560(in Chinese).
[16]	王江盼, 郭强, 刘建国. 基于PageRank的合著论文中作者贡献分配算法[J]. 电子科技大学学报, 2020, 49(6): 918-923. WANG J P, GUO Q, LIU J G. Credit allocation for each author in a multi-author paper based on PageRank[J]. Journal of University of Electronic Science and Technology of China, 2020, 49(6): 918-923(in Chinese).
[17]	LI J W, WEN X X, WU M G, et al. Identification of key nodes and vital edges in aviation network based on minimum connected dominating set[J]. Physica A-Statistical Mechanics and Its Applications, 2020, 541: 123340.
[18]	殷剑宏, 吴开亚. 图论及其算法[M]. 合肥: 中国科学技术大学出版社, 2003: 188-197. YIN J H, WU K Y. Graph theory and its algorithm[M]. Hefei: University of Science and Technology of China Press, 2003: 188-197 (in Chinese).
[19]	高鹰, 谢胜利. 免疫粒子群优化算法[J]. 计算机工程与应用, 2004(6): 4-6. doi: 10.3321/j.issn:1002-8331.2004.06.002 GAO Y, XIE S L. Particle swarm optimization algorithms with immunity[J]. Computer Engineering and Applications, 2004(6): 4-6(in Chinese). doi: 10.3321/j.issn:1002-8331.2004.06.002