-
摘要:
为应对新冠疫情影响背景下客机航线中断导致客机腹舱运力减少的风险，以最小的成本提升航空物流网络的鲁棒性，提出不确定客机腹舱运力情境下全货机航线配机及路径优化问题研究。以网络总成本最小化为目标，考虑“客机腹舱+全货机”的运输方式、城市间的航空货运需求、运输距离等因素，建立了客机腹舱运力不确定环境下航空物流网络鲁棒优化模型，并采用列与约束生成（C&CG）算法对模型进行有效求解。通过S货运航空公司案例数据，研究航空物流网络航线优化布局问题。通过案例分析证明，运用两阶段鲁棒优化方法，可有效降低航空物流网络受客机腹舱运力减少导致的成本变动；在客机航线完全中断的状况下，所提方案可以降低航空物流网络20.7%的成本。运用两阶段鲁棒优化方法有利于决策者灵活进行全货机配机选线，在客机腹舱运力不确定影响背景下兼顾航空物流网络的经济性和鲁棒性。
Abstract:To deal with the risk of reducing the capacity of passenger aircraft bellies due to the interruption of passenger aircraft routes in the context of COVID-19 pandemic, and to improve the robustness of the aviation logistics network at a minimum cost, a study on the allocation and route optimization of all-cargo aircraft routes under the situation of uncertain passenger aircraft belly storage capacity was proposed. With the objective of minimizing the total cost of the route network while considering transportation mode, air cargo demand, and transportation distance between cities, this study established a robust optimization model for the aviation logistics network under the uncertain passenger aircraft bellies. The column-and-constraint generation (C&CG) algorithm was effectively used to solve the model. In addition, this paper investigated the optimized layout of aviation logistics network routes using the case study and data of company S. The results show that the two-stage robust optimization method for aviation logistics network can effectively reduce cost changes caused by reduced passenger aircraft belly capacity, and the robust solution can reduce the cost of the aviation logistics network by 20.7% in the extreme case of complete disruption of passenger routes. Therefore, this study concluded that the use of the two-stage robust optimization method enables decision-makers to flexibly select cargo aircraft routing while considering the economy and robustness of the aviation logistics network under the influence of uncertainty surrounding passenger aircraft belly capacity.
-
图 1 不确定客机腹舱运力下货机航线路径优化
Figure 1. Route optimization of cargo aircraft under uncertainty for capacity of passenger aircraft bellies
图 2 C&CG算法迭代次数相关的收敛过程
Figure 2. Convergence process related to number of iterations of C&CG algorithm
图 3 Benders分解法迭代次数相关的收敛过程
Figure 3. Convergence process related to number of iterations of Benders algorithm
图 4 $\zeta $为0、0.1和0.2时各航空物流网络成本比较
Figure 4. Comparison of cost of each aviation logistics network plan when $\zeta $ is 0, 0.1 and 0.2 respectively
图 5 $\zeta $为0.8、0.9和1时各航空物流网络成本比较
Figure 5. Comparison of cost of each aviation logistics network plan when $\zeta $ is 0.8, 0.9 and 1 respectively
图 6 $\zeta $为0、0.5和1时各航空物流网络成本比较
Figure 6. Comparison of cost of each aviation logistics network plan when $\zeta $ is 0, 0.5 and 1 respectively
表 1 算法性能对比结果
Table 1. Algorithm performance comparison result
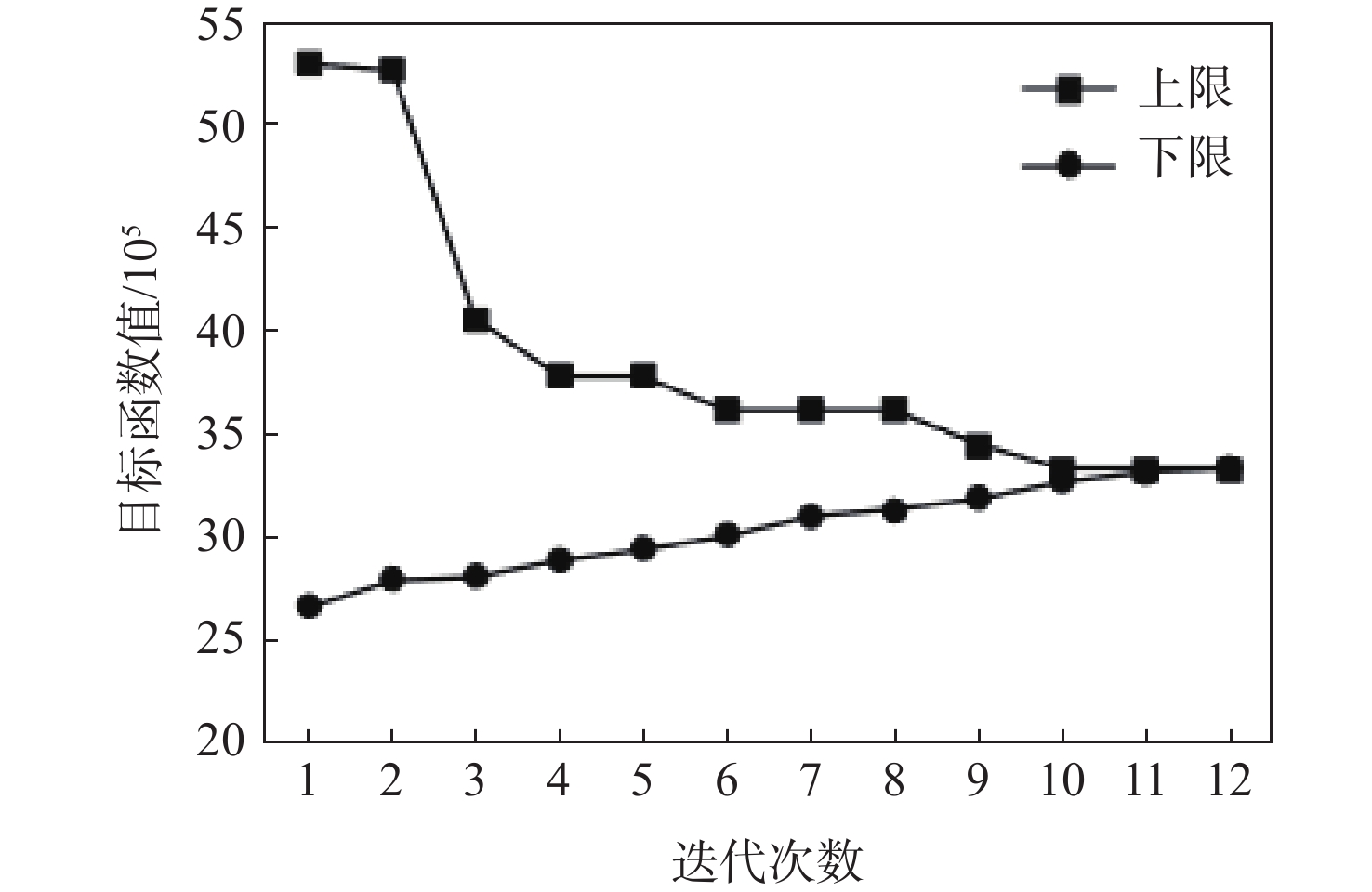
算法 $\varGamma$ 迭代次数 ${\text{gap}}$/% ${\text{UB}}$/104 时间/s Benders算法 0 18 40 603 3600 5 14 62 690 3600 10 14 51 831 3600 15 15 49 811 3600 C&CG算法 0 1 0 541 0.8 5 24 0 640 840 10 39 0 664 3367 15 17 0 666 1987 
下载: 导出CSV
表 2 不同不确定预算水平下求解的航线网络方案
Table 2. Route network solutions under different uncertain budget levels
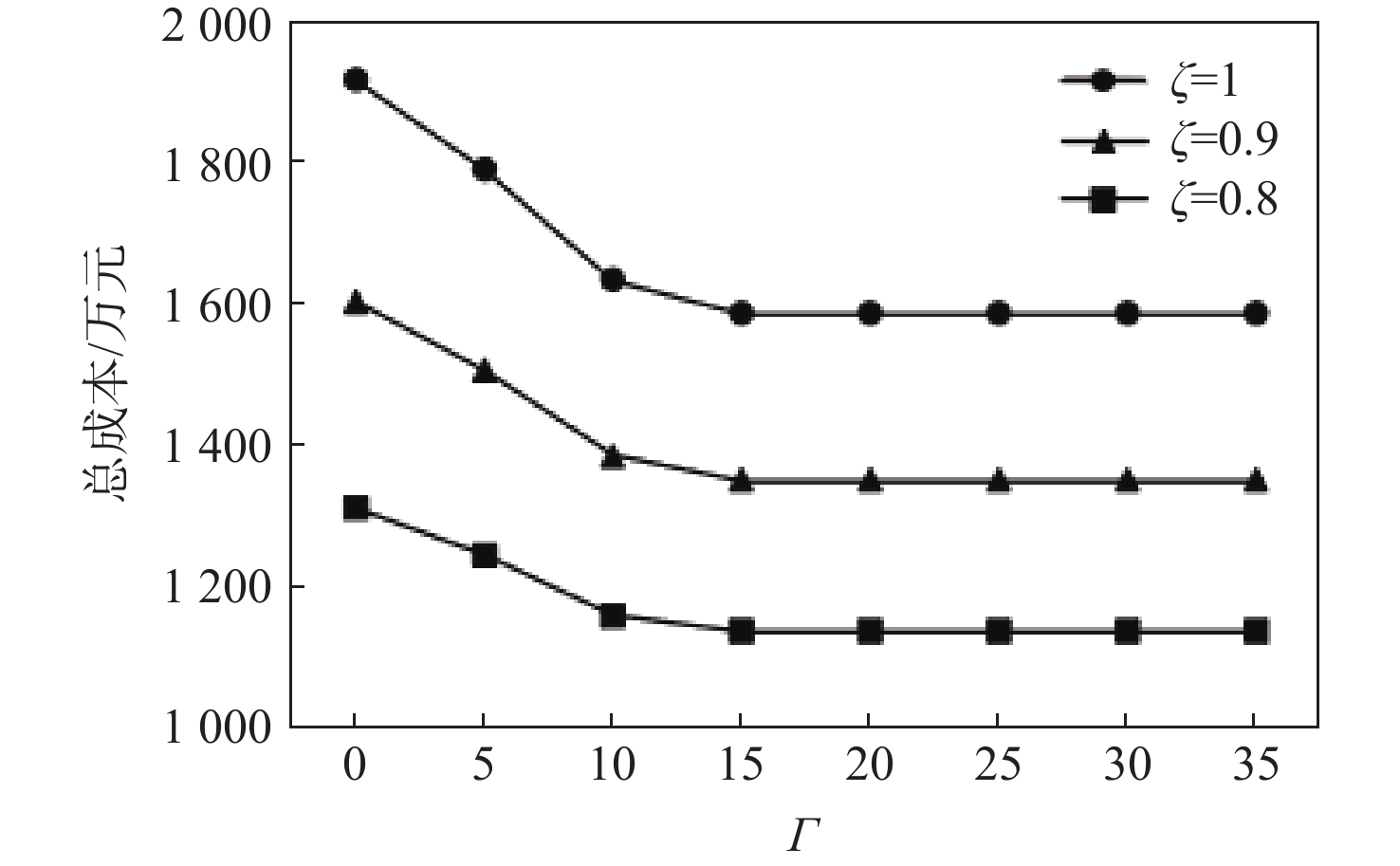
方案 Γ 货机航线路径 1 0 ①3-0-8-3;②3-2-5-3;③4-2-8-4； ④3-7-0-3;⑤3-0-9-3;⑥3-0-7-3; 2 5 ①3-9-0-3;②3-0-7-3;③3-0-8-3; ④3-2-5-3;⑤3-6-3;⑥3-0-9-3; ⑦4-2-8-4;⑧4-1-0-4; 3 10 ①3-4-3;②3-7-0-3;③4-1-0-4; ④3-9-2-3;⑤3-6-3;⑥3-0-8-3; ⑦4-2-8-4;⑧3-0-9-3;⑨3-0-7-3; ⑩3-2-5-3; 4 15 ①4-7-0-4;②3-5-2-3;③3-6-3; ④3-9-0-3;⑤3-7-1-3;⑥3-0-8-3; ⑦3-4-3;⑧4-1-7-4;⑨3-2-5-3; ⑩3-0-9-3;⑪3-0-7-3;⑫4-2-8-4; 5 20 ①4-7-0-4;②3-5-2-3;③3-6-3; ④3-9-0-3;⑤3-7-1-3;⑥3-0-8-3; ⑦3-4-3;⑧4-1-7-4;⑨3-2-5-3; ⑩3-0-9-3;⑪3-0-7-3;⑫4-2-8-4;
下载: 导出CSV
-
[1] DU W, ZHOU X, LORDAN O, et al. Analysis of the Chinese airline network as multi-layer networks[J]. Transportation Research Part E:Logistics and Transportation Review, 2016, 89: 108-116. doi: 10.1016/j.tre.2016.03.009 [2] O‘KELLY M E. A quadratic integer program for the location of interacting hub facilities[J]. European Journal of Operational Research, 1987, 32(3): 393-404. doi: 10.1016/S0377-2217(87)80007-3 [3] ZHANG J, YAN Y, TANG Q Y. Hub location of air cargo company in air alliance[J]. Journal of Southeast University (English Edition), 2020, 36(3): 341-348. [4] YAN Y, ZHANG J, TANG Q Y. Aircraft fleet route optimization based on cost and low carbon emission in aviation line alliance network[J]. Journal of Intelligent & Fuzzy Systems, 2020, 39(1): 1163-1182. [5] YU S N, YANG Z Z, YU B. Air express network design based on express path choices: Chinese case study[J]. Journal of Air Transport Management, 2017, 61: 73-80. doi: 10.1016/j.jairtraman.2016.04.008 [6] 高娇蛟. 我国快递企业航空运输网络的优化设计研究[D]. 北京: 北京交通大学, 2011: 40-46.GAO J J. Research on optimization design of air transport network of express enterprises in China[D]. Beijing: Beijing Jiaotong University, 2011: 40-46(in Chinese). [7] CHENG C, QI M, ZHANG Y, et al. A two-stage robust approach for the reliable logistics network design problem[J]. Transportation Research Part B:Methodological, 2018, 111: 185-202. doi: 10.1016/j.trb.2018.03.015 [8] LING A, SUN J, XIU N, et al. Robust two-stage stochastic linear optimization with risk aversion[J]. European Journal of Operational Research, 2017, 256(1): 215-229. doi: 10.1016/j.ejor.2016.06.017 [9] MARTINS D S, MORABITO R, CAMARGO R S. Benders decomposition applied to a robust multiple allocation incomplete hub location problem[J]. Computers & Operations Research, 2018, 89: 31-50. [10] 梁金鹏, 不确定条件下的城市轨道交通客流控制策略及接驳公交网络优化研究[D]. 北京: 北京交通大学, 2020: 85-96.LIANG J P. Research on passenger flow control policy and bus bridging service optimization under uncertainty in urban rail transit[D]. Beijing: Beijing Jiaotong University, 2020: 85-96(in Chinese). [11] WANG Z, QI M. Robust service network design under demand uncertainty[J]. Transportation Science, 2020, 54(3): 676-689. doi: 10.1287/trsc.2019.0935 [12] 陈焕平. 内支线集装箱班轮航线鲁棒优化模型[D]. 大连: 大连海事大学, 2020: 26-30.CHEN H P. Robust optimization model of container liner routes in feeder line network[D]. Dalian: Maritime Affairs University of Dalian, 2020: 26-30(in Chinese). [13] 姜涛. 航空公司中枢辐射航线网络鲁棒优化设计问题研究[D]. 南京: 南京航空航天大学, 2007.JIANG T. Research on the robust optimization design of airline’s hub and radial route network[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2007(in Chinese). [14] 张冲. 航空联盟网络设计与优化研究[D]. 南京: 南京航空航天大学, 2019: 40-49.ZHANG C. Research on design and optimization of aviation alliance network[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2019: 40-49(in Chinese). [15] BERTSIMAS D, SIM M. Robust discrete optimization and network flows[J]. Mathematical Programming, 2003, 98(1-3): 49-71. doi: 10.1007/s10107-003-0396-4 [16] GABREL V, LACROIX M, MURAT C, et al. Robust location transportation problems under uncertain demands[J]. Discrete Applied Mathematics, 2014, 164: 100-111. [17] ZENG B, ZHAO L. Solving two-stage robust optimization problems using a column-and-constraint generation method[J]. Operations Research Letters, 2013, 41(5): 457-461. doi: 10.1016/j.orl.2013.05.003 [18] 闫妍, 马啸来. 航线联营下基于转运的飞机航线路径优化[J]. 北京航空航天大学学报, 2023, 49(1): 115-127. doi: 10.13700/j.bh.1001-5965.2021.0166YAN Y, MA X L. Air freight route planning based on transshipment under air alliance[J]. Journal of Beijing University of Aeronautics and Astronautics, 2023, 49(1): 115-127(in Chinese). doi: 10.13700/j.bh.1001-5965.2021.0166 -
下载:
点击查看大图
计量
- 文章访问数: 304
- HTML全文浏览量: 551
- PDF下载量: 52
- 被引次数: 0
