超声速民机发动机短舱布局对声爆的影响

肖天航; 徐雅楠; 朱震浩; 邓双厚

doi:10.13700/j.bh.1001-5965.2021.0687

超声速民机发动机短舱布局对声爆的影响

doi: 10.13700/j.bh.1001-5965.2021.0687

南京航空航天大学航空学院，南京 210016

基金项目: 航天进入减速与着陆技术实验室开放基金 (EDL19092111)；国家自然科学基金(11672133)；陕西省自然科学基础研究计划(2021JQ-078)

详细信息

通讯作者:
Email：xthang@nuaa.edu.cn

中图分类号: V221⁺.3；TB553
计量
- 文章访问数: 236
- HTML全文浏览量: 472
- PDF下载量: 38
- 被引次数: 0
出版历程
- 收稿日期: 2021-11-16
- 录用日期: 2022-04-10
- 网络出版日期: 2022-04-21
- 整期出版日期: 2023-10-01

Effect of engine nacelle layout on sonic boom of supersonic transport

College of Aerospace Engineering，Nanjing University of Aeronautics and Astronautics，Nanjing 210016，China

Funds: Opening Foundation of EDL Laboratory (EDL19092111); National Natural Science Foundation of China (11672133); Natural Science Basic Research Programof Shaanxi (2021JQ-078)

More Information

Corresponding author: Email：xthang@nuaa.edu.cn

摘要

摘要:
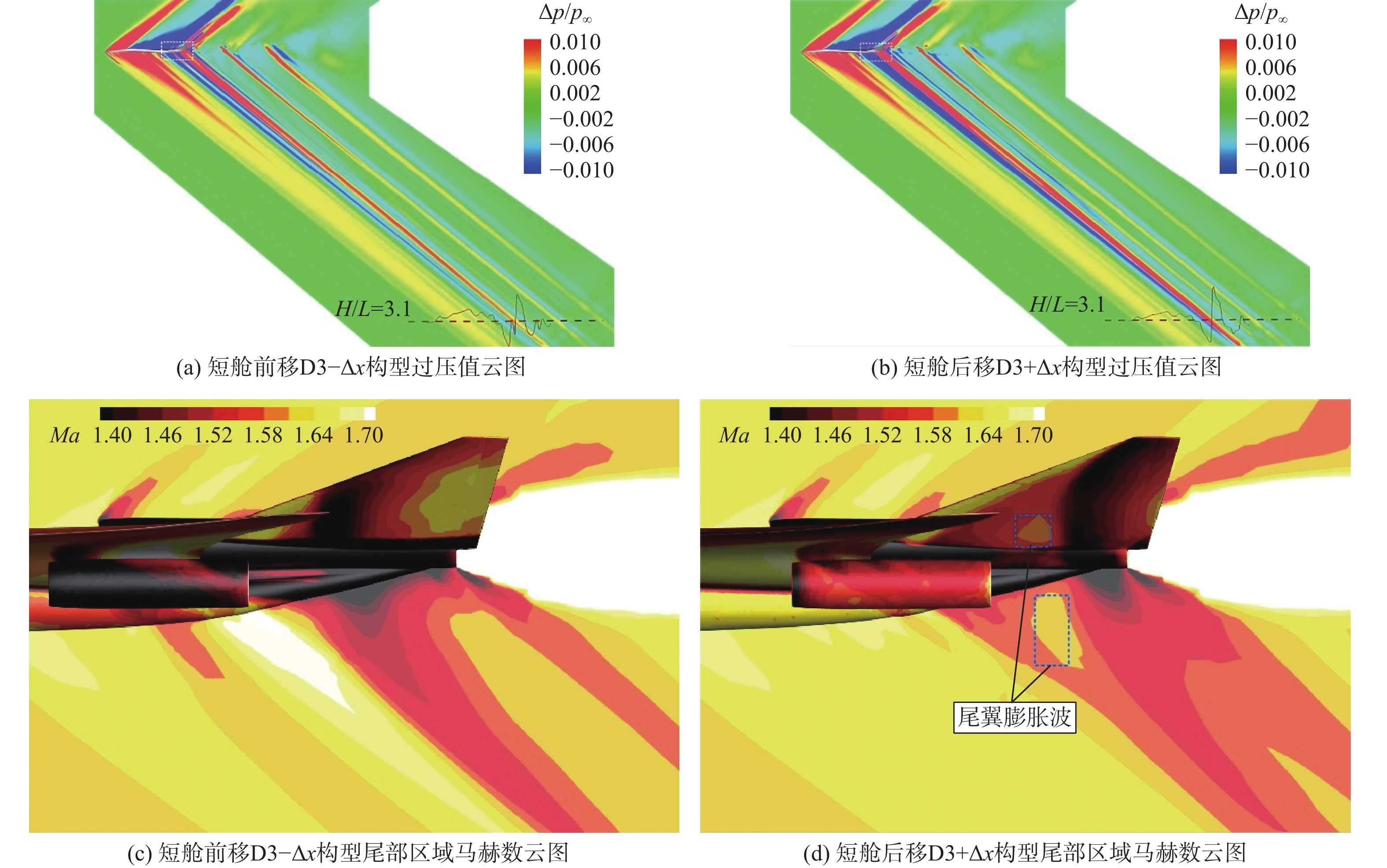
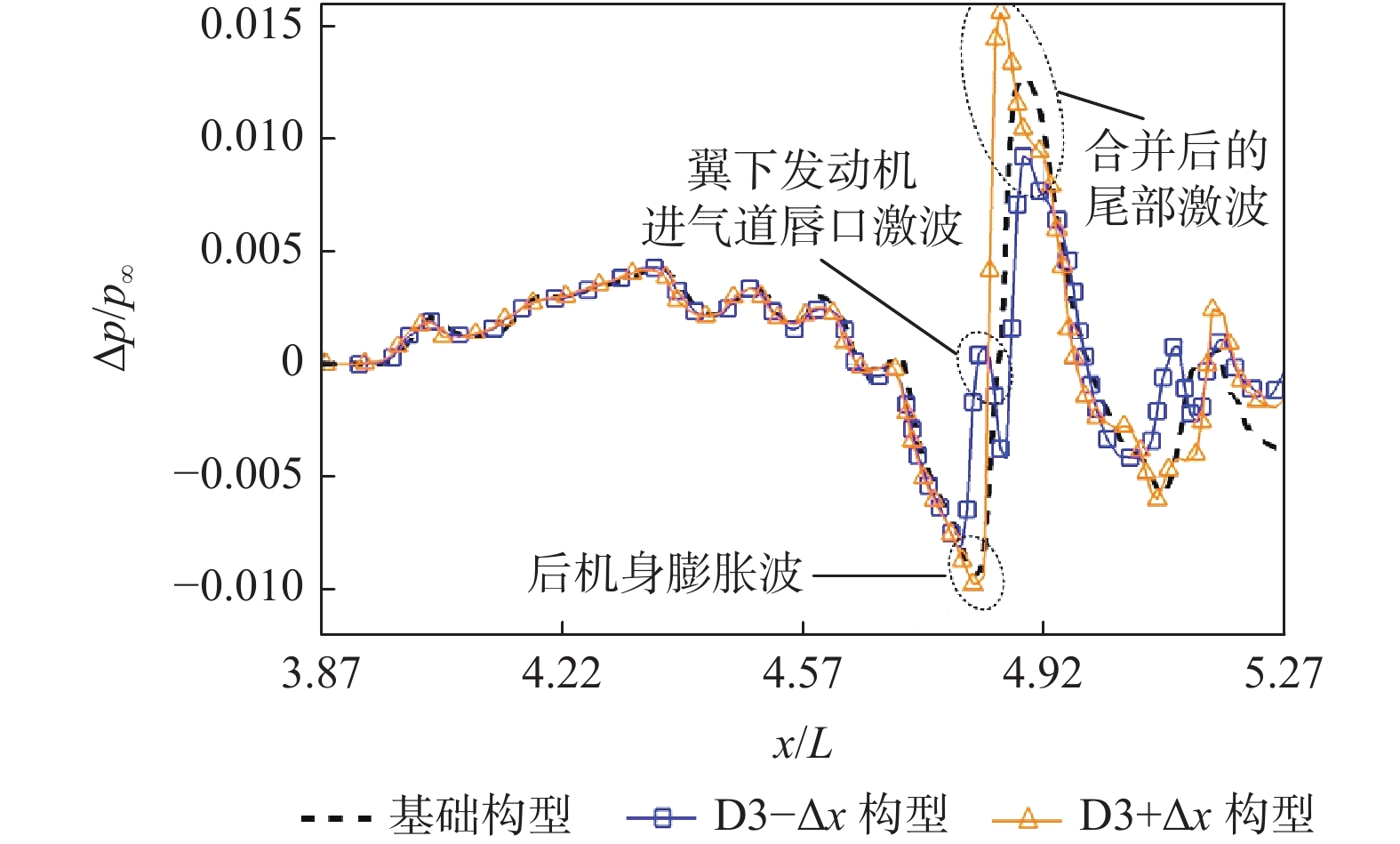
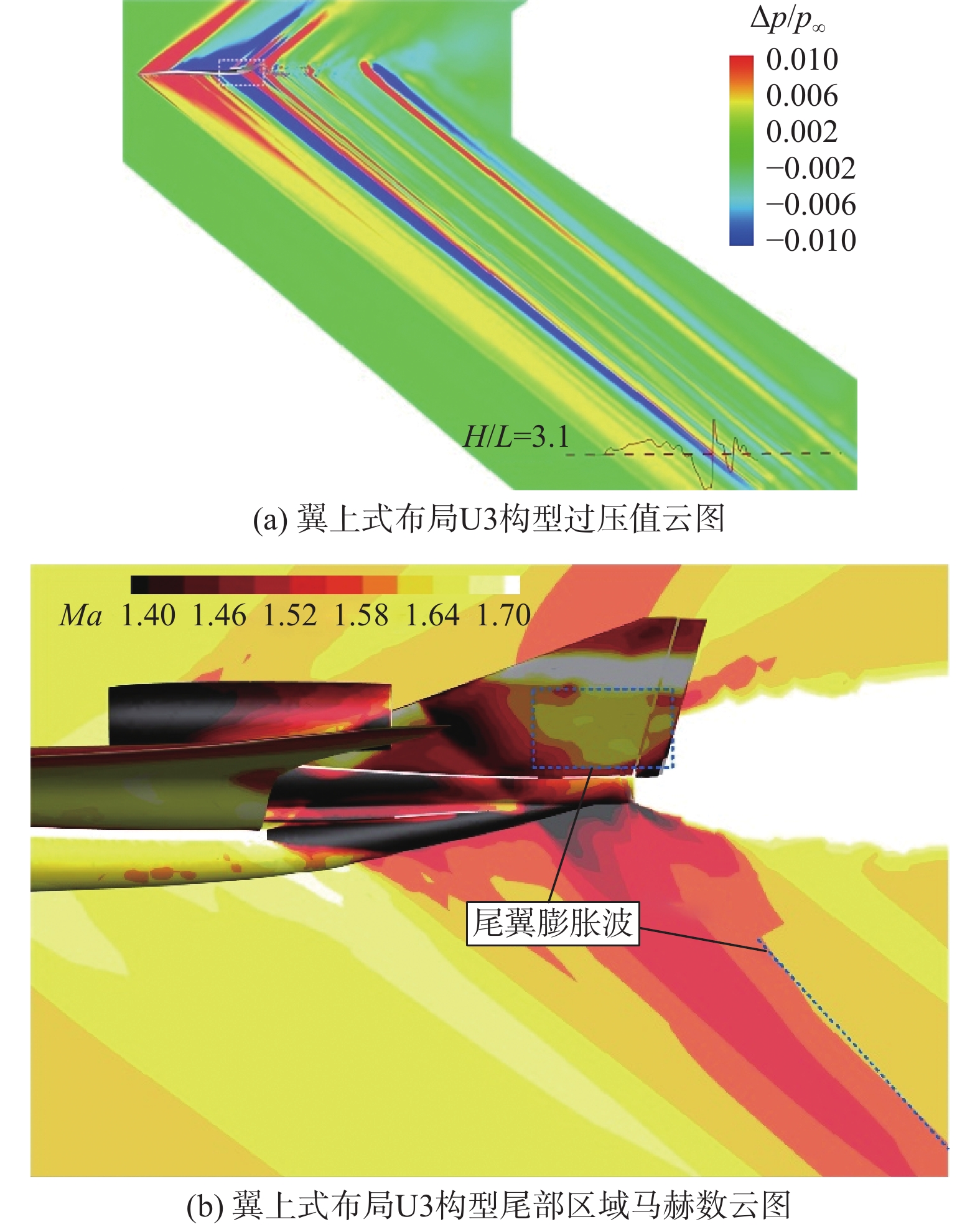
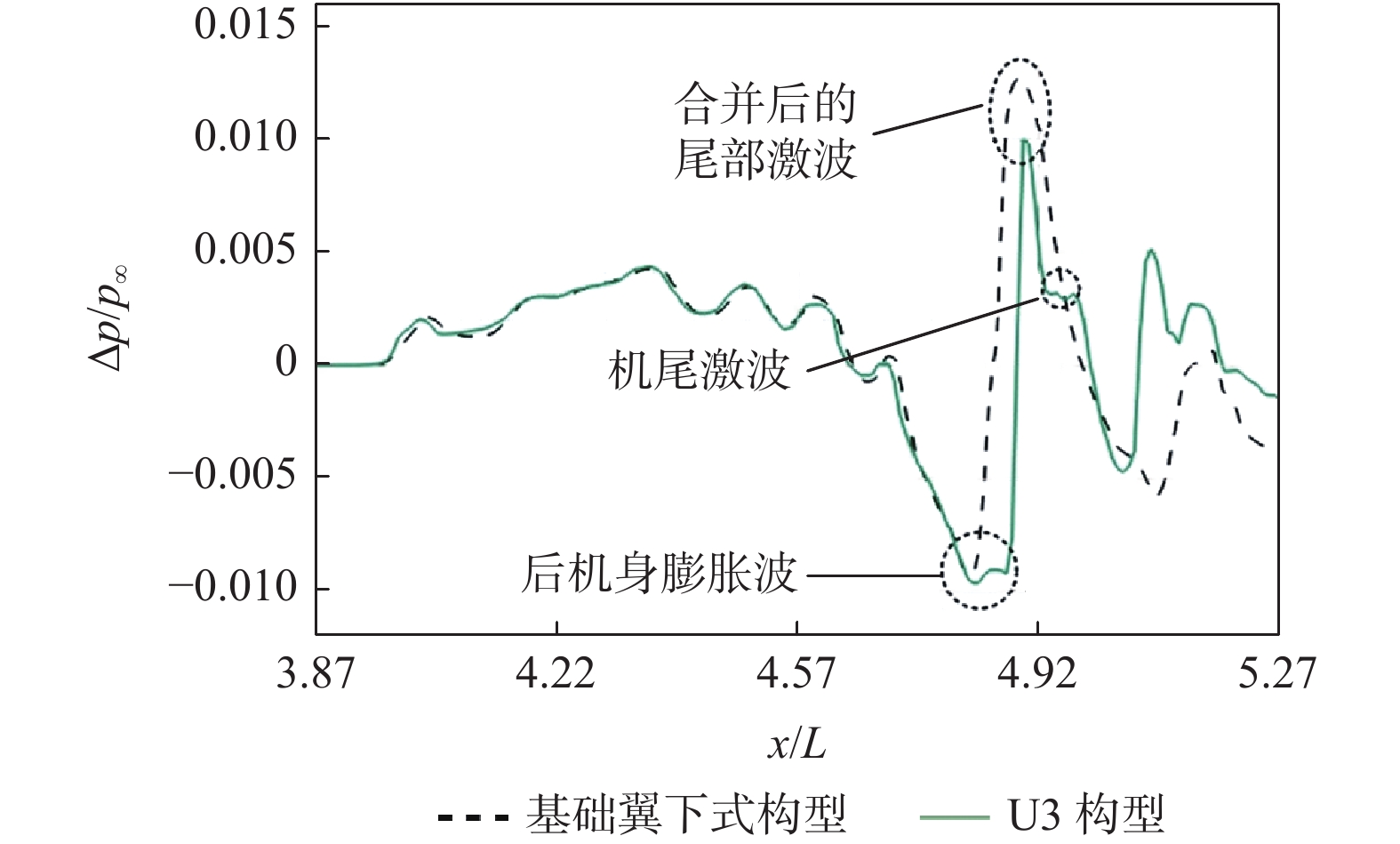
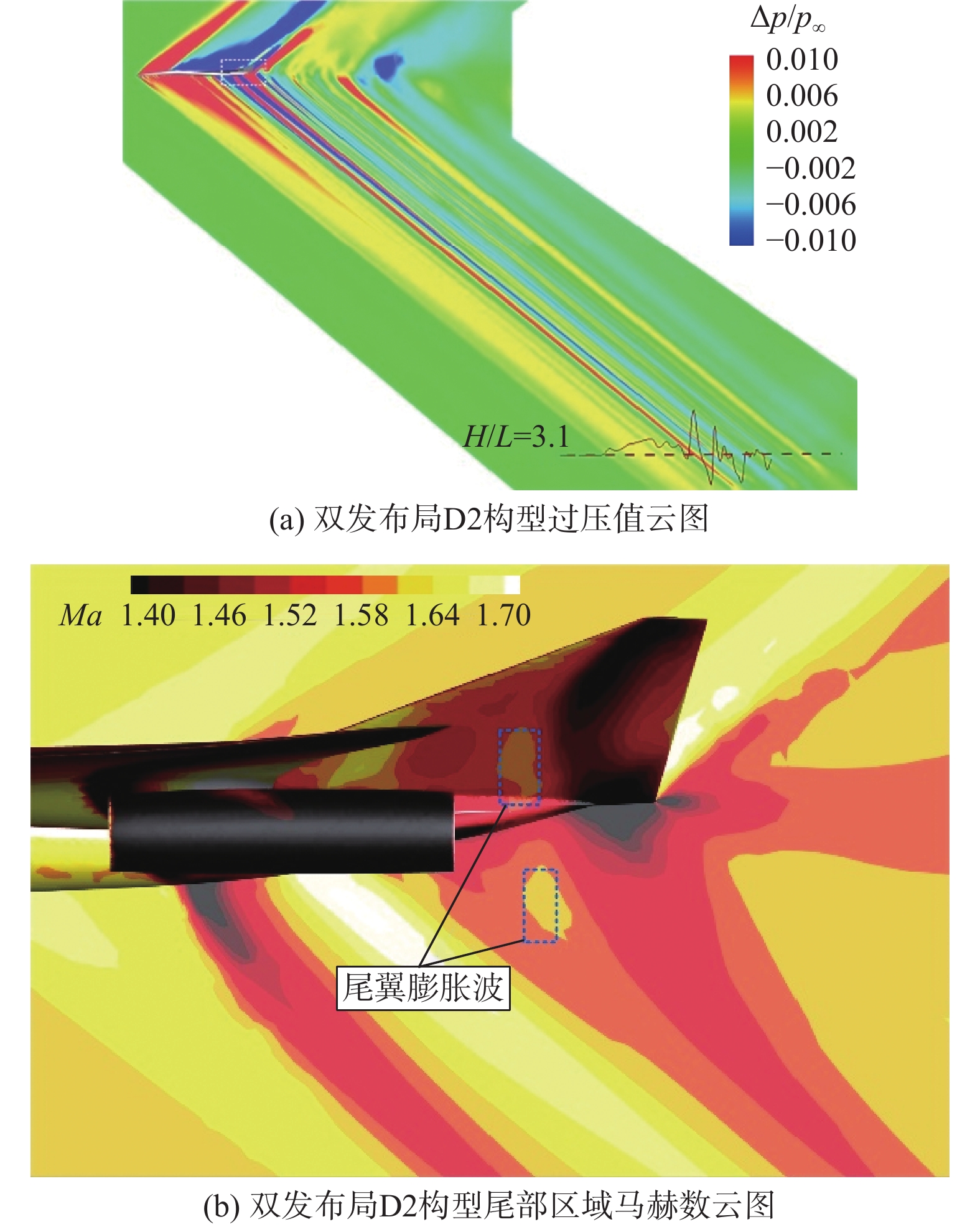
超声速民机较高的飞行速度导致发动机短舱与机翼机身存在强烈的气动干扰，且高速喷流会改变主流的激波系结构，进而影响声爆强度。以超声速民机为研究对象，对不同短舱布局开展数值模拟研究，揭示发动机短舱位置、数量对近场过压信号特性的影响规律。数值模拟运用有限体积法在直角网格上求解流体控制方程进行，通过伴随自适应网格加密生成高分辨率网格以精确捕捉近场过压信号。结果表明：发动机进气道唇口激波、尾喷口后缘激波及尾喷流与机体机翼引起的激波系存在强烈的相互干扰，一定程度上增加了近场过压幅值，从而增强了声爆强度。短舱沿弦向前移及置于机翼上方可以有效降低声爆强度，沿展向外移通过抑制尾部各激波的合并也可有效降低声爆强度；在相同总推力前提下，相比三发构型，双发构型能有效降低后体激波强度，但较大尺寸的短舱引起较强的进气道唇口激波。综合考虑喷流噪声和气动阻力因素，翼下双发布局对于新一代超声速民机并非最佳选项。
- 超声速民机 /
- 短舱布局 /
- 声爆 /
- 网格自适应 /
- 笛卡儿网格
Abstract:
The strong aerodynamic interaction between the nacelles and the wing-body at supersonic speed, and the effect of the exhaust plume on the mainstream shock wave structure could have a great influence on the sonic boom loudness. This paper carries out a numerical investigation on the effect of nacelle layout on thesonic boom for a supersonic transport configuration where the supersonic flows and near field pressure signatures are computed by using a finite volume solver and an adjoint-based Cartesian adaptive mesh refinement method. Various relative positions of nacelles with respect to the wing andthe different number of nacelles are considered. Results indicate that the shock and expansion waves caused by the wing-body combination are significantly interfered with by the lip shock at the inlet, the nozzle trailing edge shock, and the expansion and aft shock of the nozzle plume. This increases the amplitude of the pressure signatures and subsequently increases the sonic boom loudness.Compared with the baseline, the ground loudness can be effectively suppressed by moving the nacelles forward along the chord, outward along the span-wise, and mounting above the wing. Under the same overall force and with the same number of engines and nacelles, the configuration with twin nacelles can reduce the aft shock, but the larger nacelle results in a stronger lip shock at the intake, increasing the loudness of the sonic boom. Thus, considering the engine noise and aerodynamic drag, it is not recommended to deploy twin nacelles for next-generation supersonic transport.
- supersonic transport /
- nacelle layout /
- sonic boom /
- adaptive mesh /
- Cartesian mesh

HTML全文

图 1 LM1021基础构型及不同发动机短舱布局示意图

Figure 1. Baseline of LM1021 with powered nacelles and configurations with various nacelle layouts

下载: 全尺寸图片幻灯片

图 2 初始网格与边界条件

Figure 2. Initial mesh and boundary conditions

下载: 全尺寸图片幻灯片

图 3 直角网格伴随自适应技术流程

Figure 3. Flow chart for adjoint-based adaptive Cartesian mesh refinement

下载: 全尺寸图片幻灯片

图 4 LM1021模型计算结果

Figure 4. Computational results for LM1021 model

下载: 全尺寸图片幻灯片

图 5 Biconvex几何外形及伴随自适应得到的最终网格

Figure 5. Biconvex geometry and its adaptively refined mesh by adjoint method

下载: 全尺寸图片幻灯片

图 6 Biconvex模型计算结果

Figure 6. Calculation results of biconvex model

下载: 全尺寸图片幻灯片

图 7 有无尾喷流构型近场过压值与马赫数云图

Figure 7. Near-field over pressure and Mach number contours of configurations without and with powered nacelles

下载: 全尺寸图片幻灯片

图 8 有无尾喷流构型在H/L=3.1处过压信号对比

Figure 8. Comparison of pressure signatures at H/L=3.1 between configurations without and with powered nacelles

下载: 全尺寸图片幻灯片

图 9 改变短舱弦向位置的D3−Δx与D3+Δx构型近场过压值与马赫数云图

Figure 9. Near-field over pressure and Mach number contours of D3−Δx and D3+Δx configurations with different chordal nacelle layouts

下载: 全尺寸图片幻灯片

图 10 D3−Δx与D3+Δx构型在H/L=3.1处的过压信号对比

Figure 10. Comparison of pressure signatures at H/L=3.1 between D3−Δx and D3+Δx configurations

下载: 全尺寸图片幻灯片

图 11 改变短舱展向位置的D3−0.5Δy、D3+Δy与D3+2Δy构型近场过压值与马赫数云图

Figure 11. Near-field over pressure and Mach number contours of D3−0.5Δy, D3+Δy and D3+2Δy configurations with different spanwise nacelle layouts

下载: 全尺寸图片幻灯片

图 12 D3−0.5Δy、D3+Δy与D3+2Δy构型在H/L=3.1处的过压信号对比

Figure 12. Comparison of pressure signatures at H/L=3.1 between D3−0.5Δy, D3+Δy and D3+2Δy configurations

下载: 全尺寸图片幻灯片

图 13 翼上式布局U3构型近场过压值与马赫数云图

Figure 13. Near-field over pressure and Mach number contours of U3 configuration with nacelles mounted on upper wing surface

下载: 全尺寸图片幻灯片

图 14 翼上式布局U3构型在H/L=3.1处的过压信号

Figure 14. Pressure signatures at H/L=3.1 of U3 configuration with nacelles mounted on upper wing surface

下载: 全尺寸图片幻灯片

图 15 双发布局D2构型近场过压值与马赫数云图

Figure 15. Near-field over pressure and Mach number contours of D2 configuration with two nacelles

下载: 全尺寸图片幻灯片

图 16 双发布局D2构型在H/L=3.1处的过压信号

Figure 16. Pressure signatures at H/L=3.1 of D2 configuration with two nacelles

下载: 全尺寸图片幻灯片

表 1 不同构型短舱相对基础构型的偏移量

Table 1. Offsets of nacelles from baseline configuration

构型	展向偏移量/d	轴向偏移量/d	纵向偏移量/d
基础构型	0	0	0
D3−Δx	0	−0.7	0
D3+Δx	0	0.7	0
D3−0.5Δy	−0.5	0.12	0
D3+Δy	1	0.5	0
D3+2Δy	2	1	0
U3	0	0	1.54
D2	0	0	0
注：偏移量均为以喷管直径d为基准的相对量。编号含义如下：轴向从机头到机尾方向为正，展向从翼根到翼梢方向为正，采用右手坐标系。D (down)表示短舱置于机翼下方，U (up) 表示短舱置于机翼上方；3或2表示发动机数量；Δx/Δy表示短舱沿弦向/展向移动，其中，Δy等于一个喷管直径；+/−表示发动机沿对应坐标轴正/负向移动。

下载: 导出CSV

表 2 自由来流条件及发动机动力参数

Table 2. Conditions of freestream and propulsion system parameters

参数	数值
来流马赫数	1.6
来流静温/K	211.96
发动机风扇入口静压比	3.2606
发动机风扇入口密度比	14.54
发动机风扇入口马赫数	0.6093
雷诺数	8.1×10⁶
来流静压/Pa	25894.936
发动机尾喷口落压比	14.54
发动机尾喷口总温比	7.8722
发动机尾喷口马赫数	0.6620

下载: 导出CSV

参考文献(37)

[1]	朱自强, 兰世隆. 超声速民机和降低音爆研究[J]. 航空学报, 2015, 36(8): 2507-2528. ZHU Z Q, LAN S L. Study of supersonic commercial transport and reduction of sonic boom[J]. Acta Aeronautica et Astronautica Sinica, 2015, 36(8): 2507-2528(in Chinese).
[2]	钱战森, 韩忠华. 声爆研究的现状与挑战[J]. 空气动力学学报, 2019, 37(4): 601-619. QIAN Z S, HAN Z H. Progress and challenges of sonic boom research[J]. Acta Aerodynamica Sinica, 2019, 37(4): 601-619(in Chinese).
[3]	王迪, 冷岩, 杨龙, 等. 基于广义Burgers方程的声爆传播特性大气湍流影响研究[J]. 航空学报, 2023, 44(2): 626318. WANG D, LENG Y, YANG L, et al. Atmospheric turbulence effects on sonic boom propagation based on augmented Burgers equation[J]. Acta Aeronautica et Astronautica Sinica, 2023, 44(2): 626318(in Chinese).
[4]	王迪, 钱战森, 冷岩. 广义Burgers方程声爆传播模型高阶格式离散研究[J]. 航空学报, 2022, 43(1): 124916. WANG D, QIAN Z S, LENG Y. High-order scheme discretization of the sonic boom propagation model based on augmented Burgers equation[J]. Acta Aeronautica et Astronautica Sinica, 2022, 43(1): 124916(in Chinese).
[5]	冯晓强, 李占科, 宋笔锋. 超声速客机音爆问题初步研究[J]. 飞行力学, 2010, 28(6): 21-23. FENG X Q, LI Z K, SONG B F. Preliminary analysis on the sonic boom of supersonic aircraft[J]. Flight Dynamics, 2010, 28(6): 21-23(in Chinese).
[6]	王刚, 马博平, 雷知锦, 等. 典型标模音爆的数值预测与分析[J]. 航空学报, 2018, 39(1): 169-181. WANG G, MA B P, LEI Z J, et al. Simulation and analysis for sonic boom on several benchmark cases[J]. Acta Aeronautica et Astronautica Sinica, 2018, 39(1): 169-181(in Chinese).
[7]	乔建领, 韩忠华, 丁玉临, 等. 基于非线性Burgers方程的超声速客机远场声爆预测方法[C]//首届中国空气动力学大会, 2018. QIAO J L, HAN Z H, DING Y L, et al. Sonic boom prediction method for supersonic transports based on nonlinear Burgers equation[C]//The First Chinese Conference of Aerodynamics, 2018(in Chinese).
[8]	乔建领, 韩忠华, 丁玉临, 等. 基于广义Burgers方程的超声速客机远场声爆高精度预测方法[J]. 空气动力学学报, 2019, 37(4): 663-674. QIAO J L, HAN Z H, DING Y L, et al. Sonic boom prediction method for supersonic transports based on augmented Burgers equation[J]. Acta Aerodynamica Sinica, 2019, 37(4): 663-674(in Chinese).
[9]	张绎典, 黄江涛, 高正红. 基于增广Burgers方程的音爆远场计算及应用[J]. 航空学报, 2018, 39(7): 122039. ZHANG Y D, HUANG J T, GAO Z H. Far field simulation and applications of sonic boom based on augmented Burgers equation[J]. Acta Aeronautica et Astronautica Sinica, 2018, 39(7): 122039(in Chinese).
[10]	陈鹏, 李晓东. 基于Khokhlov-Zabolotskaya-Kuznetsov方程的声爆频域预测法[J]. 航空动力学报, 2010, 25(2): 359-365. CHEN P, LI X D. Frequency domain method for predicting sonic boom propagation based on Khokhlov-Zabolotskaya-Kuznetsov equation[J]. Journal of Aerospace Power, 2010, 25(2): 359-365(in Chinese).
[11]	冯晓强, 宋笔锋, 李占科. 低声爆静音锥设计方法研究[J]. 航空学报, 2013, 34(5): 1009-1017. FENG X Q, SONG B F, LI Z K. Research of low sonic boom quiet spike design method[J]. Acta Aeronautica et Astronautica Sintca, 2013, 34(5): 1009-1017(in Chinese).
[12]	李占科, 刘秧, 丁玉临, 等. 静音锥对超声速民机低声爆效果的影响[J]. 西北工业大学学报, 2019, 37(1): 203-210. doi: 10.3969/j.issn.1000-2758.2019.01.028 LI Z K, LIU Y, DING Y L, et al. Influence of quiet spike on supersonic transport for low boom effect[J]. Journal of Northwestern Polytechnical University, 2019, 37(1): 203-210(in Chinese). doi: 10.3969/j.issn.1000-2758.2019.01.028
[13]	冯晓强, 李占科, 宋笔锋. 超声速客机低音爆布局反设计技术研究[J]. 航空学报, 2011, 32(11): 1980-1986. FENG X Q, LI Z K, SONG B F. A research on inverse design method of a lower sonic boom supersonic aircraft configuration[J]. Acta Aeronautica et Astronautica Sinica, 2011, 32(11): 1980-1986(in Chinese).
[14]	韩忠华, 许晨舟, 乔建领, 等. 基于代理模型的高效全局气动优化设计方法研究进展[J]. 航空学报, 2020, 41(5): 25-65. HAN Z H, XU C Z, QIAO J L, et al. Recent progress of efficient global aerodynamic shape optimization using surrogate-based approach[J]. Acta Aeronautica et Astronautica Sinica, 2020, 41(5): 25-65(in Chinese).
[15]	韩忠华. Kriging模型及代理优化算法研究进展[J]. 航空学报, 2016, 37(11): 3197-3225. HAN Z H. Kriging surrogate model and its application to design optimization: A review of recent progress[J]. Acta Aeronautica et Astronautica Sinica, 2016, 37(11): 3197-3225(in Chinese).
[16]	乔建领, 韩忠华, 宋文萍. 基于代理模型的高效全局低音爆优化设计方法[J]. 航空学报, 2018, 39(5): 121736. QIAO J L, HAN Z H, SONG W P. An efficient surrogate-based global optimization for low sonic boom design[J]. Acta Aeronautica et Astronautica Sinica, 2018, 39(5): 121736(in Chinese).
[17]	黄江涛, 张绎典, 高正红, 等. 基于流场/声爆耦合伴随方程的超声速公务机声爆优化[J]. 航空学报, 2019, 40(5): 122505. HUANG J T, ZHANG Y D, GAO Z H, et al. Sonic boom optimization of supersonic jet based on flow/sonic boom coupled adjoint equations[J]. Acta Aeronautica et Astronautica Sinica, 2019, 40(5): 122505(in Chinese).
[18]	CAPONE F J, PUTNAM L E. Experimental determination of equivalent solid bodies to represent jets exhausting into a Mach 2.20 external stream: NASA-TN-D-5553[R]. Washington, D. C. : NASA, 1969.
[19]	BUI T. CFD analysis of the nozzle jet plume effects on sonic boom signature: AIAA 2009-1054[R]. Reston: AIAA, 2009.
[20]	CASTNER R. Analysis of plume effects on sonic boom signature for isolated nozzle configurations: AIAA 2008-3729[R]. Reston: AIAA, 2008.
[21]	CASTNER R. Analysis of exhaust plume effects on sonic boom for a 59-degree wing body model: AIAA 2011-917[R]. Reston: AIAA, 2011.
[22]	HOWE D. Engine placement for sonic boom mitigation: AIAA-2002-0148[R]. Reston: AIAA, 2002.
[23]	CASTNER R, LAKE T. Exhaust plume effects on sonic boom for a delta wing and swept wing-body model: AIAA 2012-1033[R]. Reston: AIAA, 2012.
[24]	冯晓强, 李占科, 宋笔锋, 等. 基于混合网格的声爆/气动一体化设计方法研究[J]. 空气动力学学报, 2014, 32(1): 30-37. doi: 10.7638/kqdlxxb-2012.0071 FENG X Q, LI Z K, SONG B F, et al. Optimization of sonicboom and aerodynamic based on structured/unstructured hybrid grid[J]. Acta Aerodynamica Sinica, 2014, 32(1): 30-37(in Chinese). doi: 10.7638/kqdlxxb-2012.0071
[25]	KIM H, LEE M. Flow simulation of a supersonic airplane with installed engine nacelle[J]. Aerospace Science and Technology, 2021, 117: 106900. doi: 10.1016/j.ast.2021.106900
[26]	CLIFF S E, DURSTON D, CHAN W M, et al. Computational and experimental assessment of models for the first AIAA sonic boom prediction workshop: AIAA 2014-0560[R]. Reston: AIAA, 2014.
[27]	廉筱纯, 吴虎. 航空发动机原理[M]. 西安: 西北工业大学出版社, 2005. LIAN X C, WU H. Principle of aeroengine[M]. Xi’an: Northwestern Polytechnical University Press, 2005(in Chinese).
[28]	ROE P L. Approximate riemann solvers, parameter vectors, and difference schemes[J]. Journal of Computational Physics, 1981, 43(2): 357-372. doi: 10.1016/0021-9991(81)90128-5
[29]	VENKATAKRISHNAN V. Convergence to steady state solutions of the Euler equations on unstructured grids with limiters[J]. Journal of Computational Physics, 1995, 118(1): 120-130. doi: 10.1006/jcph.1995.1084
[30]	吕凡熹, 肖天航, 余雄庆. 基于自适应直角网格的二维全速势方程有限体积解法[J]. 计算力学学报, 2016, 33(3): 424-430. LYU F X, XIAO T H, YU X Q. A finite volume method for 2D full-potential equation on adaptive Cartesian grids[J]. Chinese Journal of Computational Mechanics, 2016, 33(3): 424-430(in Chinese).
[31]	吕凡熹, 李正洲, 邓经枢, 等. 面向飞行器概念设计的全速域气动分析工具[J]. 空气动力学学报, 2017, 35(5): 625-632. LYU F X, LI Z Z, DENG J S, et al. An aerodynamic analysis tool for aircraft conceptual design at full speed range[J]. Acta Aerodynamica Sinica, 2017, 35(5): 625-632(in Chinese).
[32]	WARREN G, ANDERSON W, THOMAS J, et al. Grid convergence for adaptive methods: AIAA-91-1592[R]. Reston: AIAA, 1991.
[33]	李立, 白文, 梁益华. 基于伴随方程方法的非结构网格自适应技术及应用[J]. 空气动力学学报, 2011, 29(3): 309-316. LI L, BAI W, LIANG Y H. An adjoint-based method for unstructured mesh adaptation and its applications[J]. Acta Aerodynamica Sinica, 2011, 29(3): 309-316(in Chinese).
[34]	朱震浩, 肖天航, 徐雅楠, 等. 基于直角网格伴随自适应的声爆预测[J/OL]. 北京航空航天大学学报, 2022(2022-01-25) [2022-04-01]. https://link.cnki.net/urlid/11.2625.V.20220124.1709.004. ZHU Z H, XIAO T H, XU Y N, et al. Adjoint-based adaptive cartesian mesh refinement for sonic boom prediction[J/OL]. Journal of Beijing University of Aeronautics and Astronautics, 2022(2022-01-25) [2022-04-01]. https://link.cnki.net/urlid/11.2625.V.20220124.1709.004(in Chinese).
[35]	PARK M A, MORGENSTERN J M. Summary and statistical analysis of the first AIAA sonic boom prediction workshop[J]. Journal of Aircraft, 2016, 53(2): 578-598. doi: 10.2514/1.C033449
[36]	WINSKI C S, CARTER M B, ELMILIGUI A A, et al. Computational and experimental study of plume and shock interaction effects on sonic boom in the NASA Ames 9×7 supersonic wind tunnel: AIAA 2018-0331[R]. Reston: AIAA, 2018.
[37]	PARK M A, NEMEC M. Nearfield summary and statistical analysis of the second AIAA sonic boom prediction workshop[J]. Journal of Aircraft, 2019, 56(3): 851-875. doi: 10.2514/1.C034866