-
摘要:
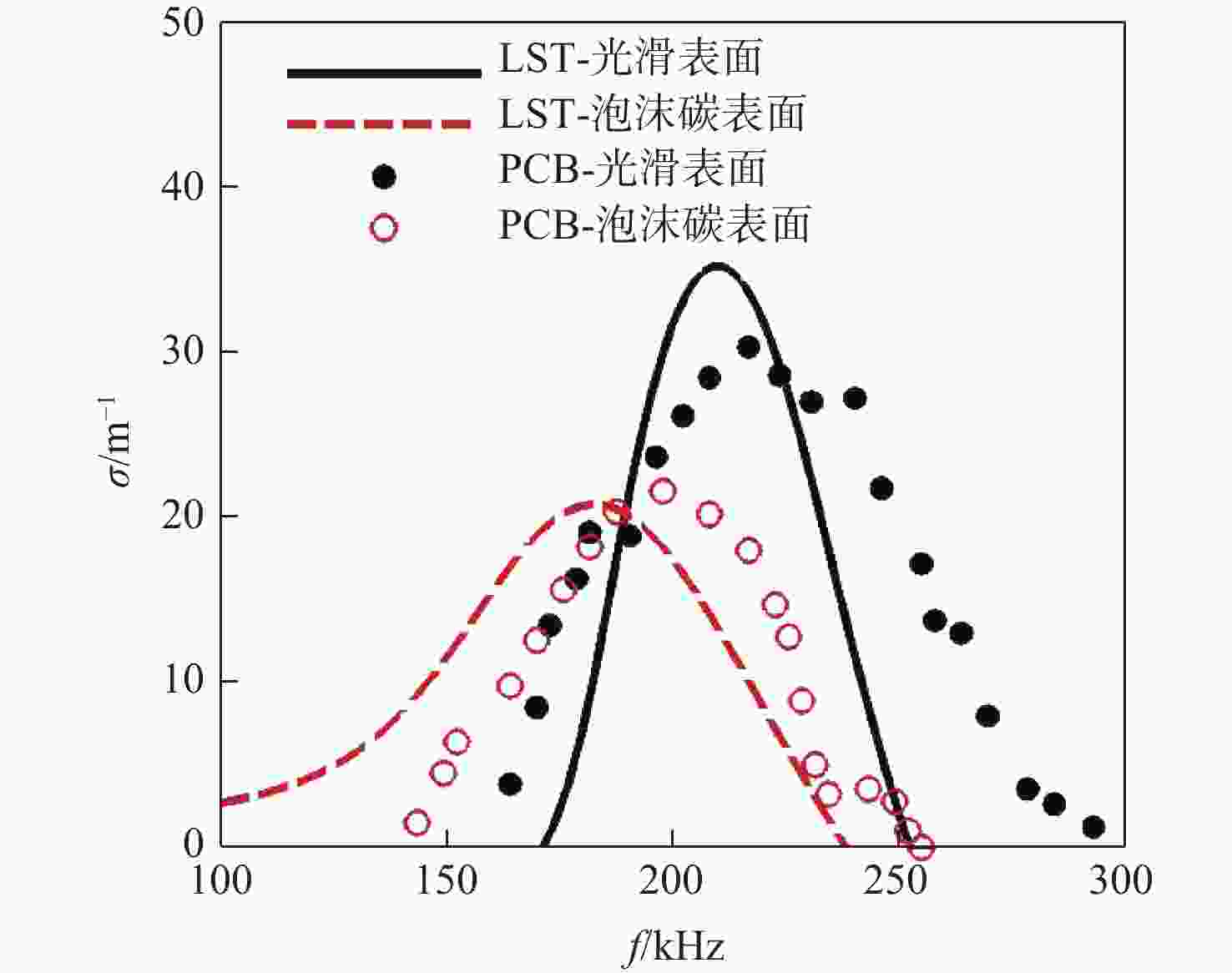
高超声速边界层转捩会使壁面摩阻和热流显著增加，严重影响飞行器的性能。微孔隙表面在不明显改变平均流场的同时，能够有效抑制边界层转捩，具有较大的应用潜力。在马赫数为6 的Ludwieg 管风洞中研究泡沫碳孔隙材料对尖锥边界层中不稳定波的影响规律，试验结果表明：尖锥边界层存在明显的第2模态波，其特征频率随着流向位置增加而减小。相比于光滑表面，泡沫碳表面使不同流向位置上的第2模态波增长率均有明显下降，至少延长第2模态传播区域21.6%。此外，采用阻抗管测量泡沫碳表面的声学特性获取阻抗模型系数，并结合线性稳定性理论预测了泡沫碳表面扰动模态增长率，理论结果与试验结果变化趋势相同。
Abstract:Hypersonic boundary-layer transition generates a significant increase in skin friction and heat flux, which leads to severe restrictions on the performance of hypersonic vehicles. Micropore surfaces have a significant deal of potential for application since they can successfully prevent boundary layer transition without clearly altering the average flow field.The effects of carbon foam material on unstable modes in hypersonic boundary-layer are studied in Mach 6 Ludwieg wind tunnel, the experimental results indicate that there exists an obvious second mode wave in the boundary layer, and its characteristic frequency decreases downstream. The carbon foam surface, when compared to a smooth surface, at various streamwise positions retards the formation of the second mode wave and increases the second mode propagation area by at least 21.6%. In addition, the impedance tube is used to measure the acoustic characteristics of the carbon foam surface to obtain impedance model coefficients. The linear stability theory is used to predict the growth rate of disturbance mode on the carbon foam surface, and the theoretical results have the same trend as the experimental results.
-
图 2 Ludwieg管风洞的归一化压力波动
Figure 2. Normalized pressure fluctuations in Ludwieg wind tunnel
图 8 不同尖锥表面沿流向的功率谱密度
Figure 8. Power spectral density along flow direction of different sharp cone surfaces
图 10 尖锥光滑与泡沫碳表面的增长率
Figure 10. Growth rate between sharp cone smooth surface and foamed carbon surface
-
[1] FEDOROV A. Transition and stability of high-speed boundary layers[J]. Annual Review of Fluid Mechanics, 2011, 43: 79-95. doi: 10.1146/annurev-fluid-122109-160750 [2] 李锋, 解少飞, 毕志献, 等. 超高速飞行器中若干气动难题的实验研究[J]. 现代防御技术, 2014, 42 (5): 1-7.LI F, XIE S F, BI Z X, et al. Experimental study of several on aerodynamic problems on hypersonic vehicles[J]. Moderm Defence Technology, 2014, 42 (5): 1-7(in Chinese). [3] 陈坚强, 涂国华, 张毅锋, 等. 高超声速边界层转捩研究现状与发展趋势[J]. 空气动力学学报, 2017, 35(3): 311-337.CHEN J Q, TU G H, ZHANG Y F, et al. Hypersnonic boundary layer transition: What we know, where shall we go[J]. Acta Aerodynamica Sinica, 2017, 35(3): 311-337(in Chinese). [4] SCHNEIDER S P. Hypersonic laminar-turbulent transition on circular cones and scramjet forebodies[J]. Progress in Aerospace Sciences, 2004, 40(1-2): 1-50. doi: 10.1016/j.paerosci.2003.11.001 [5] WEIHS H, LONGO J, TURNER J. The sharp edge flight experiment SHEFEX II, a mission overview and status[C]//Proceedings of the 15th AIAA International Space Planes and Hypersonic Systems and Technologies Conference. Reston: AIAA, 2008. [6] MACK L M. Boundary-layer linear stability theory. Special course on stability and transition of laminar flow advisory group for aerospace research and development: N84-33757 [R]. Washington, D. C. : NASA, 1984: 23-34. [7] MORKOVIN M V. Transition at hypersonic speeds: NASA-CR-178315[R]. Washington, D. C. : NASA, 1987. [8] KIMMEL R. Aspects of hypersonic boundary layer transition control[C]//Proceedings of the 41st Aerospace Sciences Meeting and Exhibit. Reston: AIAA, 2003. [9] FEDOROV A V, MALMUTH N D, RASHEED A, et al. Stabilization of hypersonic boundary layers by porous coatings[J]. AIAA Journal, 2001, 39(4): 605-610. doi: 10.2514/2.1382 [10] WARTEMANN V, LÜDEKE H, SANDHAM N. Stability analysis of hypersonic boundary layer flow over microporous surfaces[C]// Proceedings of the 16th AIAA/DLR/DGLR International Space Planes and Hypersonic Systems and Technologies Conference. Reston: AIAA, 2009. [11] 涂国华, 陈坚强, 袁先旭, 等. 多孔表面抑制第二模态失稳的最优开孔率和孔半径分析[J]. 空气动力学学报, 2018, 36(2): 273-278.TU G H, CHEN J Q, YUAN X X, et al. Optimal porosity and pore radius of porous surfaces for damping the second-mode instability[J]. Acta Aerodynamica Sinica, 2018, 36(2): 273-278(in Chinese). [12] FEDOROV A, SHIPLYUK A, MASLOV A, et al. Stabilization of a hypersonic boundary layer using an ultrasonically absorptive coating[J]. Journal of Fluid Mechanics, 2003, 479: 99-124. doi: 10.1017/S0022112002003440 [13] TRITARELLI R C, LELE S K, FEDOROV A. Stabilization of a hypersonic boundary layer using a felt-metal porous coating[J]. Journal of Fluid Mechanics, 2015, 769: 729-739. doi: 10.1017/jfm.2015.156 [14] TIAN X, ZHAO R, LONG T, et al. Reverse design of ultrasonic absorptive coating for the stabilization of mack modes[J]. AIAA Journal, 2019, 57(6): 2264-2269. doi: 10.2514/1.J058105 [15] 赵瑞, 严昊, 席柯, 等. 声学超表面抑制第一模态研究[J]. 航空科学技术, 2020, 31(11): 104-112.ZHAO R, YAN H, XI K, et al. Research on acoustic metasurfaces for the suppression of the first mode[J]. Aeronautical Science & Technology, 2020, 31(11): 104-112(in Chinese). [16] 王蔚彰, 孔维萱, 严昊, 等. 声学超表面抑制高速边界层内宽频不稳定模态研究[J]. 北京航空航天大学学报, 2023, 49(2): 388-396.WANG W Z, KONG W X, YAN H, et al. Acoustic metasurfaces for stabilization of broadband unstable modes in high speed boundary layer[J]. Journal of Beijing University of Aeronautics and Astronautics, 2023, 49(2): 388-396(in Chinese). [17] EGOROV I V, FEDOROV A V, SOUDAKOV V G. Receptivity of a hypersonic boundary layer over a flat plate with a porous coating[J]. Journal of Fluid Mechanics, 2008, 601: 165-187. doi: 10.1017/S0022112008000669 [18] WANG X W, ZHONG X L. Numerical Simulations on mode S growth over feltmetal and regular porous coatings of a Mach 5.92 flow[C]//Proceedings of the 49th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition. Reston: AIAA, 2011. [19] WANG X W, ZHONG X L. The stabilization of a hypersonic boundary layer using local sections of porous coating[J]. Physics of Fluids, 2012, 24(3): 034105. doi: 10.1063/1.3694808 [20] ZHAO R, LIU T, WEN C Y, et al. Theoretical modeling and optimization of porous coating for hypersonic laminar flow control[J]. AIAA Journal, 2018, 56(8): 2942-2946. doi: 10.2514/1.J057272 [21] ZHAO R, ZHANG X X, WEN C Y. Theoretical modeling of porous coatings with simple microstructures for hypersonic boundary-layer stabilization[J]. AIAA Journal, 2020, 58(2): 981-986. doi: 10.2514/1.J058403 [22] ZHAO R, WEN C Y, LONG T H, et al. Spatial direct numerical simulation of the hypersonic boundary-layer stabilization using porous coatings[J]. AIAA Journal, 2019, 57(11): 5061-5065. doi: 10.2514/1.J058467 [23] RASHEED A, HORNUNG H G, FEDOROV A V, et al. Experiments on passive hypervelocity boundary-layer control using an ultrasonically absorptive surface[J]. AIAA Journal, 2002, 40: 481-489. doi: 10.2514/2.1671 [24] CHOKANI N, BOUNTIN D A, SHIPLYUK A N, et al. Nonlinear aspects of hypersonic boundary-layer stability on a porous surface[J]. AIAA Journal, 2005, 43(1): 149-155. doi: 10.2514/1.9547 [25] MASLOV A, SHIPLYUK A, SIDORENKO A, et al. Hypersonic laminar flow control using a porous coating of random microstructure[C]//Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit. Reston: AIAA, 2006. [26] WAGNER A, KUHN M, SCHRAMM J M, et al. Experiments on passive hypersonic boundary layer control using ultrasonically absorptive carbon-carbon material with random microstructure[J]. Experiments in Fluids, 2013, 54(10): 1606. doi: 10.1007/s00348-013-1606-3 [27] LUKASHEVICH S V, MOROZOV S O, SHIPLYUK A N. Experimental study of the effect of a passive porous coating on disturbances in a hypersonic boundary layer 2. Effect of the porous coating location[J]. Journal of Applied Mechanics and Technical Physics, 2016, 57(5): 873-878. doi: 10.1134/S002189441605014X [28] ZHU W K, SHI M T, ZHU Y D, et al. Experimental study of hypersonic boundary layer transition on a permeable wall of a flared cone[J]. Physics of Fluids, 2020, 32(1): 011701. doi: 10.1063/1.5139546 [29] ZHU W K, CHEN X, ZHU Y D, et al. Nonlinear interactions in the hypersonic boundary layer on the permeable wall[J]. Physics of Fluids, 2020, 32(10): 104110. doi: 10.1063/5.0028698 [30] 赵家权, 司马学昊, 黄冉冉, 等. 一种采用双弯管储气段布局的高超声速Ludwieg管设计[J]. 空气动力学学报, 2022, 40(4): 90-100.ZHAO J Q, SIMA X H, HUANG R R, et al. Design of a hypersonic Ludwieg tunnel with a double-bent storage tube[J]. Acta Aerodynamica Sinica, 2022, 40(4): 90-100(in Chinese). [31] LUDWIEG H. The tubular wind tunnel[J]. Journal of Flight Sciences, 1955, 3(7): 206-216. [32] MARINEAU E C, MORARU G C, LEWIS D R, et al. Mach 10 boundary layer transition experiments on sharp and blunted cones [C]//Proceedings of the 19th AIAA International Space Planes and Hypersonic Systems and Technologies Conference. Reston: AIAA, 2014. [33] MACK L M. Linear stability theory and the problem of supersonic boundary- layer transition[J]. AIAA Journal, 1975, 13(3): 278-289. doi: 10.2514/3.49693 [34] MALIK M R. Numerical methods for hypersonic boundary layer stability[J]. Journal of Computational Physics, 1990, 86(2): 376-413. doi: 10.1016/0021-9991(90)90106-B [35] 全国声学标准化技术委员会. 声学阻抗管中吸声系数和声阻抗的测量 第2部分: 传递函数法: GB/T 18696.2-2002[S]. 北京: 国家质量监督检验检疫总局, 2002.The National Technical Committee for Acoustics Standardization. Acoustics-measurement of sound absorption coefficient and impedance in impedance tubes-Part 2: Transfer-function method: GB/T 18696.2-2002 [S]. Beijing: General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China, 2002 (in Chinese). [36] WAGNER A. Passive hypersonic boundary layer transition control using ultrasonically absorptive carbon−carbon ceramic with random microstructure[D]. Belgium: Katholieke Universiteit Leuven, 2014. -
下载:
点击查看大图
计量
- 文章访问数: 74
- HTML全文浏览量: 489
- PDF下载量: 8
- 被引次数: 0
