Joint estimation of DOA and polarization parameters based on uniform circle array with vector sensor
-
摘要:
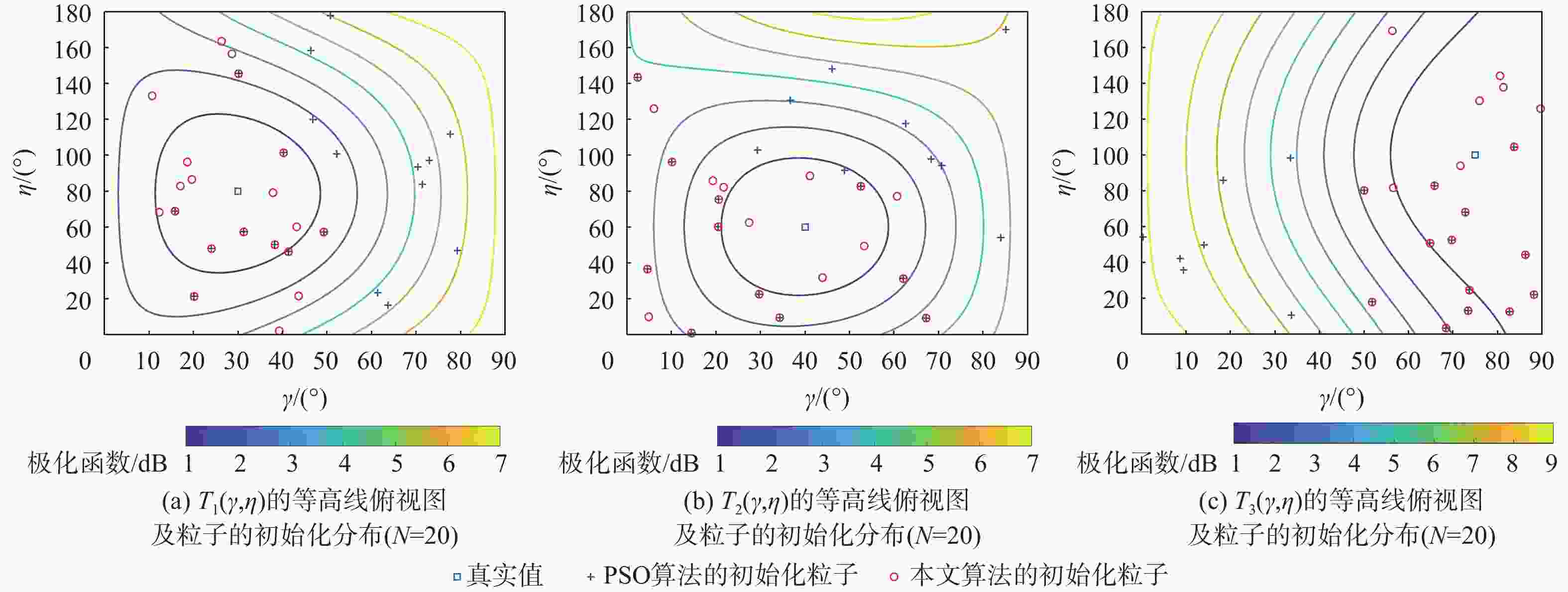
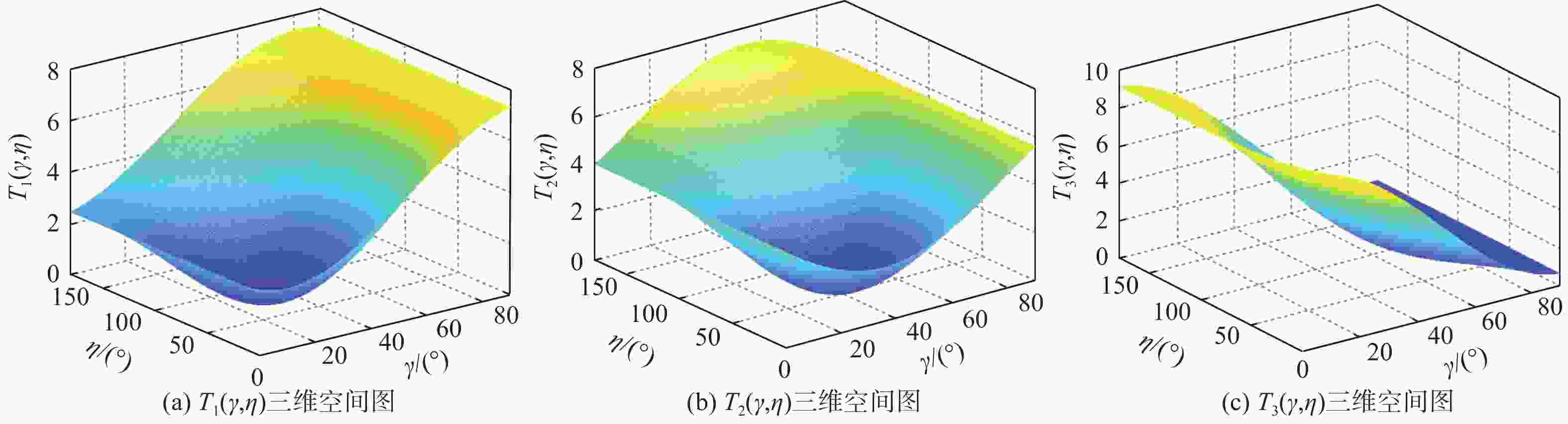
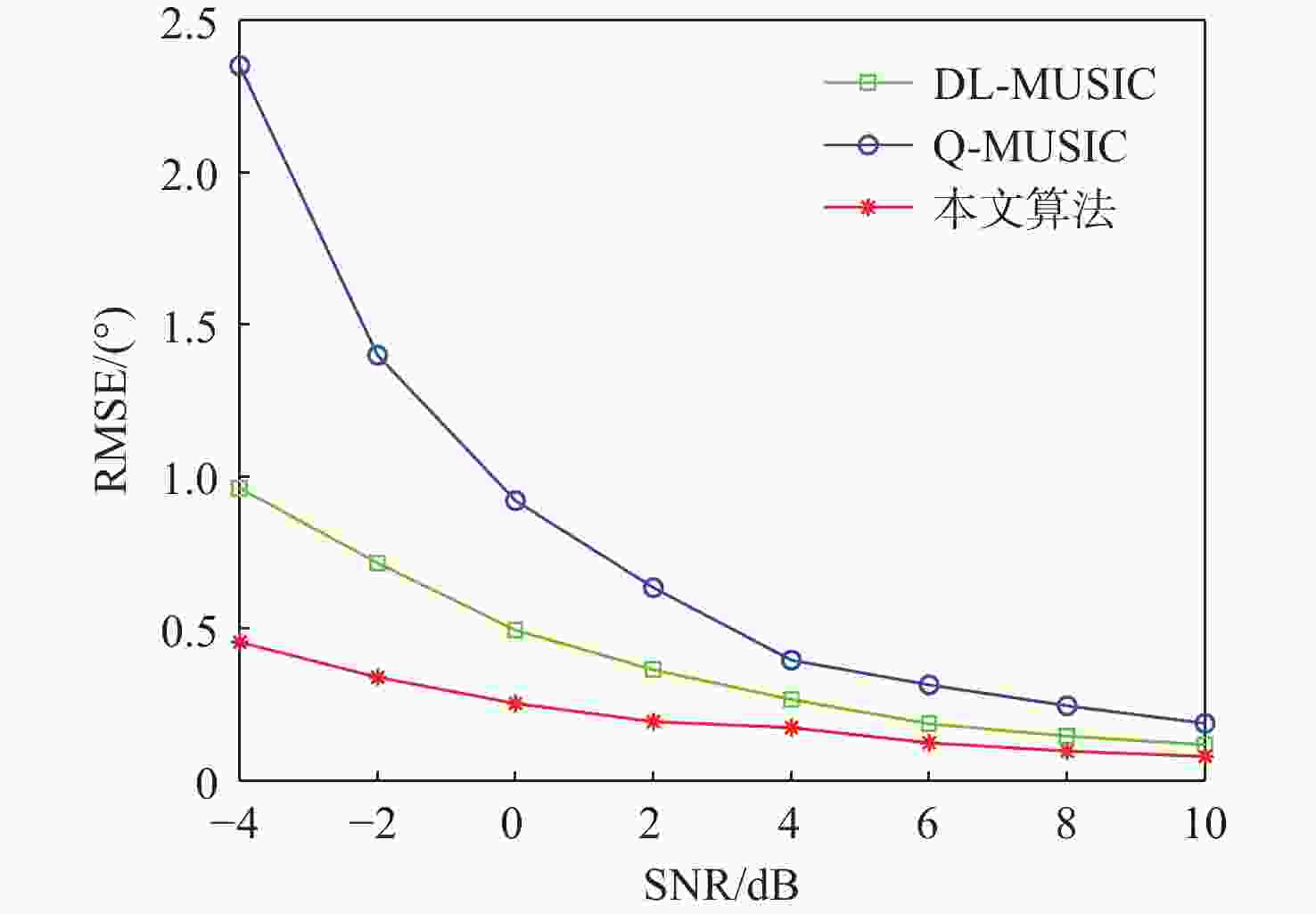
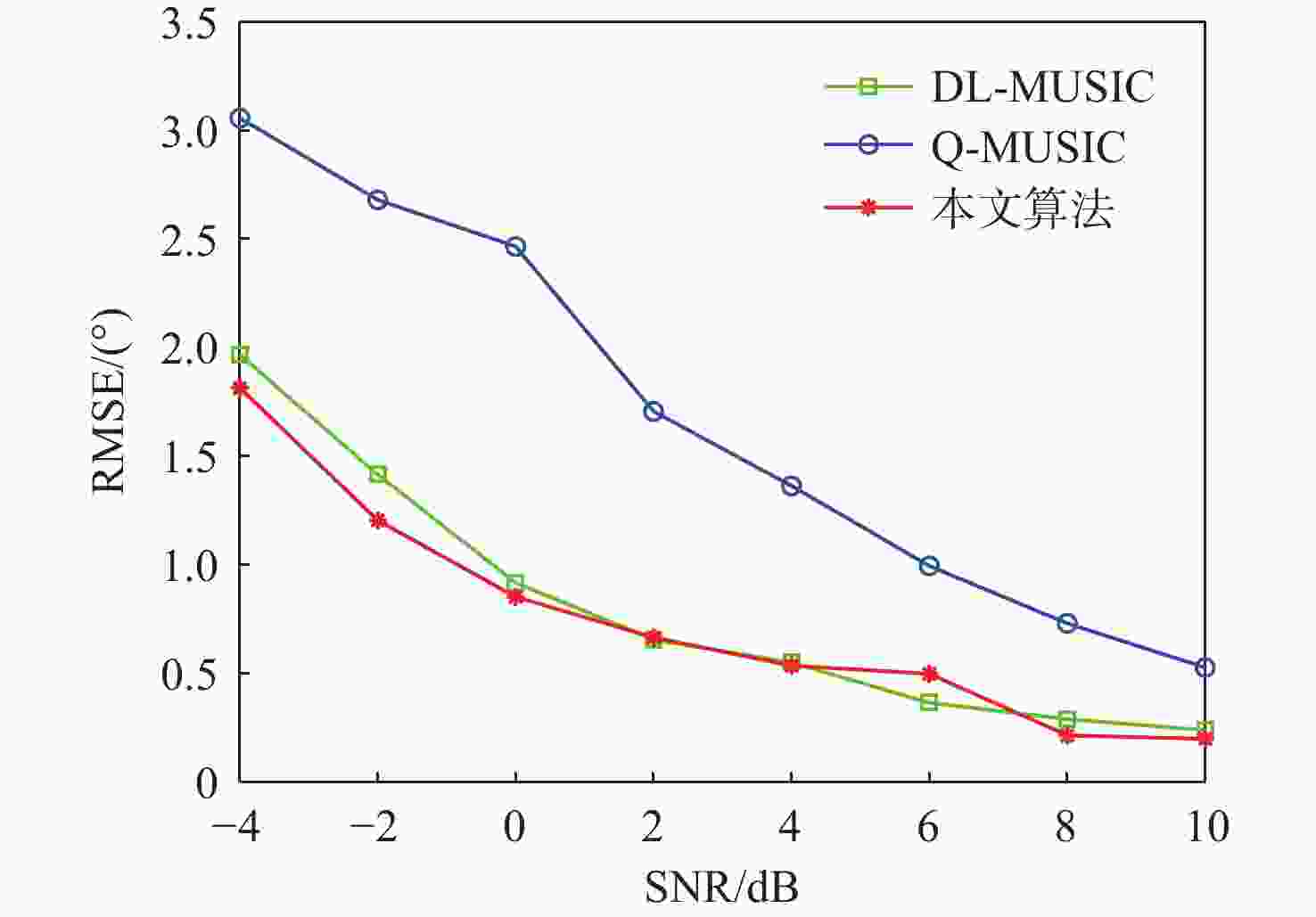
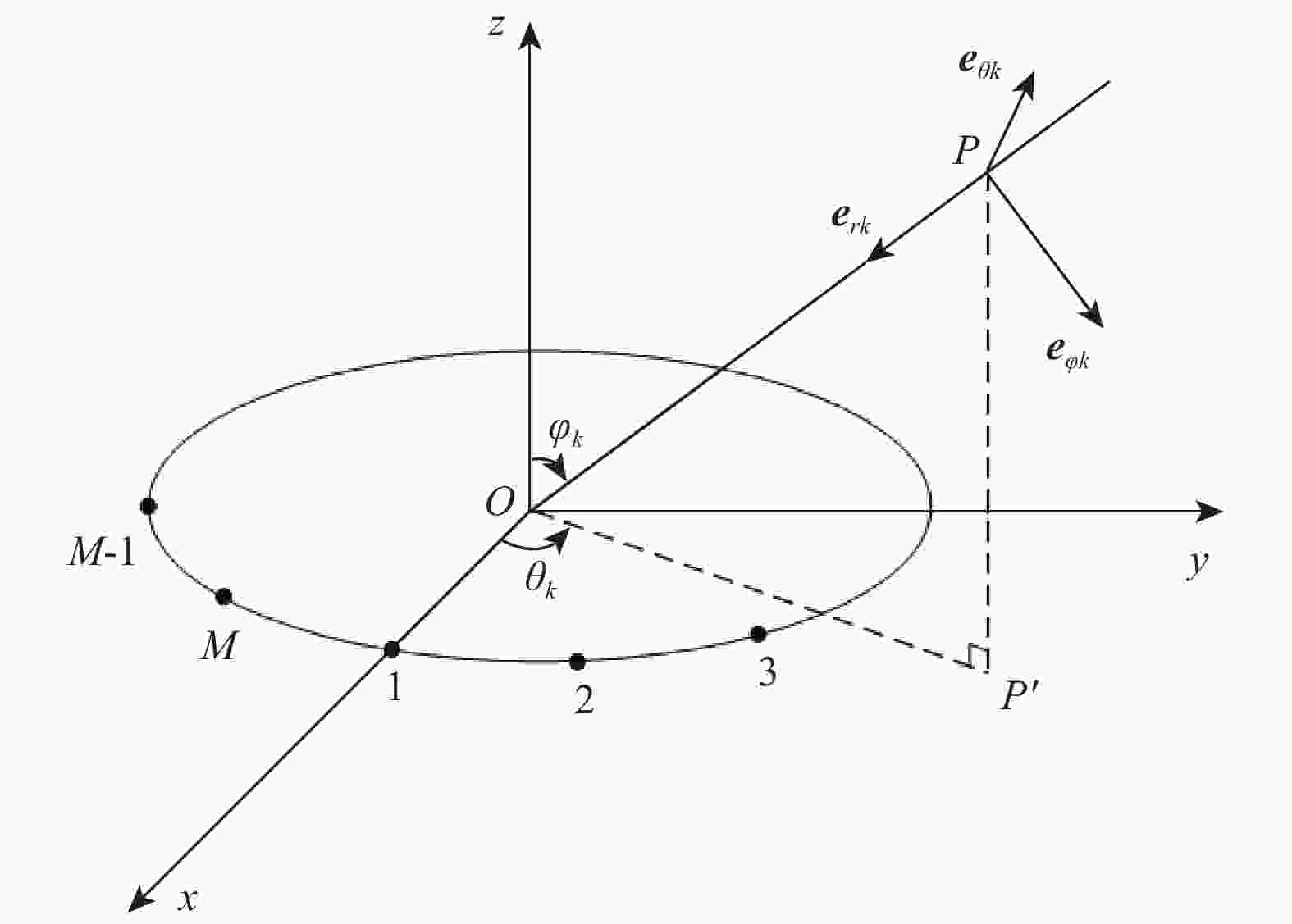
针对极化敏感阵列中非完备电磁矢量传感器多参数联合估计问题，提出一种基于三维电磁矢量传感器平面圆阵模型的波达方向(DOA)和极化参数联合估计方法。利用阵列流型矩阵的特性将四维谱函数进行解耦，严格证明DOA搜索与极化参数估计的不相关性，将四维谱搜索优化为仅与DOA相关的二维谱搜索，提出DOA谱峰搜索二级比较策略，可使雷达自动识别谱峰坐标，有效提高信号处理的实时性；提出一种基于精英反向学习和Lévy飞行的改进粒子群算法对极化参数进行估计，提高极化参数的收敛性能。通过与同类算法进行仿真对比，结果表明：所提算法在牺牲少量时间的条件下可提高估计精度。
Abstract:To address the problem of multi-parameter joint estimation of incomplete electromagnetic vector sensors in the polarization sensitive array, a joint estimation method of Direction of Arrival (DOA) and polarization parameters is proposed based on a circle array model of the three-dimensional electromagnetic vector sensor plane. Using the characteristics of the array flow pattern matrix, a four-dimensional spectral function is decoupled, and the uncorrelation between DOA search and polarization parameter estimation is rigorously proved. A four-dimensional spectral search is optimized to a two-dimensional spectral search only related to DOA. A two-level comparison strategy of the DOA spectral peak search is proposed, which enables the radar to automatically identify the spectral peak coordinates and effectively improve the real-time performance of signal processing. The improved particle swarm optimization algorithm based on elite reverse learning and Lévy flight is proposed to estimate the polarization parameters, which improves the convergence performance of polarization parameters. The simulation results show that the proposed algorithm improves the estimation accuracy at the expense of a small amount of time.
-
图 1 入射波与圆阵中心的示意图
Figure 1. Schematic diagram of incident wave and center of circle array
图 2 电场的正交分解示意图
Figure 2. Schematic diagram of orthogonal decomposition of electric field
图 7 Tk(γ,η)的等高线俯视图及粒子的初始化分布(N=20)
Figure 7. Top view of Tk(γ,η) contour, and initial distribution of particles (N=20)
图 11 极化幅角γ随SNR的RMSE
Figure 11. RMSE of polarization amplitude angle φ with respect to SNR
图 12 极化相位差η随SNR的RMSE
Figure 12. RMSE of polarization phase angle η with respect to SNR
表 1 不同信源数下识别谱峰所需时间
Table 1. Time required to identify spectral peaks with different numbers of sources
s 信源数 所需时间 人为输入 采用DOA二级比较策略 1 约3 2×10 −3 2 约6 7.4×10 −3 3 约9 7.6×10 −3 4 约12 8.2×10 −3 5 约15 18.4×10 −3 
下载: 导出CSV
表 2 不同粒子数的PSO算法和本文算法收敛性能
Table 2. Convergence performance between PSO algorithm and the proposed algorithm with different particle numbers
N Cinteration Ctime/s Cvalue PSO算法 本文算法 PSO算法 本文算法 PSO算法 本文算法 10 25.6 13.4 0.0059 0.0082 0.0076 0.0071 15 17.86 10.66 0.0067 0.0091 0.0066 0.0062 20 16.8 8.66 0.0085 0.0099 0.0054 0.0053 25 15.73 8.46 0.0103 0.0116 0.0054 0.0053 30 13.8 8.53 0.0108 0.0129 0.0054 0.0053
下载: 导出CSV
表 3 不同数据类型进行乘、加运算产生的运算量
Table 3. Amount of computation generated by multiplication and addition of different data types
数据类型 运算类型 运算量/次 实数 复数 四元数 实数 相乘 1（乘法） 相加 1（加法） 复数 相乘 2（乘法） 4（乘法）
2（加法）8（乘法）
4（加法）相加 1（加法） 2（加法） 2（加法） 四元数 相乘 16次（乘法）
12次（加法）相加 4（加法）
下载: 导出CSV
表 4 MUSIC类算法运算性能
Table 4. Computational performance among 4 MUSIC-type algorithms
算法 D $ {\widehat {\boldsymbol{R}}_{\boldsymbol{X}}} $运算量/次 $ (\theta ,\varphi ) $运算量/次 $ (\gamma ,\eta ) $搜索时间/s 总运行时间/s LV-MUSIC 6 1.4746×107（乘法）
7.3728×106（加法）7.2402×1012（乘法）
3.6200×1012（加法）46047.9 DL-MUSIC 2 1.6384×106（乘法）
8.1920×105（加法）4.7369×107（乘法）
2.6984×107（加法）0.0108 1.3044 Q-MUSIC 2 1.6384×106（乘法）
8.1920×105（加法）4.5166×107（乘法）
2.2258×107（加法）0.1604 1.5627 本文算法 3 3.6864×106（乘法）
1.8432×106（加法）1.0672×108（乘法）
5.3363×107（加法）0.0813 2.1682
下载: 导出CSV
-
[1] SCHMIDT R O. Multiple emitter location and signal parameter estimation[J]. IEEE Transactions on Antennas and Propagation, 1986, 34(3): 276-280. doi: 10.1109/TAP.1986.1143830 [2] CHENG Q, HUA Y B. Further study of the pencil-MUSIC algorithm[J]. IEEE Transactions on Aerospace and Electronic Systems, 1996, 32(1): 284-299. [3] LI J, COMPTON R T J. Two-dimensional angle and polarization estimation using the ESPRIT algorithm[J]. IEEE Transactions on Antennas and Propagation, 1992, 40(5): 550-555. [4] ROY R, PAULRAJ A, KAILATH T. ESPRIT: A subspace rotation approach to estimation of parameters of cisoids in noise[J]. IEEE Transactions on Acoustics, Speech and Signal Processing, 1986, 34(5): 1340-1342. [5] MIR H S, SAHR J D. Passive direction finding using airborne vector sensors in the presence of manifold perturbations[J]. IEEE Transactions on Signal Processing, 2007, 55(1): 156-164. doi: 10.1109/TSP.2006.882056 [6] BLISS B, CHAN A, EAPEN A. Vector sensors array design[M]. Lexington: MIT Lincoin Laborary Presentation, 2004: 118-123. [7] FERRARA E, PARKS T. Direction finding with an array of antennas having diverse polarizations[J]. IEEE Transactions on Antennas and Propagation, 1983, 31(2): 231-236. doi: 10.1109/TAP.1983.1143038 [8] HUA Y. A pencil-MUSIC algorithm for finding two-dimensional angles and polarizations using crossed dipoles[J]. IEEE Transactions on Antennas and Propagation, 1993, 41(3): 370-376. [9] CHENG Q, HUA Y B. Performance analysis of the MUSIC and Pencil-MUSIC algorithms for diversely polarized array[J]. IEEE Transactions on Signal Processing, 1994, 42(11): 3150-3165. doi: 10.1109/78.330374 [10] GUO W N, YANG M L, CHEN B X, et al. Joint DOA and polarization estimation using MUSIC method in polarimetric MIMO radar[C]//Proceedings of the IET International Conference on Radar Systems. London: IET Press, 2012. [11] 王群. 基于极化敏感阵列的DOA估计算法研究[D]. 长春: 吉林大学, 2011: 17-23.WANG Q. Study of algorithm on DOA estimation based on polarization sensitive array[D]. Changchun: Jilin University, 2011: 17-23(in Chinese). [12] 李京书, 陶建武. 信号DOA和极化信息联合估计的降维四元数MUSIC方法[J]. 电子与信息学报, 2011, 33(1): 106-111.LI J S, TAO J W. The dimension reduction quaternion MUSIC algorithm for jointly estimating DOA and polarization[J]. Journal of Electronics & Information Technology, 2011, 33(1): 106-111(in Chinese). [13] 司伟建, 朱曈, 张梦莹. 平面极化天线阵列的DOA及极化参数降维估计方法[J]. 通信学报, 2014, 35(12): 28-35.SI W J, ZHU T, ZHANG M Y. Dimension-reduction MUSIC for jointly estimating DOA and polarization using plane polarized arrays[J]. Journal on Communications, 2014, 35(12): 28-35(in Chinese). [14] HU X Y, LYU T T, ZHANG M, et al. MUSIC and improved MUSIC algorithms for parameter estimation using a polarization sensitive array[C]//Proceedings of the 2021 IEEE 21st International Conference on Communication Technology. Piscataway: IEEE Press, 2021: 117-126. [15] 黄志强, 缪晨, 唐辉, 等. 基于联合GA_MUSIC算法的均匀圆阵快速DOA估计[J]. 微波学报, 2021, 37(S1): 166-169.HUANG Z Q, MIAO C, TANG H, et al. Fast DOA estimation of uniform circular array based on joint GA_MUSIC algorithm[J]. Journal of Microwaves, 2021, 37(S1): 166-169 (in Chinese). [16] JIN Y, HOU Y S, JIANG M. Frequency-DOA joint estimation by ant colony optimization[C]//Proceedings of the 2010 International Conference on Computer Application and Systems Modeling. Piscataway: IEEE Press, 2010: 314-319. [17] YANG Y P, HOU Y S, LIU X X. Two dimensional DOA estimation by ant colony optimization[C]//Proceedings of the 2010 International Conference on Intelligent System Design and Engineering Application. Piscataway: IEEE Press, 2010: 789-792. [18] 栾鹏程, 吴瑛. 改进遗传算法在DOA搜索中的应用[J]. 电光与控制, 2006, 13(3): 65-68.LUAN P C, WU Y. Application of improved genetic algorithm in DOA search algorithm[J]. Electronics Optics & Control, 2006, 13(3): 65-68(in Chinese). [19] 王炎. 极化敏感阵列的二维DOA与极化参数估计算法研究[D]. 哈尔滨: 哈尔滨工程大学, 2019: 13-20.WANG Y. Research on the estimation algorithms of two-dimensional DOA and polarization parameters[D]. Harbin: Harbin Engineering University, 2019: 13-20(in Chinese). [20] 张贤达. 信号处理中的线性代数[M]. 北京: 科学出版社, 1997: 27-31.ZHANG X D. Linear algebra in signal processing[M]. Beijing: Science Press, 1997: 27-31(in Chinese). [21] SHI Y H, EBERHART R. A modified particle swarm optimizer[C]//Proceedings of the 1998 IEEE International Conference on Evolutionary Computation. Piscataway: IEEE Press, 1998: 69-73. [22] SHI Y H, EBERHART R C. Empirical study of particle swarm optimization[C]//Proceedings of the 1999 Congress on Evolutionary Computation. Piscataway: IEEE Press, 1999: 1945-1950. [23] 何庆, 黄闽茗, 王旭. 基于精英反向学习的逐维改进蜻蜓算法[J]. 南京师大学报(自然科学版), 2019, 42(3): 65-72.HE Q, HUANG M M, WANG X. Elite opposition learning-based dimension by dimension improved dragonfly algorithm[J]. Journal of Nanjing Normal University(Natural Science Edition), 2019, 42(3): 65-72(in Chinese). [24] 谢承旺, 王志杰, 夏学文. 应用档案精英学习和反向学习的多目标进化算法[J]. 计算机学报, 2017, 40(3): 757-772.XIE C W, WANG Z Z, XIA X W. Multi-objective evolutionary algorithm based on archive-elite learning and opposition-based learning[J]. Chinese Journal of Computers, 2017, 40(3): 757-772(in Chinese). [25] 于建芳, 刘升, 王俊杰, 等. 融合莱维飞行与黄金正弦的蚁狮优化算法[J]. 计算机应用研究, 2020, 37(8): 2349-2353.YU J F, LIU S, WANG J J, et al. Ant lion optimization algorithm integrating with Lévy flight and golden sine[J]. Application Research of Computers, 2020, 37(8): 2349-2353(in Chinese). [26] MIRJALILI S, LEWIS A. The whale optimization algorithm[J]. Advances in Engineering Software, 2016, 95(5): 51-67. -
下载:
点击查看大图
计量
- 文章访问数: 228
- HTML全文浏览量: 101
- PDF下载量: 15
- 被引次数: 0
