Study of tensile properties of laminates containing microvascular channels with different diameters
-
摘要:
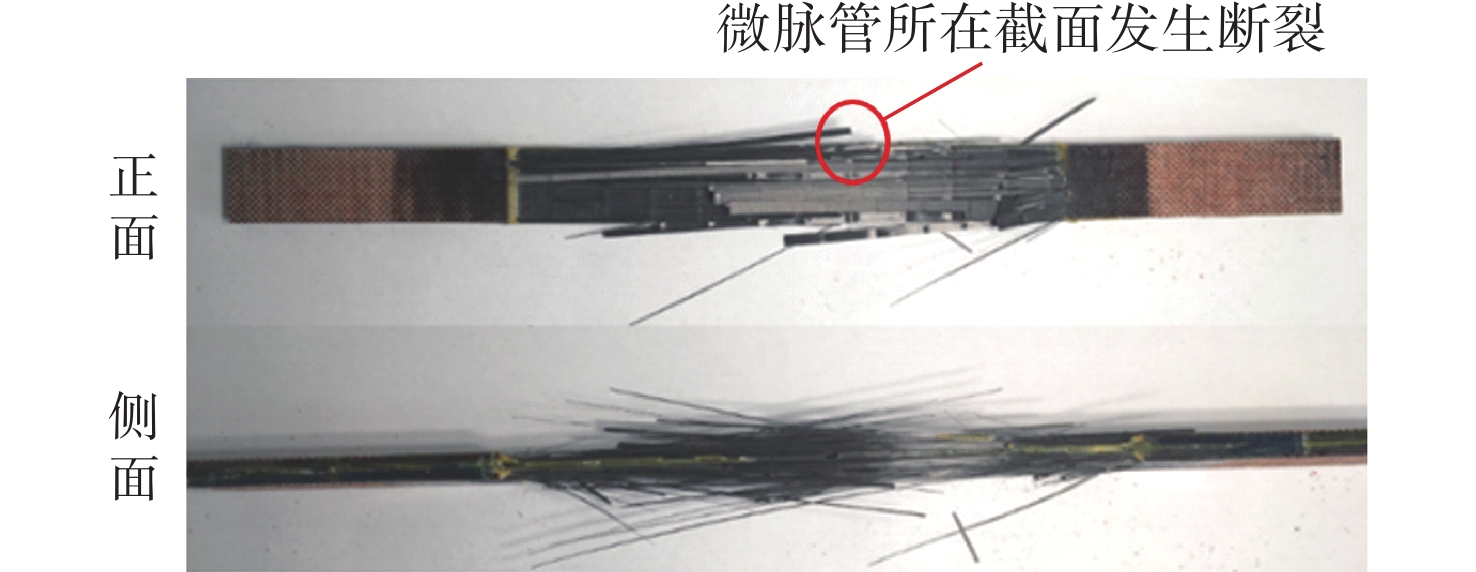
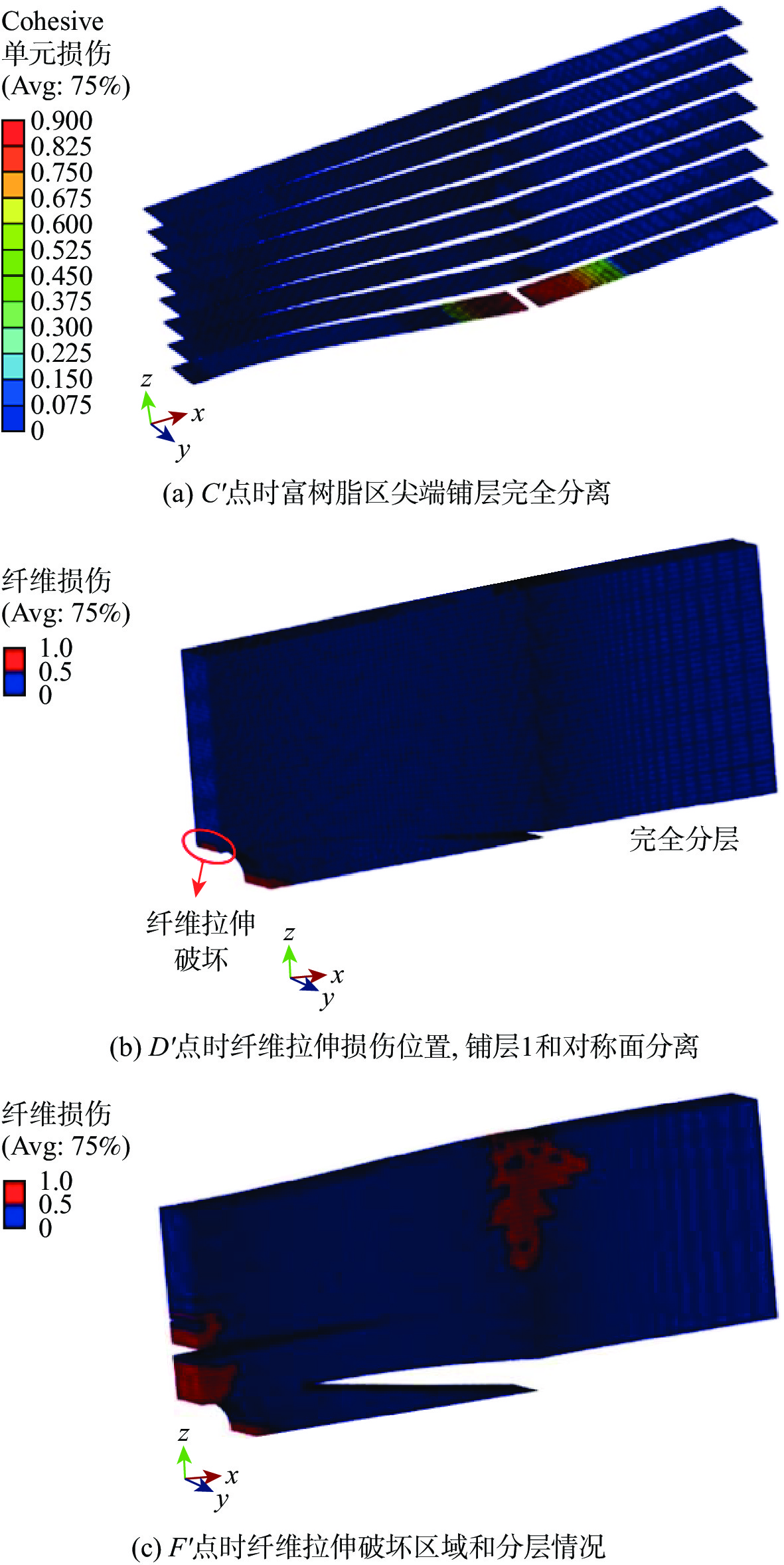
针对微脉管对层合板力学性能的影响，对含不同直径微脉管层合板的拉伸性能进行研究。通过试验测量了含不同直径微脉管层合板的拉伸性能，得到拉伸强度与刚度。建立了含微脉管层合板的精细化有限元模型，综合考虑了微脉管引起的富树脂区、纤维局部弯曲和铺层局部纤维体积分数变化的影响，对含微脉管层合板的拉伸性能进行了分析，并得到了试验结果的验证。在此基础上，分别就微脉管方向和直径对层合板拉伸性能的影响进行了研究。结果表明：当微脉管垂直铺层方向时，使单向层合板纵向拉伸强度降低，但刚度基本不受影响；微脉管直径越大，拉伸强度降低幅度越大；与不含微脉管层合板相比，含直径为0.255 mm和0.4 mm微脉管的层合板拉伸强度分别下降了21.9%和39.9%，但刚度变化不超过1.4%；当微脉管直径变化时，层合板拉伸损伤与破坏过程也发生变化。
Abstract:In view of the influence of microvascular on the mechanical properties of laminates, the tensile properties of laminates with varying diameter microvascular channels were studied. Tensile strength and stiffness of laminates with varying diameter microvascular channels were determined by experimental measurements. A refined finite element model of laminates containing microvascular channels was established, which considered the effects of resin-rich area, fiber bending, and fiber volume fraction change, and the tensile properties of laminates containing microvascular channels were analyzed and the results were verified by the experimental results. On this basis, the effects of microvascular channel orientation and diameter were investigated. The results show that when the microvascular channel is laid in a vertical ply direction, it decreases the longitudinal tensile strength of the unidirectional laminate, but the stiffness is basically unaffected. The larger the diameter of the microvascular channel, the greater the reduction of tensile strength. The structural strength of the laminate containing microvascular channels with diameters of 0.255 mm and 0.4 mm is reduced by 21.9% and 39.9%, respectively, when compared to the tensile properties of the laminate without the channels; however, its stiffness changes less than 1.4%.The damage process of laminate also changes with the microvascular channel diameter.
-
Key words:
- composite laminate /
- self-healing /
- microvascular /
- tensile properties /
- finite element
-
图 1 试验件中微脉管位置示意图
Figure 1. Schematic diagram of microvascular channels’ position in specimen
图 5 微脉管周围富树脂区长度和各铺层厚度测量
Figure 5. Measurement of length of resin-rich zone around microvascular channel and thickness of each layer
图 7 富树脂区上方铺层纤维体积分数分布
Figure 7. Fiber volume fraction distribution in layers above resin-rich zone
图 8 根据纤维体积分数赋予材料后的细化网格模型
Figure 8. Refined mesh model after assigning material according to fiber volume fraction
图 10 含0.255 mm直径微脉管层合板破坏前的应力分布
Figure 10. Stress distribution of laminate with 0.255 mm microvascular channel before damage
图 12 微脉管上方应变位置和无微脉管上方应变位置
Figure 12. Location of strain above microvascular channel and location of strain above non-microvascular channel
图 13 有限元模型微脉管处和无微脉管处应变
Figure 13. Finite element model strains at microvascular channel and non-microvascular channel
图 15 第2组试验件端部位移0.0075 mm时层合板表层纵向应变${\varepsilon _{11}}$分布
Figure 15. Laminate surface longitudinal strain ${\varepsilon _{11}} $ distribution at 0.0075 mm shift of the end of group 2 specimens
图 16 不考虑纤维体积分数变化时有限元载荷-位移曲线
Figure 16. Calculation load-displacement curves without considering change in volume fraction of fibers
图 20 第3组试验件有限元载荷-位移曲线各点对应损伤情况
Figure 20. Damage corresponding to each point of finite element load-displacement curve of specimen in group 3
表 1 试验件参数
Table 1. Parameters of specimen
组别 铺层 尺寸(长×宽×厚)/
(mm×mm×mm)微脉管
直径d/mm微脉管
间距/mm微脉管
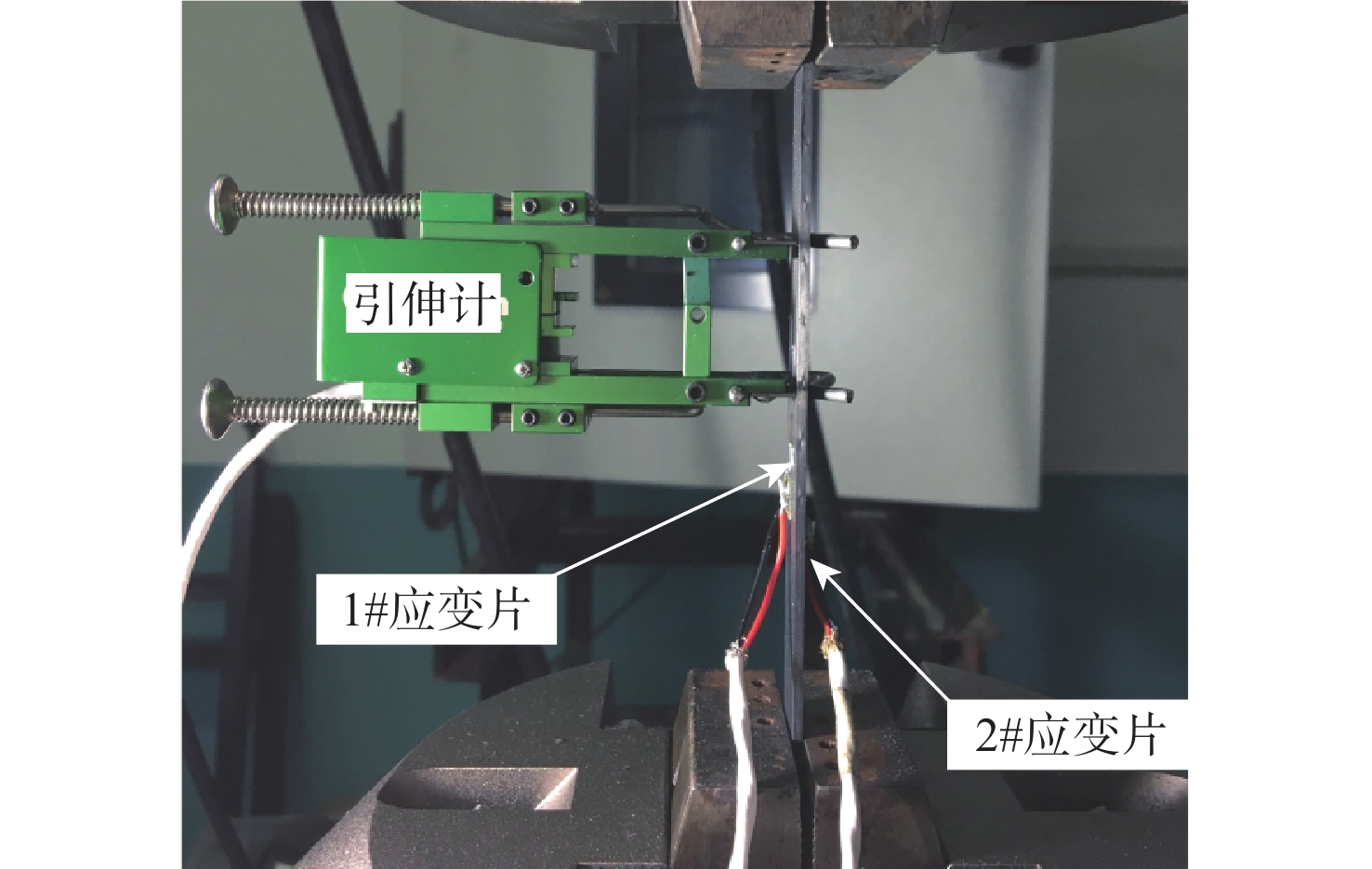
层数数量 1 [016] 250×15×2 0 6 2 [08/(r)/08] 250×15×2 0.255 10 1 5 3 [08/(r)/08] 250×15×2 0.4 10 1 5 4 [908/(r)/908] 175×25×2 0.255 10 1 5 
下载: 导出CSV
表 2 拉伸试验结果对比
Table 2. Comparison of tensile test results
组别 试验件
编号微脉管
直径/
mm1#应变片所测
刚度(微脉
管处)/GPa1#应变片所测
刚度平均值
(微脉管处)/GPa1#应变片所测
刚度离散度
(微脉管处)/%2#应变片所测
刚度(无微脉
管处)/GPa2#应变片所测
刚度平均值
(无微脉管处)/GPa2#应变片所测
刚度离散度
(无微脉管处)/%1 无管 2 1# 0.255 146.6 147.2 0.4 138.4 139.7 0.8 2# 0.255 147.8 140.6 3# 0.255 147.1 140.1 3 1# 0.4 151.8 151.8 0.2 139.7 139.6 0.1 2# 0.4 152.1 139.6 3# 0.4 151.6 139.5 4 1# 0.255 7.75 7.73 0.37 8.02 8.00 1.00 2# 0.255 7.68 7.90 3# 0.255 7.75 8.12 4# 0.255 7.73 8.00 5# 0.255 7.73 7.97
下载: 导出CSV
组别 试验件
编号引伸计所测
刚度/GPa引伸计所测刚度
平均值/GPa引伸计所测刚度
平均下降比例/%引伸计所测刚度
离散度/%拉伸强度/
MPa拉伸强度
平均值/MPa拉伸强度平均
下降比例/%拉伸强度
离散度/%1 无管 140.3 1.5 2 372.0 2.65 2 1# 138.9 139.3 0.7 0.7 1 902.27 1 852.09 21.9 3.0 2# 140.5 1 793.35 3# 138.6 1 860.65 3 1# 135.0 138.3 1.4 2.1 1 454.54 1 424.59 39.9 2.2 2# 140.5 1 391.91 3# 139.5 1 427.32 4 1# 48.08 48.05 3.53 2# 47.99 3# 45.52 4# 48.40 5# 50.28
下载: 导出CSV
$E_{11}^{\text{f}}$/GPa $E_{22}^{\text{f}}$/GPa $G_{12}^{\text{f}}$/GPa $G_{23}^{\text{f}}$/GPa $\mu _{12}^{\text{f}}$ $\mu _{23}^{\text{f}}$ $E_{}^{\text{m}}$/GPa $G_{}^{\text{m}}$/GPa ${\mu ^{\text{m}}}$ 230.0 15.0 24.0 5.0 0.3 0.49 3.45 1.28 0.35
下载: 导出CSV
表 4 单向预浸带性能Chamis模型计算值和实际测量值对比
Table 4. Comparison of Chamis model calculation results and actual measured performance of unidirectional prepreg tape
测试方法 $E_{11}^{}$/GPa $E_{11}^{}$误差/％ $E_{22}^{}$/GPa $E_{22}^{}$误差/％ $\mu _{12}^{}$ $\mu _{12}^{}$误差/％ G12/GPa G12误差/％ G23/GPa G23误差/％ Chamis模型计算值 139 0.93 8.55 6.88 0.32 7.78 3.8 5.94 3.02 2.58 实际测量值 140.3 8.00 0.347 4.04 3.1
下载: 导出CSV
失效模式 失效判据 纤维拉伸失效
($ {\sigma _{11}} \geqslant 0 $)$ {\left(\dfrac{{\sigma }_{11}}{{X}_{\text{T}}}\right)}^{2}+{\left(\dfrac{{\tau }_{12}^{}}{{S}_{12}^{}}\right)}^{2}\geqslant 1 $ 纤维压缩失效
($ {\sigma _{11}} < 0 $)$ {\left(\dfrac{{\sigma }_{11}}{{X}_{\text{C}}}\right)}^{2}\geqslant 1 $ 基体拉伸失效
($ {\sigma _{22}} \geqslant 0 $)$ {\left( {\dfrac{{{\sigma _{22}}}}{{Y_{\text{T}}^{}}}} \right)^2} + {\left( {\dfrac{{\tau _{12}^{}}}{{S_{12}^{}}}} \right)^2} \geqslant 1 $ 基体压缩失效
($ {\sigma _{22}} < 0 $)$ {\left( {\dfrac{{{\sigma _{22}}}}{{2S_{13}^{}}}} \right)^2} + \left[ {{{\left( {\dfrac{{{Y_{\text{C}}}}}{{2{S_{23}}}}} \right)}^2} - 1} \right]\dfrac{{{\sigma _{22}}}}{{{Y_{\text{C}}}}} + {\left( {\dfrac{{\tau _{12}^{}}}{{S_{12}^{}}}} \right)^2} \geqslant 1 $
下载: 导出CSV
表 6 不同失效模式下材料刚度退化系数
Table 6. Degradation factor of material stiffness for different failure modes
失效模式 退化系数 纤维拉伸失效 $ {E_{11}} = 0.07{E_{11}},{G_{12}} = 0.07{G_{12}},{\mu _{12}} = 0.07{\mu _{12}} $ 纤维压缩失效 $ {E_{11}} = 0.14{E_{11}},{G_{12}} = 0.14{G_{12}},{\mu _{12}} = 0.14{\mu _{12}} $ 基体拉伸失效 ${E_{22}} = 0.2{E_{22}},{G_{12}} = 0.2{G_{12}},{G_{23}} = 0.2{G_{23}},$ ${\mu _{12}} = 0.2{\mu _{12}},{\mu _{23}} = 0.2{\mu _{23}}$ 基体压缩失效 $ {E}_{22}=0.4{E}_{22},{G}_{12}=0.4{G}_{12},{G}_{23}=0.4{G}_{23}， $ ${\mu _{12}} = 0.4{\mu _{12}},{\mu _{23}} = 0.4{\mu _{23}}$
下载: 导出CSV
表 7 富树脂区长度和各铺层厚度实际测量尺寸
Table 7. Actual measured length of resin-rich zone and thickness of each layer
mm $d$ $w$ ${h_1}$ ${h_2}$ ${h_3}$ ${h_4}$ ${h_5}$ ${h_6}$ ${h_7}$ ${h_8}$ 0.28 2.543 0.092 0.099 0.105 0.11 0.111 0.113 0.115 0.116
下载: 导出CSV
表 8 T700/LT03A单向预浸带和Cohesive单元参数
Table 8. T700/LT03A unidirectional prepreg tape and Cohesive unit parameters
参数 数值 T700/LT03A
单向预浸带$ {E_{11}}/{\text{GPa}} $ 139 $ {E_{22}}/{\text{GPa}} $ 8.55 $ {G_{12}}/{\text{GPa}} $ 3.8 $ {G_{23}}/{\mathrm{GPa}} $ 3.02 $\mu _{12}^{}$ 0.32 $\mu _{23}^{}$ 0.3 $ {X_{\text{T}}}/{\text{MPa}} $ 2372 $ {X_{\text{C}}}/{\text{MPa}} $ 1234 $ {Y_{\text{T}}}/{\text{MPa}} $ 55.0 $ {Y_{\text{C}}}/{\text{MPa}} $ 178 $ {S_{12}}/{\text{MPa}} $ 125.0 $ {S_{23}}/{\text{MPa}} $ 107 数值Cohesive
单元[16]${K}_{\text{nn}}^{0}/\text{(MPa} $·$ \text{mm}^{{-1}}$) 105 $ {K}_{\text{ss}}^{0}/\text{(MPa} $·$ \text{{mm}}^{{-1}} $) 105 $ {K}_{\text{tt}}^{0}/\text{(MPa} $·$ \text{mm}^{{-1}} $) 105 $t_{\text{n}}^{\text{0}}/{\text{MPa}}$ 55 $t_{\text{s}}^{\text{0}}/{\text{MPa}}$ 80 $t_{\text{t}}^{\text{0}}/{\text{MPa}}$ 80 $ {G}_{\text{C,n}}/$(N·$ \text{m}{\text{m}}^{{-1}} $) 0.276 $ {G}_{\text{C,s}}/ $(N·$ \text{m}{\text{m}}^{{-1}} $) 0.807 $ {G}_{\text{C,t}}/ $(N·$ \text{m}{\text{m}}^{{-1}} $) 0.807 $\eta $ 1.75 注：T700/LT03A单向预浸带参数根据Chamis 模型纤维体积分数为0.6时计算。
下载: 导出CSV
表 9 LT03A树脂力学性能
Table 9. Mechanical properties of LT03A resin
${E^{\text{m}}}/{\text{GPa}}$ ${\mu ^{\text{m}}}$ S/MPa 3.45 0.35 120
下载: 导出CSV
表 10 试验和有限元所得拉伸强度对比
Table 10. Comparison of tensile strength obtained from tests and finite elements
组别 微脉管直径/mm 拉伸强度/MPa 相对误差/% 试验 有限元 2 0.255 1852.09 1803.7 −2.6 3 0.4 1424.59 1430.0 0.38
下载: 导出CSV
表 11 第2组试验件2处刚度有限元和试验结果对比
Table 11. Comparison of finite element and test results of stiffness at two locations for group 2 specimens
位置 刚度/GPa 相对误差/% 有限元 试验 a点微脉管处 156.0 147.25 6.0 b点无微脉管处 138.5 139.7 −0.9
下载: 导出CSV
表 12 不考虑纤维体积分数变化时有限元拉伸强度和原模型及试验结果对比
Table 12. Comparison of calculated tensile strength without considering change in fiber volume fraction with original model and test results
组别 拉伸强度/MPa 相对误差/% 试验 有限元（不考虑纤维体积分数变化） 有限元（考虑纤维体积分数变化） 不考虑纤维体积分数变化 考虑纤维体积分数变化 2 1852.1 1525.8 1603.7 −17.62 −13.41 3 1 424.6 1 269 1 430 −10.92 0.38
下载: 导出CSV
-
[1] 马埸浩, 杜晓渊, 胡仁伟, 等. 微脉管型自修复复合材料研究进展[J]. 高分子材料科学与工程, 2018, 34(1): 166-172.MA Y H, DU X Y, HU R W, et al. Development of self-healing composite materials with microvascular networks[J]. Polymer Materials Science and Engineering, 2018, 34(1): 166-172(in Chinese). [2] 李元杰, 律微波, 孟宪铎. 微胶囊自修复聚合物材料的研究进展[J]. 工程塑料应用, 2005, 33(1): 68-70.LI Y J, LV W B, MENG X D. Research progress in self-repair polymer materials with microcapsule[J]. Engineering Plastics Application, 2005, 33(1): 68-70(in Chinese). [3] 梁大开, 杨红. 采用空心光纤自诊断、自修复智能结构的研究[J]. 压电与声光, 2002, 24(4): 261-263.LIANG D K, YANG H. Research on self-diagnose and self-repair net in smart composite structure by hollow-center optic fiber[J]. Piezoelectrics & Acoustooptics, 2002, 24(4): 261-263(in Chinese). [4] 印明勋, 齐德胜, 云庆文. 航空复合材料自修复研究进展[C]//2015年第二届中国航空科学技术大会. 北京: 中国航空学会, 2015: 185-189.YIN M X, QI D S, YUN Q W. Research development of self-healing aeronautical composite materials[C]//Proceedings of the 2nd China Aviation Science and Technology Conference in 2015. Beijing: Chinese Society of Aeronautics and Astronautics, 2015: 185-189(in Chinese). [5] KOUSOURAKIS A, BANNISTER M K, MOURITZ A P. Tensile and compressive properties of polymer laminates containing internal sensor cavities[J]. Composites Part A:Applied Science and Manufacturing, 2008, 39(9): 1394-1403. doi: 10.1016/j.compositesa.2008.05.003 [6] KOUSOURAKIS A, MOURITZ A P, BANNISTER M K. Interlaminar properties of polymer laminates containing internal sensor cavities[J]. Composite Structures, 2006, 75(1-4): 610-618. doi: 10.1016/j.compstruct.2006.04.086 [7] KOUSOURAKIS A, MOURITZ A P. The effect of self-healing hollow fibres on the mechanical properties of polymer composites[J]. Smart Materials and Structures, 2010, 19(8): 085021. doi: 10.1088/0964-1726/19/8/085021 [8] HUANG C Y, TRASK R S, BOND I P. Characterization and analysis of carbon fibre-reinforced polymer composite laminates with embedded circular vasculature[J]. Journal of the Royal Society Interface, 2010, 7(49): 1229-1241. doi: 10.1098/rsif.2009.0534 [9] HUANG C Y, TRASK R S, BOND I P. Analytical study of vascular networks for self-healing composite laminates[C]//Proceedings of the 17th International Conference on Composite Materials. Edinburgh: ICCM, 2009: 27-31. [10] LUTERBACHER R, TRASK R S, BOND I P. Static and fatigue tensile properties of cross-ply laminates containing vascules for self-healing applications[J]. Smart Materials and Structures, 2016, 25(1): 015003. [11] TRASK R S, BOND I P. Bioinspired engineering study of plantae vascules for self-healing composite structures[J]. Journal of the Royal Society Interface, 2010, 7(47): 921-931. doi: 10.1098/rsif.2009.0420 [12] NGUYEN A T T, ORIFICI A C. Structural assessment of microvascular self-healing laminates using progressive damage finite element analysis[J]. Composites Part A:Applied Science and Manufacturing, 2012, 43(11): 1886-1894. doi: 10.1016/j.compositesa.2012.06.005 [13] AL-SHAWK A, TANABI H, SABUNCUOGLU B. Investigation of stress distributions in the resin rich region and failure behavior in glass fiber composites with microvascular channels under tensile loading[J]. Composite Structures, 2018, 192: 101-114. doi: 10.1016/j.compstruct.2018.02.061 [14] TANABI H, AL-SHAWK A, SABUNCUOGLU B. Stress concentrations in composites with microvascular channels[J]. Procedia Structural Integrity, 2017, 6: 56-63. doi: 10.1016/j.prostr.2017.11.009 [15] ASTM. Standard test method for tensile properties of polymer matrix composite materials: ASTM D3039[S]. West Conshohocken: ASTM, 2000.ASTM. Standard test method for tensile properties of polymer matrix composite materials: ASTM D3039[S]. West Conshohocken: ASTM, 2000. [16] 马埸浩. 含自修复微脉管层合板力学性能研究[D]. 北京: 北京航空航天大学, 2021: 87.MA Y H. Mechanical properties of composite laminates with self-healing microvascular networks[D]. Beijing: Beihang University, 2021: 87(in Chinese). [17] CHAMIS C C. Mechanics of composite materials: past, present, and future[J]. Journal of Composites, Technology and Research, 1989, 11(1): 3-14. doi: 10.1520/CTR10143J [18] YOUNES R, HALLAL A, FARDOUN F, et al. Comparative review study on elastic properties modeling for unidirectional composite materials[M]//HU N. Composites and their properties. London: IntechOpen, 2012, 17: 391-408. [19] HASHIN Z. Failure criteria for unidirectional fiber composite[J]. Journal of Applied Mechanics, 1980, 47(2): 329-334. doi: 10.1115/1.3153664 -
下载:
点击查看大图
计量
- 文章访问数: 171
- HTML全文浏览量: 142
- PDF下载量: 6
- 被引次数: 0
