Meshing theory and performance analysis of point-contact conjugate involute worm gear pair
-
摘要:
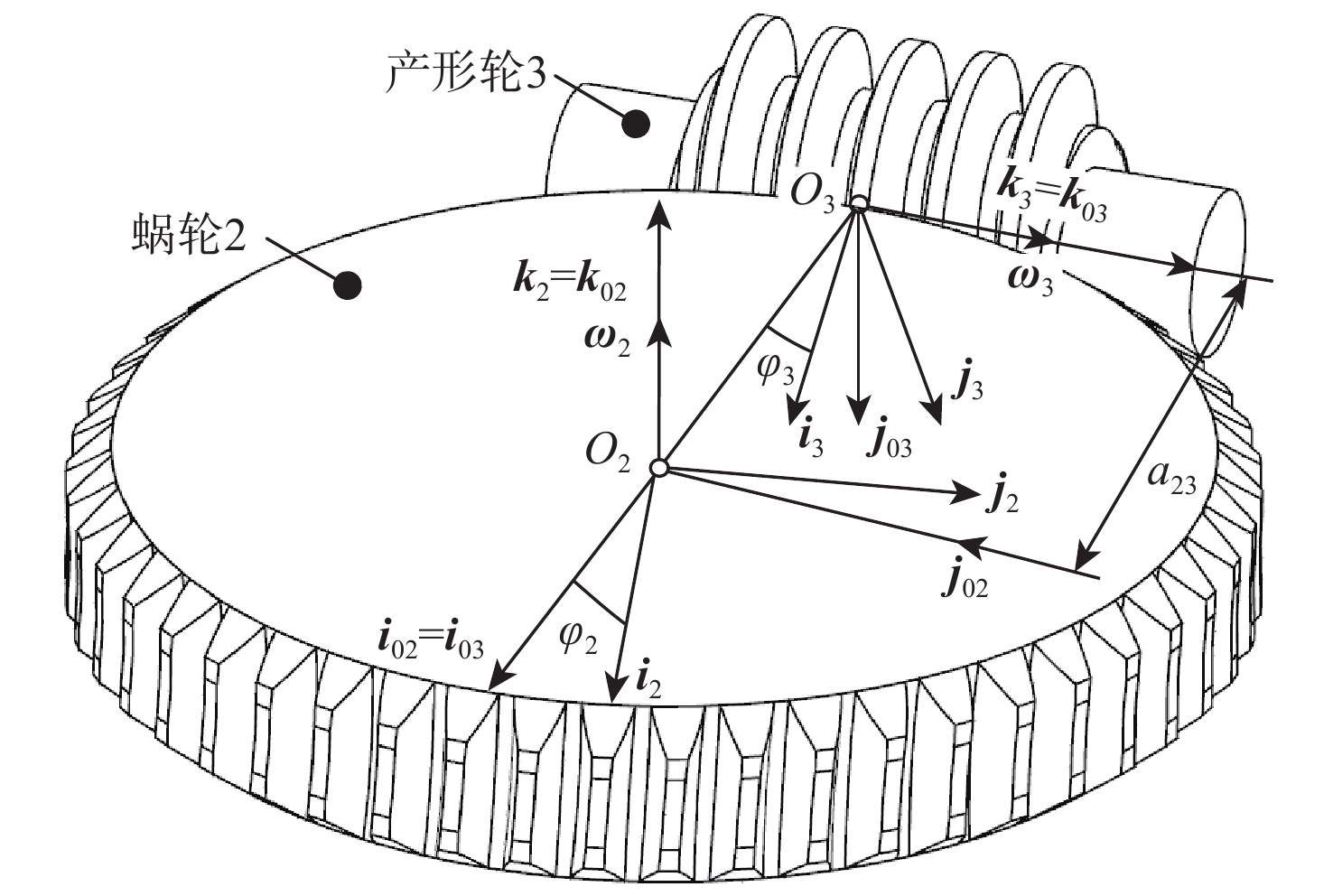
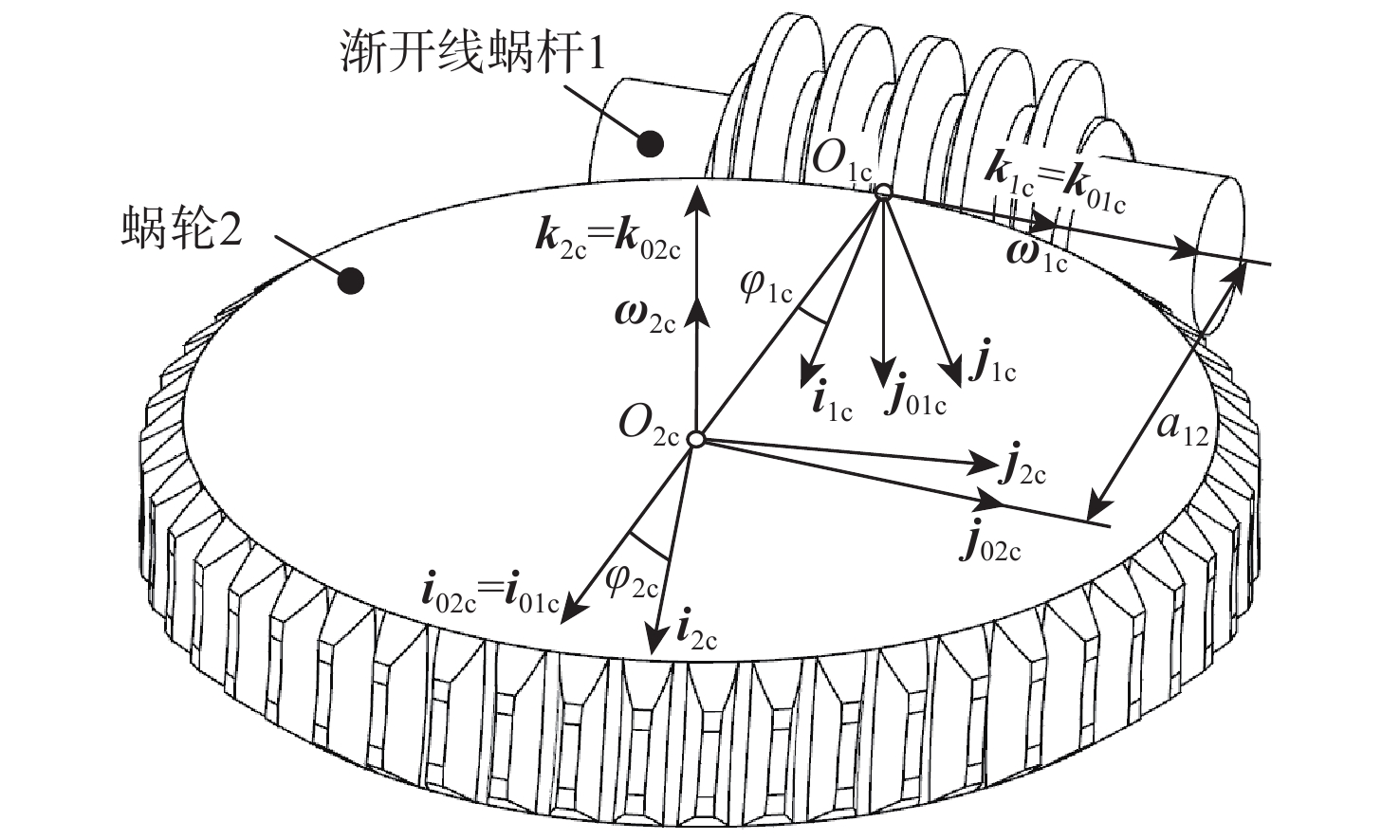
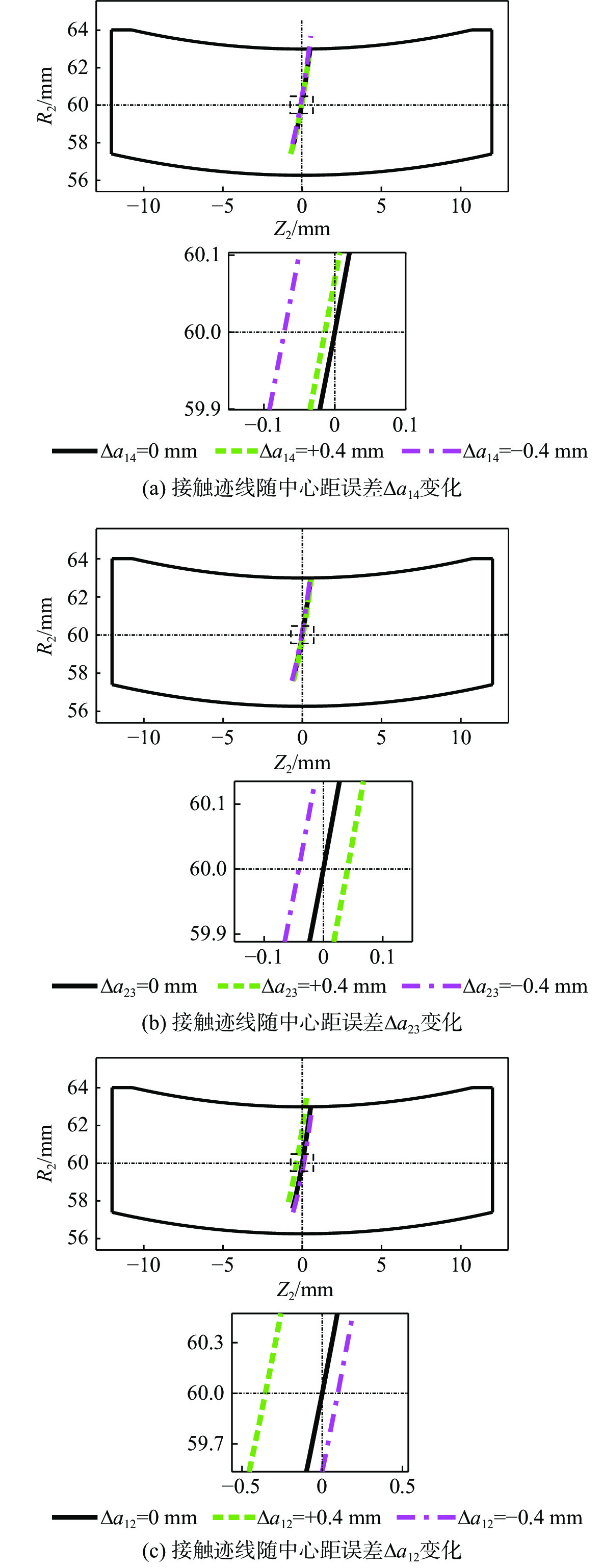
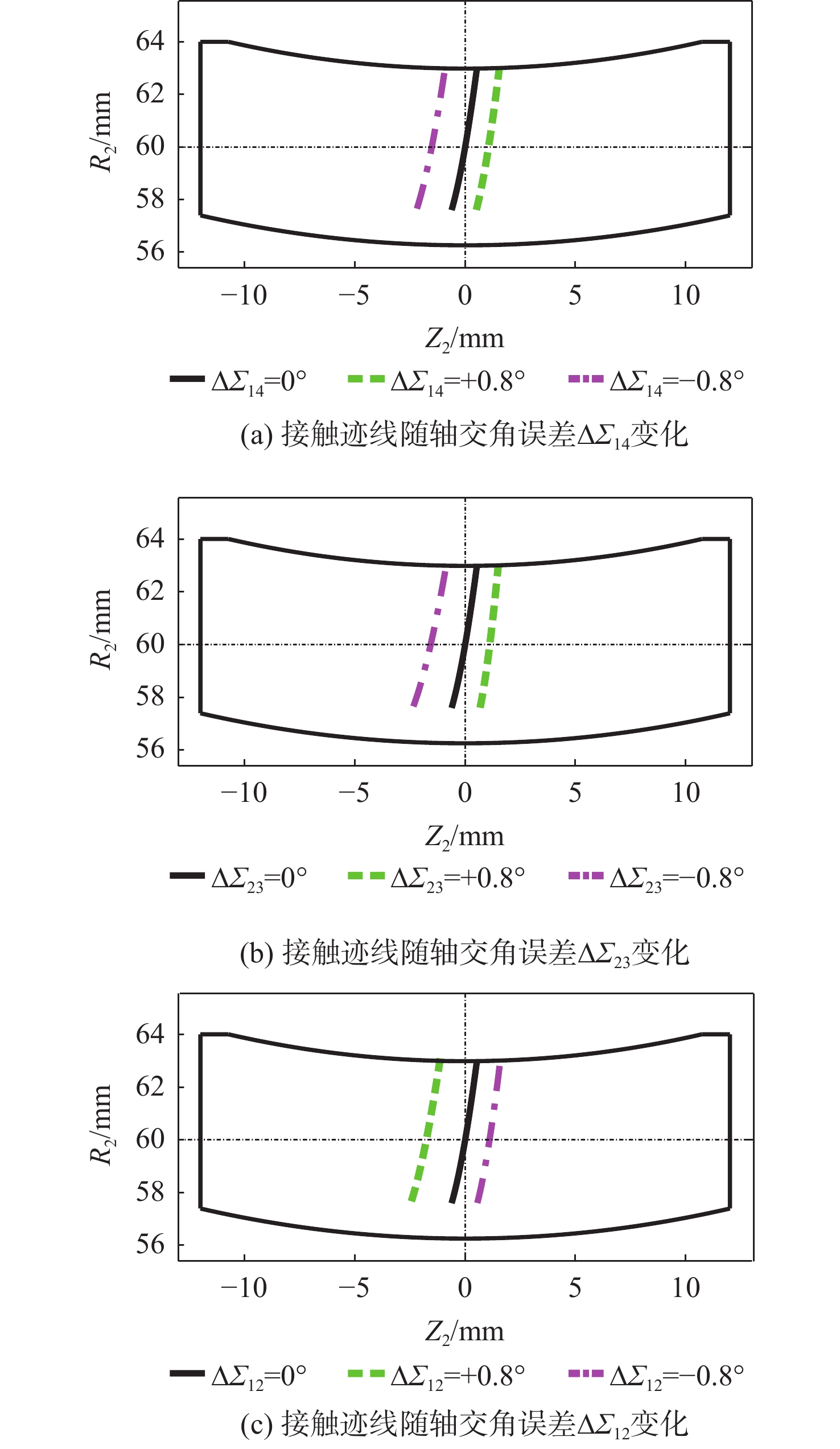
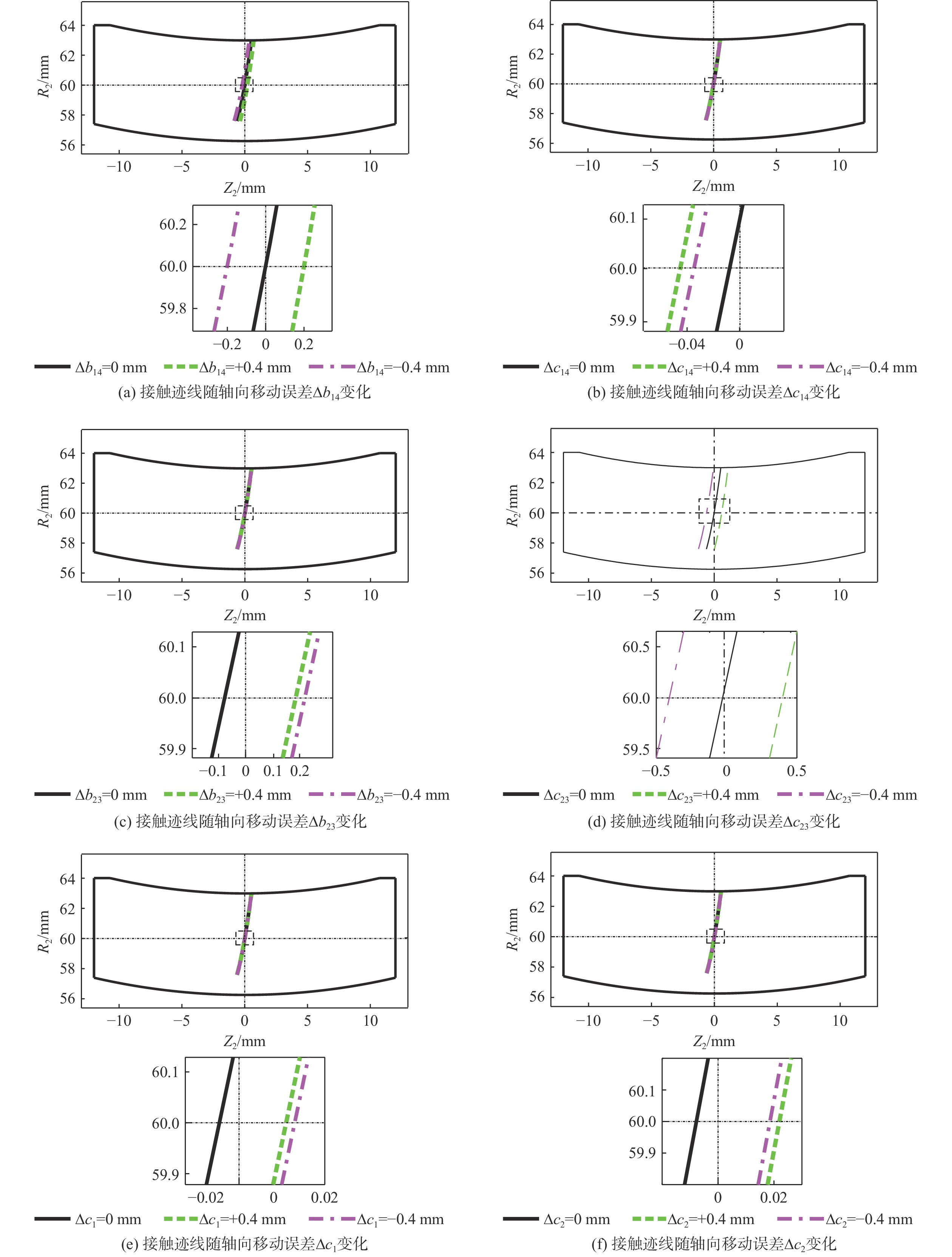
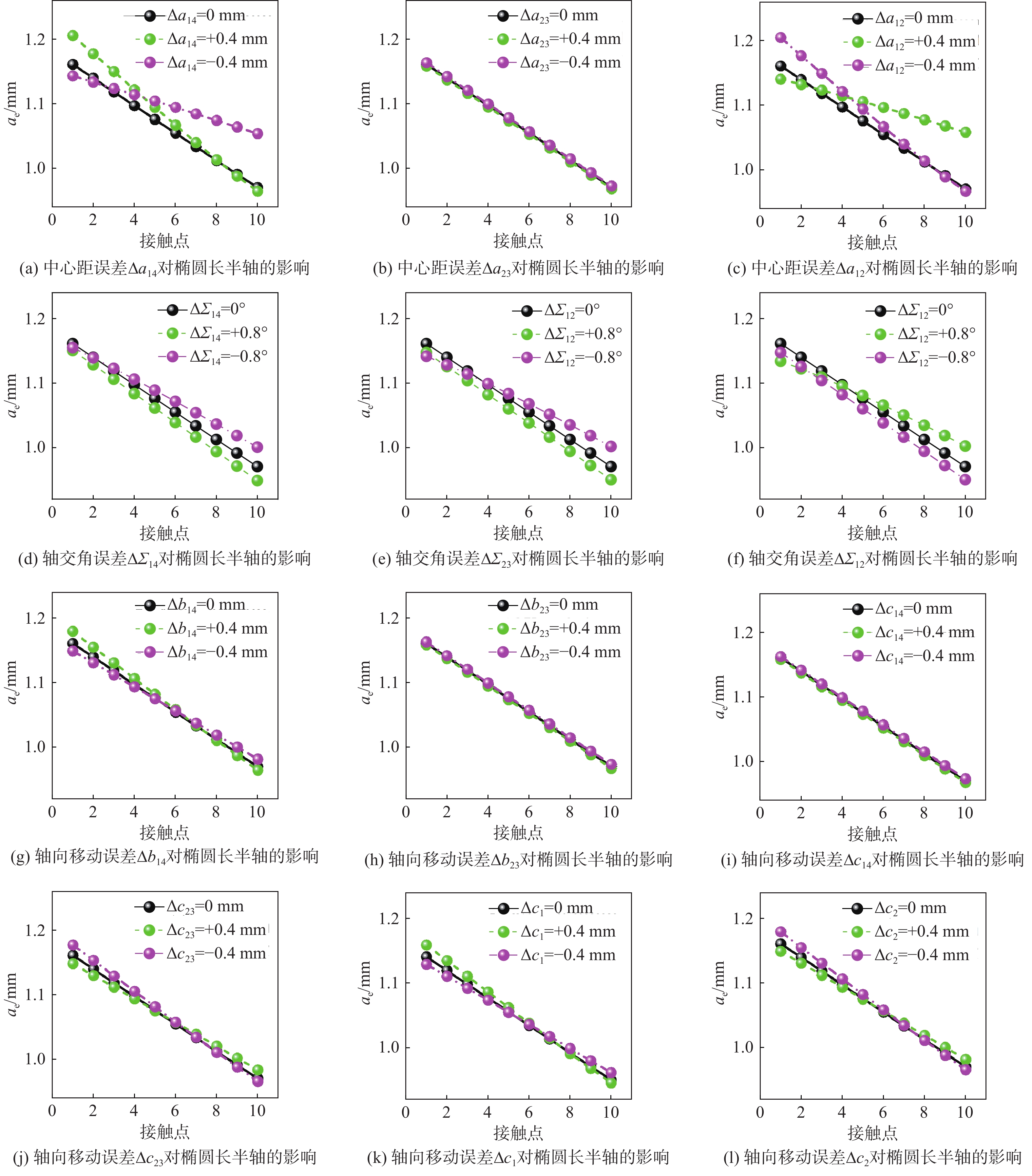
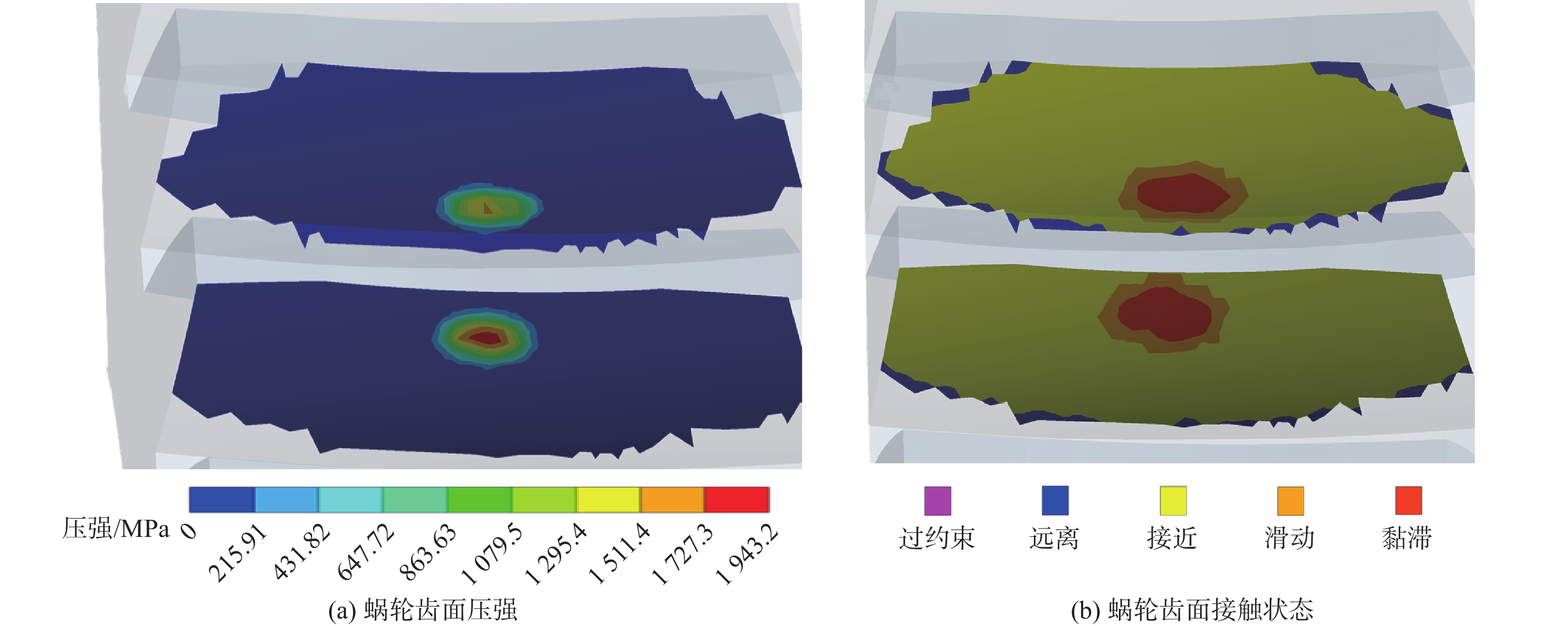
点接触共轭渐开线蜗杆传动可以降低蜗杆副对制造、装配等误差的敏感性,并能保证传动精度。基于微分几何和齿轮啮合原理,构建了含有制造和安装误差的点接触共轭渐开线蜗杆传动数学模型,研究了螺旋角、模数、压力角等主要设计参数对啮合性能的影响规律,并进行了传动副对制造和安装误差敏感性的研究。通过仿真实验验证了接触区的分布情况。研究结果表明,含有制造和安装误差时,传动副的瞬时传动比恒定;选取适中的螺旋角、较小的模数及较小的压力角会增大接触区的面积,提高传动副的重合度;传动副对制造和安装误差均不敏感,但应尽量减小或避免制造和安装轴交角造成的误差。通过仿真分析得到接触区和重合度与理论计算结果一致,验证了所提理论的正确性。
Abstract:Point-contact conjugate involute worm drive can reduce the sensitivity of the worm gear pair to manufacturing and assembly errors and ensure transmission accuracy. On the basis of differential geometry and gear meshing theory, this paper constructed a mathematical model of point-contact conjugate involute worm drive containing manufacturing and assembly errors, studied the influence of main design parameters such as helix angle, modulus, and pressure angle on the meshing performance, and analyzed the sensitivity of the worm gear pair to manufacturing and assembly errors. Finally, the distribution of the contact area was verified through simulation. The results show that the instantaneous drive ratio of the worm gear pair remains constant with manufacturing and assembly errors. The selection of a moderate helix angle, a small modulus, and a small pressure angle will increase the contact area and raise the contact ratio of the worm gear pair. The worm gear pair is insensitive to manufacturing and assembly errors, but the manufacturing and assembly errors of crossed axis angle should be minimized or avoided. The contact area and contact ratio obtained from the simulation analysis agree with the theoretical calculations, verifying the correctness of the theory.
-
Key words:
- point contact /
- conjugate /
- involute worm /
- meshing performance /
- error analysis
-
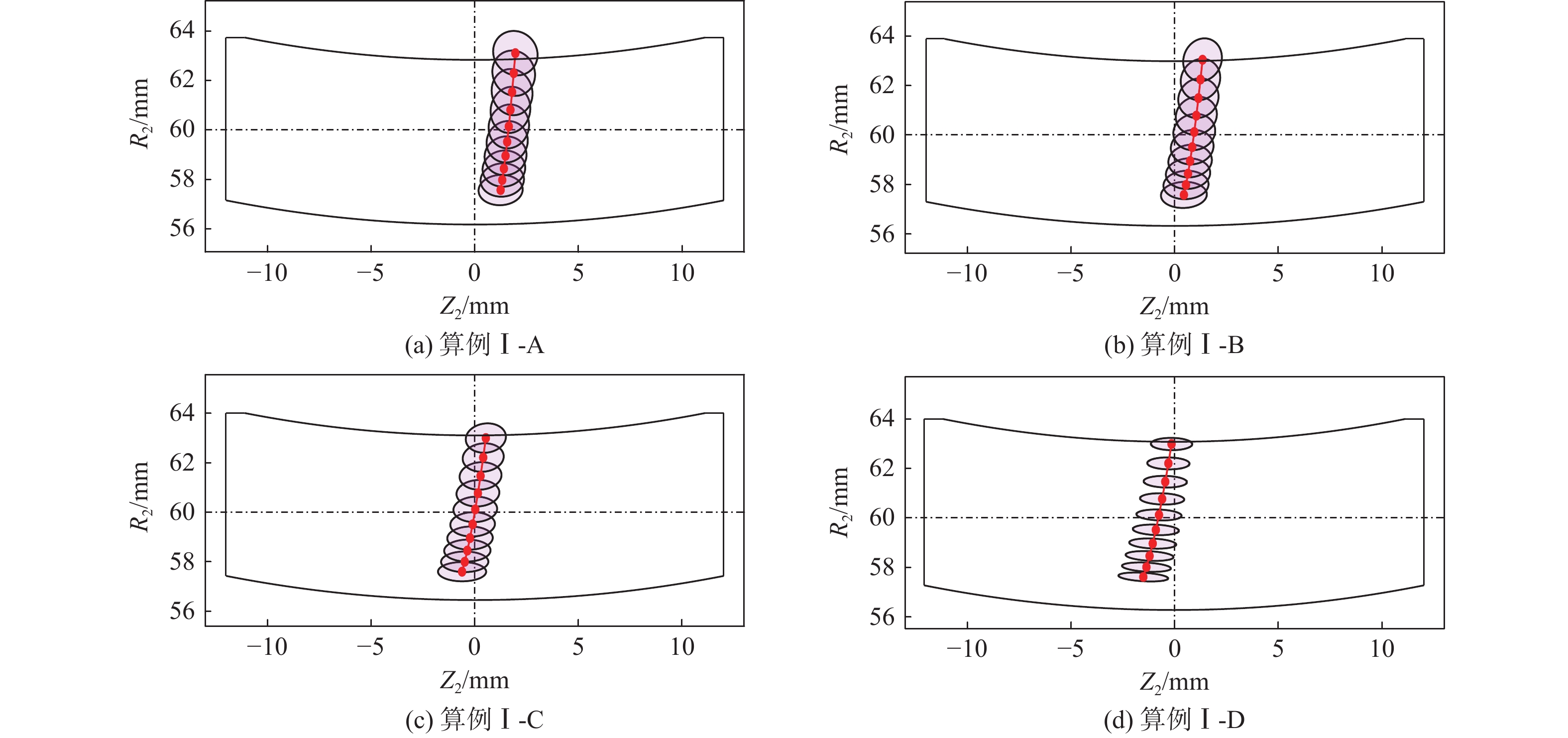
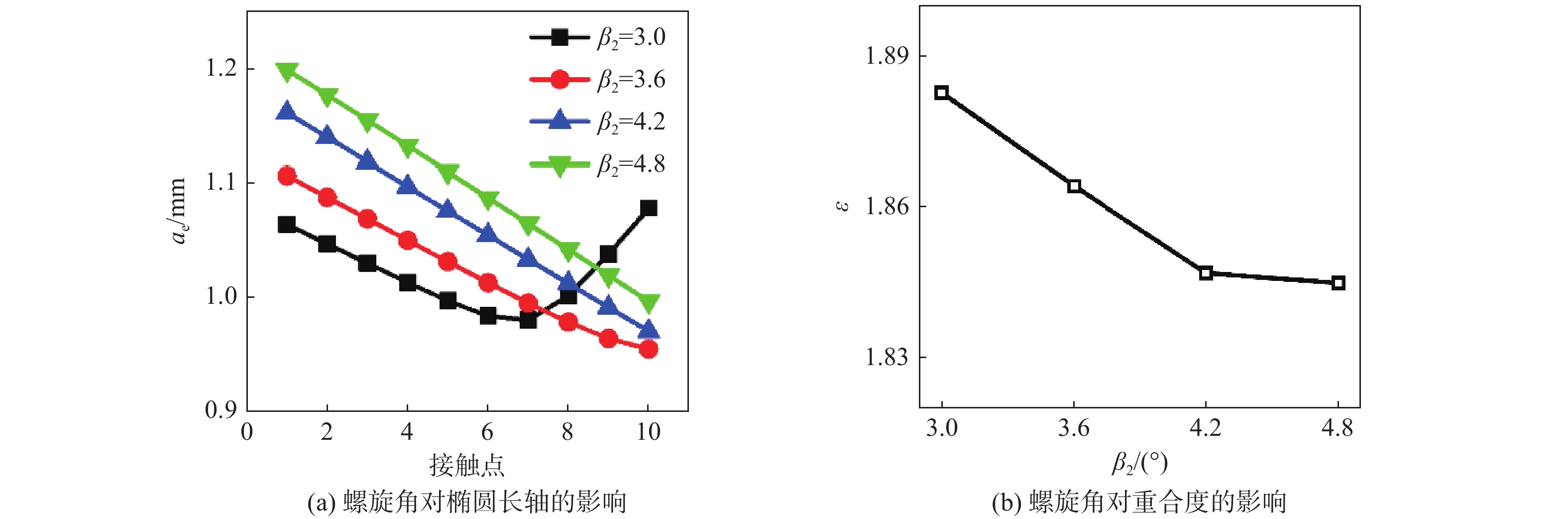
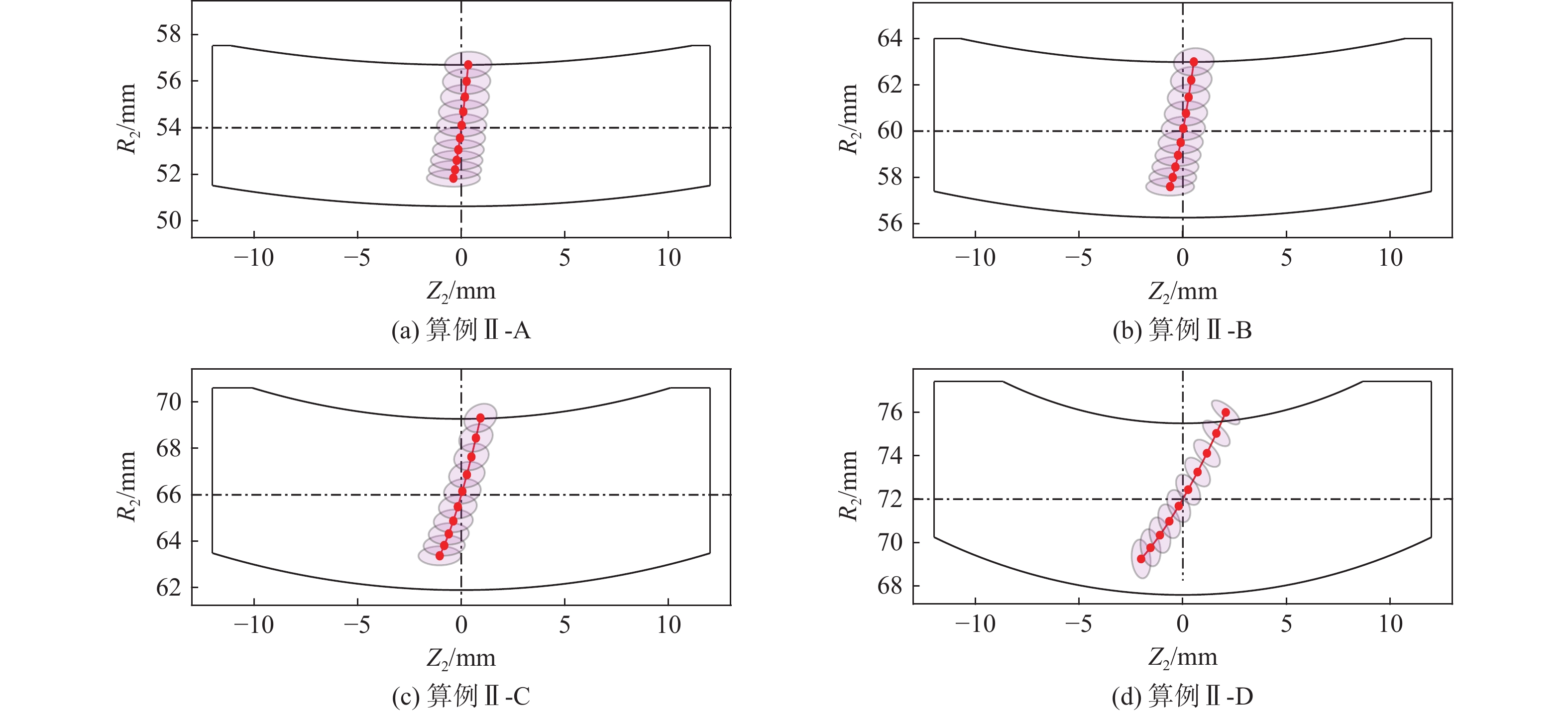
表 1 螺旋角几何参数
Table 1. Geometric parameters of helix angle
算例 螺旋角β2/(°) 算例Ⅰ-A 3.0 算例Ⅰ-B 3.6 算例Ⅰ-C 4.2 算例Ⅰ-D 4.8 表 2 模数几何参数
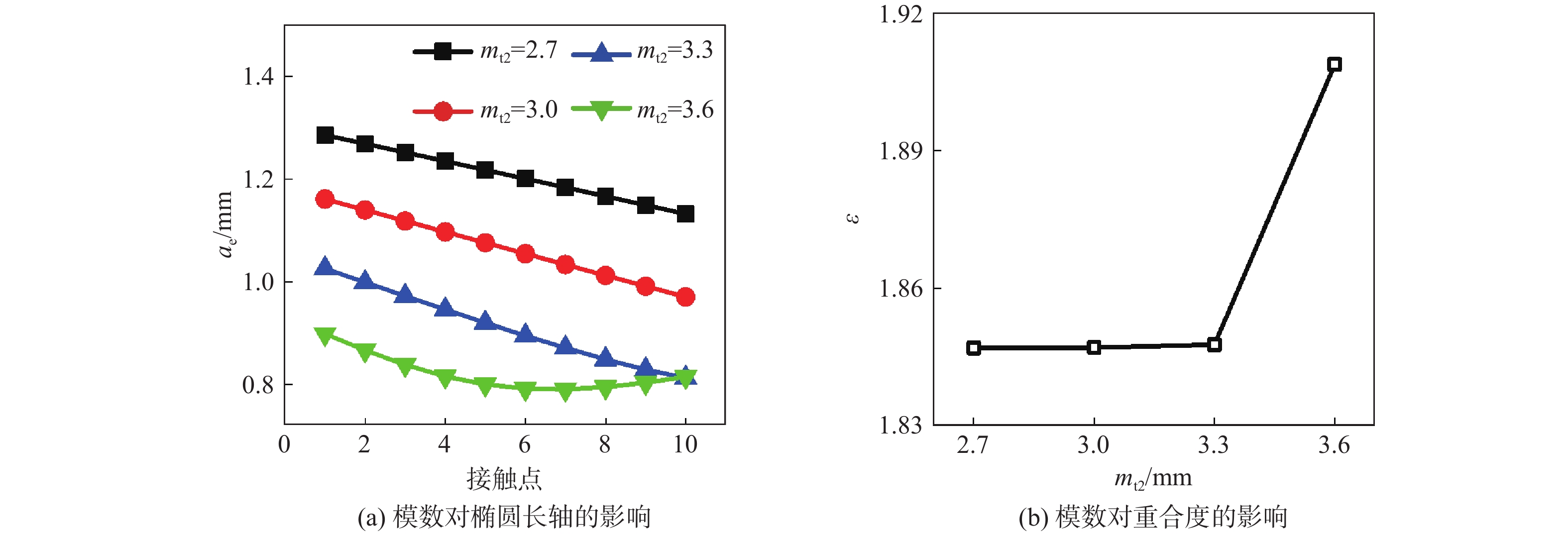
Table 2. Geometric parameters of modulus
算例 端面模数mt2/ mm 算例Ⅱ-A 2.7 算例Ⅱ-B 3.0 算例Ⅱ-C 3.3 算例Ⅱ-D 3.6 表 3 压力角几何参数
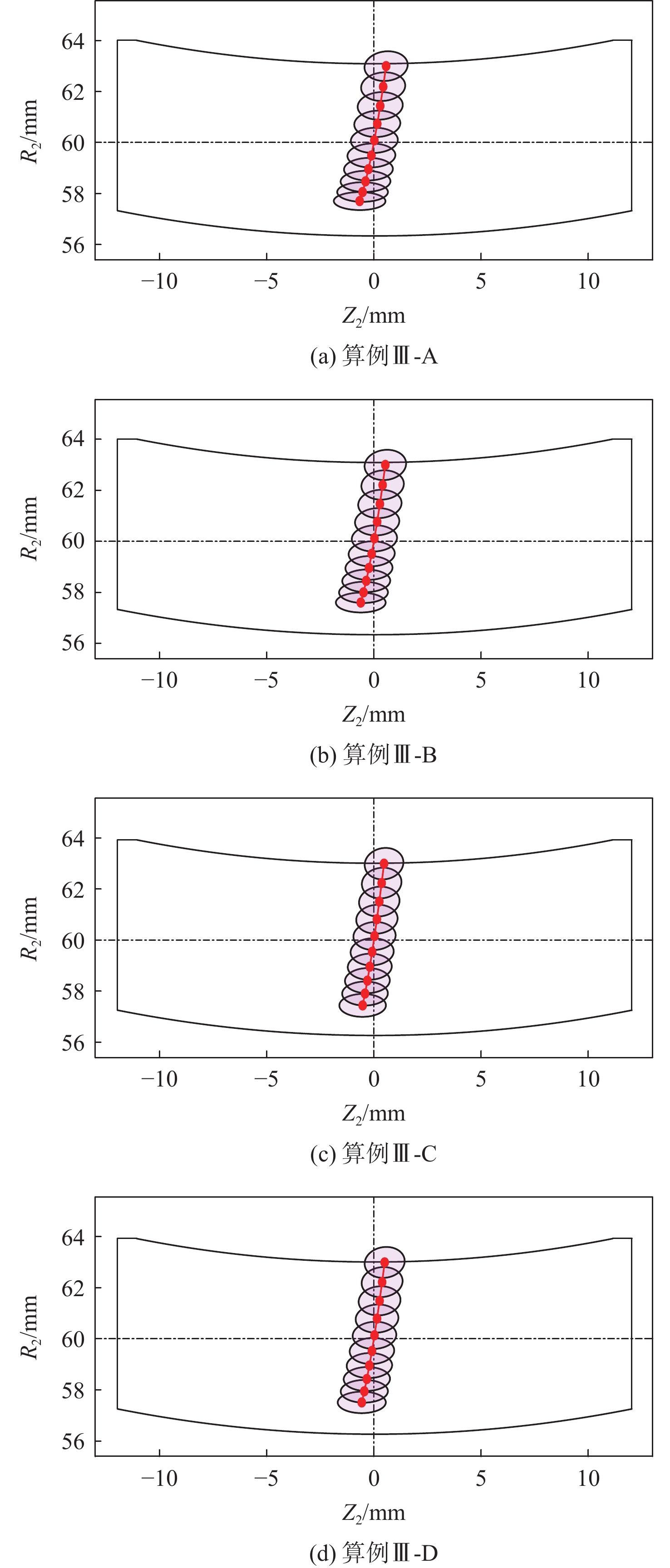
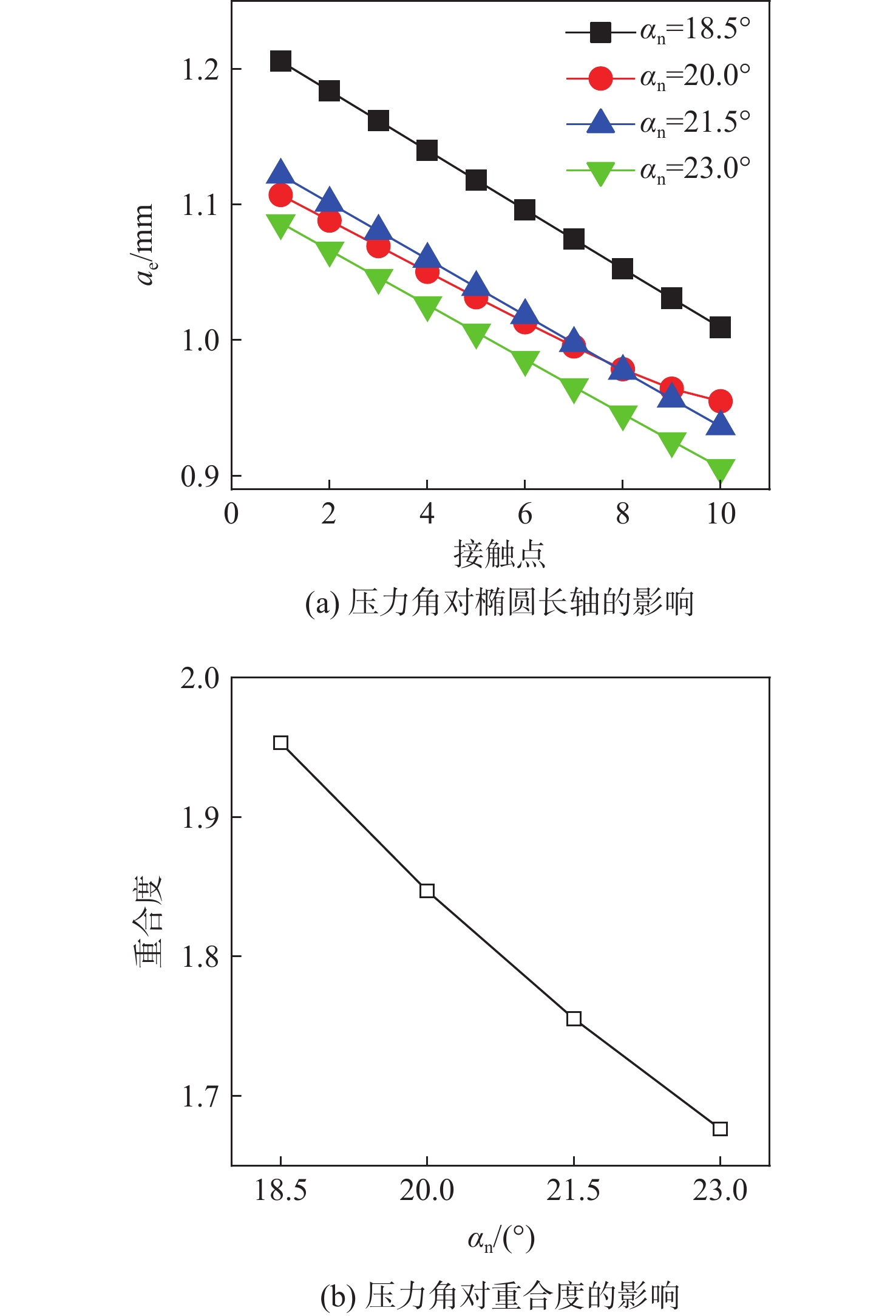
Table 3. Geometric parameters of pressure angle
算例 压力角αn/(°) 算例Ⅲ-A 18.5 算例Ⅲ-B 20.0 算例Ⅲ-C 21.5 算例Ⅲ-D 23.0 表 4 误差几何参数
Table 4. Geometric parameters of errors
参数 误差值 参数 误差值 ∆a14/mm ±0.4 ∆Σ14/(°) ±0.8 ∆a23/mm ±0.4 ∆Σ23/(°) ±0.8 ∆a12/mm ±0.4 ∆Σ12/(°) ±0.8 ∆b14/mm ±0.4 ∆c14/mm ±0.4 ∆b23/mm ±0.4 ∆c23/mm ±0.4 ∆c1/mm ±0.4 ∆c2/mm ±0.4 -
[1] 王树人, 刘平娟. 圆柱蜗杆传动啮合原理[M]. 天津: 天津科学技术出版社, 1982.WANG S R, LIU P J. Meshing principle of cylindrical worm drive[M]. Tianjin: Tianjin Scientific & Technical Publishers, 1982 (in Chinese). [2] LITVIN F L, FUENTES A. Gear geometry and applied theory[M]. 2nd ed. New York: Cambridge University Press, 2004. [3] 吴鸿业, 张亚雄, 齐麟. 蜗杆传动设计[M]. 北京: 机械工业出版社, 1986.WU H Y, ZHANG Y X, QI L. Worm drive design[M]. Beijing: China Machine Press, 1986(in Chinese). [4] 申永胜. 机械原理教程[M]. 2版. 北京: 清华大学出版社, 2005: 162-165.SHEN Y S. Theory of machines and mechanisms[M]. 2nd ed. Beijing: Tsinghua University Press, 2005: 162-165(in Chinese). [5] 田培棠. 齿轮刀具设计与选用手册[M]. 北京: 国防工业出版社, 2011: 73-77.TIAN P T. Handbook of gear cutter design and selection[M]. Beijing: National Defense Industry Press, 2011: 73-77(in Chinese). [6] 彭泽良. 渐开线圆柱蜗杆传动承载啮合理论与实验研究[D]. 重庆: 重庆大学, 2003: 34-39.PENG Z L. Theoretical and experimental research on loaded meshing of involute cylinder worm-drive[D]. Chongqing: Chongqing University, 2003: 34-39(in Chinese). [7] 赵迪, 方舟, 范庆明, 等. 渐开线蜗轮蜗杆参数化设计及装配误差分析[J]. 西安工业大学学报, 2019, 39(2): 152-158.ZHAO D, FANG Z, FAN Q M, et al. Parametric design and assembly error analysis of an involute worm gear[J]. Journal of Xi’an Technological University, 2019, 39(2): 152-158(in Chinese). [8] 沈谦. 点接触圆柱蜗杆传动的设计原理[J]. 南京航空航天大学学报, 1991, 23(2): 48-54.SHEN Q. A design principle of cylindrical worm drive with point contact tooth surface[J]. Journal of Nanjing University of Aeronautics & Astronautics, 1991, 23(2): 48-54(in Chinese). [9] 苏代忠, 陈兵奎, 秦大同, 等. 失配渐开线圆柱蜗杆传动的弹性啮合分析、加工仿真及相关技术的理论与试验研究[J]. 机械工程学报, 2002, 38(增刊1): 56-60.SU D Z, CHEN B K, QIN D T, et al. Elastic meshing analysis, machining simulation and theoretical and experimental research on related technologies of mismatched involute cylindrical worm drive[J]. Chinese Journal of Mechanical Engineering, 2002, 38(Sup 1): 56-60(in Chinese). [10] YE X X, CHEN Y H, LU B B, et al. Study on a novel backlash-adjustable worm drive via the involute helical beveloid gear meshing with dual-lead involute cylindrical worm[J]. Mechanism and Machine Theory, 2022, 167: 104466. doi: 10.1016/j.mechmachtheory.2021.104466 [11] SIMON V. Load distribution in cylindrical worm gears[J]. Journal of Mechanical Design, 2003, 125(2): 356-364. doi: 10.1115/1.1561043 [12] SIMON V. The influence of gear hobbing on worm gear characteristics[J]. Journal of Manufacturing Science and Engineering, 2007, 129(5): 919-925. doi: 10.1115/1.2752524 [13] SIMON V. Computer aided loaded tooth contact analysis in cylindrical worm gears[J]. Journal of Mechanical Design, 2005, 127(5): 973-981. doi: 10.1115/1.1904050 [14] 秦大同, 张光辉, 加藤正名. 失配点啮合环面蜗杆传动的研究[J]. 机械工程学报, 1995, 31(3): 79-83.QIN D T, ZHANG G H, KATO M. Research on mismatch point-contact hourglass worm[J]. Chinese Journal of Mechanical Engineering, 1995, 31(3): 79-83(in Chinese). [15] 秦大同, 张光辉, 加藤正名. 锥面包络环面蜗杆与直廓环面蜗轮失配啮合传动的研究[J]. 重庆大学学报(自然科学版), 1995, 18(4): 15-20.QIN D T, ZHANG G H, KATO M. Study on mismatched engagement of hourglass worm gearing with the worm generated by a cone and the wheel generated by a straight profile hob[J]. Journal of Chongqing University (Natural Science Edition), 1995, 18(4): 15-20(in Chinese). [16] MENG Q X, ZHAO Y P, YANG Z Y, et al. Meshing theory and error sensitivity of mismatched conical surface enveloping conical worm pair[J]. Mechanism and Machine Theory, 2020, 145: 103681. doi: 10.1016/j.mechmachtheory.2019.103681 [17] MENG Q X, ZHAO Y P, CUI J, et al. Meshing theory of mismatched ZC1 worm drive[J]. Mechanism and Machine Theory, 2020, 150: 103869. doi: 10.1016/j.mechmachtheory.2020.103869 [18] CHI Y F, ZHAO Y P, ZHU X Y, et al. Mismatched gearing composed of hourglass worm and spur gear: Meshing theory, tooth contact simulation, comprehensive design[J]. Mechanism and Machine Theory, 2022, 174: 104883. doi: 10.1016/j.mechmachtheory.2022.104883 [19] 赵超飞, 魏冰阳. 直廓环面蜗杆-圆柱斜齿轮传动的几何建模与接触特性分析[J]. 机械传动, 2018, 42(11): 123-126.ZHAO C F, WEI B Y. Geometric modeling and contact characteristic analysis of hindley worm and cylindrical helical gear transmission[J]. Journal of Mechanical Transmission, 2018, 42(11): 123-126(in Chinese). [20] 董学朱. 齿轮啮合理论基础[M]. 北京: 机械工业出版社,1989.DONG X Z. Foundation of gear engagement theory[M]. Beijing: China Machine Press, 1989(in Chinese). [21] REN W, LI H T, XU Z K, et al. A point-contact conjugate hourglass worm drive based on the meshing theory of conjugate tooth surfaces generated by two generating surfaces[J]. Mechanism and Machine Theory, 2022, 174: 104877. doi: 10.1016/j.mechmachtheory.2022.104877 [22] 董学朱, 李海清, 魏文军. 双自由度齿轮啮合理论及应用[M]. 北京: 机械工业出版社,2011.DONG X Z, LI H T, WEI W J. Theory and application of gear meshing with two degrees of freedom[M]. Beijing: China Machine Press, 2011(in Chinese). -







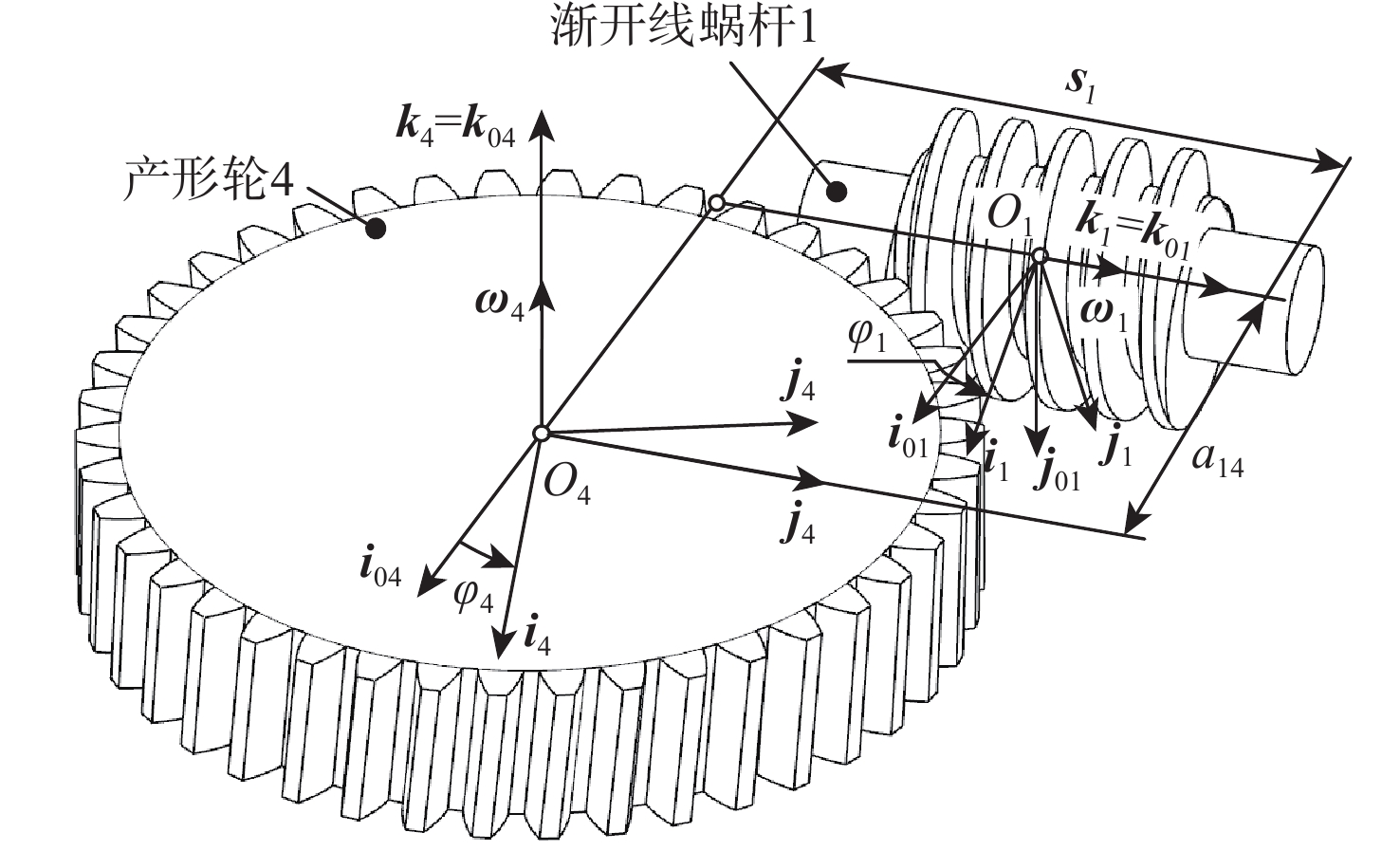
 下载:
下载: