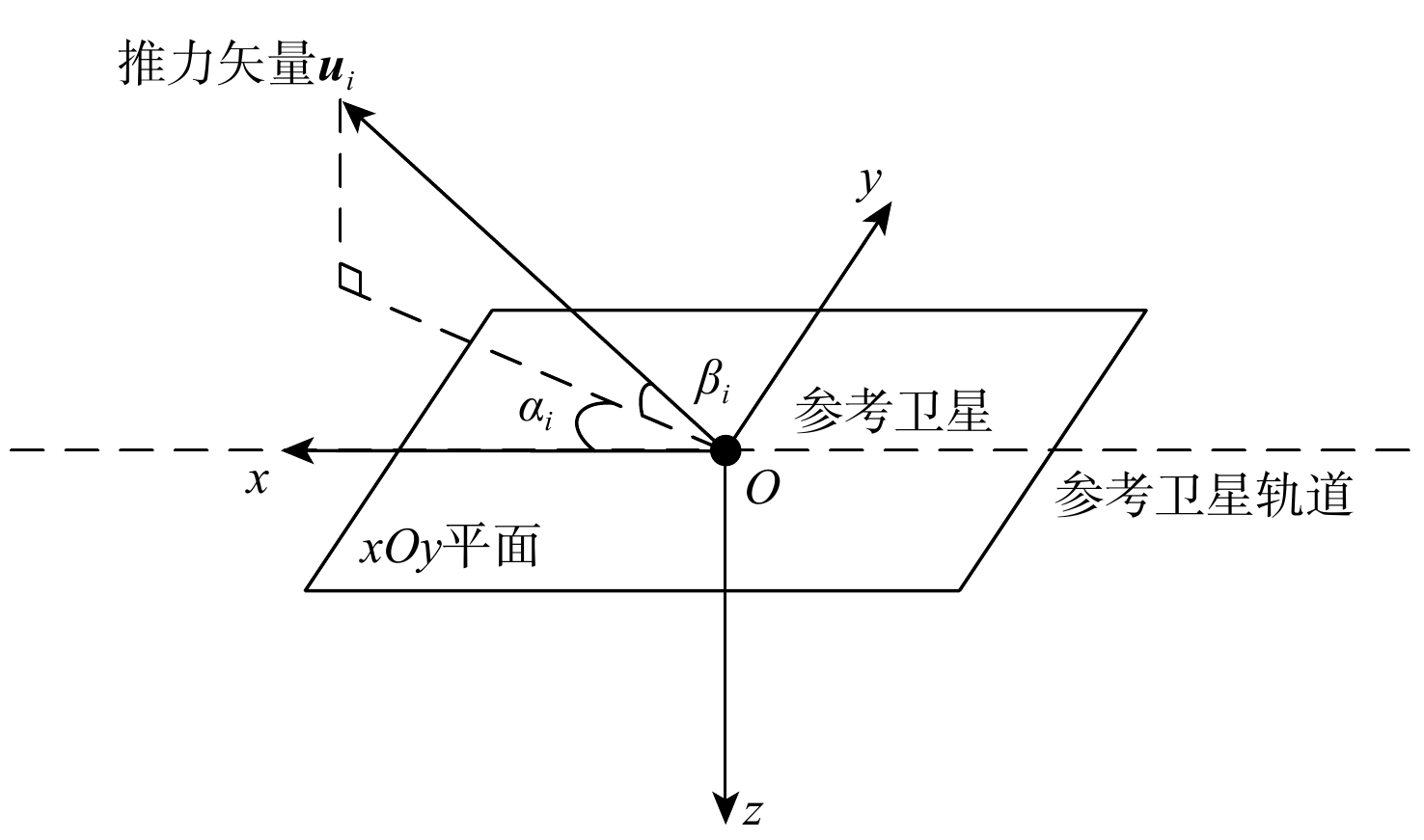
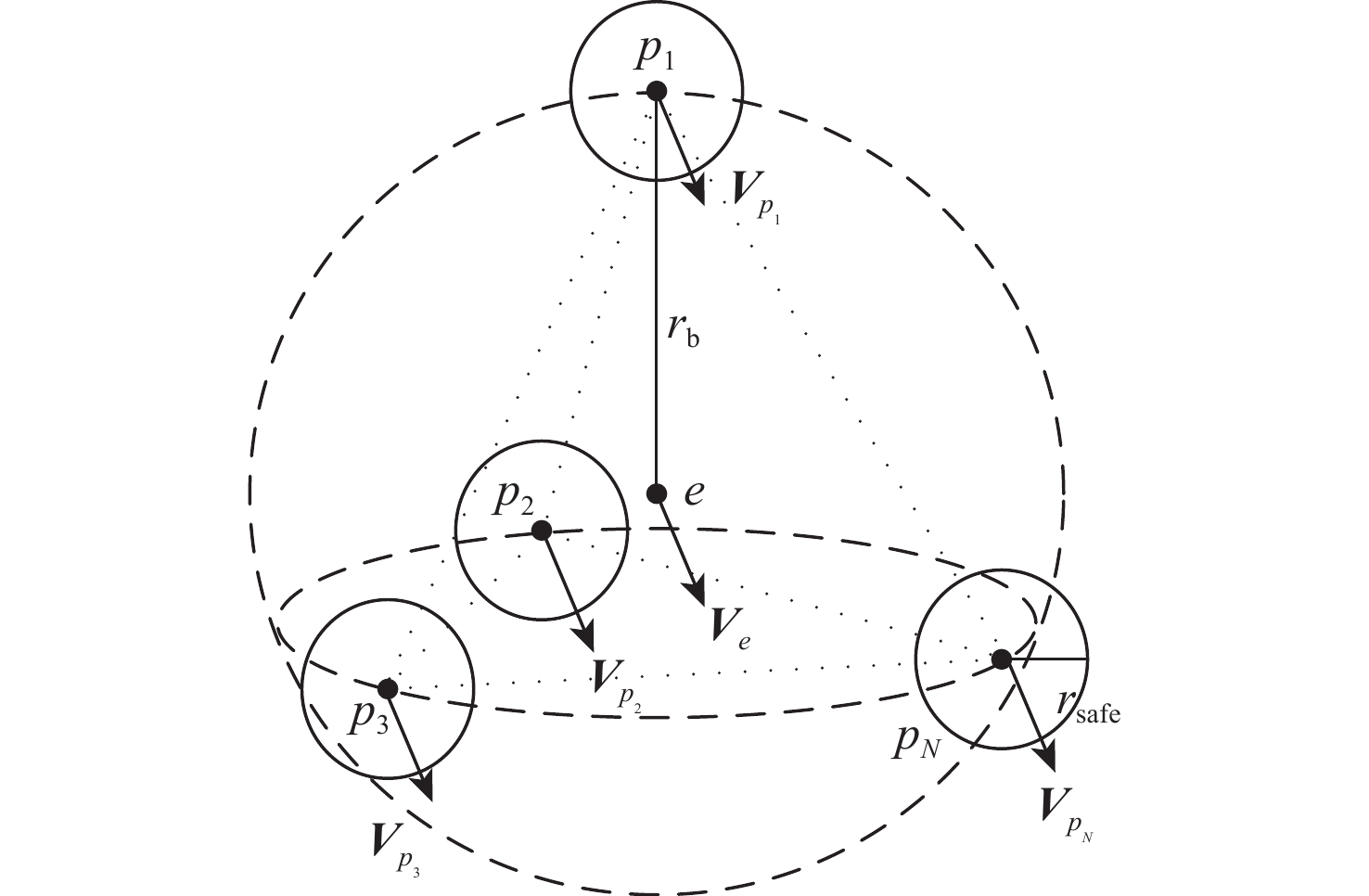
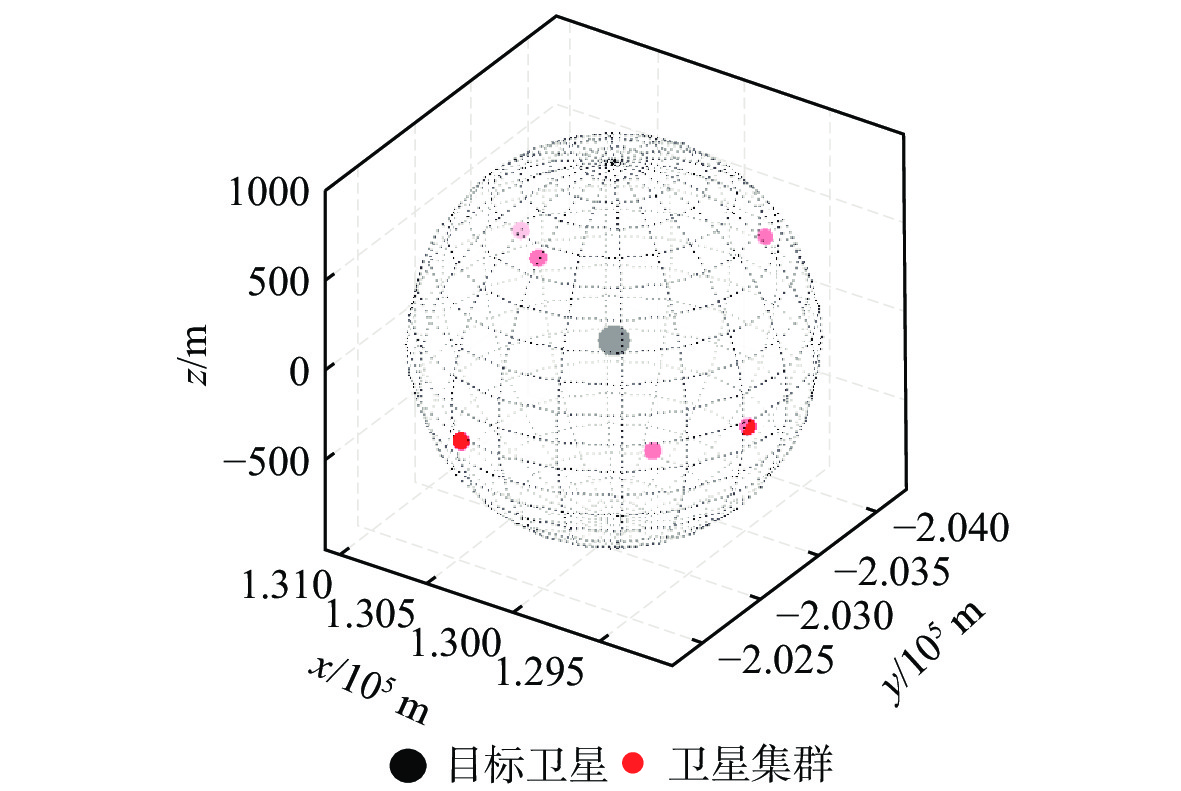
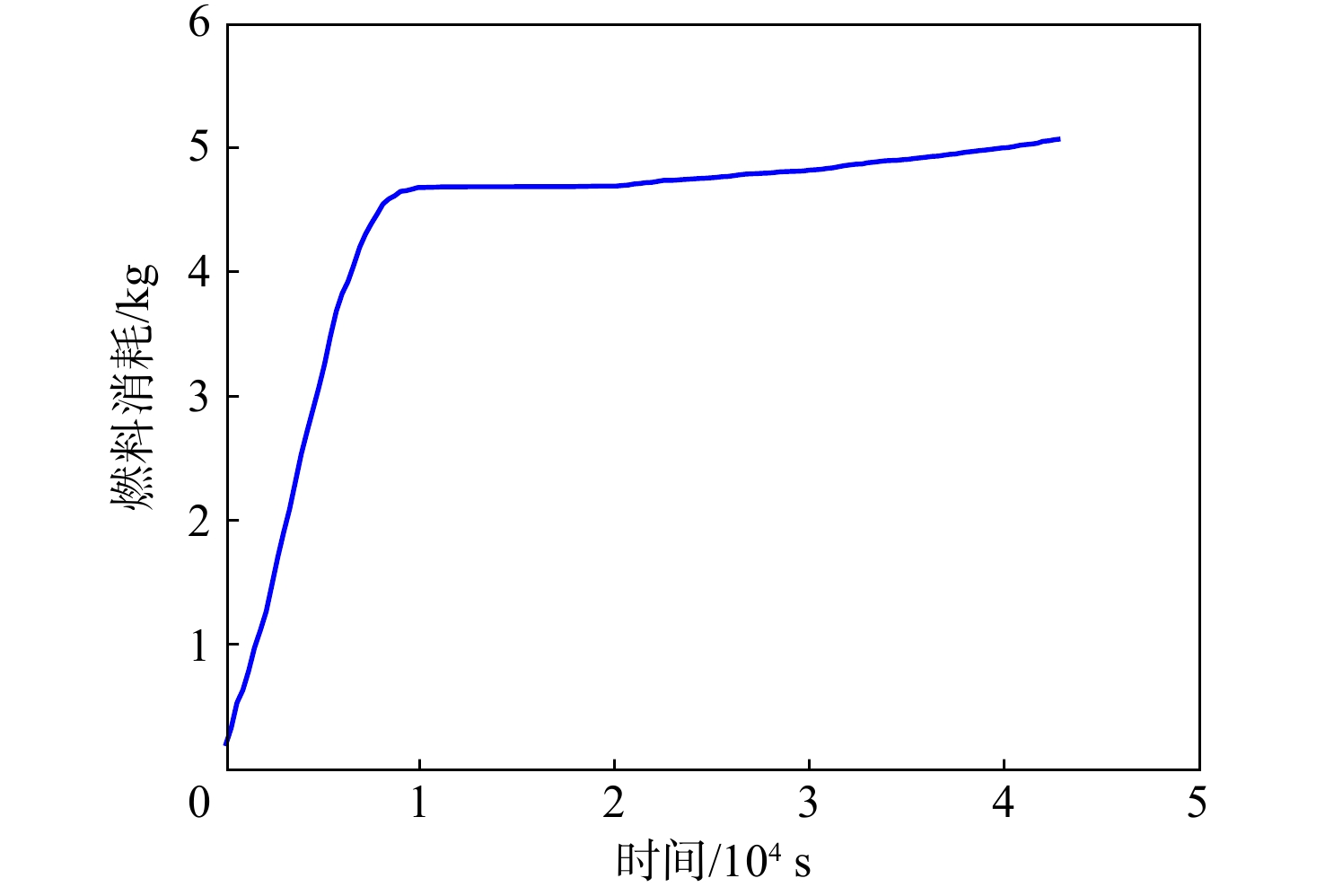
Round-up method of space target by satellites swarm based on wolf pack optimization
-
摘要:
卫星集群对空间目标形成围捕态势是空间轨道竞争的重要一环，利于卫星集群对目标实施全方位强实时探测感知、多角度追逃博弈。现有研究多基于微分对策理论求解一追一逃博弈模型，星群协同配合实现围捕则是研究难点。针对由卫星集群围捕空间目标这一难题，提出了基于狼群优化算法的空间目标围捕方法。将围捕任务总时长离散为多个决策周期，设计了综合考虑集群位置分布、合围态势、燃料消耗与安全防撞的适应度函数，通过对每个决策周期卫星集群内各星推力矢量的协同优化，控制卫星集群接近空间目标并近似均布在以目标为球心的球面上。仿真结果表明：所提方法能够快速获得卫星集群对空间目标围捕的机动策略，构建出集群围捕态势，有效支持空间轨道竞争任务。
Abstract:An essential part of space orbit competition research is the way the satellites swarm together to generate a round-up situation for space targets. The round-up situation can effectively support the satellite swarm to implement detection and perception with all-around and real-time performance and multi-angle pursuit-evasion game for space targets. Most of the existing research on the problem of space target pursuit-evasion was based on the differential game theory to establish the game model to solve one pursuit and one escape problem. It is a research difficulty for satellite swarms to cooperate and realize round-up in the form of groups. Aiming at the problem of rounding up a space target with a satellite swarm, a method based on a wolf pack optimization algorithm is proposed. Discrete the total duration of the round-up task into multiple decision-making cycles, and design a fitness function that comprehensively considers the position distribution of the satellite swarm, the round-up situation, the fuel consumption and safety collision avoidance. Control the satellite swarm to close to the target satellite and to distribute approximately evenly on the sphere with the target as the center via co-optimizing the thrust vector of satellite swarm in the decision-making cycle. The outcomes of the simulation demonstrate that the approach is capable of obtaining the satellite swarm's maneuvering strategy to swiftly round up the space target, create a round-up scenario, and efficiently support the space orbit competition task.
-
Key words:
- satellites swarm /
- space round-up /
- wolf pack algorithm /
- maneuver control /
- collaborative optimization
-
图 5 目标卫星与围捕卫星5相对参考卫星速度曲线
Figure 5. The velocity curves of the target satellite and the No. 5 round-up satellite relative to the reference satellite
图 6 卫星集群与目标卫星之间距离变化曲线
Figure 6. Curves of distance change between satellites swarm and target satellite
图 7 卫星集群与目标卫星位置分布示意图
Figure 7. Schematic diagram of position distribution of satellites swarm and target satellite
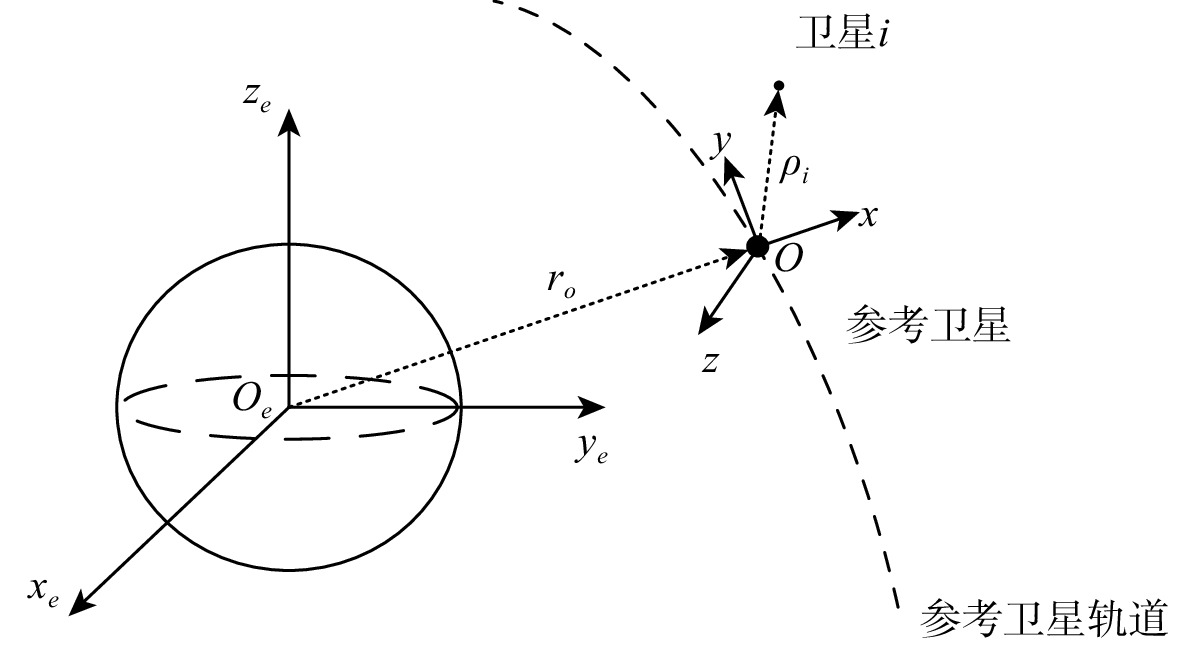
表 1 围捕任务所需参数
Table 1. Parameters required for the round-up mission
参数 数值 围捕半径${r_{\mathrm{b}}}$/m 1 000 “避碰区”半径$ {r_{{\mathrm{safe}}}} $/ m 1 000 任务总时长${T_{\text{f}}}$/s 43 200 决策周期时长/s 300 集群各卫星质量${m_i}$/kg 100 集群各卫星最大轨控推力${u_{\max }}$/N 10 集群各卫星最小轨控推力${u_{\min }}$/N 8 
下载: 导出CSV
表 2 参考卫星轨道根数
Table 2. Orbital elements of reference satellite
半长轴/
km偏心率 轨道倾角/
rad升交点赤经/
rad近地点幅角/
rad平近点角/
rad42164.2 0 0 0 0 3.496734
下载: 导出CSV
表 3 目标卫星与卫星集群相对参考卫星初始状态
Table 3. Initial state of target satellite and satellites swarm relative to the reference satellite
卫星 $x$/m $y$/m ${\textit{z}}$/m $\dot x$/
(m·s−1)$\dot y$ /
( m·s−1)$\dot {\textit{z}}$/
( m·s−1)目标卫星 50396.8 −290.8 −0.22 0 −5.53 0 围捕卫星1 −29.64 50000.0 0 6.73 −2.72 0.176 围捕卫星2 −24.82 44999.6 −0.22 7.50 0 0 围捕卫星3 −11.69 30000.0 0 6.13 −4.32 0.082 围捕卫星4 −8.18 24999.8 0 7.50 0 0 围捕卫星5 −1.73 9999.4 0 7.47 0 0 围捕卫星6 −11.17 − 30000 0 7.32 −1.62 −0.11
下载: 导出CSV
-
[1] 许旭升, 党朝辉, 宋斌, 等. 基于多智能体强化学习的轨道追逃博弈方法[J]. 上海航天, 2022, 39(2): 24-31.XU X S, DANG Z H, SONG B, et al. Method for cluster satellite orbit pursuit-evasion game based on multi-agent deep deterministic policy gradient algorithm[J]. Aerospace Shanghai, 2022, 39(2): 24-31(in Chinese). [2] 吴其昌, 张洪波. 基于生存型微分对策的航天器追逃策略及数值求解[J]. 控制与信息技术, 2019(4): 39-43.WU Q C, ZHANG H B. Spacecraft pursuit strategy and numerical solution based on survival differential strategy[J]. Control and Information Technology, 2019(4): 39-43(in Chinese). [3] BATHER J A, ISAACS R. Differential games: A mathematical theory with applications to warfare and pursuit, control and optimization[J]. Journal of the Royal Statistical Society Series A (General), 1966, 129(3): 474. [4] BERKOVITZ L D. Necessary conditions for optimal strategies in a class of differential games and control problems[J]. SIAM Journal on Control, 1967, 5(1): 1-24. doi: 10.1137/0305001 [5] FRIEDMAN A. On the definition of differential games and the existence of value and of saddle points[J]. Journal of Differential Equations, 1970, 7(1): 69-91. [6] OSHMAN Y, RAD D A. Differential-game-based guidance law using target orientation observations[J]. IEEE Transactions on Aerospace and Electronic Systems, 2006, 42(1): 316-326. doi: 10.1109/TAES.2006.1603425 [7] YE D, SHI M M, SUN Z W. Satellite proximate pursuit-evasion game with different thrust configurations[J]. Aerospace Science and Technology, 2020, 99: 105715. doi: 10.1016/j.ast.2020.105715 [8] GONG H R, GONG S P, LI J F. Pursuit-evasion game for satellites based on continuous thrust reachable domain[J]. IEEE Transactions on Aerospace and Electronic Systems, 2020, 56(6): 4626-4637. doi: 10.1109/TAES.2020.2998197 [9] 张秋华, 孙松涛, 谌颖, 等. 时间固定的两航天器追逃策略及数值求解[J]. 宇航学报, 2014, 35(5): 537-544.ZHANG Q H, SUN S T, CHEN Y, et al. Strategy and numerical solution of pursuit-evasion with fixed duration for two spacecraft[J]. Journal of Astronautics, 2014, 35(5): 537-544(in Chinese). [10] YU D T, WANG H, ZHOU J P, et al. Capturability analysis of TPN guidance law for circular orbital pursuit-evasion[J]. Transactions of the japan society for Aeronautical And Space Sciences, 2017, 60(6): 347-354. doi: 10.2322/tjsass.60.347 [11] 陈木生. 基于微分对策及协同进化算法的三航天器追逃策略求解[D]. 哈尔滨: 哈尔滨工业大学, 2021: 43-51.CHEN M S. Solution of three spacecraft pursuit strategy based on differential game and co-evolution algorithm[D]. Harbin: Harbin Institute of Technology, 2021: 43-51(in Chinese). [12] SARGENT L H, COVERSTONE V L, RODRIGUEZ N, et al. Toward reinforcement learning identification for swarms engaged in cooperative pursuit[C]//Proceedings of the AIAA SCITECH 2022 Forum. Reston: AIAA, 2022. [13] 刘暾, 赵钧. 空间飞行器动力学[M]. 哈尔滨: 哈尔滨工业大学出版社, 2003: 83-101.LIU T, ZHAO J. Spacecraft dynamics[M]. Harbin: Harbin Institute of Technology Press, 2003: 83-101(in Chinese). [14] CLOHESSY W H, WILTSHIRE R S. Terminal guidance system for satellite rendezvous[J]. Journal of the Aerospace Sciences, 1960, 27(9): 653-658. doi: 10.2514/8.8704 [15] 刘源, 李玉玲, 郝勇, 等. 航天器三维空间追逃问题研究[J]. 系统工程与电子技术, 2018, 40(4): 868-877. doi: 10.3969/j.issn.1001-506X.2018.04.22LIU Y, LI Y L, HAO Y, et al. Research on three dimensional space pursuit-evasion of spacecraft[J]. Systems Engineering and Electronics, 2018, 40(4): 868-877(in Chinese). doi: 10.3969/j.issn.1001-506X.2018.04.22 [16] 吴虎胜, 张凤鸣, 吴庐山. 一种新的群体智能算法: 狼群算法[J]. 系统工程与电子技术, 2013, 35(11): 2430-2438.WU H S, ZHANG F M, WU L S. New swarm intelligence algorithm—Wolf pack algorithm[J]. Systems Engineering and Electronics, 2013, 35(11): 2430-2438(in Chinese). [17] 刘永兰, 李为民, 吴虎胜, 等. 基于狼群算法的无人机航迹规划[J]. 系统仿真学报, 2015, 27(8): 1838-1843.LIU Y L, LI W M, WU H S, et al. Track planning for unmanned aerial vehicles based on wolf pack algorithm[J]. Journal of System Simulation, 2015, 27(8): 1838-1843(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 322
- HTML全文浏览量: 93
- PDF下载量: 1
- 被引次数: 0
