-
摘要:
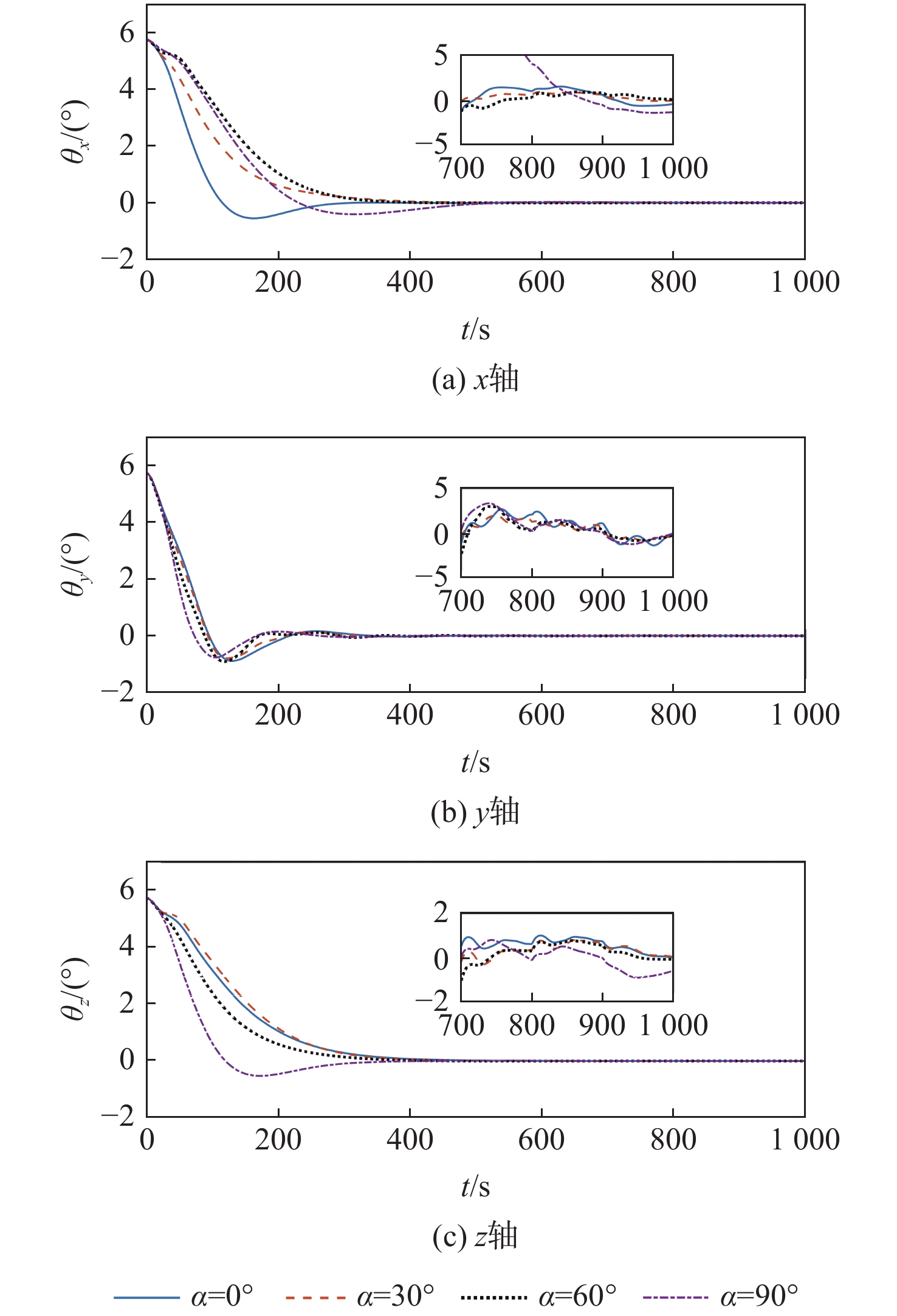
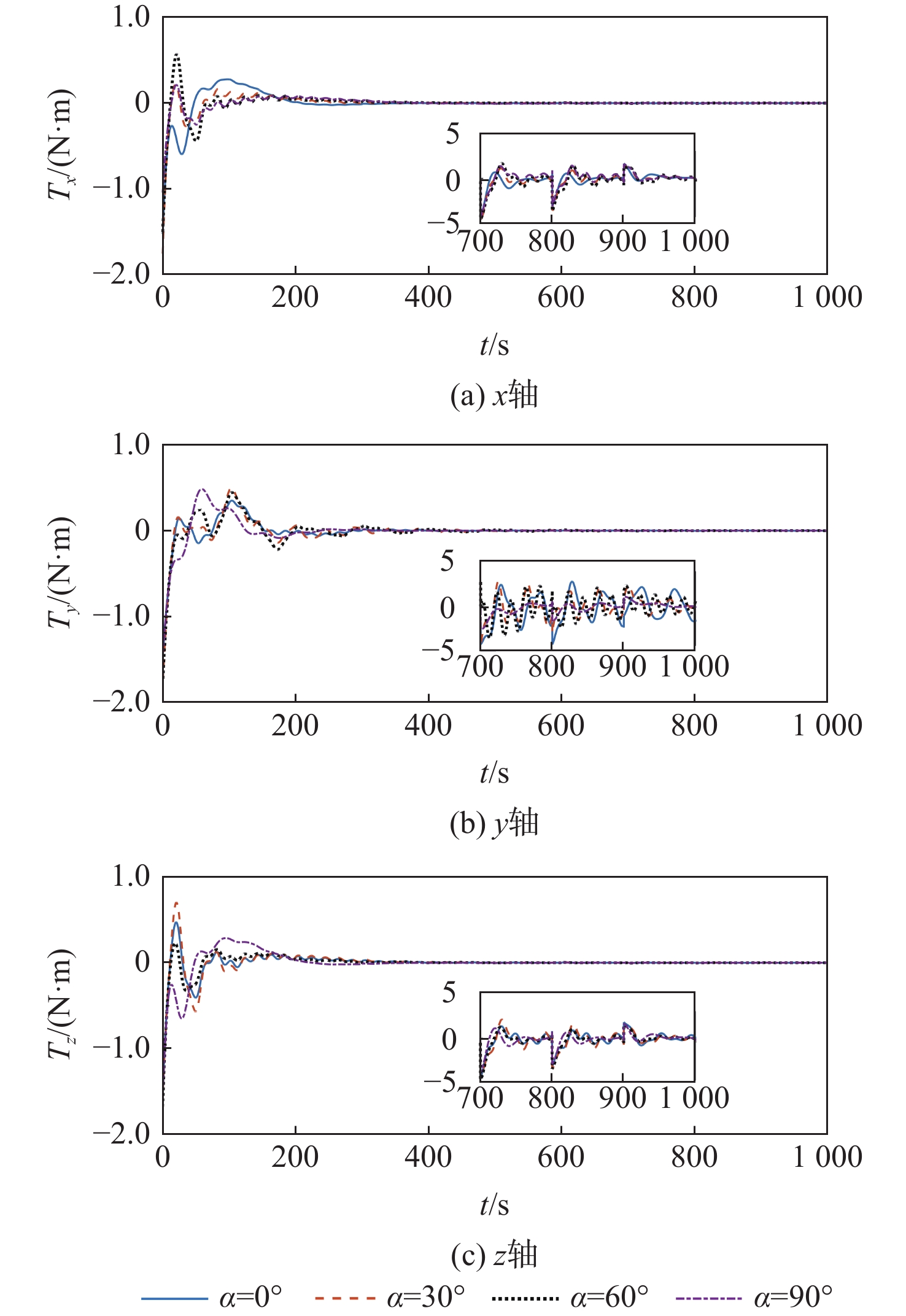
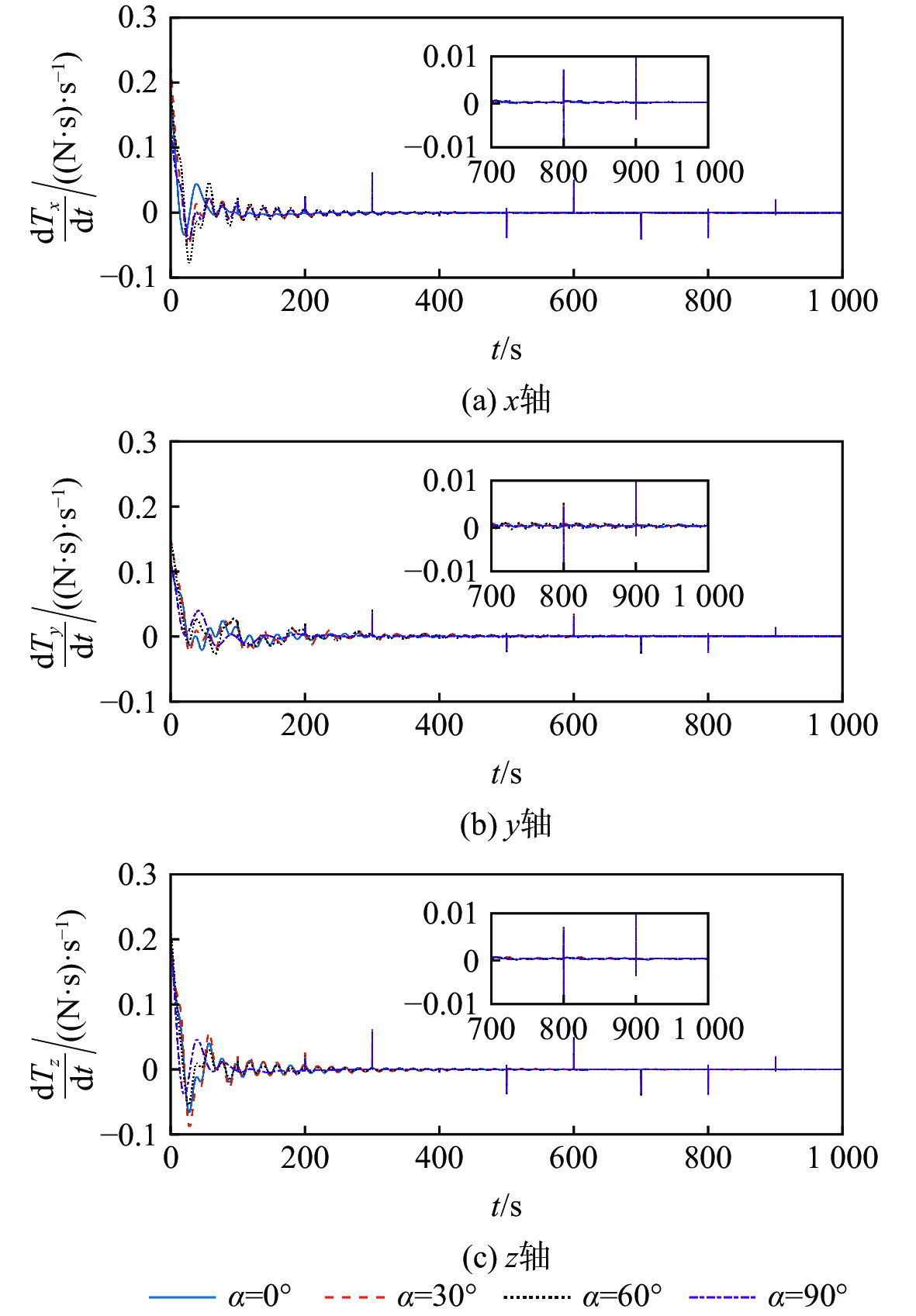
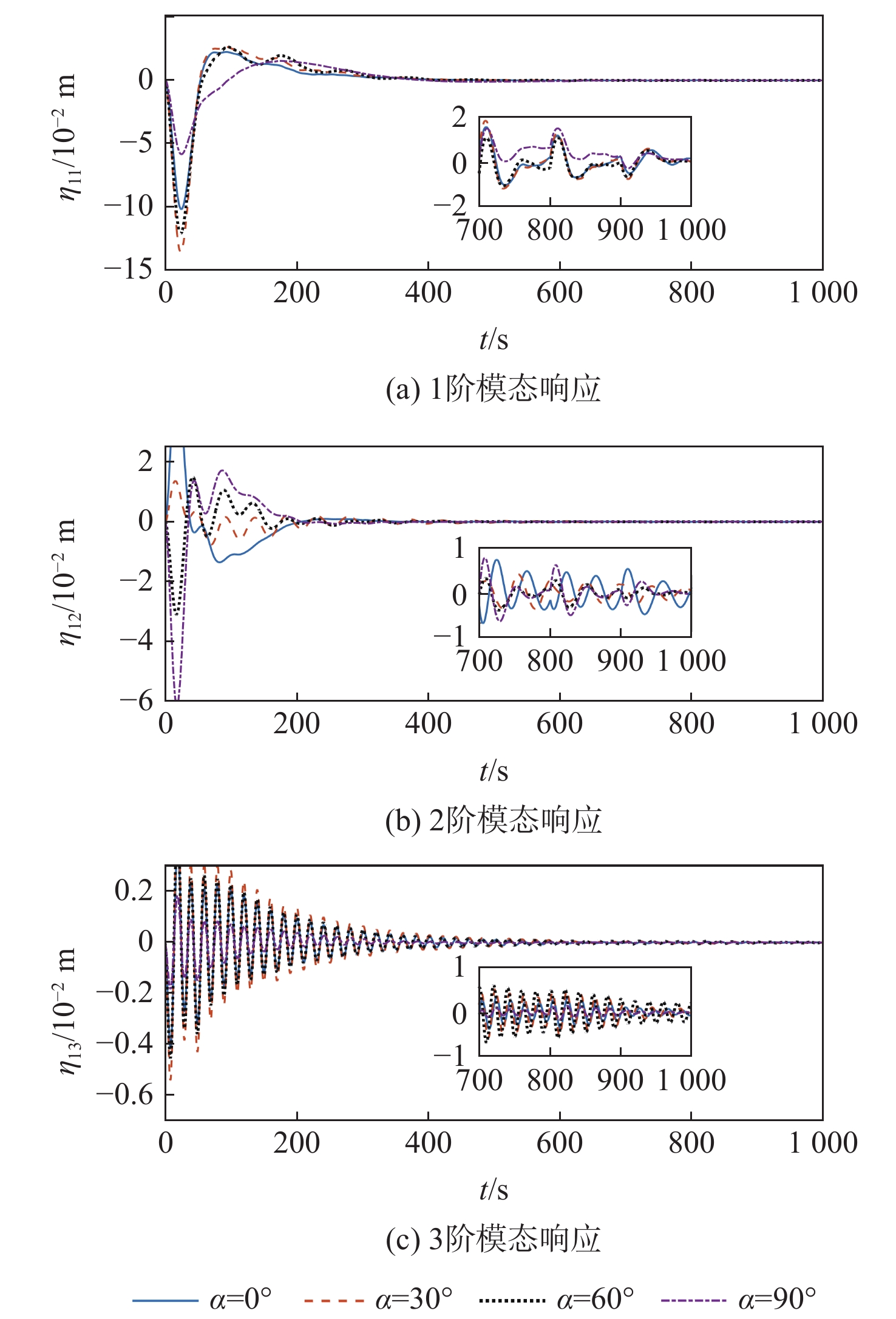
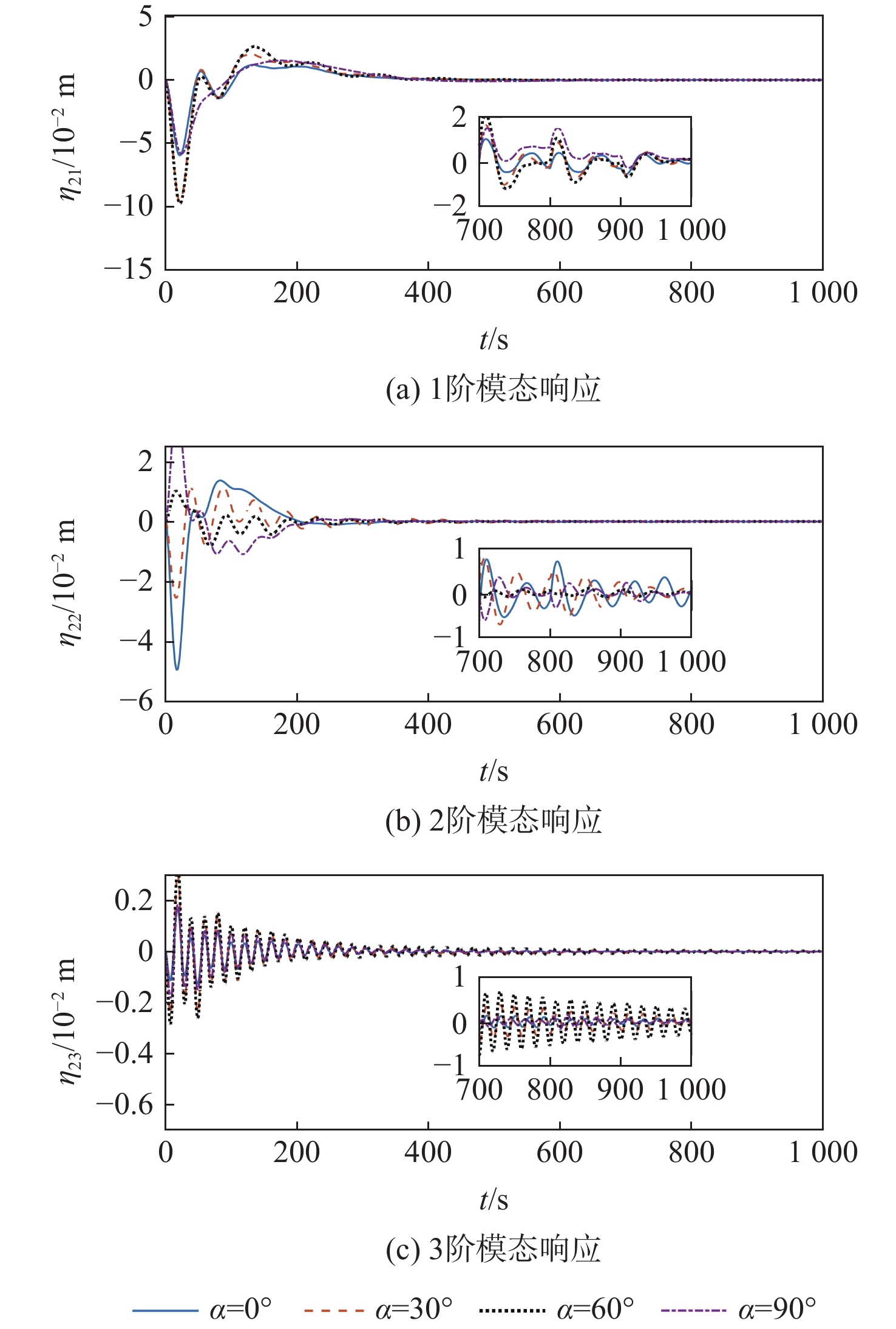
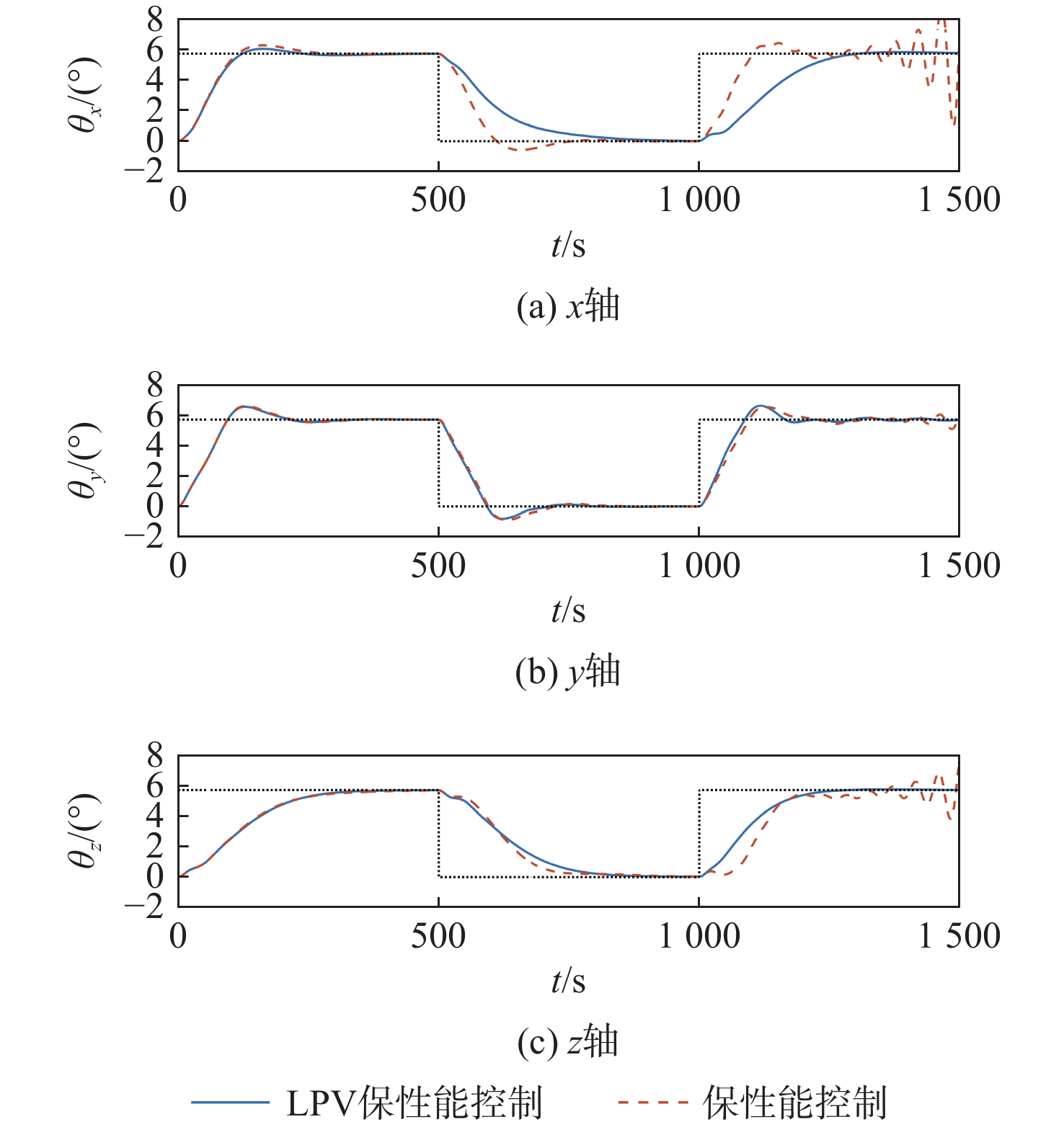
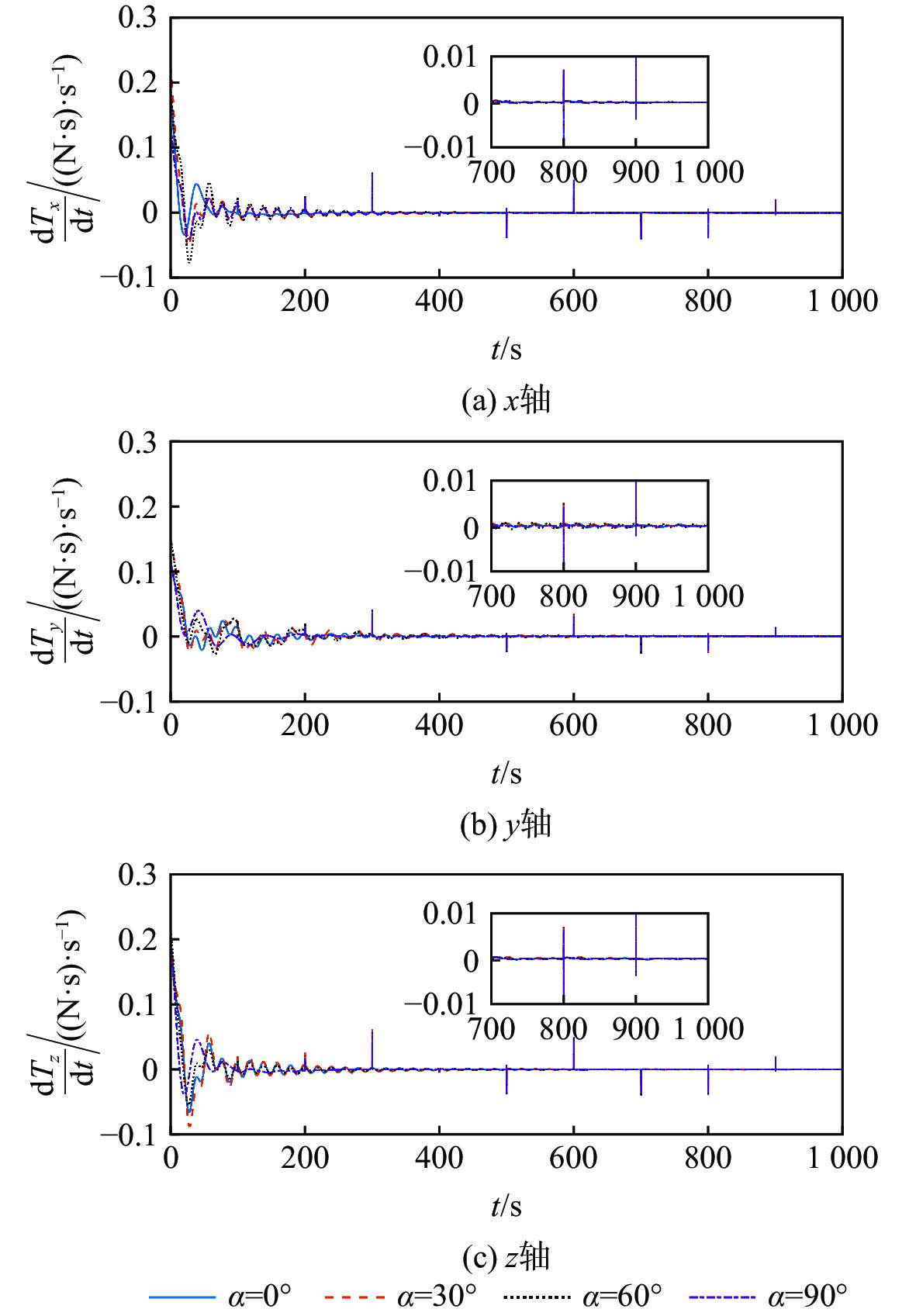
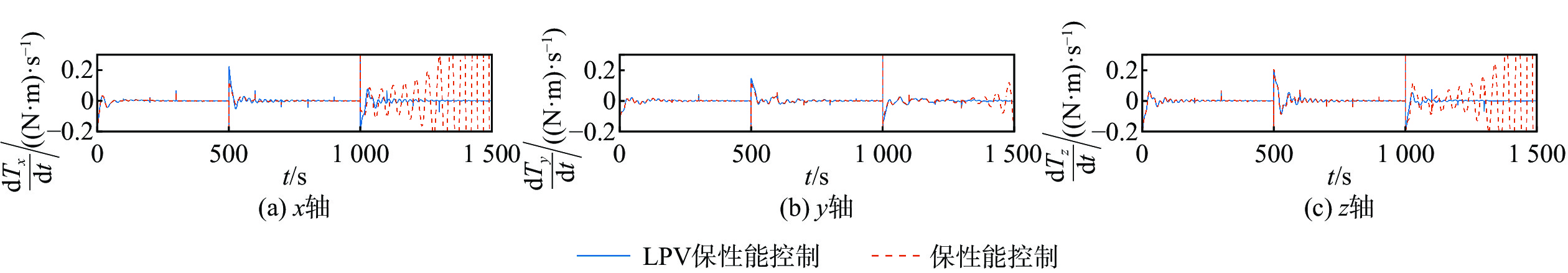
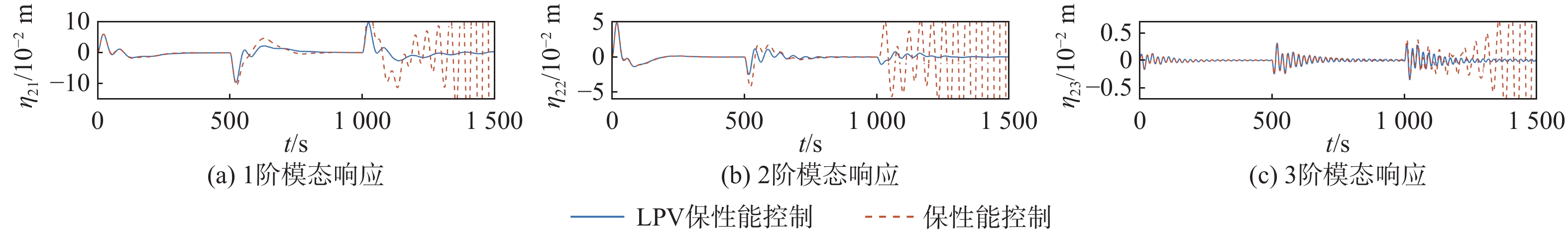
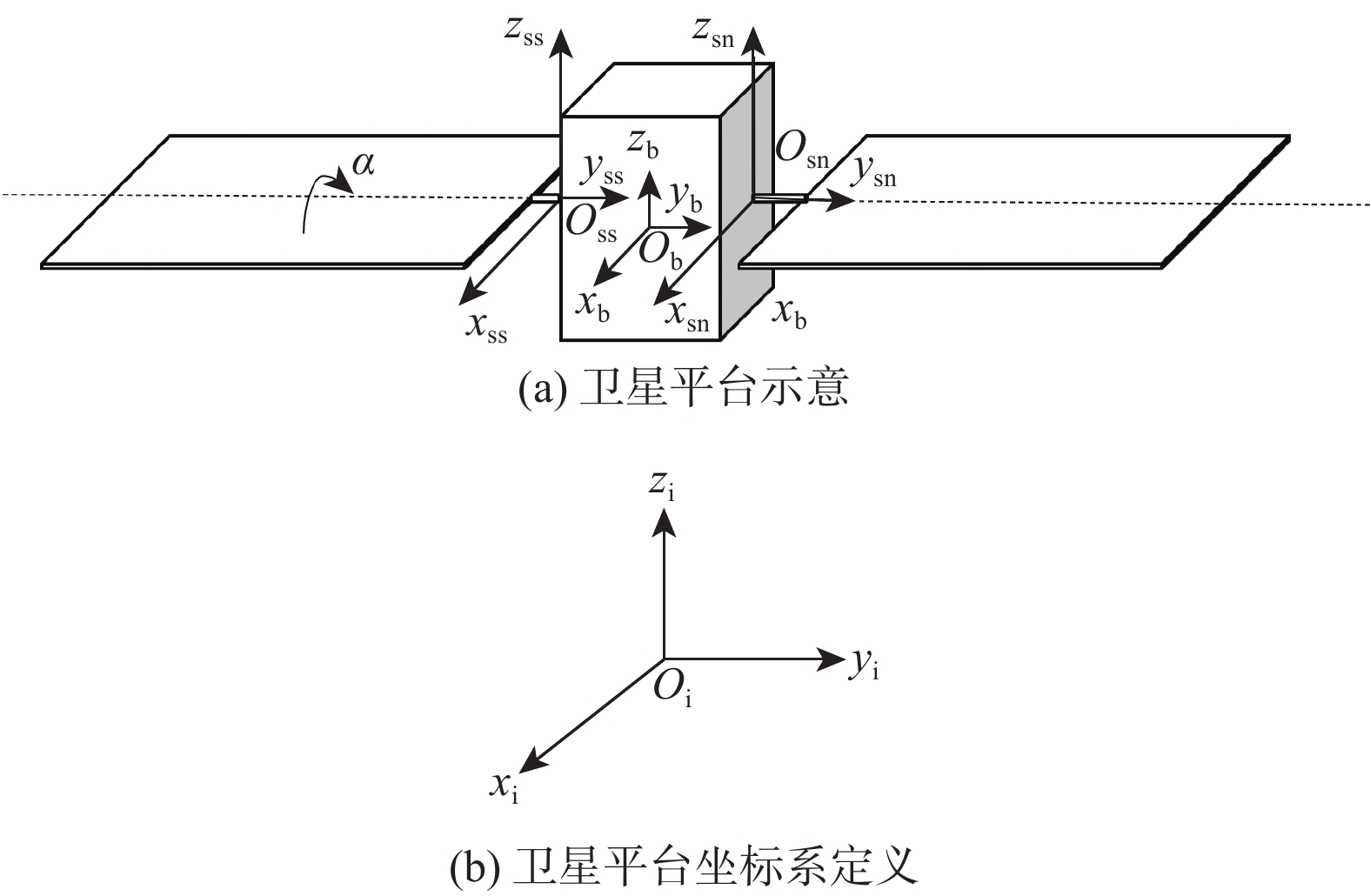
针对带转动挠性帆板航天器的动力学参数存在不确定性的问题，提出一种利用线性变参数理论(LPV)的鲁棒保性能姿态控制器设计方法。以太阳帆板转角作为变参数，建立挠性航天器姿态动力学LPV模型。考虑输入受限及输入信号变化率受限的约束，并将二次型控制指标上限最优化，通过线性矩阵不等式(LMI)方法求解得到随变参数调度的LPV状态反馈控制器，所求得的控制器能够在系统参数大范围变化的情况下保持鲁棒稳定性，并具备抗干扰能力。用数值方法进行仿真验证，并与定常鲁棒保性能控制器相比较，验证了所提出的LPV鲁棒保性能控制器的适应性与鲁棒性。
Abstract:The dynamic parameters of spacecraft with rotating flexible panels are uncertain. In view of this problem, a robust attitude controller design method based on linear parameter varying (LPV) theory was proposed. Firstly, the LPV model of attitude dynamics of flexible spacecraft was established by taking the angles of rotations of solar panels as the variable parameter. The limited input and rates of change of input signals were considered, and the quadratic control indicator limit was optimized. Based on the linear matrix inequality (LMI) method, An LPV state feedback controller with variable parameter scheduling was obtained. The obtained controller can maintain robust stability under the condition of a wide range of system parameters and has anti-interference ability. Finally, the simulation was verified by numerical method and compared with the steady robust controller. The adaptability and robustness of the proposed LPV robust controller were verified.
-
图 4 算例1航天器的控制力矩变化率
Figure 4. Rates of change of control torques of spacecraft in condition 1
图 9 算例2航天器的控制力矩变化率
Figure 9. Rates of change of control torques of spacecraft in condition 2
-
[1] SHAMMA J S, ATHANS M. Guaranteed properties of gain scheduled control for linear parameter-varying plants[J]. Automatica, 1991, 27(3): 559-564. doi: 10.1016/0005-1098(91)90116-J [2] GAHINET P, APKARIAN P. A linear matrix inequality approach to H∞ control[J]. International Journal of Robust and Nonlinear Control, 1994, 4(4): 421-448. doi: 10.1002/rnc.4590040403 [3] APKARIAN P, ADAMS R J. Advanced gain-scheduling techniques for uncertain systems[C]// IEEE Transactions on Control Systems Technology. Piscataway: IEEE Press, 1998: 21-32. [4] LIM S. Analysis and control of linear-parameter-varying systems[M]. Stanford: Stanford University, 1999. [5] DABIRI A, KULCSÁR B, KÖROĞLU H. Distributed LPV state-feedback control under control input saturation[J]. IEEE Transactions on Automatic Control, 2017, 62(5): 2450-2456. doi: 10.1109/TAC.2016.2598967 [6] WU H M, TAFRESHI R. Air–fuel ratio control of lean-burn SI engines using the LPV-based fuzzy technique[J]. IET Control Theory & Applications, 2018, 12(10): 1414-1420. [7] CASTRO R, SALTON A, FLORES J, et al. Variable frequency resonant controller for load reduction in wind turbines[J]. Control Engineering Practice, 2017, 66: 76-88. doi: 10.1016/j.conengprac.2017.06.007 [8] SCHAAB K, HAHN J, WOLKOV M, et al. Robust control for voltage and transient stability of power grids relying on wind power[J]. Control Engineering Practice, 2017, 60: 7-17. doi: 10.1016/j.conengprac.2016.12.003 [9] WEISS Y, ALLERHAND L I, AROGETI S. Yaw stability control for a rear double-driven electric vehicle using LPV-H∞ methods[J]. Science China (Information Sciences), 2018, 61(7): 78-90. [10] BLOEMERS T, OOMEN T, TOTH R. Frequency response data-based LPV controller synthesis applied to a control moment gyroscope[J]. IEEE Transactions on Control Systems Technology, 2022, 30(6): 2734-2742. doi: 10.1109/TCST.2022.3152626 [11] OLIVEIRA L A L, BARBOSA M V C, SILVA L F P, et al. Exponential stabilization of LPV systems under magnitude and rate saturating actuators[J]. IEEE Control Systems Letters, 2021, 6: 1418-1423. [12] SHIMOMURA T, OGURA H, KANATA S. Attitude control of a spacecraft as an LPV model using the ILQ method[J]. Transactions of the Japan Society For Aeronautical and Space Sciences, Aerospace Technology Japan, 2020, 18(6): 344-349. doi: 10.2322/tastj.18.344 [13] NEMATI F, SAFAVI HAMAMI S M, ZEMOUCHE A. A nonlinear observer-based approach to fault detection, isolation and estimation for satellite formation flight application[J]. Automatica, 2019, 107: 474-482. doi: 10.1016/j.automatica.2019.06.007 [14] JIN R Y, CHEN X Q, GENG Y H, et al. LPV gain-scheduled attitude control for satellite with time-varying inertia[J]. Aerospace Science and Technology, 2018, 80: 424-432. doi: 10.1016/j.ast.2018.07.020 [15] 金荣玉. 基于LPV模型的卫星姿态容错控制[D]. 哈尔滨: 哈尔滨工业大学, 2017.JIN R Y. Fault-tolerant control of satellite attitude based on LPV model[D]. Harbin: Harbin Institute of Technology, 2017 (in Chinese). [16] 李立涛, 荣思远. 航天器姿态动力学与控制[M]. 哈尔滨: 哈尔滨工业大学出版社, 2019.LI L T, RONG S Y. Attitude dynamics and control of spacecraft[M]. Harbin: Harbin Institute of Technology Press, 2019 (in Chinese). [17] 俞立. 鲁棒控制: 线性矩阵不等式处理方法[M]. 北京: 清华大学出版社, 2002.YU L. Robust control: A method to deal with linear matrix inequalities[M]. Beijing: Tsinghua University Press, 2002 (in Chinese). [18] 庞辉, 王延, 刘凡. 考虑参数不确定性的主动悬架H2/H∞保性能控制[J]. 控制与决策, 2019, 34(3): 470-478.PANG H, WANG Y, LIU F. H2/H∞ guaranteed cost control for active suspensions considering parameter uncertainty[J]. Control and Decision, 2019, 34(3): 470-478 (in Chinese). [19] 刘康志, 姚郁. 线性鲁棒控制[M]. 北京: 科学出版社, 2013.LIU K Z, YAO Y. Linear robust control[M]. Beijing: Science Press, 2013 (in Chinese). [20] 于启学. 输入与状态受限的线性系统控制[D]. 南京: 东南大学, 2002.YU Q X. Control of linear systems with input and state constraints[D]. Nanjing: Southeast University, 2002 (in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 162
- HTML全文浏览量: 83
- PDF下载量: 0
- 被引次数: 0
