操纵方式对翼伞系统雀降性能的影响

程杨; 仇博文; 余莉; 李岩军; 曹皓然

doi:10.13700/j.bh.1001-5965.2022.0914

操纵方式对翼伞系统雀降性能的影响

doi: 10.13700/j.bh.1001-5965.2022.0914

程杨¹,
仇博文¹,
余莉^{1, 2, ,},
李岩军^{1, 2},
曹皓然¹

1.
南京航空航天大学飞行器环境控制与生命保障工业和信息化部重点实验室，南京 210016
2.
南京航空航天大学航空学院，南京 210016

基金项目: 国家自然科学基金(11972192)

详细信息

通讯作者:
E-mail：yuli_happy@163.com

中图分类号: V224.2
计量
- 文章访问数: 155
- HTML全文浏览量: 89
- PDF下载量: 0
- 被引次数: 0
出版历程
- 收稿日期: 2022-11-10
- 录用日期: 2022-12-19
- 网络出版日期: 2023-01-03
- 整期出版日期: 2024-12-31

Influence of maneuvering modes on flared landing performance of parafoil system

CHENG Yang¹,
QIU Bowen¹,
YU Li^{1, 2
, ,},
LI Yanjun^{1, 2},
CAO Haoran¹

1.
Key Laboratory of Aircraft Environment Control and Life Support，Ministry of Industry and Information Technology，Nanjing University of Aeronautics and Astronautics，Nanjing 210016，China
2.
College of Aerospace Engineering，Nanjing University of Aeronautics and Astronautics，Nanjing 210016，China

Funds: National Natural Science Foundation of China (11972192)

More Information

Corresponding author: E-mail：yuli_happy@163.com

摘要

摘要:
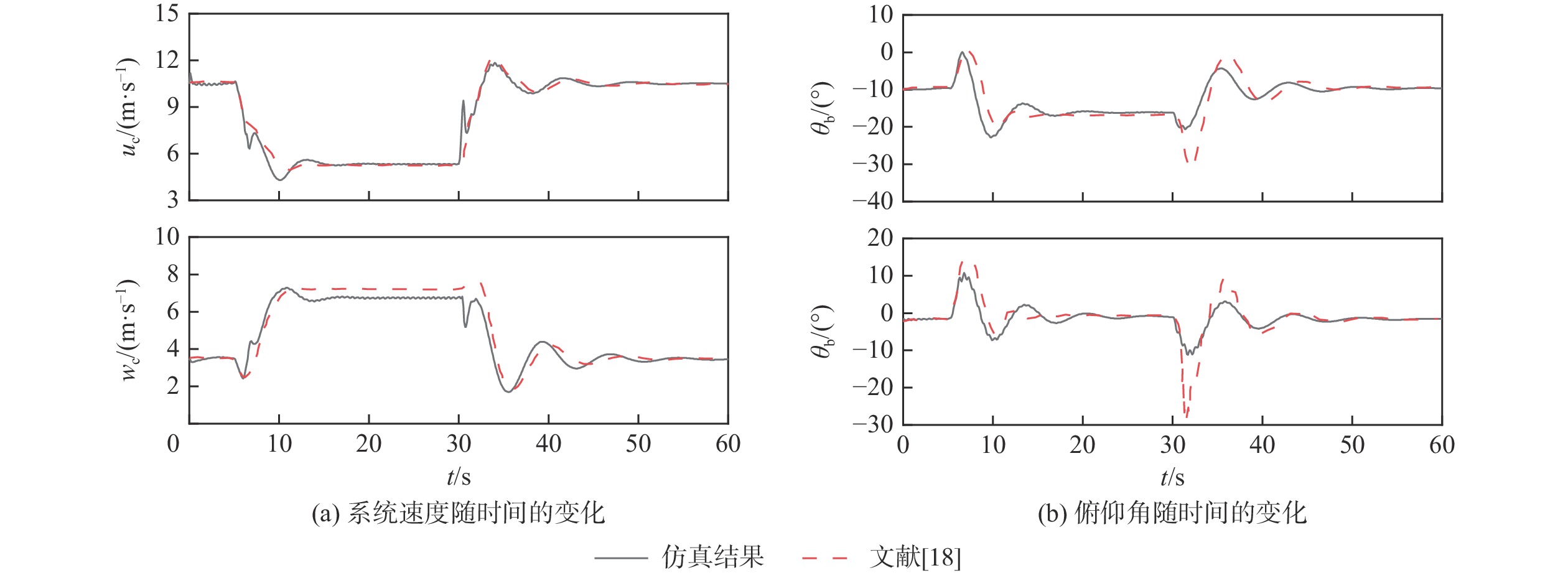
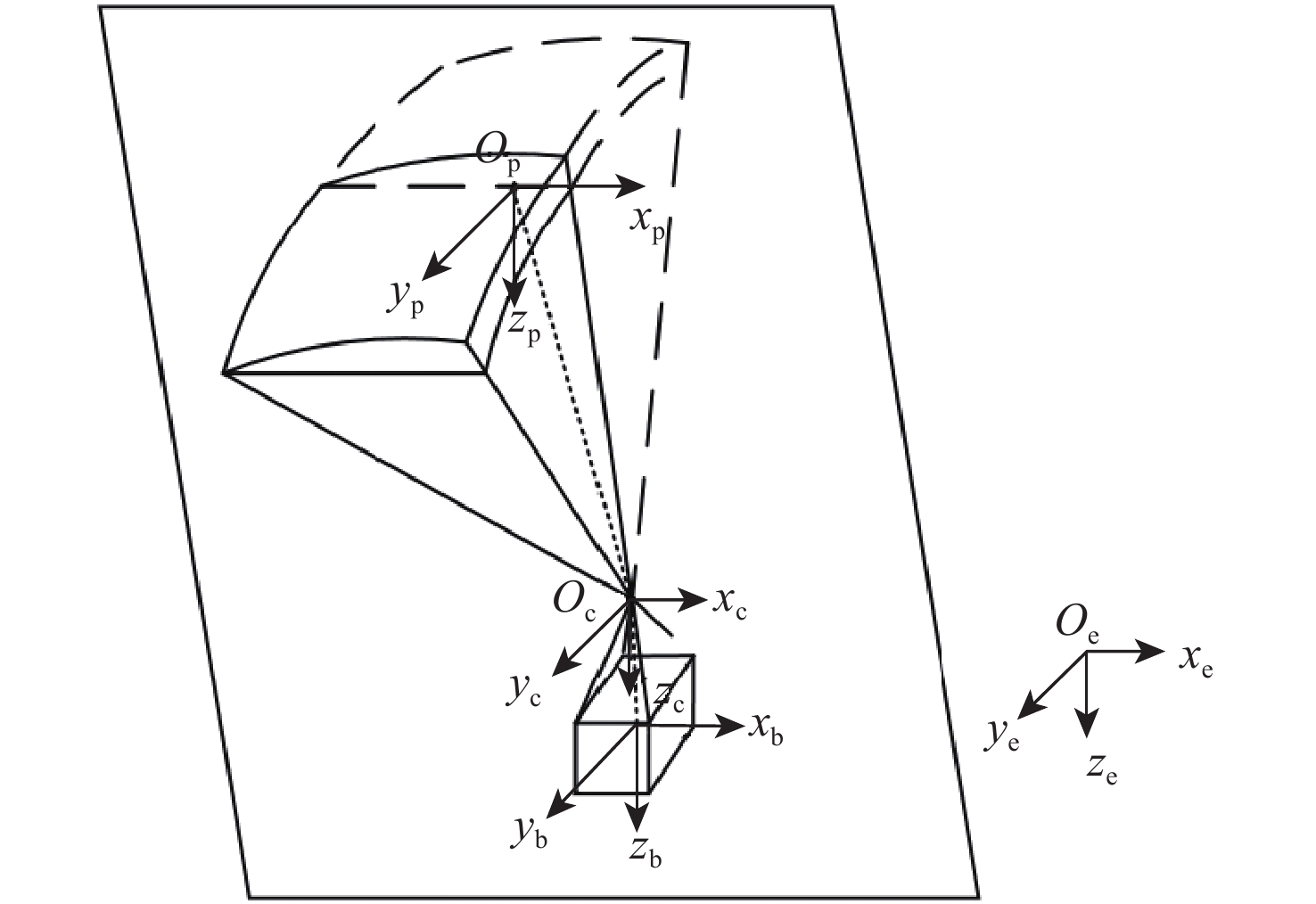
为获得操纵方式对翼伞系统雀降性能的影响规律，建立了翼伞系统两体9自由度动力学模型，采用龙格库塔法进行了动力学编程计算，系统速度、翼伞及载荷姿态的数值结果和文献规律一致，最大误差为8.2%。开展了分级操纵及操纵速度对翼伞系统雀降性能的影响研究，得到了翼伞最小水平速度、最小垂直速度及最大迎角的变化规律。研究结果表明：一次操纵的雀降性能优于分级操纵；操纵时间过长或过短均会导致垂直速度增加；采用“先快后慢”和“匀速下拉”均能保证良好的雀降性能，操纵速度的选择应同时考虑最小垂直速度及翼伞飞行的稳定性。
- 翼伞 /
- 雀降性能 /
- 9自由度模型 /
- 操纵方式 /
- 操纵速度
Abstract:
A two-body 9-degree of freedom (DOF) dynamics model of the parafoil system was established, and the Runge-Kutta method was used for dynamic programming calculation. The numerical results of the system velocities and parafoil and load attitudes were consistent with the results in the literature, and the maximum error was 8.2%. On this basis, the influence of hierarchical maneuvering and maneuvering velocity on the flared landing performance of the parafoil system was investigated. The variations of minimum horizontal velocity, minimum vertical velocity, and maximum angle of attack of parafoil were obtained. The results show that the flared landing performance under one-time maneuvering is better than that under hierarchical maneuvering. If the maneuvering duration is too long or too short, the vertical velocity will increase. Both maneuverings featuring first accelerating and then decelerating and constant velocity can ensure good flared landing performance. The minimum vertical velocity and the flight stability of the parafoil should both be considered in the selection of the maneuvering velocity.
- parafoil /
- flared landing performance /
- 9-DOF model /
- maneuvering modes /
- maneuvering velocity

HTML全文

图 1 翼伞系统基本坐标系示意图

Figure 1. Basic coordinate system of parafoil system

下载: 全尺寸图片幻灯片

图 2 翼伞气动特性曲线

Figure 2. Aerodynamic characteristic curves of parafoil

下载: 全尺寸图片幻灯片

图 3 模型和文献结果对比

Figure 3. Comparison of model and results in literature

下载: 全尺寸图片幻灯片

图 4 分级操纵工况

Figure 4. Hierarchical maneuvering conditions

下载: 全尺寸图片幻灯片

图 5 不同分级操纵制动量下翼伞水平速度变化

Figure 5. Variation of horizontal velocity of parafoil under different hierarchical maneuvering conditions

下载: 全尺寸图片幻灯片

图 6 不同分级操纵制动量下翼伞垂直速度变化

Figure 6. Variation of vertical velocity of parafoil under different hierarchical maneuvering conditions

下载: 全尺寸图片幻灯片

图 7 不同分级操纵制动量下翼伞迎角变化

Figure 7. Variation of angle of attack of parafoil under different hierarchical maneuvering conditions

下载: 全尺寸图片幻灯片

图 8 匀速下拉时翼伞速度、攻角最值随操纵时长变化

Figure 8. Variation of velocity and angle of attack of parafoil with maneuvering durations under constant velocity

下载: 全尺寸图片幻灯片

图 9 变速下拉时操纵速度变化率对翼伞速度、攻角最值的影响

Figure 9. Influence of variation of maneuvering velocity on velocity and angle of attack of parafoil under changin velocity

下载: 全尺寸图片幻灯片

表 1 翼伞系统结构参数

Table 1. Structural parameters of parafoil system

展长 b/m	弦长 c/m	切口高度 e/m	翼伞质量 m_p/kg	载荷质量 m_b/kg	伞衣有效面积S_p/m²	载荷有效面积S_b/m²	翼伞中心与系统质心距离R_p/m	载荷中心与系统质心距离R_b/m	安装角 μ/(°)
7.5	3.75	0.675	5	135	28	0.5	−7.5	0.5	9

下载: 导出CSV

表 2 初始运动状态参数

Table 2. Initial motion state parameters

（u_c,v_c,w_c）/（m·s⁻¹）	（${\varphi _{\mathrm{p}}},{\theta _{\mathrm{p}}},{\phi _{\mathrm{p}}}$）/(°)	(x,y,z)/m
(10.5,0,3.5)	(0,−10,0)	（0,0,−1000）

下载: 导出CSV

表 3 本文和文献翼伞系统稳定运动特性对比

Table 3. Comparison of stable motion characteristics of parafoil systems in this paper and in literature

运动阶段	u_c/（m·s⁻¹）		u_c本文相对文献误差/%	w_c/（m·s⁻¹）		w_c本文相对文献误差/%	滑翔比		滑翔比本文相对文献误差/%
运动阶段	文献	本文	u_c本文相对文献误差/%	文献	本文	w_c本文相对文献误差/%	文献	本文	滑翔比本文相对文献误差/%
滑翔阶段（0～5 s, 50～60 s）	10.50	10.52	0.2	3.50	3.53	0.9	3.00	2.98	0.7
雀降阶段（20～30 s）	5.25	5.31	1.1	7.20	6.75	6.3	0.73	0.79	8.2

下载: 导出CSV

表 4 不同操纵方式下翼伞速度、迎角最值

Table 4. Extreme values of velocity and angle of attack of parafoil under different maneuvering conditions

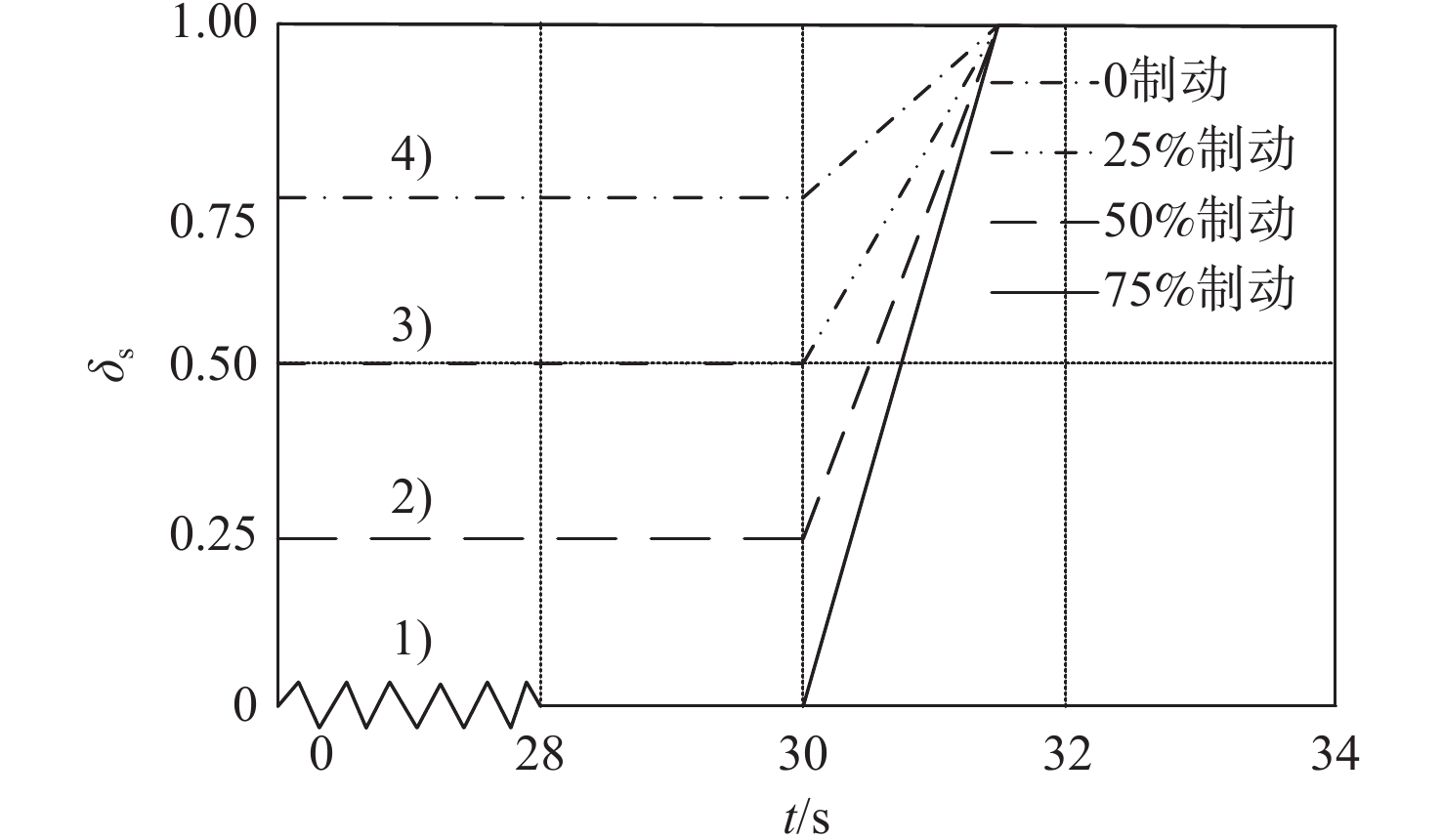
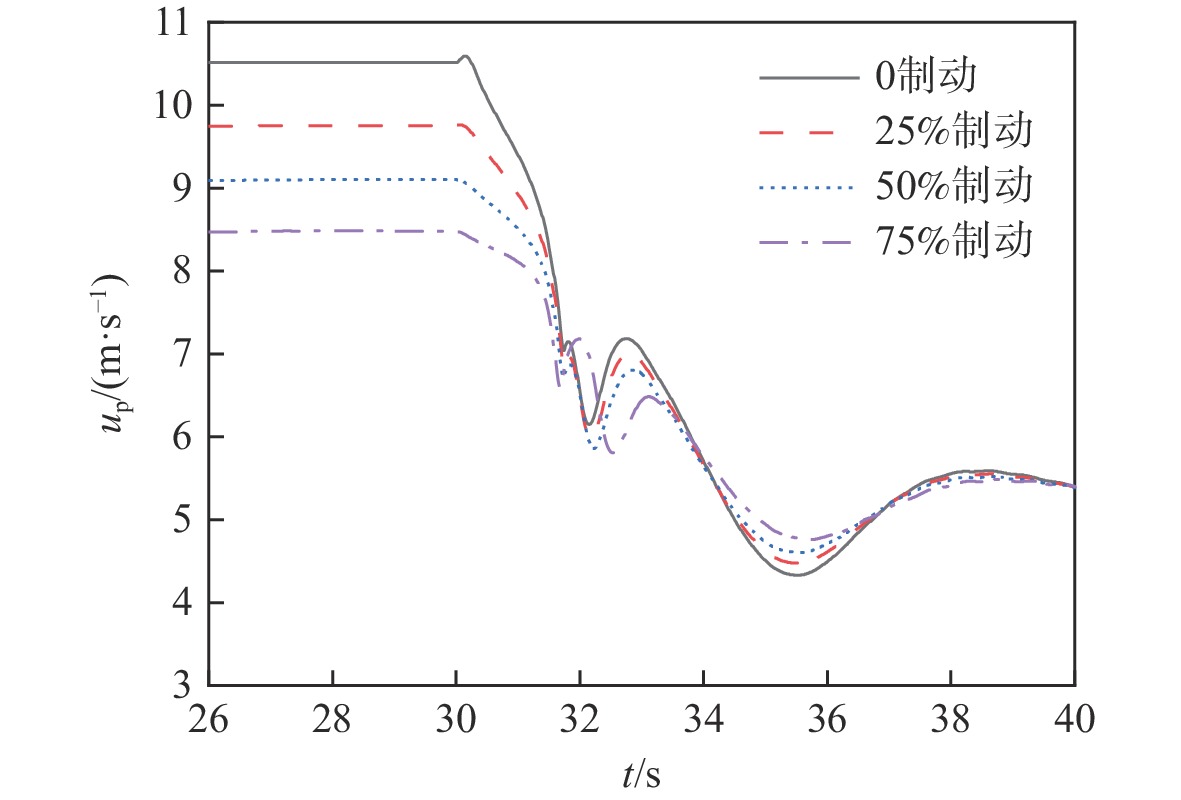
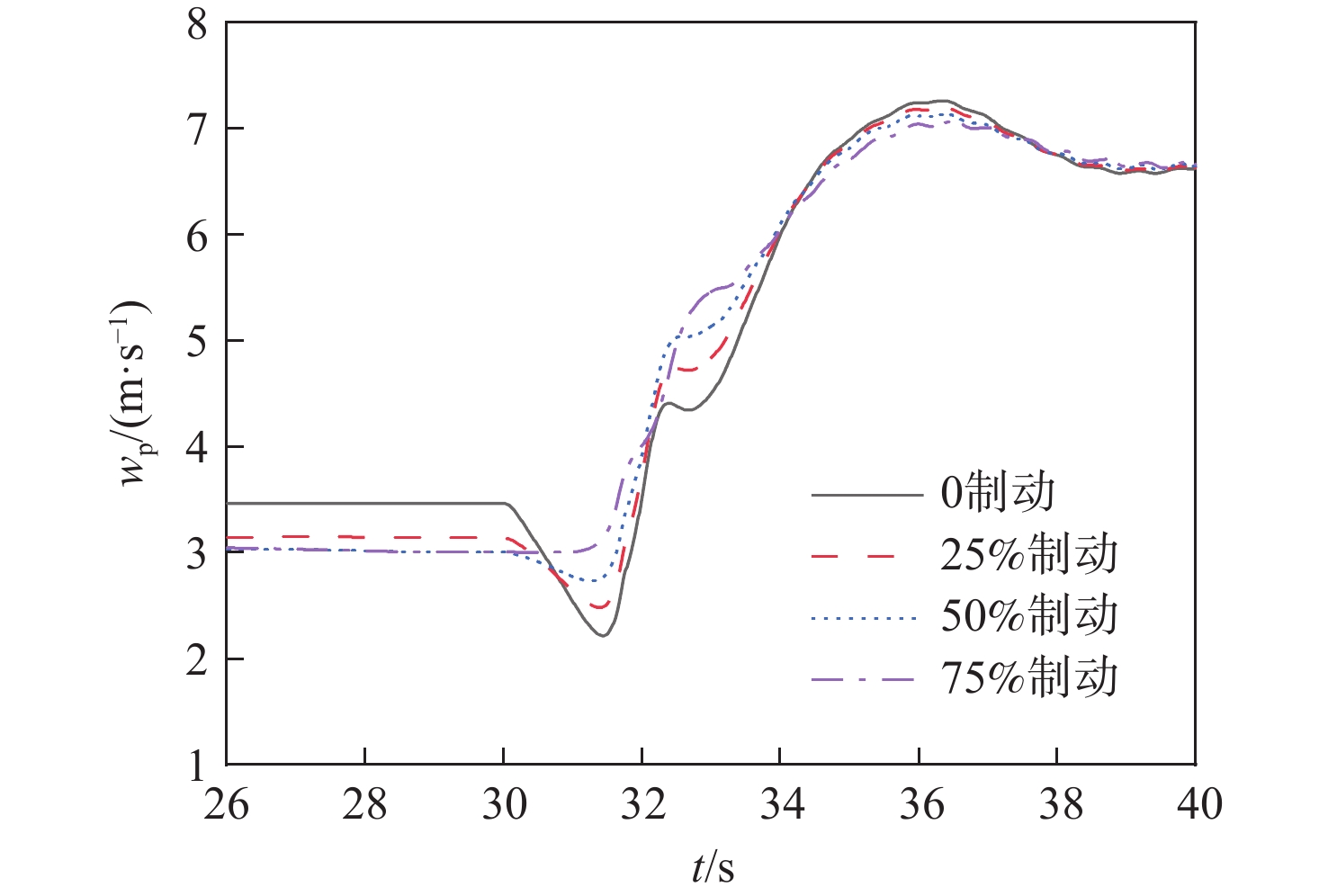
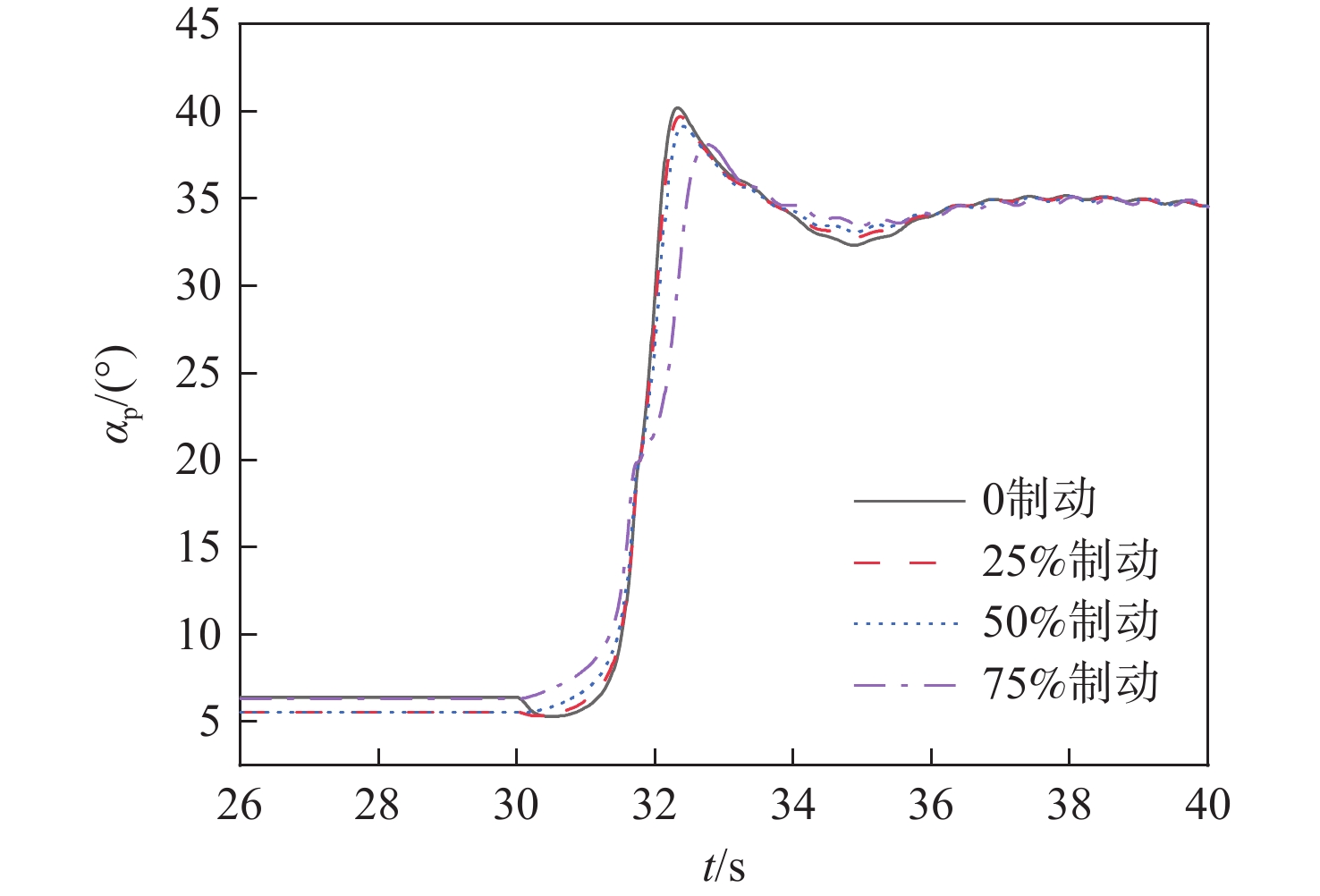
操纵方式	$ {u_{\min }} $/（m·s⁻¹）	$ {w_{\min }} $/（m·s⁻¹）	$ {\alpha _{\max }} $/(°)
0制动（一次操纵）	4.33	2.22	40.18
25%制动	4.48	2.48	39.69
50%制动	4.6	2.73	39.14
75%制动	4.76	3	38.07

下载: 导出CSV

表 5 操纵方式对比工况

Table 5. Comparison of maneuvering conditions

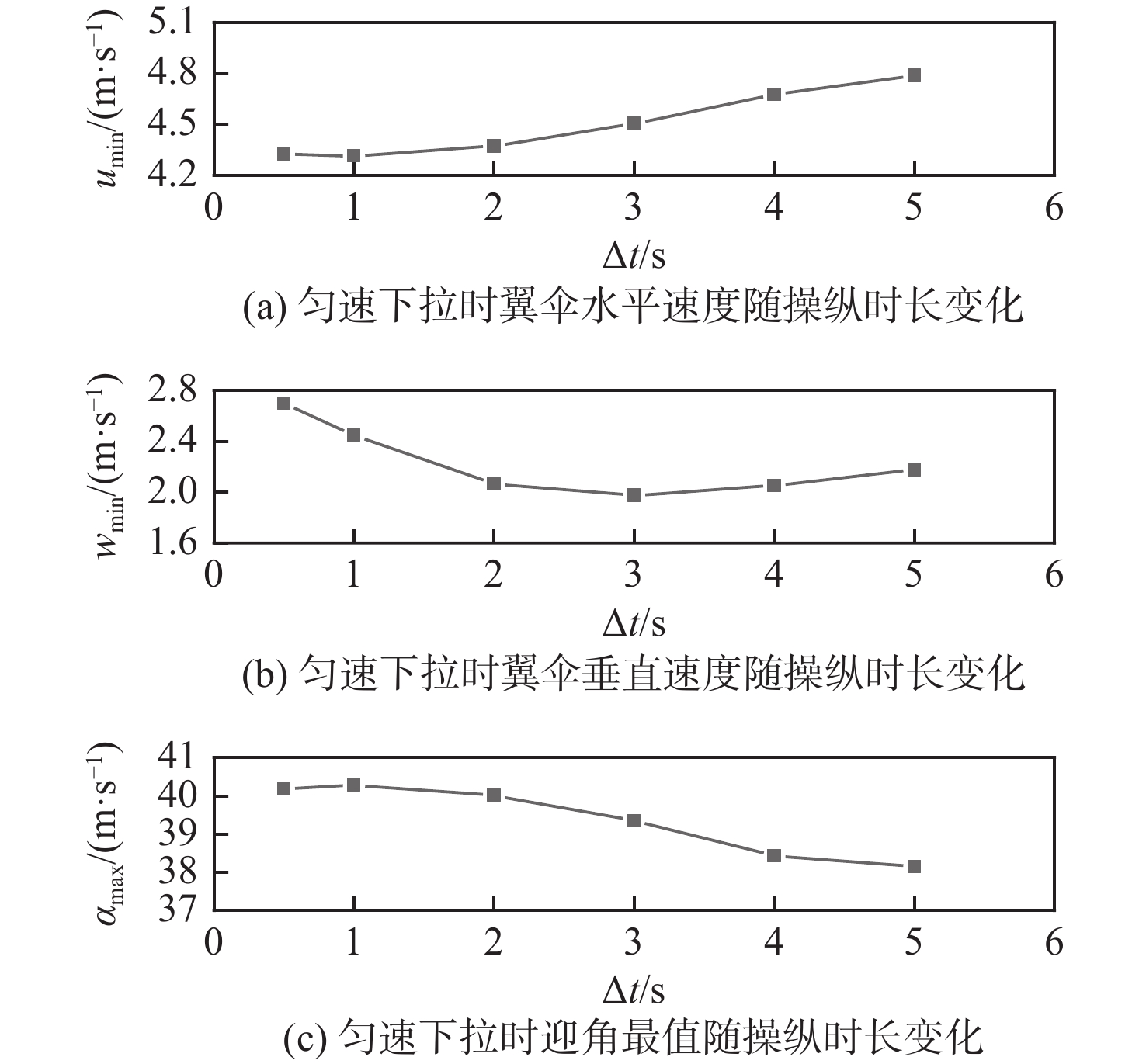
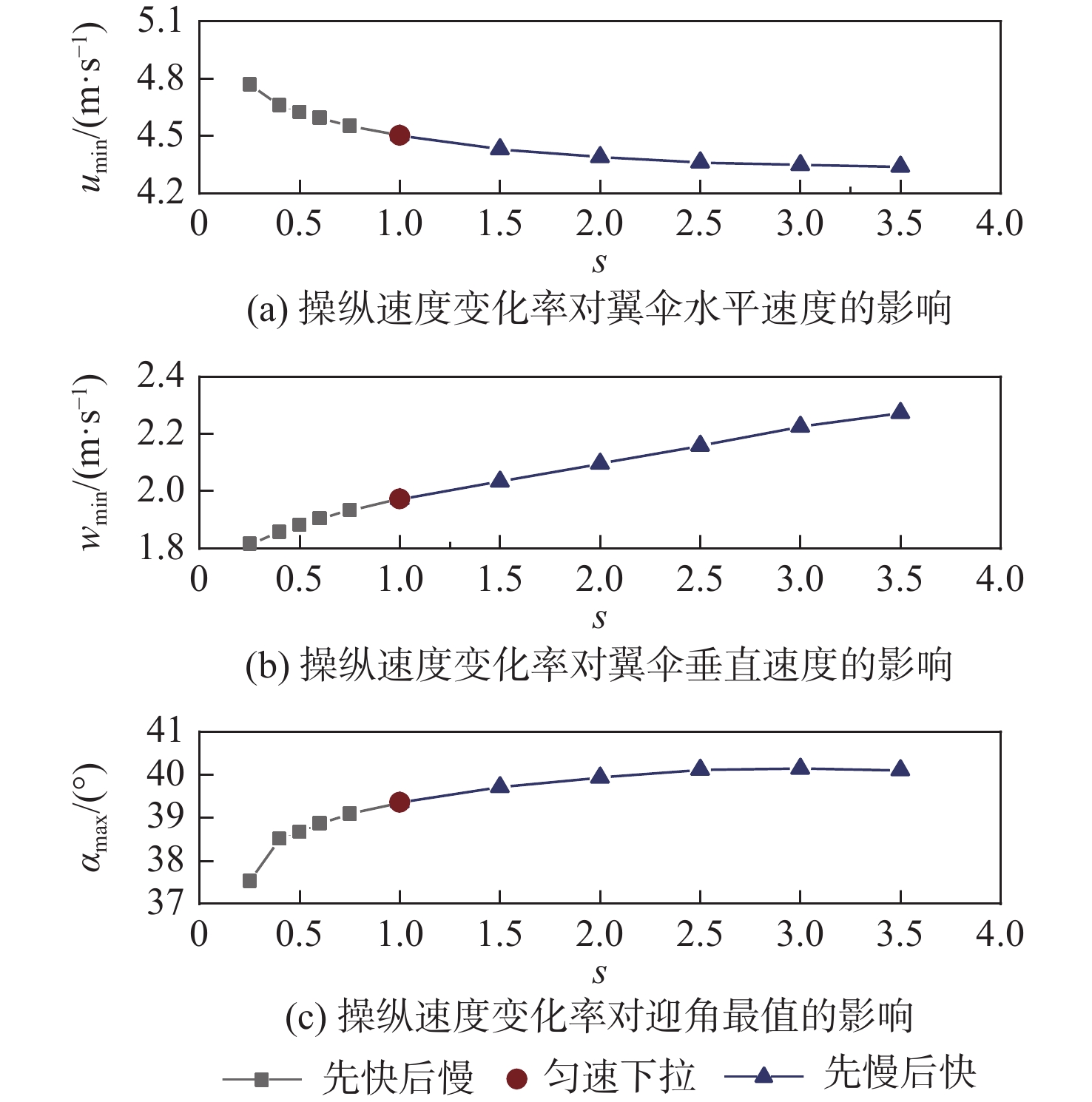
操纵方式	$\Delta t$/s	s
匀速下拉	0.5，1，2，3，4，5	1
先快后慢	3	0.25，0.4，0.5，0.6，0.75
先慢后快	3	1.5，2，2.5，3，3.5

下载: 导出CSV

参考文献(20)

[1]	赵秋艳. 翼伞雀降技术[J]. 航天返回与遥感, 1999(2): 5-9. ZHAO Q Y. Parafoil bird landing technology[J]. Space Return and Remote Sensing, 1999(2): 5-9 (in Chinese).
[2]	王利荣. 降落伞理论与应用[M]. 北京:宇航出版社, 1997: 528-530. WANG L R. Parachute theory and application[M]. Beijing: Aerospace Press, 1997: 528-530 (in Chinese).
[3]	赵培尧. 单层翼伞回收末段飞行特性研究[D]. 北京: 北京理工大学, 2017: 59-63. ZHAO P Y. Study on flight characteristics of recovery terminal of single-layer parafoil[D]. Beijing: Beijing Institute of Technology, 2017: 59-63 (in Chinese).
[4]	余莉. 气动减速技术[M]. 北京: 科学出版社, 2018: 273-276. YU L. Aerodynamic deceleration technology[M]. Beijing: Science Press, 2018: 273-276 (in Chinese).
[5]	SLEGERS N, GORMAN C. Comparison and analysis of multi-body parafoil models with varying degrees of freedom[C]// 21st AIAA Aerodynamic Decelerator Systems Technology Conference and Seminar. Reston: AIAA, 2011: 2615.
[6]	TAN P L, SUN M W, SUN Q L, et al. Dynamic modeling and experimental verification of powered parafoil with two suspending points[J]. IEEE Access, 2020, 8: 12955-12966. doi: 10.1109/ACCESS.2020.2965541
[7]	GOODRICK T. Simulation studies of the flight dynamics of gliding parachute systems[C]// 6th Aerodynamic Decelerator and Balloon Technology Conference. Reston: AIAA, 1979: 417.
[8]	GOODRICK T. Comparison of simulation and experimental data for a gliding parachute in dynamic flight[C]// 7th Aerodynamic Decelerator and Balloon Technology Conference. Reston: AIAA, 1981: 1924.
[9]	常冠清, 张泽, 仇海涛, 等. 翼伞系统动力学建模与仿真研究[J]. 导航与控制, 2016, 15(6): 33-40. CHANG G Q, ZHANG Z, QIU H T, et al. Dynamic modeling and simulation of parafoil aerial delivery system[J]. Navigation and Control, 2016, 15(6): 33-40 (in Chinese).
[10]	ZHANG Z X, ZHAO Z N, FU Y L. Dynamics analysis and simulation of six DOF parafoil system[J]. Cluster Computing, 2019, 22(5): 12669-12680.
[11]	张兴会, 朱二琳. 翼伞系统雀降性能及控制研究[J]. 航天控制, 2012, 30(1): 29-32. ZHANG X H, ZHU E L. The study of the flare-landing performance and control of parafoil system[J]. Aerospace Control, 2012, 30(1): 29-32 (in Chinese).
[12]	OCHI Y. Modeling and simulation of flight dynamics of a relative-roll-type parafoil[C]// AIAA Scitech 2020 Forum. Reston: AIAA, 2020: 1643.
[13]	GANG Y. Nine-degree of freedom modeling and flight dynamic analysis of parafoil aerial delivery system[J]. Procedia Engineering, 2015, 99: 866-872. doi: 10.1016/j.proeng.2014.12.614
[14]	SLEGERS N, COSTELLO M. Aspects of control for a parafoil and payload system[J]. Journal of Guidance, Control, and Dynamics, 2003, 26(6): 898-905. doi: 10.2514/2.6933
[15]	MORIYOSHI T, YAMADA K, NISHIDA H. The effect of rigging angle on longitudinal direction motion of parafoil-type vehicle: basic stability analysis and wind tunnel test[J/OL]. International Journal of Aerospace Engineering, 2020: 8861714 (2020-12-07)[2022-10-11]. https://doi.org/10.1155/2020/8861714.
[16]	张晓今. 航天器可控翼伞回收系统雀降性能研究[J]. 国防科技大学学报, 1996, 18(2): 5. ZHANG X J. Research on lark landing performance of spacecraft controllable parafoil recovery system[J]. Journal of National University of Defense Technology, 1996, 18(2): 5 (in Chinese).
[17]	MÜLLER S, WAGNER O, SACHS G. A high-fidelity nonlinear multibody simulation model for parafoil systems[C]// 17th AIAA Aerodynamic Decelerator Systems Technology Conference and Seminar. Reston: AIAA, 2003: 2120.
[18]	PRAKASH O, ANANTHKRISHNAN N. Modeling and simulation of 9-DOF parafoil-payload system flight dynamics[C]// AIAA Atmospheric Flight Mechanics Conference and Exhibit. Reston: AIAA, 2006: 6130.
[19]	VISHNIAK A. Simulation of the payload-parachute-wing system flight dynamics[C]// Aerospace Design Conference. Reston: AIAA, 1993: 1250.
[20]	PRAKASH O, DAFTARY A, ANANTHKRISHNAN N. Trim and stability analysis of parafoil/payload system using bifurcation methods[C]// 18th AIAA Aerodynamic Decelerator Systems Technology Conference and Seminar. Reston: AIAA, 2005: 1666.