Hysteresis characteristics of pneumatic artificial muscle based on improved Maxwell model
-
摘要:
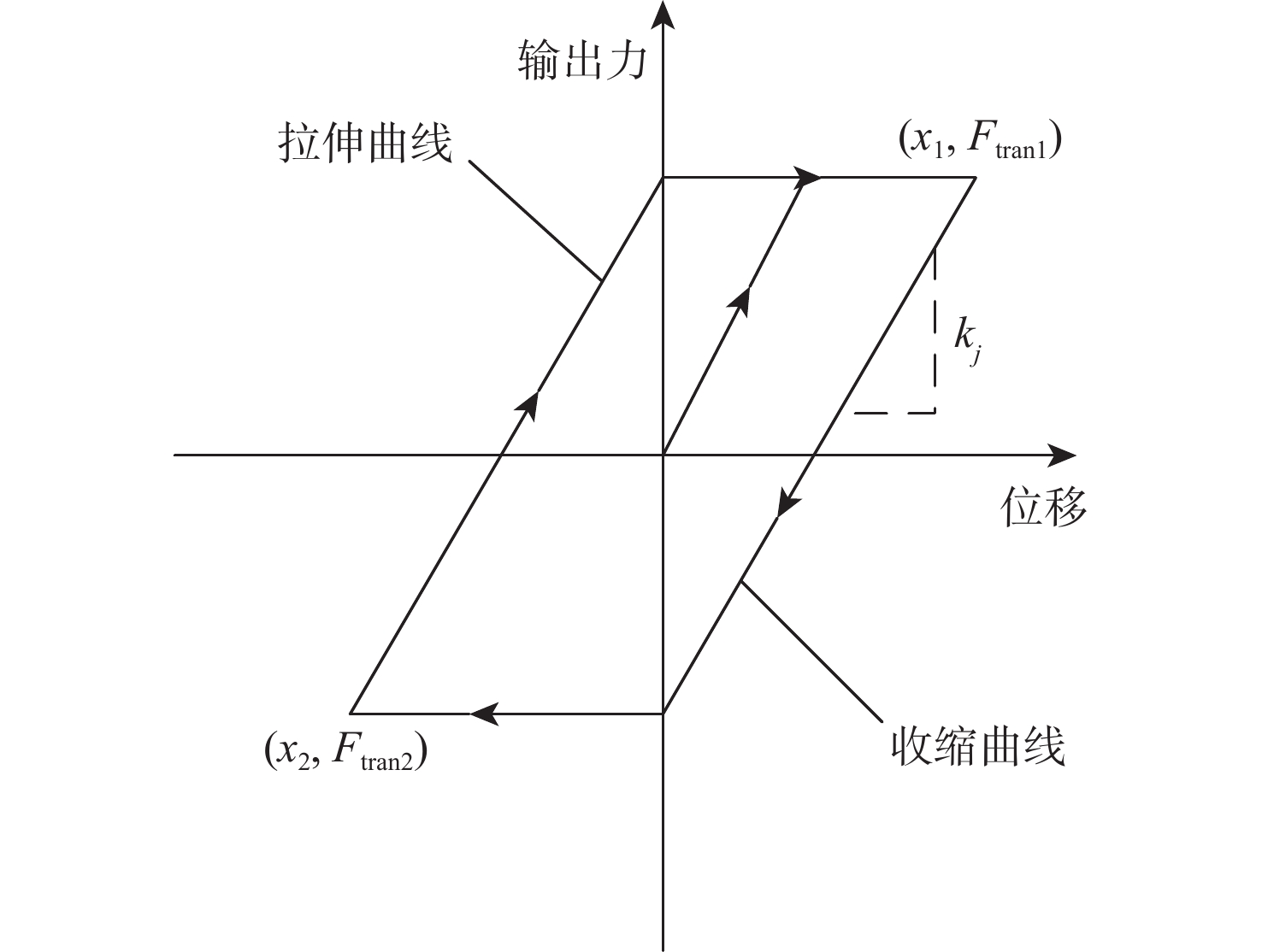
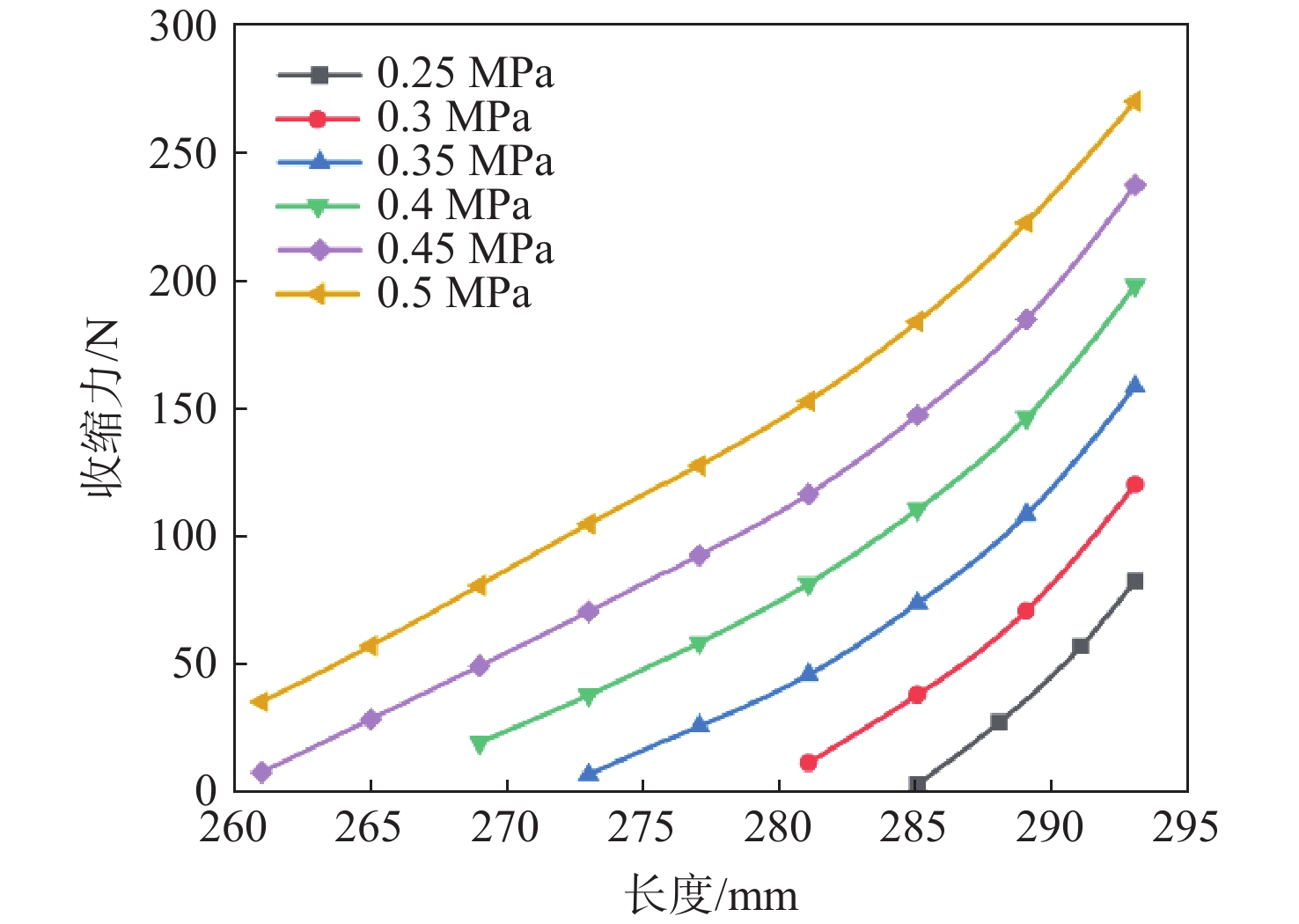
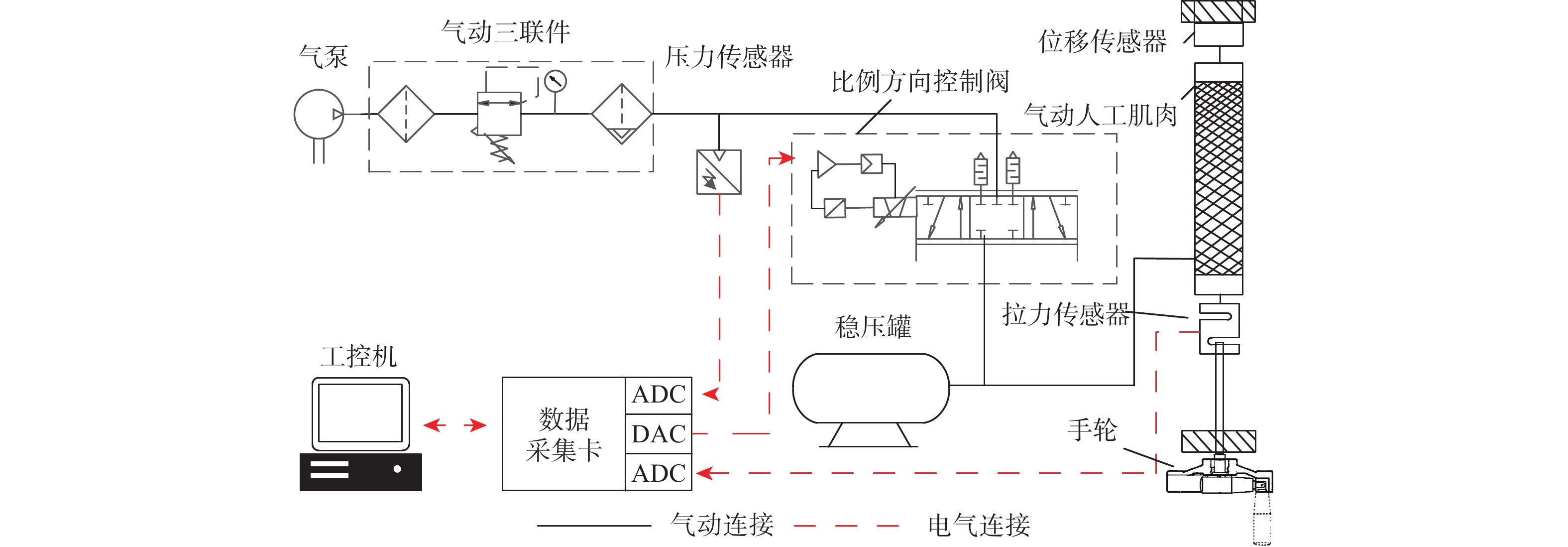
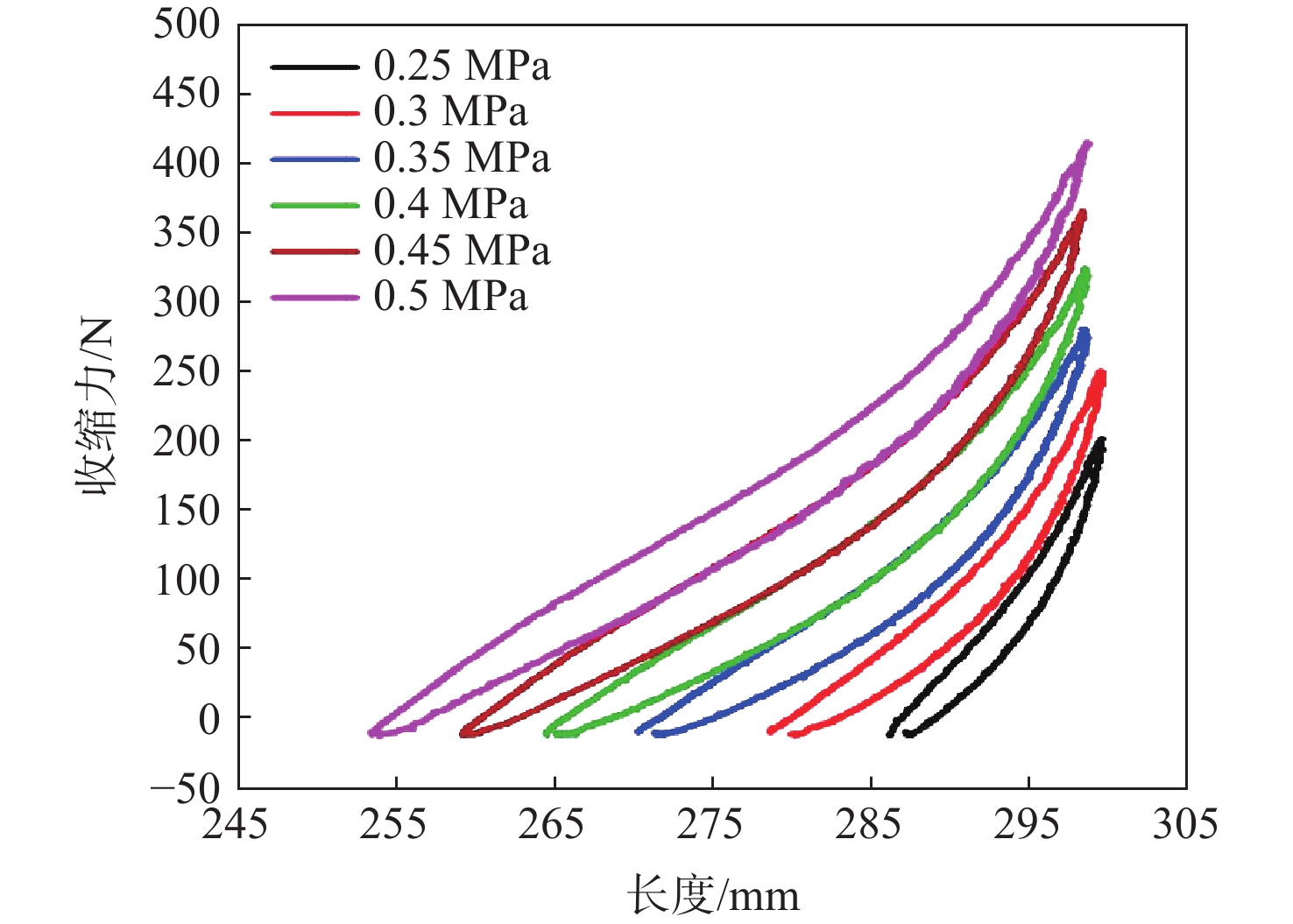
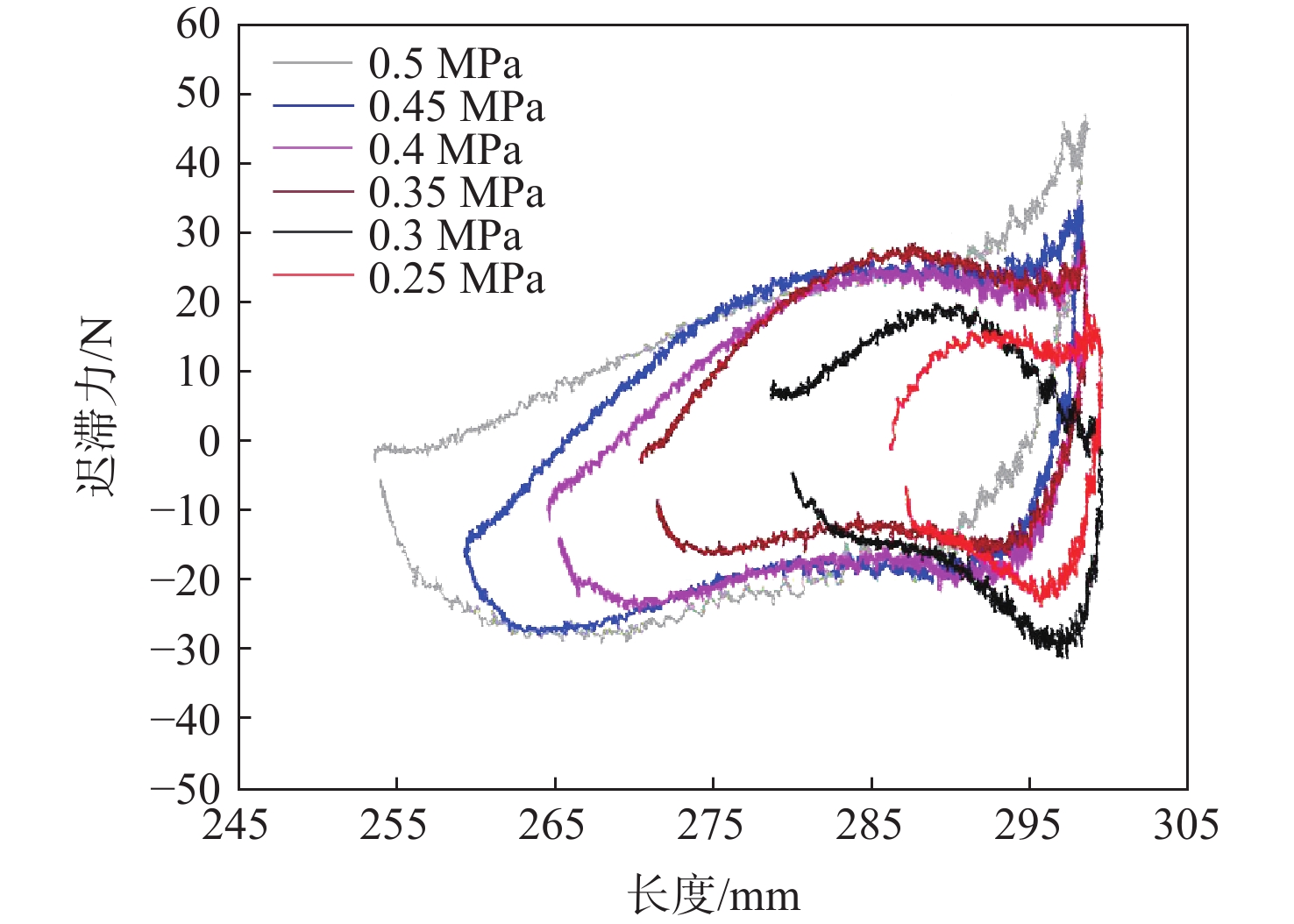
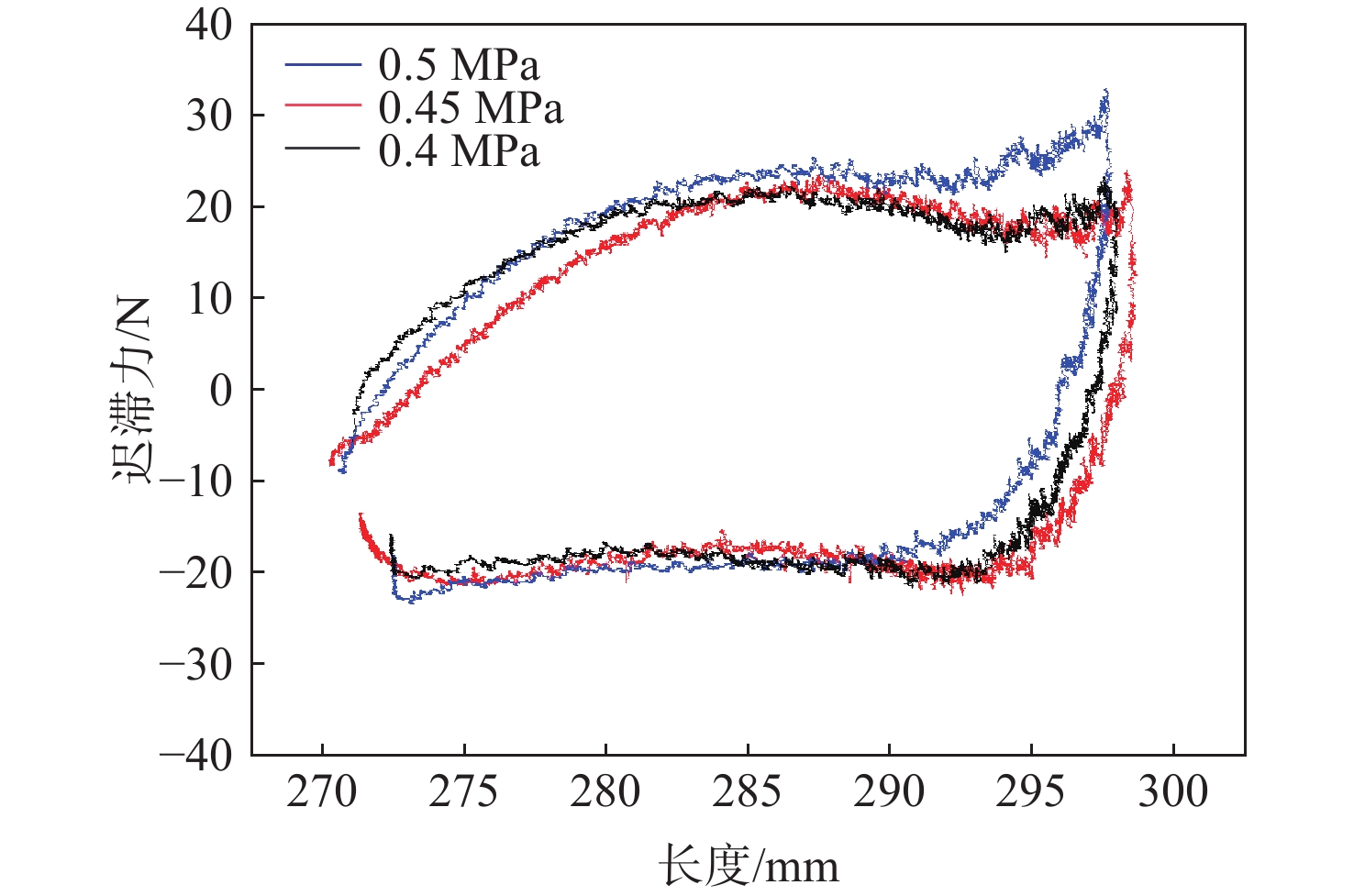
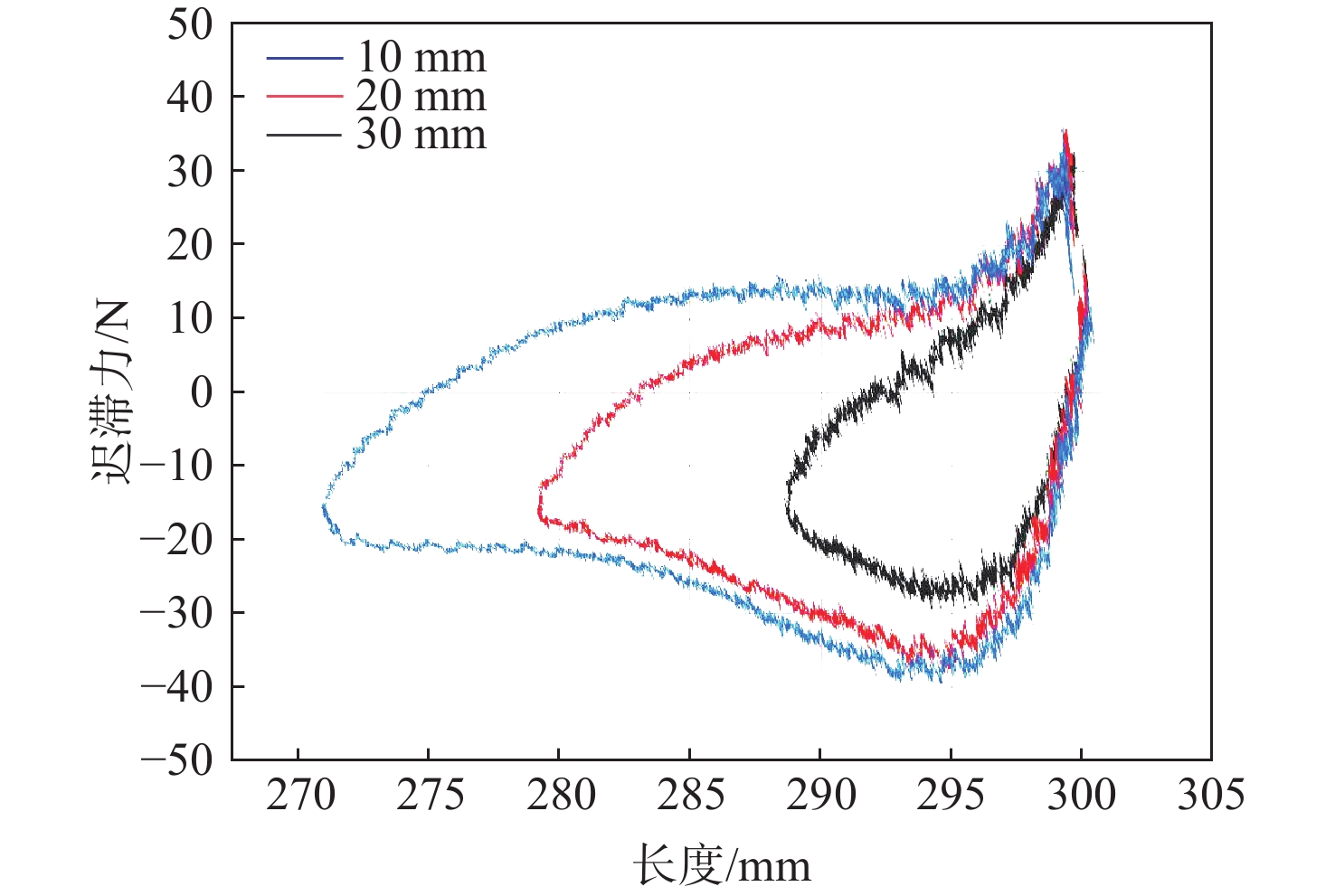
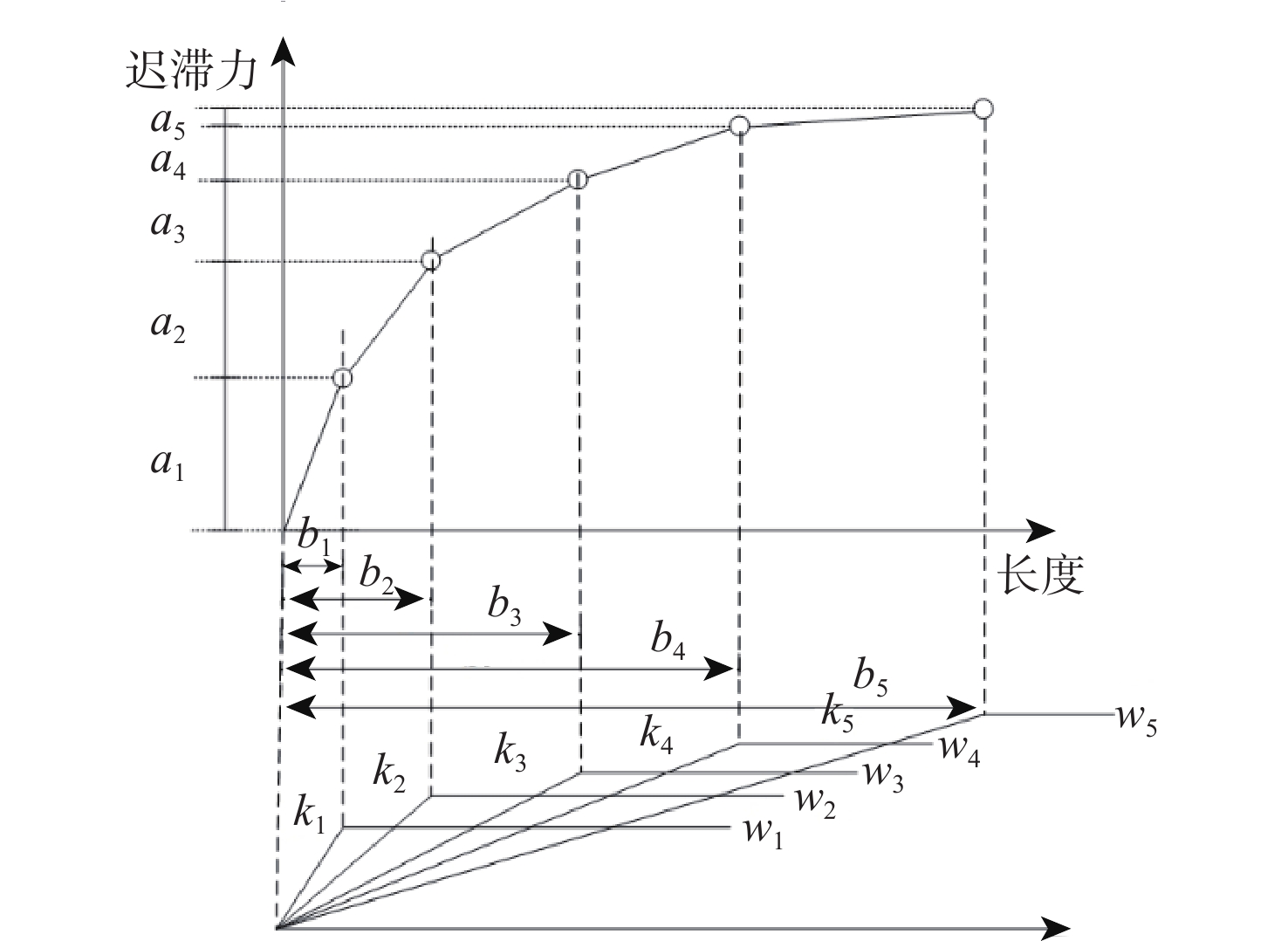
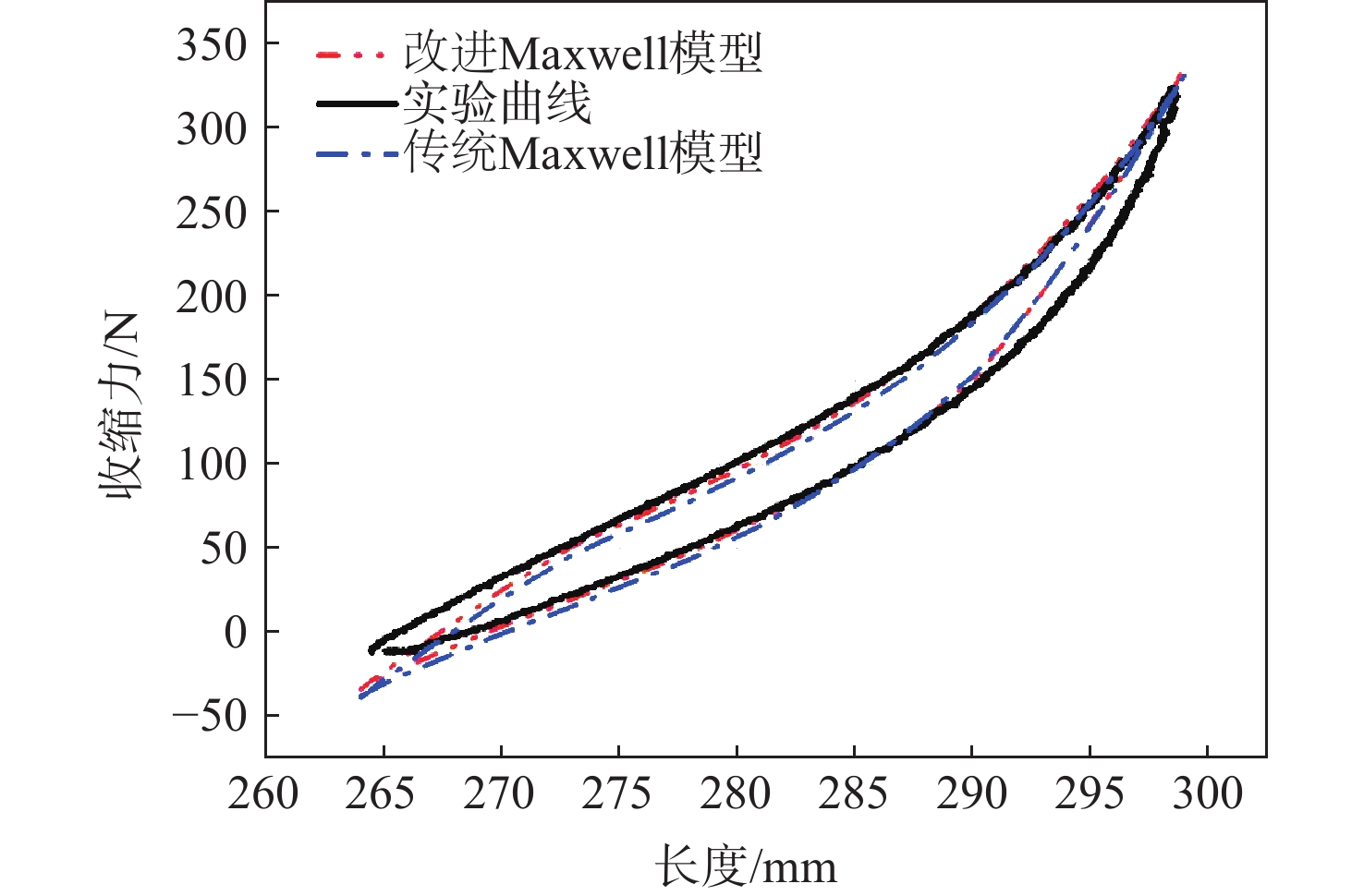
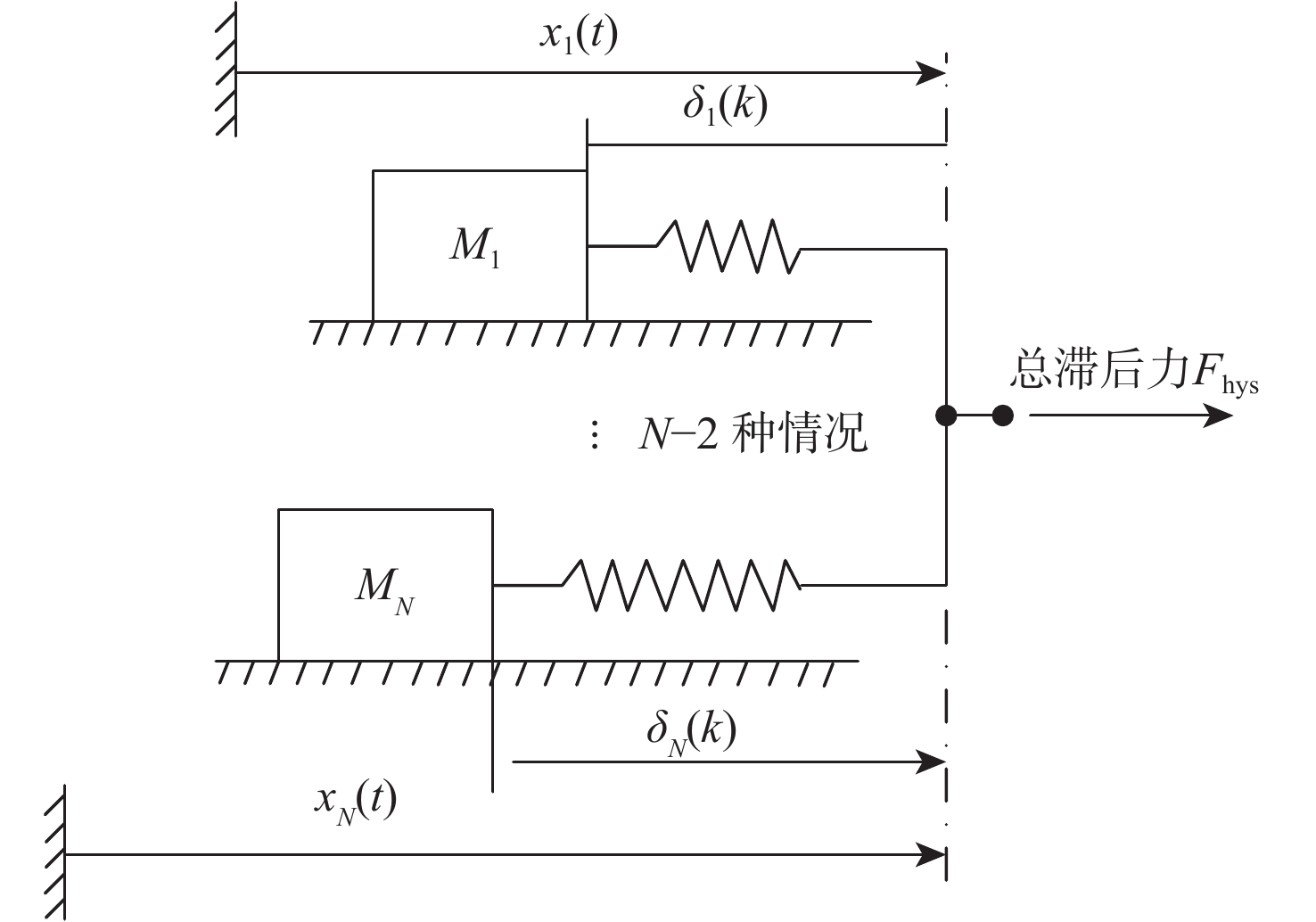
针对气动人工肌肉非线性程度高、迟滞模型建立困难的问题，研究了气动人工肌肉的迟滞特性，提出了一种基于滑移算子的改进Maxwell模型。搭建了气动人工肌肉的收缩力测试平台，进行了静态力实验和动态力实验，得到了气动人工肌肉的迟滞力曲线，并分别考虑了不同行程、不同充气压力和不同伸缩速率对迟滞环的影响，得到了气动人工肌肉的各项迟滞特性。针对传统Maxwell模型无法很好地表现气动人工肌肉的非对称迟滞特性，将迟滞力曲线分别从上部和下部收缩，得到的各项算子较好地表现了气动人工肌肉收缩力曲线的非局部记忆性和非对称性，将最大误差控制在了6.8%以内，中段误差控制在了2.0%以内，较好地匹配了实验曲线，验证了所提模型的普适性。
Abstract:In view of the high nonlinearity of pneumatic artificial muscle and the difficulty in establishing a hysteresis model, the hysteresis characteristics of pneumatic artificial muscle were studied, and an improved Maxwell model based on slip operator was proposed. First of all, the contraction force test platform of pneumatic artificial muscle was set,on which the static force experiment and dynamic force experiment were carried out. The hysteresis force curve of the pneumatic artificial muscle was obtained, and the effects of different strokes, different inflation pressures, and different expansion rates on the hysteresis loop were considered respectively.The hysteresis characteristics of the pneumatic artificial muscle were obtained. Then, since the traditional Maxwell model could not represent the asymmetrical hysteresis characteristics of pneumatic artificial muscle very well, the hysteresis curves were contracted from the upper and lower parts, respectively, and the obtained operators could represent the non-local memory and asymmetry of the contraction curves of the pneumatic artificial muscle better. The maximum error was controlled within 6.8%, andthe error of the middle section was controlled within 2.0%, matching the experimental curve well and verifyingthe universality of the model.
-
图 5 等长实验的压强与收缩力关系
Figure 5. Relationship between pressure and contraction force in isometric test
图 14 基于原始曲线的Maxwell算子参数
Figure 14. Maxwell operator parameters based on original curve
图 16 0.40 MPa充气压力下的拟合情况
Figure 16. Fitting situation at inflation pressure of 0.40 MPa
表 1 等长实验主要元件
Table 1. Main element of isometric test
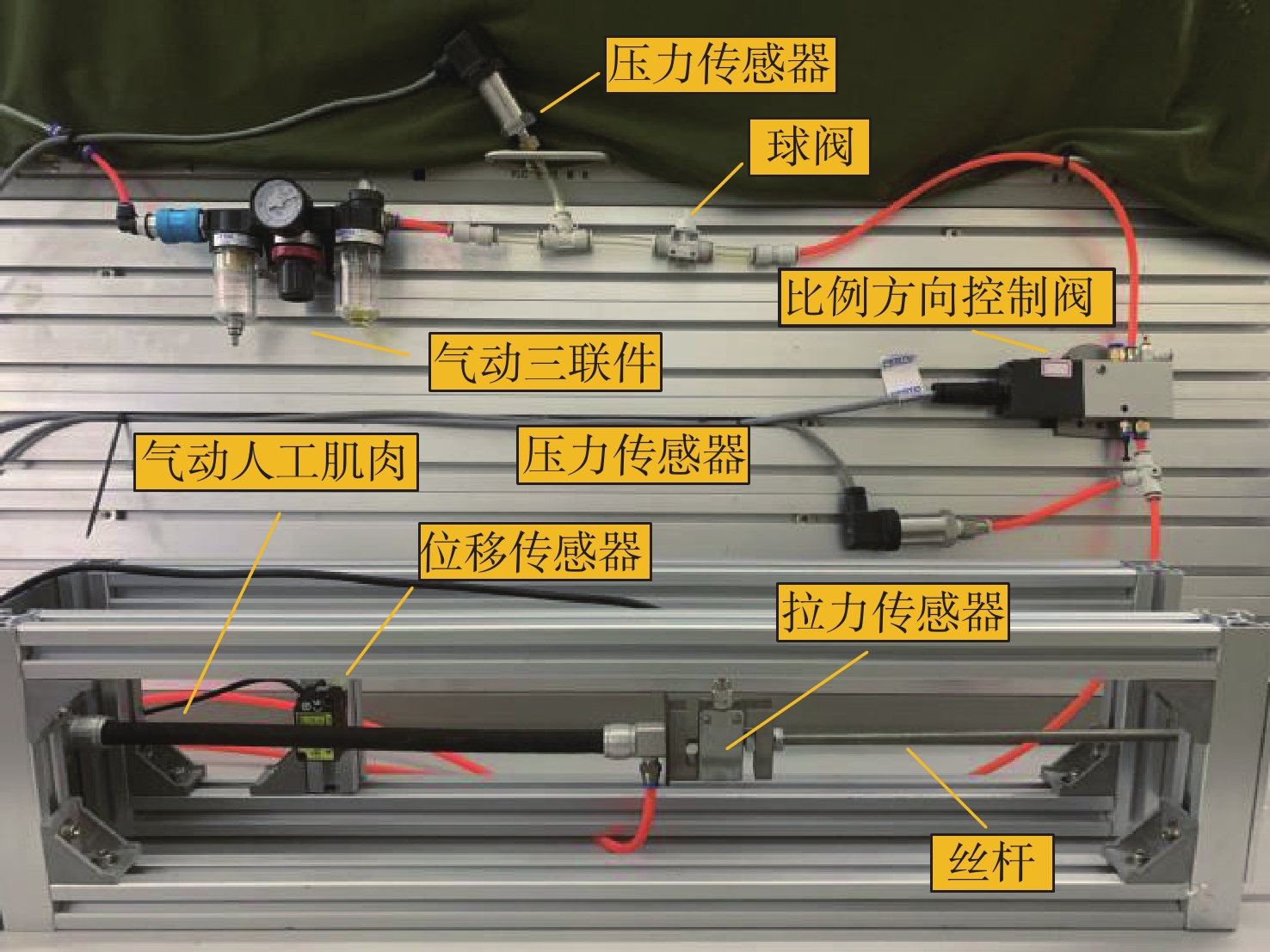
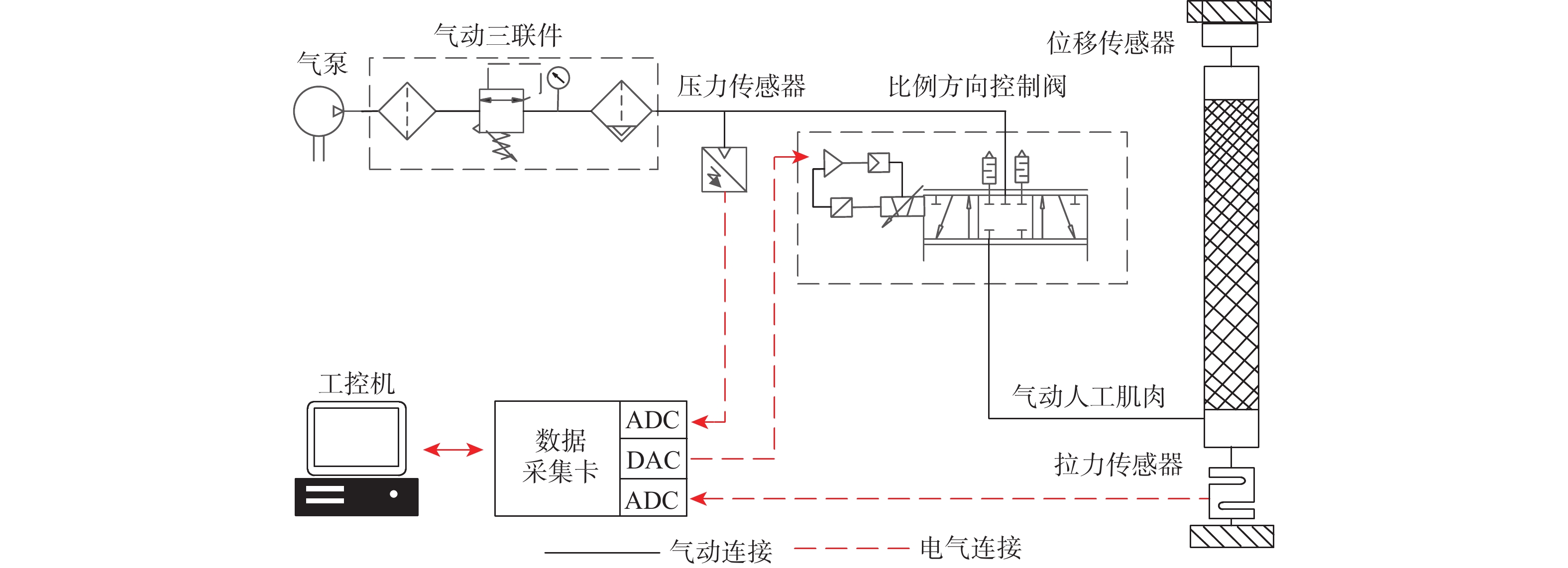
元件名称 元件型号 主要性能指标 气动人工肌肉 DSMP-10-300N-RM-RM 初始长度300 mm，最大抗拉力600 N 数据采集卡 NI PCIE-6353 16位计数器，输出电压−10～10 V 拉力传感器 NTJL-1 0～500 N 空气压缩机 HX750A 最大供气压力1 MPa 压力传感器 MIK-P300 测量范围−0.1～60 MPa 比例方向控制阀 FESTO MPYE-5-M5-010-B 最大工作压力：1 MPa 位移传感器 PANASONIC HG-C1200 测量范围0～40 mm，精度0.01 mm 
下载: 导出CSV
表 2 参数拟合结果
Table 2. Parameter fitting results
$ P/\mathrm{\rm{M}Pa} $ $ \alpha (P) $ $ \beta (P) $ $ \gamma (P) $ $ \lambda (P) $ 0.25 0.0336 −28.703 8188.214 − 779376.024 0.3 0.0262 −22.197 6276.948 − 592287.396 0.35 0.00994 −8.201 2259.785 − 207967.509 0.4 0.00785 −6.431 1760.549 − 161040.129 0.45 0.0057 −4.619 1252.389 − 113560.093 0.5 0.0047 −3.808 1033.544 − 93900.544
下载: 导出CSV
表 3 滑移算子的代表性参数
Table 3. Representative parameters of slip operators
参数 数值 参数 数值 k1 4.0797 w1 18.3585 k2 1.3121 w2 5.9045 k3 0.7226 w3 3.252 k4 0.4981 w4 2.2415 k5 0.0541 w5 0.2435 k6 4.8055 w6 21.625 k7 0.5834 w7 2.6225 k8 0.6996 w8 3.148 k9 0.4940 w9 2.223 k10 0.0841 w10 0.3748
下载: 导出CSV
-
[1] TSAGARAKIS N G, CALDWELL D G. Development and control of a ‘soft-actuated’ exoskeleton for use in physiotherapy and training[J]. Autonomous Robots, 2003, 15(1): 21-33. doi: 10.1023/A:1024484615192 [2] 周彬滨, 邹任玲. 气动人工肌肉在康复器械中的应用现状[J]. 中国康复理论与实践, 2020, 26(4): 463-466. doi: 10.3969/j.issn.1006-9771.2020.04.014ZHOU B B, ZOU R L. Application of pneumatic artificial muscle in rehabilitation equipment (review)[J]. Chinese Journal of Rehabilitation Theory and Practice, 2020, 26(4): 463-466(in Chinese). doi: 10.3969/j.issn.1006-9771.2020.04.014 [3] 高建文, 梁全, 刘慧芳. 气动人工肌肉静态特性实验及模型仿真研究[J]. 机床与液压, 2019, 47(1): 9-11. doi: 10.3969/j.issn.1001-3881.2019.01.003GAO J W, LIANG Q, LIU H F. Static characteristic experiments and model simulation of pneumatic muscle actuator[J]. Machine Tool & Hydraulics, 2019, 47(1): 9-11(in Chinese). doi: 10.3969/j.issn.1001-3881.2019.01.003 [4] INOUE K. Rubbertuators and applications for robots[M]. Cambridge: MIT Press, 1988. [5] CHOU C P, HANNAFORD B. Measurement and modeling of McKibben pneumatic artificial muscles[J]. IEEE Transactions on Robotics and Automation, 1996, 12(1): 90-102. [6] 陈轶珩. 单气动人工肌肉系统的非线性控制[D]. 天津: 南开大学, 2021.CHEN Y H. Nonlinear control of single pneumatic artificial muscle systems[D]. Tianjin: Nankai University, 2021(in Chinese). [7] 孙建民, 赵国浩, 刘祥, 等. 基于气动人工肌肉理论的半主动空气悬架研究[J]. 浙江工业大学学报, 2022, 50(3): 276-283. doi: 10.3969/j.issn.1006-4303.2022.03.006SUN J M, ZHAO G H, LIU X, et al. Research on thesemi-active control air suspension basedon the pneumatic artificial muscle theory[J]. Journal of Zhejiang University of Technology, 2022, 50(3): 276-283(in Chinese). doi: 10.3969/j.issn.1006-4303.2022.03.006 [8] 孙丽娜, 毕庆. 基于改进模型的气动肌肉神经网络串级控制[J]. 机床与液压, 2018, 46(7): 82-85. doi: 10.3969/j.issn.1001-3881.2018.07.018SUN L N, BI Q. Neural network cascade control of pneumatic artificial muscles based on modified model[J]. Machine Tool & Hydraulics, 2018, 46(7): 82-85(in Chinese). doi: 10.3969/j.issn.1001-3881.2018.07.018 [9] 郭振武, 黄继清, 王飞洋, 等. McKibben型气动肌肉模型改进与性能测试[J]. 中国机械工程, 2019, 30(19): 2313-2318. doi: 10.3969/j.issn.1004-132X.2019.19.007GUO Z W, HUANG J Q, WANG F Y, et al. Improvement and performance testing of McKibben pneumatic muscle model[J]. China Mechanical Engineering, 2019, 30(19): 2313-2318(in Chinese). doi: 10.3969/j.issn.1004-132X.2019.19.007 [10] RAMOS J, LYNCH S, JONES D, et al. Hysteresis in muscle[J]. International Journal of Bifurcation and Chaos, 2017, 27(1): 1730003. doi: 10.1142/S0218127417300038 [11] WANG K, ZHANG Y, JONES R W. The modelling of hysteresis in magnetorheological dampers using a generalised Prandtl-Ishlinskii approach[C]//Proceedings of the Conference on Smart Materials, Adaptive Structures and Intelligent Systems. New York: ASME, 2011: 437-442. [12] 李超, 刘成, 于飞, 等. 一种压电陶瓷建模与控制的新方法[J]. 航天返回与遥感, 2021, 42(1): 100-107. doi: 10.3969/j.issn.1009-8518.2021.01.012LI C, LIU C, YU F, et al. A new method for hysteresis modeling and control of piezoelectric ceramics[J]. Spacecraft Recovery & Remote Sensing, 2021, 42(1): 100-107(in Chinese). doi: 10.3969/j.issn.1009-8518.2021.01.012 [13] 龚瑾, 龚云琪, 梅顺齐. 炭黑/液体硅橡胶复合材料的迟滞性和拉伸响应特性[J]. 仪表技术与传感器, 2020(4): 15-19. doi: 10.3969/j.issn.1002-1841.2020.04.004GONG J, GONG Y Q, MEI S Q. Hysteresis and tensile response characteristics of carbon black/liquid silicone rubber composites[J]. Instrument Technique and Sensor, 2020(4): 15-19(in Chinese). doi: 10.3969/j.issn.1002-1841.2020.04.004 [14] 付婵君. 非对称迟滞建模与迟滞系统辨识方法研究[D]. 泉州: 华侨大学, 2016: 2-10.FU C J. Research on asymmetric hysteresis modeling and hysteresis system identification method[D]. Quanzhou: Huaqiao University, 2016: 2-10(in Chinese) . [15] 谢胜龙, 刘海涛, 梅江平. 气动人工肌肉迟滞-蠕变特性研究现状与进展[J]. 系统仿真学报, 2018, 30(3): 809-823.XIE S L, LIU H T, MEI J P. Achievements and developments of hysteresis and creep of pneumatic artificial muscles[J]. Journal of System Simulation, 2018, 30(3): 809-823(in Chinese). [16] VO-MINH T, TJAHJOWIDODO T, RAMON H, et al. A new approach to modeling hysteresis in a pneumatic artificial muscle using the Maxwell-slip model[J]. IEEE/ASME Transactions on Mechatronics, 2011, 16(1): 177-186. doi: 10.1109/TMECH.2009.2038373 [17] MINH T V, KAMERS B, RAMON H, et al. Modeling and control of a pneumatic artificial muscle manipulator joint–Part I: Modeling of a pneumatic artificial muscle manipulator joint with accounting for creep effect[J]. Mechatronics, 2012, 22(7): 923-933. [18] VAN DAMME M, BEYL P, VANDERBORGHT B, et al. Modeling hysteresis in pleated pneumatic artificial muscles[C]//Proceedings of the IEEE Conference on Robotics, Automation and Mechatronics. Piscataway: IEEE Press, 2008: 471-476. [19] ZANG X Z, LIU Y X, HENG S, et al. Position control of a single pneumatic artificial muscle with hysteresis compensation based on modified Prandtl-Ishlinskii model[J]. Bio-Medical Materials and Engineering, 2017, 28(2): 131-140. doi: 10.3233/BME-171662 [20] XIE S L, LIU H T, WANG Y. A method for the length-pressure hysteresis modeling of pneumatic artificial muscles[J]. Science China Technological Sciences, 2020, 63(5): 829-837. doi: 10.1007/s11431-019-9554-y [21] ZHAO J, ZHONG J, FAN J Z. Position control of a pneumatic muscle actuator using RBF neural network tuned PID controller[J]. Mathematical Problems in Engineering, 2015, 2015: 810231. [22] ZHONG J, FAN J Z, ZHU Y H, et al. One nonlinear PID control to improve the control performance of a manipulator actuated by a pneumatic muscle actuator[J]. Advances in Mechanical Engineering, 2014, 6: 172782. doi: 10.1155/2014/172782 [23] 魏琼, 陆浩, 刘伟恒, 等. 双阀气动伺服系统的LuGre摩擦模型补偿研究[J]. 机械科学与技术, 2023, 42(10): 1609-1616.WEI Q, LU H, LIU W H, et al. Research on LuGre friction model compensation of dual-valve pneumatic servo system[J]. Mechanical Science and Technology for Aerospace Engineering, 2023, 42(10): 1609-1616(in Chinese). [24] SWEVERS J, AL-BENDER F, GANSEMAN C G, et al. An integrated friction model structure with improved presliding behavior for accurate friction compensation[J]. IEEE Transactions on Automatic Control, 2000, 45(4): 675-686. [25] RUDERMAN M. Presliding hysteresis damping of LuGre and Maxwell-slip friction models[J]. Mechatronics, 2015, 30: 225-230. -
下载:
点击查看大图
计量
- 文章访问数: 200
- HTML全文浏览量: 63
- PDF下载量: 1
- 被引次数: 0
