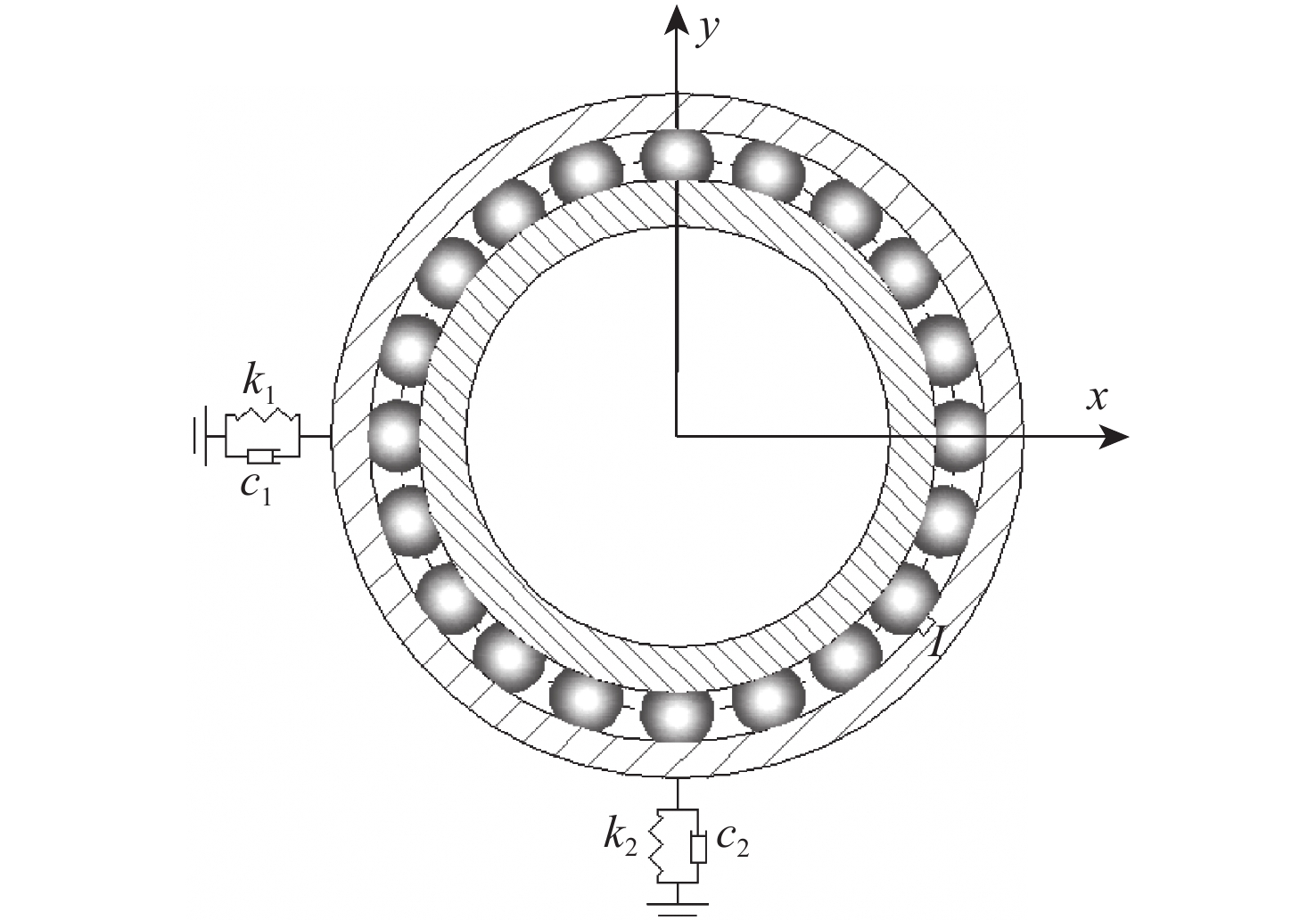
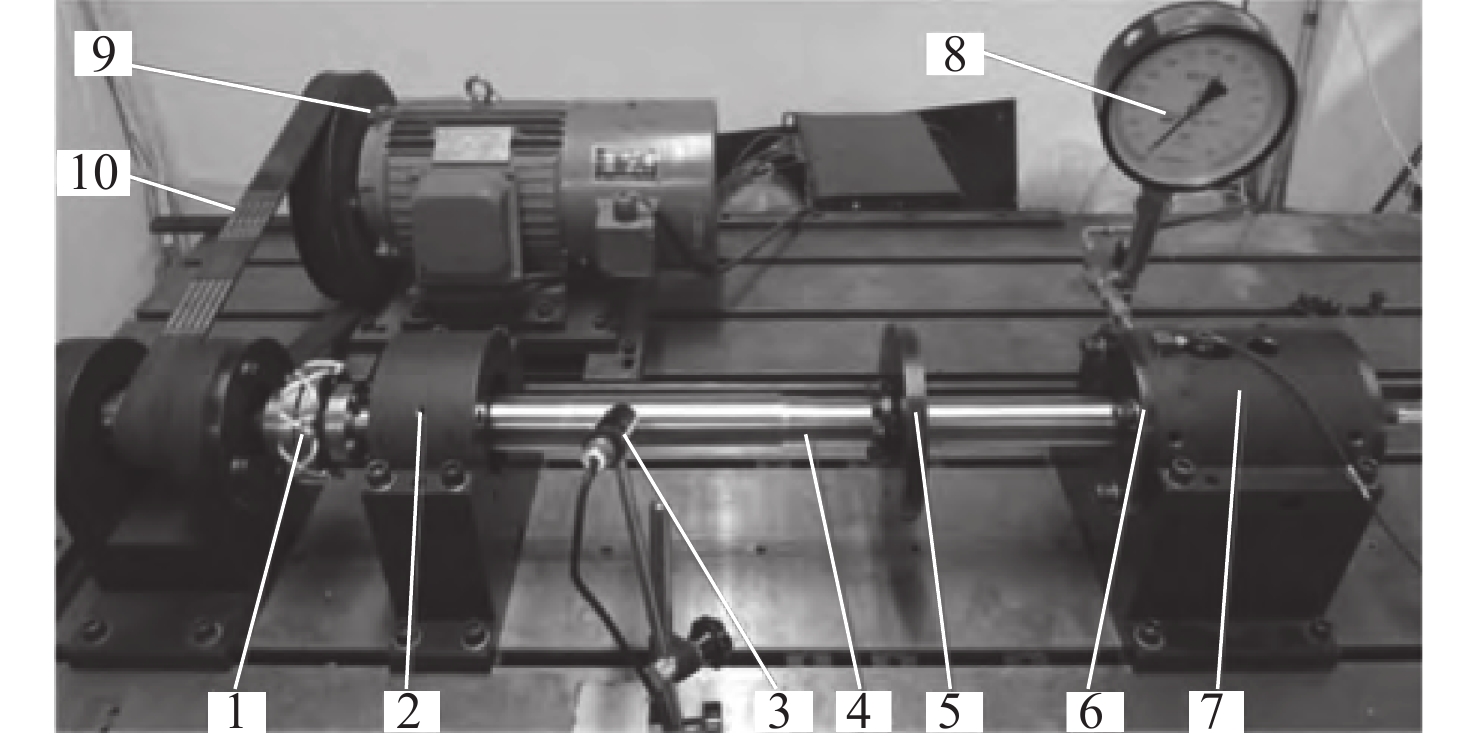
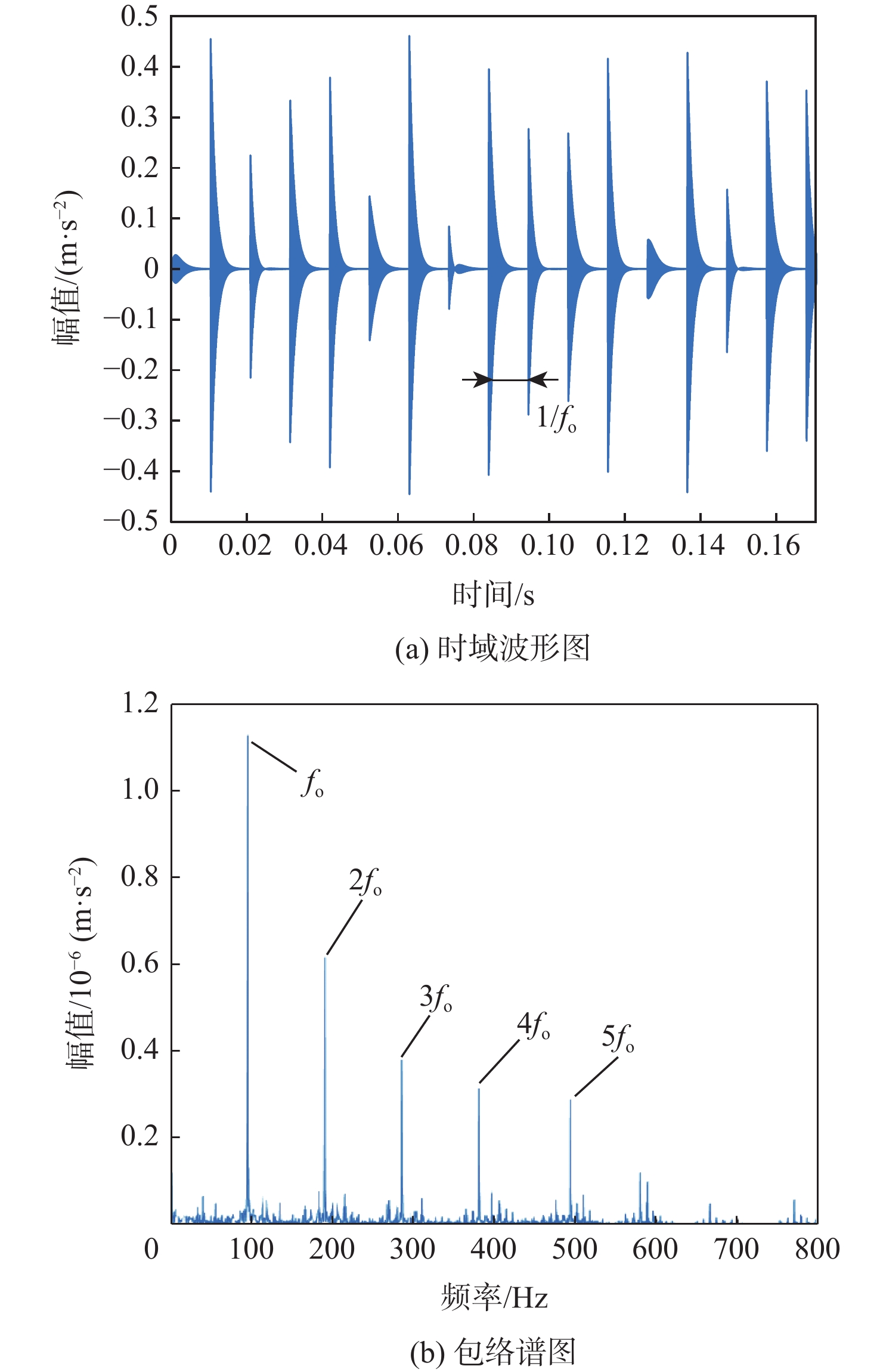
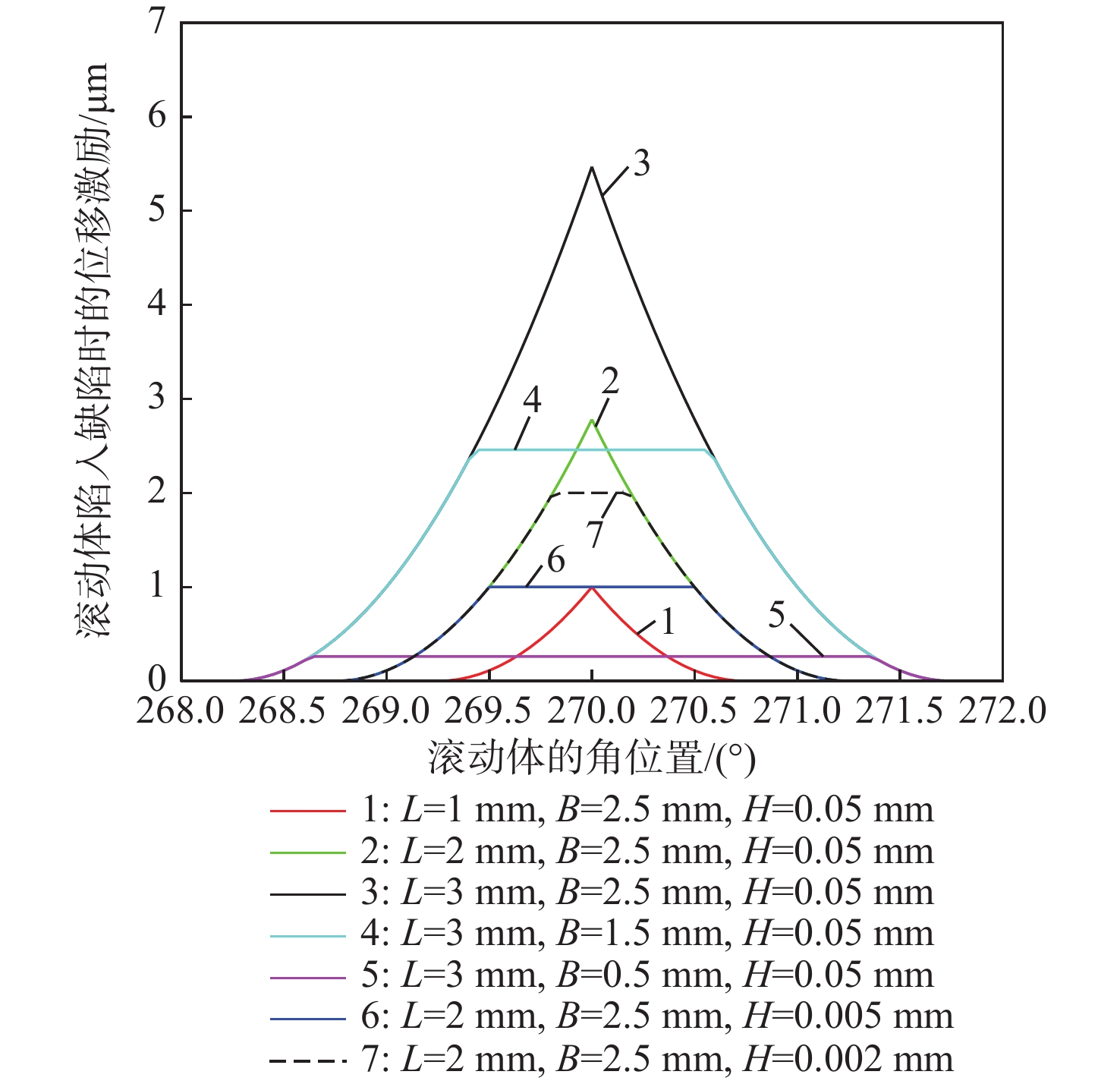
Time-varying displacement excitation and dynamic modeling of local defects in angular contact ball bearings
-
摘要:
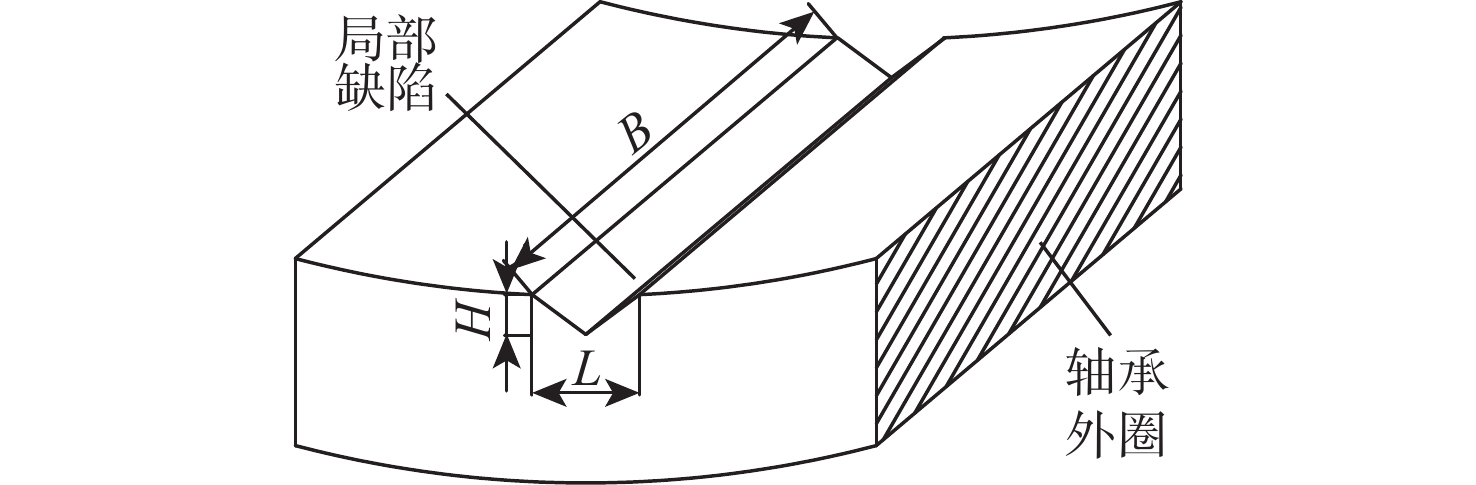
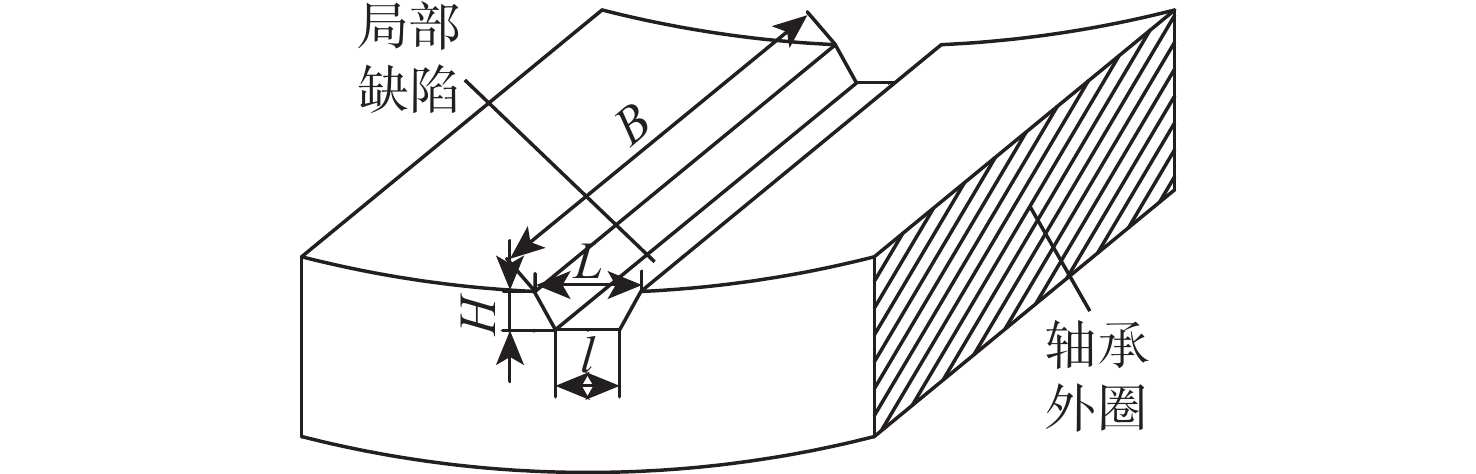
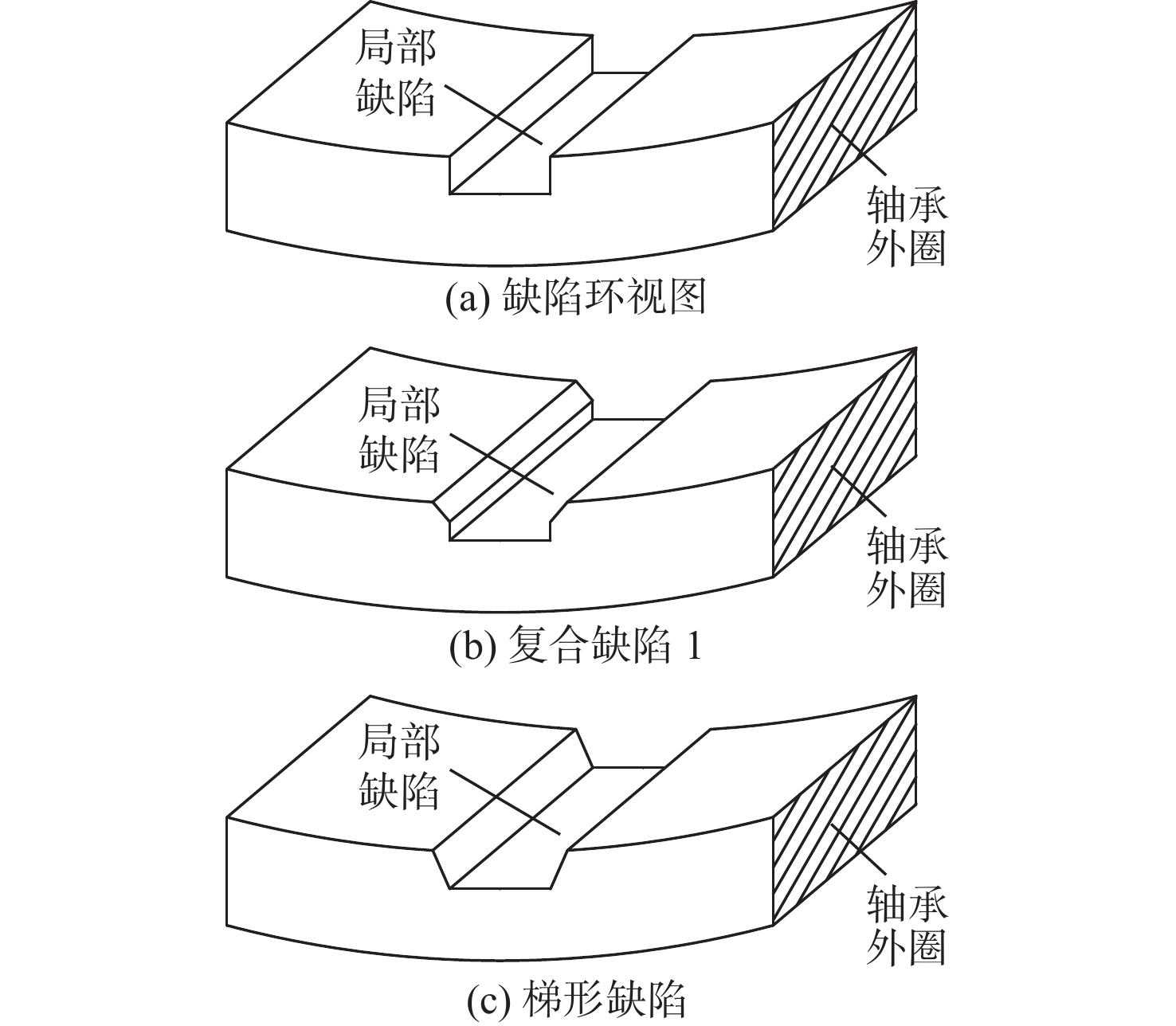
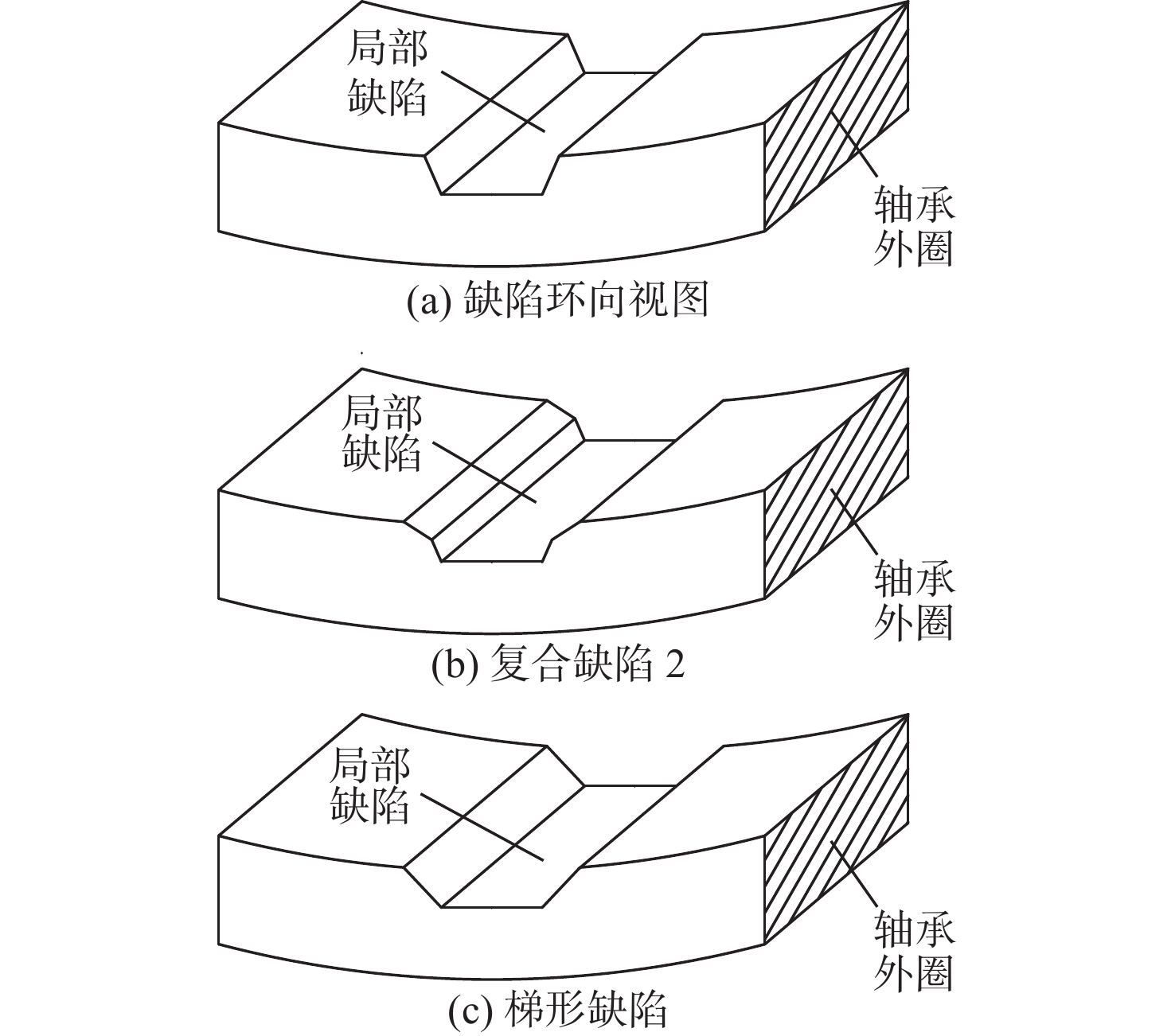
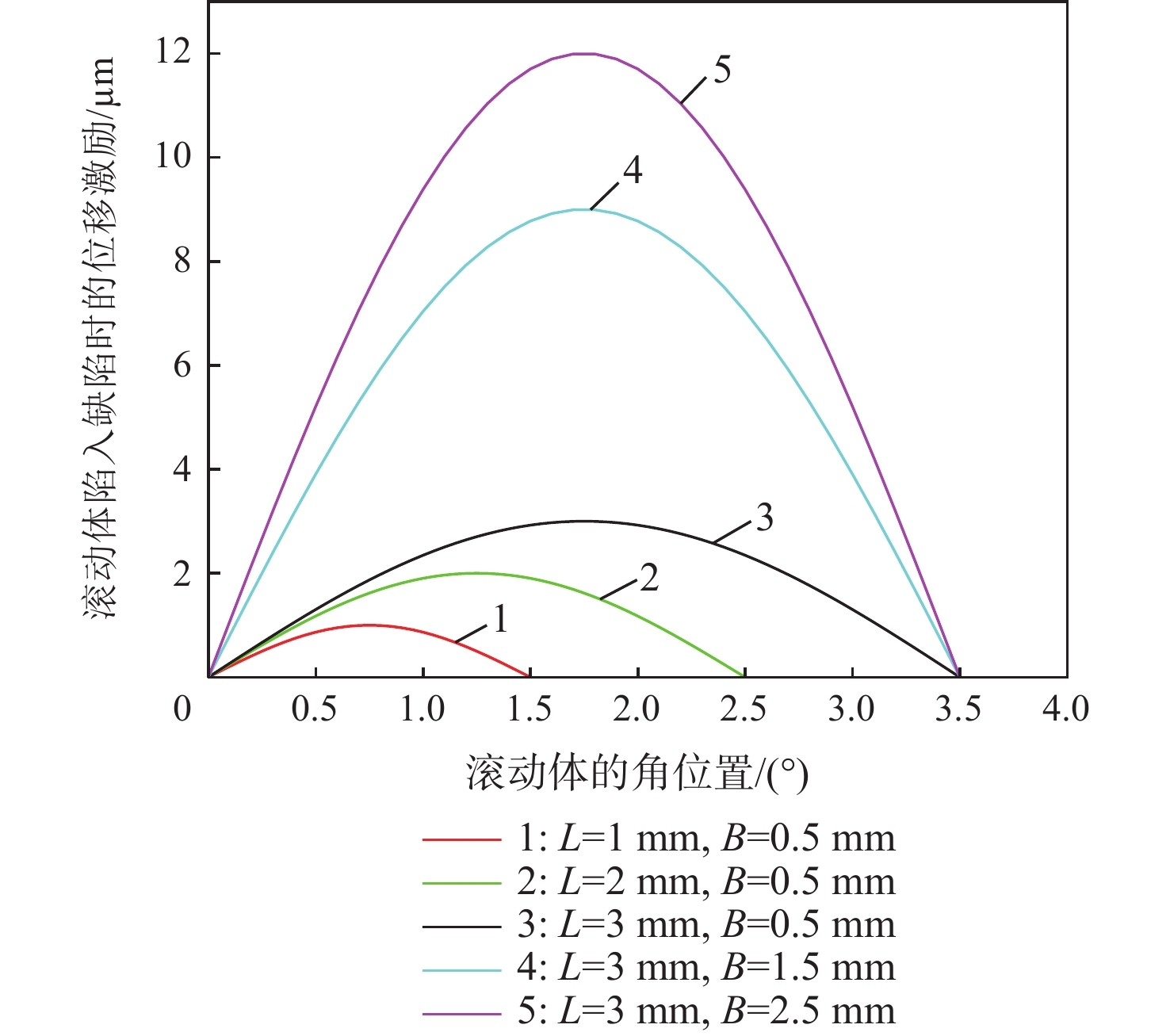
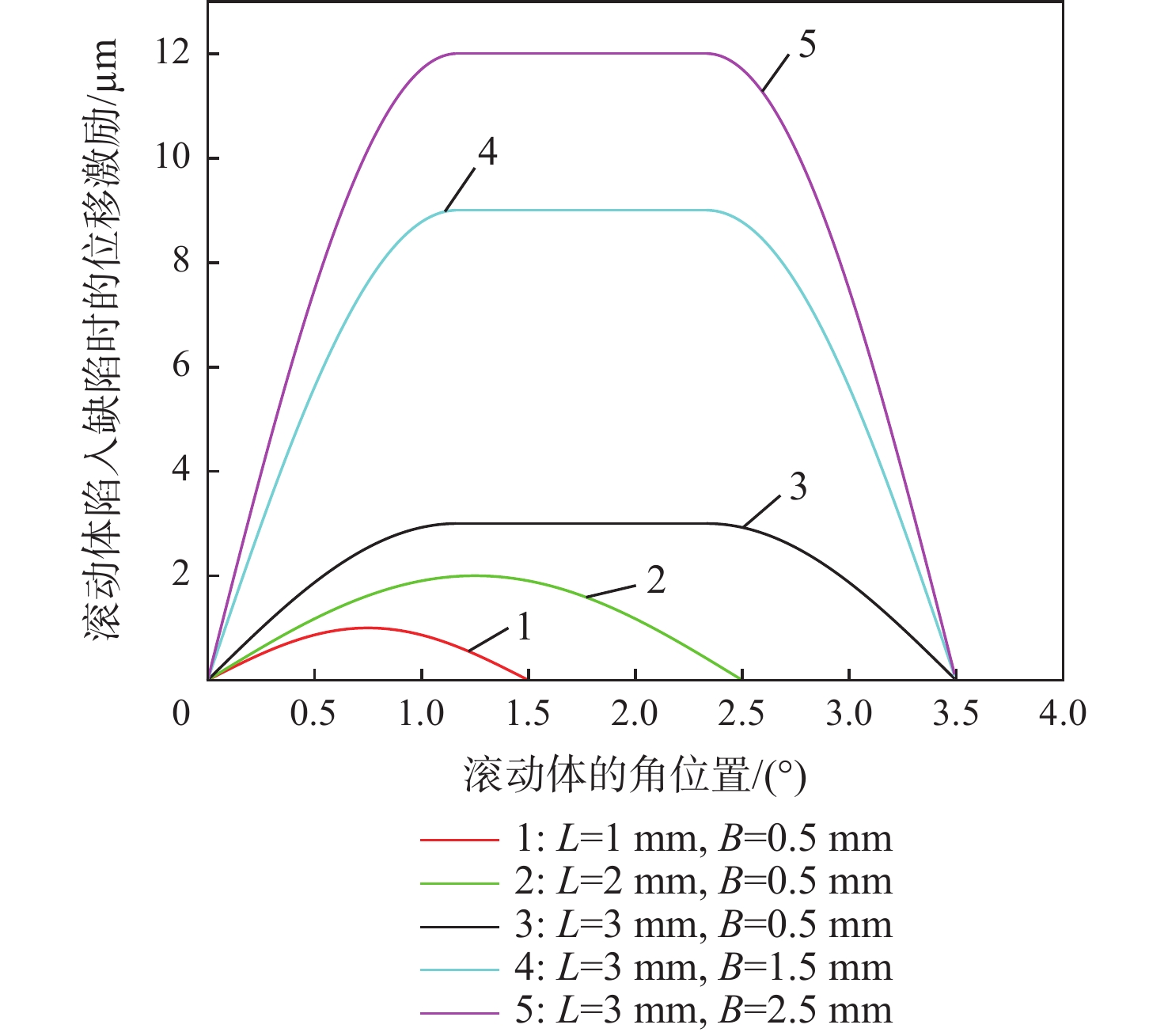
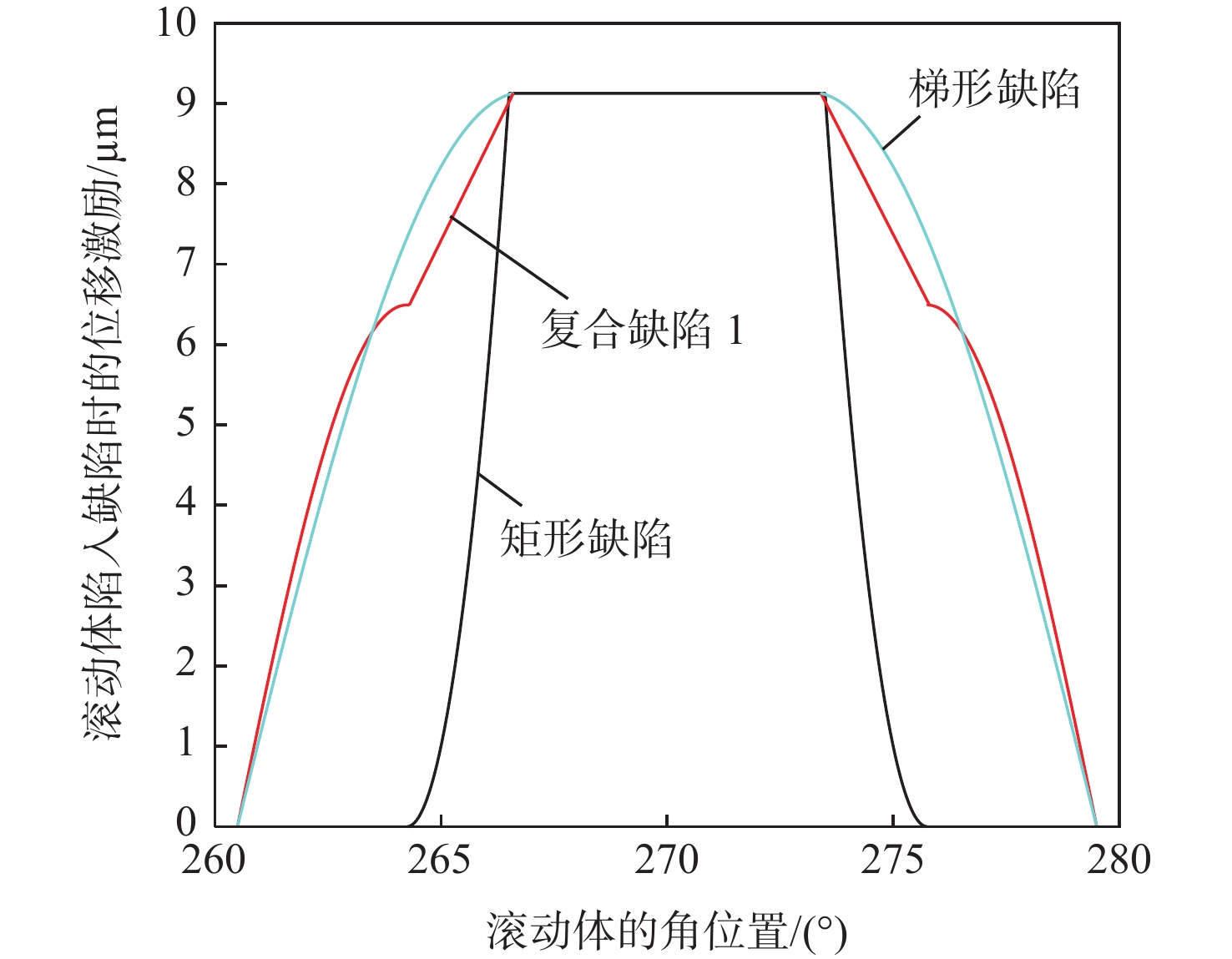
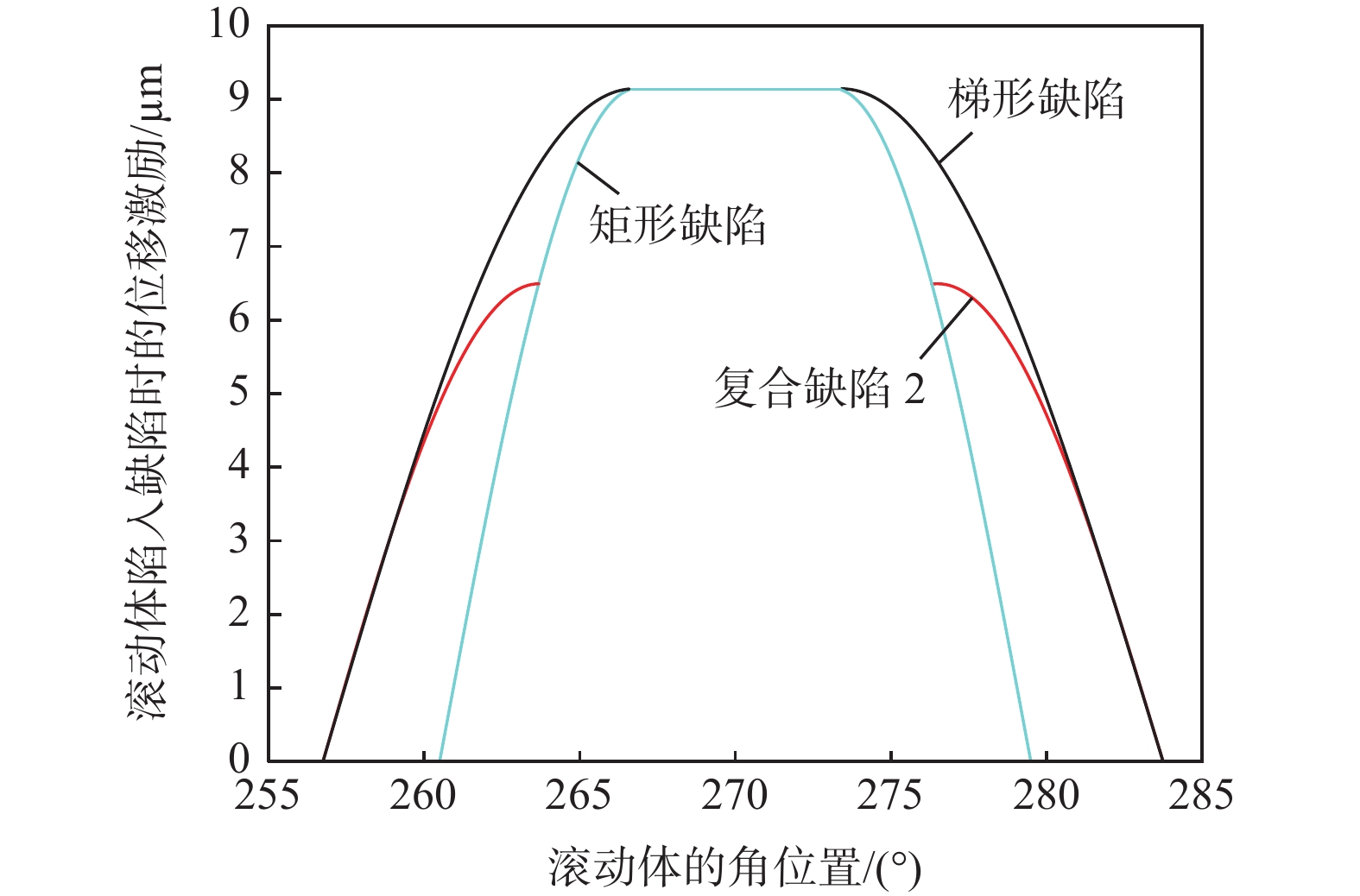
角接触球轴承在长时间工作的情况下，会产生故障损伤从而影响系统正常运行。以外圈具有局部缺陷的角接触球轴承为研究对象，提出不同局部缺陷轮廓的判别方法，建立角接触球轴承局部缺陷时变位移激励广义表征模型，研究局部缺陷演化过程及其位移激励机理。在此基础上，考虑轴承缺陷引起的时变位移对动力学特性的影响，基于赫兹接触理论，建立角接触球轴承故障动力学模型，并通过实验验证了所建模型的正确性。分析结果表明：矩形局部缺陷最终会演化成梯形形态；不同缺陷形貌诱发的位移激励变化趋势是不同的；与局部缺陷的长度相比，宽度对位移激励的影响更大。研究结果对轴承优化设计和故障诊断提供了理论基础。
Abstract:Angular contact ball bearings will suffer from fault damage after working for a long time, thus affecting the normal operation of the system. In this paper, angular contact ball bearingswith local defects in the outer ring were taken as the research objects, and the discrimination methods of different local defect contours were proposed. A generalized representation model of time-varying displacement excitation of local defects in angular contact ball bearing was established, and the evolution process of local defects and its displacement excitation mechanism were studied. On this basis, the influence of time-varying displacement caused by bearing defects on dynamic characteristics was explored, and a fault dynamic model of angular contact ball bearings was established based on Hertz contact theory. The correctness of the model was verified by experiments. The results show that the rectangular local defects will eventually evolve into trapezoidal local defects. The changing trend of displacement excitation induced by different defect morphologies is different. Compared with the length of the local defect, the width has a greater influence on the displacement excitation. The research results provide a theoretical basis for bearing optimization design and fault diagnosis.
-
图 2 局部缺陷轮廓类别划分简图
Figure 2. Diagram of classification of local defect contour categories
图 3 轴承外圈矩形缺陷轮廓表征
Figure 3. Characterization of rectangular defect contour in outer ring of bearing
图 4 轴承外圈三角形缺陷轮廓表征
Figure 4. Characterization of triangular defect contour in outer ring of bearing
图 5 轴承外圈梯形缺陷轮廓表征
Figure 5. Characterization of trapezoidal defect contour in outer ring of bearing
图 10 本文动力学模型外圈损伤振动响应
Figure 10. Vibration response of fault in outer ring of the proposed dynamics model
图 11 滚动体陷入矩形缺陷时的位移激励
Figure 11. Displacement excitation of rolling element trapped in rectangular defect
图 12 滚动体陷入三角形缺陷时的位移激励
Figure 12. Displacement excitation of rolling element trapped in triangular defect
图 13 滚动体陷入梯形缺陷时的位移激励
Figure 13. Displacement excitation of rolling element trapped in trapezoidal defect
图 14 滚动体陷入矩形局部缺陷时的位移激励
Figure 14. Displacement excitation of rolling element trapped in rectangular local defect
图 15 滚动体陷入梯形局部缺陷时的位移激励
Figure 15. Displacement excitation of rolling element trapped in trapezoidal local defect
图 16 本文动力学模型外圈局部损伤振动响应
Figure 16. Vibration response of local fault in outer ring of proposed dynamics model
表 1 H7013C轴承基本参数
Table 1. Basic parameters of H7013C bearing
内圈
直径/mm外圈
直径/mm滚动体
直径/mm滚动体
个数轴承
宽度/mm初始
接触角/
(°)滚动体
密度/
(g·cm−3)65 100 8.73 25 18 15 3.2 
下载: 导出CSV
-
[1] 邓子豪, 李录平, 刘瑞, 等. 基于SCADA提取的风电机组偏航齿轮箱故障诊断方法研究[J]. 动力工程学报, 2021, 41(1): 43-50.DENG Z H, LI L P, LIU R, et al. Research on fault diagnosis method of yaw gearbox of wind turbine based on SCADA extraction[J]. Journal of Power Engineering, 2021, 41(1): 43-50(in Chinese). [2] 张建平, 唐五湘. 我国数控机床行业发展战略研究[J]. 企业经济, 2018, 37(9): 66-72.ZHANG J P, TANG W X. Research on development strategy of china's CNC machine tool industry[J]. Enterprise Economy, 2018, 37(9): 66-72(in Chinese). [3] 曹宏瑞, 景新, 苏帅鸣, 等. 中介轴承故障动力学建模与振动特征分析[J]. 机械工程学报, 2020, 56(21): 89-99. doi: 10.3901/JME.2020.21.089CAO H R, JING X, SU S M, et al. Dynamic modeling and vibration analysis for inter-shaft bearing fault[J]. Journal of Mechanical Engineering, 2020, 56(21): 89-99(in Chinese). doi: 10.3901/JME.2020.21.089 [4] PATEL U K A, UPADHYAY S H. Nonlinear dynamic response of cylindrical roller bearing–rotor system with 9 degree of freedom model having a combined localized defect at inner–outer races of bearing[J]. Tribology Transactions, 2017, 60(2): 284-299. doi: 10.1080/10402004.2016.1163759 [5] 徐东, 徐永成, 陈循, 等. 单表面故障的滚动轴承系统非线性动力学研究[J]. 机械工程学报, 2010, 46(21): 61-68. doi: 10.3901/JME.2010.21.061XU D, XU Y C, CHEN X, et al. Study on nonlinear dynamics of rolling bearing system with single surface fault[J]. Journal of Mechanical Engineering, 2010, 46(21): 61-68(in Chinese). doi: 10.3901/JME.2010.21.061 [6] 吕延军, 曹治军, 黑棣, 等. 具有陀螺效应的转子-轴承系统的非线性动力学行为[J]. 西安理工大学学报, 2010, 26(1): 13-19. doi: 10.3969/j.issn.1006-4710.2010.01.003LYU Y J, CAO Z J, HEI D, et al. Nonlinear dynamic behaviors of unsymmetrical rotor-bearing system with gyroscopic effect[J]. Journal of Xi’an University of Technology, 2010, 26(1): 13-19(in Chinese). doi: 10.3969/j.issn.1006-4710.2010.01.003 [7] CHENG H C, ZHANG Y M, LU W J, et al. Research on time-varying stiffness of bearing based on local defect and varying compliance coupling[J]. Measurement, 2019, 143: 155-179. doi: 10.1016/j.measurement.2019.04.079 [8] 马辉, 李鸿飞, 俞昆, 等. 含局部故障的滚动轴承动力学建模及振动分析[J]. 东北大学学报(自然科学版), 2020, 41(3): 343-348. doi: 10.12068/j.issn.1005-3026.2020.03.008MA H, LI H F, YU K, et al. Dynamic modeling and vibration analysis of rolling bearing with local faults[J]. Journal of Northeast University (Natural Science Edition), 2020, 41(3): 343-348(in Chinese). doi: 10.12068/j.issn.1005-3026.2020.03.008 [9] 常斌全, 剡昌锋, 苑浩, 等. 多事件激励的滚动轴承动力学建模[J]. 振动与冲击, 2018, 37(17): 16-24.CHANG B Q, YAN C F, YUAN H, et al. Dynamic modeling for rolling bearings under multi-event excitation[J]. Journal of Vibration and Shock, 2018, 37(17): 16-24(in Chinese). [10] 李志农, 李云龙, 任帅, 等. 局部点蚀故障滚动体的滚动轴承动力学模型研究[J]. 振动工程学报, 2020, 33(3): 597-603.LI Z N, LI Y L, REN S, et al. Study on dynamic model of rolling bearing with local pitting failure[J]. Journal of Vibration Engineering, 2020, 33(3): 597-603(in Chinese). [11] PATEL V N, TANDON N, PANDEY R K. A dynamic model for vibration studies of deep groove ball bearings considering single and multiple defects in races[J]. Journal of Tribology, 2010, 132(4): 041101. doi: 10.1115/1.4002333 [12] JAFARI S M, ROHANI R, RAHI A. Experimental and numerical study of an angular contact ball bearing vibration response with spall defect on the outer race[J]. Archive of Applied Mechanics, 2020, 90(11): 2487-2511. doi: 10.1007/s00419-020-01733-z [13] 王凯, 剡昌锋, 王风涛, 等. 深沟球轴承复合故障动力学特征[J]. 哈尔滨工业大学学报, 2020, 52(1): 133-140. doi: 10.11918/201809184WANG K, YAN C F, WANG F T, et al. Dynamic characteristics of compound fault in deep groove ball bearing[J]. Journal of Harbin Institute of Technology, 2020, 52(1): 133-140(in Chinese). doi: 10.11918/201809184 [14] 李志农, 周泽文, 胡茑庆, 等. 考虑冲击力的球轴承复合缺陷动力学建模[J]. 沈阳工业大学学报, 2022, 44(2): 173-179. doi: 10.7688/j.issn.1000-1646.2022.02.09LI Z N, ZHOU Z W, HU P Q, et al. Dynamic modeling of composite defects of ball bearing with consideration of impact force[J]. Journal of Shenyang University of Technology, 2022, 44(2): 173-179(in Chinese). doi: 10.7688/j.issn.1000-1646.2022.02.09 [15] ASTEKAR A, SADEGHI F, STACHE L E. Surface defects effects on bearing dynamics[J]. Proceedings of the Institution of Mechanical Engineers, Part J: Journal of Engineering Tribology, 2010, 224(1): 25-35. doi: 10.1243/13506501JET578 [16] CUI L L, CHEN X, CHEN S J. Dynamics modeling and analysis of local fault of rolling element bearing[J]. Advances in Mechanical Engineering, 2015, 7(1): 262351. doi: 10.1155/2014/262351 [17] 刘静. 滚动轴承缺陷非线性激励机理与建模研究[D]. 重庆: 重庆大学, 2014.LIU J. Research on nonlinear excitation mechanism and modeling of rolling bearing defects[D]. Chongqing: Chongqing University, 2014(in Chinese). [18] 丁为民, 潘帅航, 张执南. 含缺陷滚动轴承内部载荷分布[J]. 机械设计与研究, 2016, 32(4): 79-84.DING W M, PAN S H, ZHANG Z N. Load distribution of rolling bearing with localized defect[J]. Machine Design & Research, 2016, 32(4): 79-84(in Chinese). [19] 王震, 杨正伟, 何浩浩, 等. 非规则轴承故障的动力学建模与仿真[J]. 北京航空航天大学学报, 2021, 47(8): 1580-1593.WANG Z, YANG Z W, HE H H, et al. Dynamic modeling and simulation of irregular bearing failure[J]. Journal of Beijing University of Aeronautics and Astronautics, 2021, 47(8): 1580-1593(in Chinese). [20] 周智, 朱永生, 闫柯, 等. 考虑缺陷因素的角接触球轴承七自由度动力学模型[J]. 计算机集成制造系统, 2014, 20(11): 2836-2842.ZHOU Z, ZHU Y S, YAN K, et al. Seven degrees of freedom dynamic model of angular contact ball bearing with defects[J]. Computer Integrated Manufacturing Systems, 2014, 20(11): 2836-2842(in Chinese). [21] PETERSEN D, HOWARD C, PRIME Z. Varying stiffness and load distributions in defective ball bearings: analytical formulation and application to defect size estimation[J]. Journal of Sound and Vibration, 2015, 337: 284-300. doi: 10.1016/j.jsv.2014.10.004 -
下载:
点击查看大图
计量
- 文章访问数: 270
- HTML全文浏览量: 95
- PDF下载量: 8
- 被引次数: 0
