Influence of hypersonic thermo-chemical non-equilibrium on aerodynamic thermal environments
-
摘要:
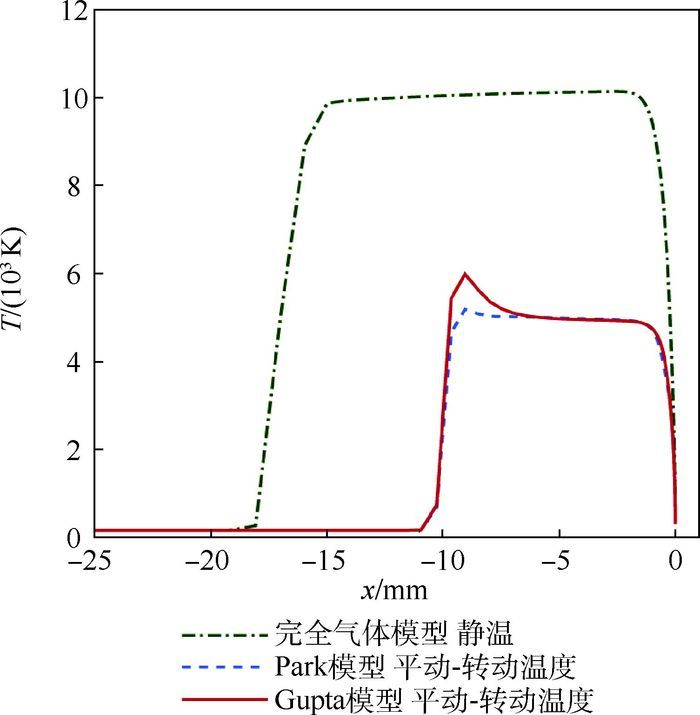
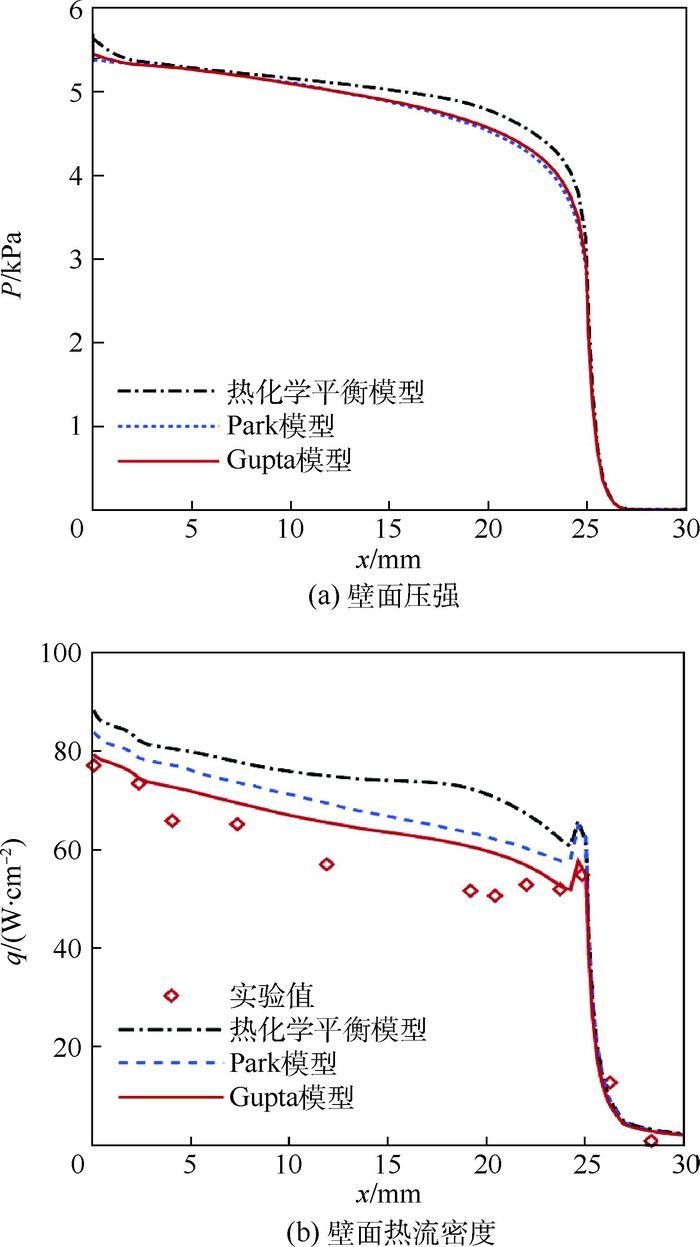
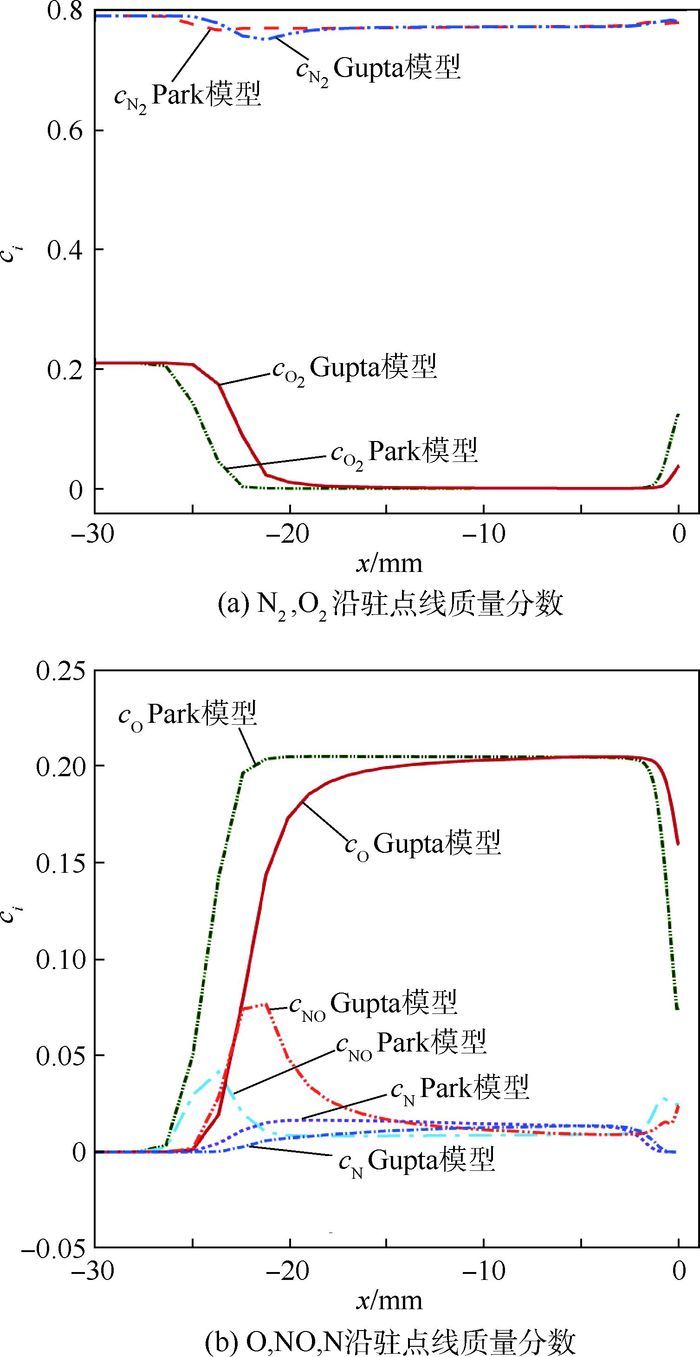
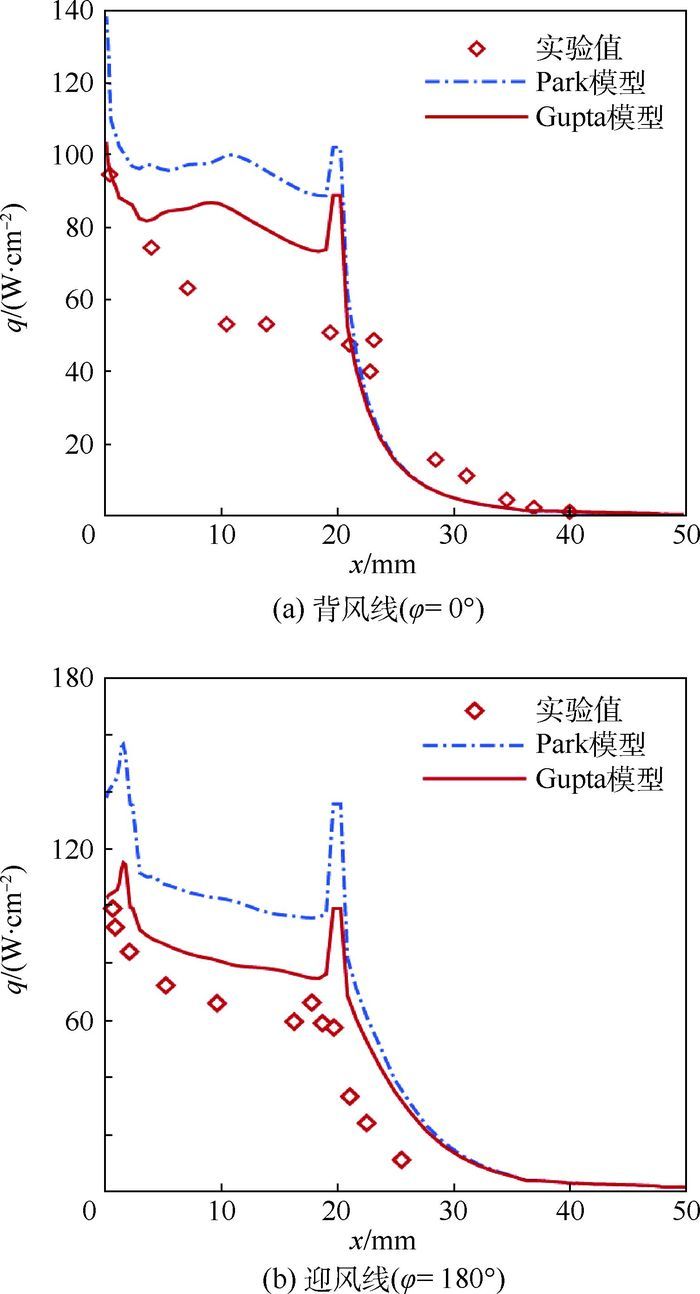
高超声速气动加热严重，考虑热化学非平衡对气动热环境影响，可以为热防护系统设计提供有效保障。采用Park和Gupta热化学非平衡模型，数值计算研究5组元（N2，O2，N，O，NO），17组化学反应的热化学非平衡效应对高超声速飞行器气动热环境影响，并与完全气体和热化学平衡模型进行对比分析。热化学非平衡模型流场温度及激波距离均比完全气体模型小。激波后气体密度因离解、化学反应而增大，且气体密度越大，激波距离越小，热化学平衡模型激波距离最小。完全气体和热化学平衡模型热流载荷计算值均比实验值偏大。Park和Gupta热化学非平衡模型数值计算激波距离及气动力载荷差别小。Park模型热流载荷计算值偏大，Gupta模型与实验结果相符，它可对气动热环境可靠预测。
Abstract:Severe aerodynamic heating phenomenon occurs in hypersonic flight. Thermal protection system design can be effectively guided by considering the influence of hypersonic thermo-chemical non-equilibrium on aerodynamic thermal environment. Park and Gupta's thermo-chemical non-equilibrium models were used to numerically calculate the 5 species (N2, O2, N, O, NO) and 17 groups of chemical reactions, and the influence of their thermo-chemical non-equilibrium on hypersonic vehicles' aerodynamic thermal environments was compared with that obtained from perfect gas and thermo-chemical equilibrium models. In the thermo-chemical non-equilibrium model, flow field temperatures are lower and shock standoff distances are smaller than those of the perfect gas model. The larger the gas density after shock wave is, the smaller the shock standoff distance is. Therefore, the shock standoff distance of thermo-chemical equilibrium model is the smallest due to the larger gas density caused by molecular dissociation and chemical reaction effects. The numerical heat flux loads of perfect gas and thermo-chemical equilibrium models are larger than the experimental data. There are small differences between Park's and Gupta's thermo-chemical non-equilibrium model when they are used to numerically calculate the shock standoff distance and aerodynamic load. The calculated values of heat flux load of Park's model are larger, while those of Gupta's model are in good agreement with the experiments. Therefore, Gupta's model is more reliable to predict hypersonic vehicles' aerodynamic thermal environments.
-
图 1 完全气体与热化学非平衡气体模型马赫数
Figure 1. Mach numbers of perfect gas and thermo-chemical non-equilibrium gas models
图 3 完全气体模型静温与热化学非平衡气体模型平动-转动温度
Figure 3. Static temperatures of perfect gas model and translational-rotational temperatures of thermo-chemical non-equilibrium gas model
图 4 驻点线不同温度分布
Figure 4. Different temperature distributions along stagnation point line
图 5 完全气体模型与热化学非平衡气体模型壁面压强和热流密度
Figure 5. Wall pressures and heat fluxes of perfect gas and thermo-chemical non-equilibrium gas models
图 7 热化学平衡与非平衡气体模型流场平动-转动温度
Figure 7. Flow field translational-rotational temperatures of thermo-chemical equilibrium and non-equilibrium gas models
图 8 热化学平衡与非平衡气体模型壁面压强和热流密度
Figure 8. Wall pressures and heat fluxes of thermo-chemical equilibrium and non-equilibrium gas models
图 10 Park与Gupta模型壁面热流密度分布
Figure 10. Wall heat flux distributions of Park's and Gupta's model
图 11 壁面压强沿背风与迎风线分布
Figure 11. Distribution of wall pressures along leeward and windward lines
图 12 壁面热流密度沿背风与迎风线分布
Figure 12. Distribution of wall heat fluxes along leeward and windward lines
表 1 Gupta化学反应模型
Table 1. Gupta's chemical reaction model
化学反应式 Af/(cm3·(mol·s)-1) Bf Cf/K N2+M1⇌2N+M1 1.92×1017 -0.5 113 100 N2+N⇌2N+N 4.15×1022 -1.5 113 100 O2+M2⇌2O+M2 3.61×1018 -1 59 400 NO+M2⇌N+O+M2 3.97×1020 -1.5 75 600 N2+O⇌NO+N 6.75×1013 0 37 500 NO+O⇌O2+N 3.18×109 1 19 700 注：M1=N2, O2, O, NO; M2=N2, O2, N, O, NO。 
下载: 导出CSV
表 2 Park化学反应模型
Table 2. Park's chemical reaction model
化学反应式 Af/(cm3·(mol·s)-1) Bf Cf/K N2+M1⇌2N+M1 3.0×1022 -1.6 113 200 N2+M2⇌2N+M2 7.0×1021 -1.6 113 200 O2+M1⇌2O+M1 1.0×1022 -1.5 59 360 O2+M2⇌2O+M2 2.0×1021 -1.5 59 360 NO+M3⇌N+O+M3 1.1×1017 0 75 500 NO+M4⇌N+O+M4 5.0×1015 0 75 500 N2+O⇌NO+N 5.7×1012 0.42 42 938 NO+O⇌O2+N 8.4×1012 0 19 400 注：M1=N, O; M2=N2, O2, NO; M3=N, O, NO; M4=N2, O2。
下载: 导出CSV
-
[1] SCALABRIN L C, BOYD I D.Numerical simulation of weakly ionized hypersonic flow for reentry configurations:AIAA-2006-3773[R].Reston:AIAA, 2006. [2] PANDOLFI M, ARINA R, BOTTA N.Nonequilibrium hypersonic flows over corners[J].AIAA Journal, 1991, 29(2):235-241. doi: 10.2514/3.10569 [3] FREDERICKSON K, LEONOV S, NISHIHARA M, et al.Energy conversion in high enthalpy flows and non-equilibrium plasmas[J].Progress in Aerospace Sciences, 2015, 72:49-65. doi: 10.1016/j.paerosci.2014.09.004 [4] AIT-ALI-YAHIA D, HABASHI W G.Finite element adaptive method for hypersonic thermochemical nonequilibrium flows[J].AIAA Journal, 1997, 35(8):1294-1302. doi: 10.2514/2.260 [5] YUMUSAK M, EYI S.Aerothermodynamic shape optimization of hypersonic blunt bodies:AIAA-2013-2693[R].Reston:AIAA, 2013. [6] BHUTTA B A, LEWIS C H.Three-dimensional hypersonic nonequilibrium flows at large angles of attack[J].Journal of Spacecraft and Rockets, 1989, 26(3):158-166. doi: 10.2514/3.26048 [7] ZOBY E V, LEE K P, GUPTA R N, et al.Viscous shock-layer solutions with nonequilibrium chemistry for hypersonic flows past slender bodies[J].Journal of Spacecraft and Rockets, 1989, 26(4):221-228. doi: 10.2514/3.26058 [8] PEZZELLA G, VOTTA R.Finite rate of chemistry effects on the high altitude aerodynamics of an Apollo-shaped reentry capsule:AIAA-2009-7306[R].Reston:AIAA, 2009. [9] 柳军, 刘伟, 曾明, 等.高超声速三维化学非平衡流场的数值模拟[J].力学学报, 2003, 35(6):730-734.LIU J, LIU W, ZENG M, et al.Numerical simulation of 3D hypersonic thermochemical nonequilibrium flow[J].Acta Mechanica Sinica, 2003, 35(6):730-734(in Chinese). [10] 董维中, 丁明松, 高铁锁, 等.热化学非平衡模型和表面温度对气动热计算影响分析[J].空气动力学学报, 2013, 31(6):692-698. http://www.cnki.com.cn/Article/CJFDTOTAL-KQDX201306002.htmDONG W Z, DING M S, GAO T S, et al.The influence of thermo-chemical non-equilibrium model and surface temperature on heat transfer rate[J].Acta Aerodynamica Sinica, 2013, 31(6):692-698(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-KQDX201306002.htm [11] 李康, 胡宗民, 姜宗林.热化学非平衡流动中粘性干扰和化学反应对HB2气动力的影响[J].中国科学:物理学, 力学和天文学, 2015, 45(4):044702. http://www.cnki.com.cn/Article/CJFDTOTAL-JGXK201504008.htmLI K, HU Z M, JIANG Z L.Effect of viscosity and chemical reactions on aerodynamic force in chemical nonequilibrium flow[J].Scientia Sinica:Physica, Mechanica & Astronomica, 2015, 45(4):044702(in Chinese). http://www.cnki.com.cn/Article/CJFDTOTAL-JGXK201504008.htm [12] 周禹, 阎超.高超声速热化学非平衡空间格式的扩展与改进[J].北京航空航天大学学报, 2010, 36(2):193-197.ZHOU Y, YAN C.Extension and improvement for schemes in hypersonic thermal and chemical non-equilibrium flows[J]. Journal of Beijing University of Aeronautics and Astronautics, 2010, 36(2):193-197(in Chinese). [13] 周印佳, 孟松鹤, 解维华, 等.高超声速飞行器热环境与结构传热的多场耦合数值研究[J].航空学报, 2016, 37(9):2739-2748.ZHOU Y J, MENG S H, XIE W H, et al.Multi-field coupling numerical analysis of aerothermal environment and structural heat transfer of hypersonic vehicles[J].Acta Aeronautica et Astronautica Sinica, 2016, 37(9):2739-2748(in Chinese). [14] FORD D I, JOHNSON R E.Dependence of rate rate constants on vibrational temperatures:An arrhenius description:AIAA-1988-0461[R].Reston:AIAA, 1988. [15] GUPTA R N, YOS J M, THOMPSON R A, et al.A review of reaction rates and thermodynamic and transport properties for an 11-species air model for chemical and thermal nonequilibrium calculations to 30000K:NASA RP-1232[R].Washington, D.C.:NASA, 1990. [16] PARK C.On convergence of computation of chemical reacting flows:AIAA-1985-0247[R].Reston:AIAA, 1985. [17] PARK C.Problems of rate chemistry in the flight regimes of aeroassisted orbital transfer vehicles:AIAA-1984-1730[R].Reston:AIAA, 1984. [18] CANDLER G V, MACCORMACK R W.The computation of hypersonic ionized flows in chemical and thermal nonequilibrium:AIAA-1988-0511[R].Reston:AIAA, 1988. [19] WILKE C R.A viscosity equation for gas mixtures[J].Journal of Chemical Physics, 1950, 18(4):517-519. doi: 10.1063/1.1747673 [20] YOON B, RASMUSSEN M L.Diffusion effects in hypersonic flows with a ternary mixture[J].KSME International Journal, 1999, 13(5):432-442. doi: 10.1007/BF02939331 [21] MACLEAN M, MARINEAU E, PARKER R, et al.Effect of surface catalysis on measured heat transfer in expansion tunnel facility[J].Journal of Spacecraft and Rockets, 2013, 50(2):470-474. doi: 10.2514/1.A32327 [22] HORNUNG H G, WEN C Y.Nonequilibrium dissociationg flow over spheres:AIAA-1995-0091[R].Reston:AIAA, 1995. [23] STEWART D A, CHEN Y K.Hypersonic convective heat transfer over 140-deg blunt cones in different gases[J].Journal of Spacecraft and Rockets, 1994, 31(5):735-743. doi: 10.2514/3.26506 -
下载:
点击查看大图
计量
- 文章访问数: 822
- HTML全文浏览量: 85
- PDF下载量: 464
- 被引次数: 0
