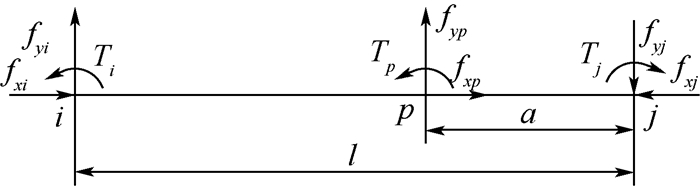
Stiffness modeling of micro-positioning platform considering unit interface relevance characteristics
-
摘要:
设计了一种含折叠梁的并联微定位平台，具有大行程、低阻力的特点。采用传递矩阵法求解其刚度，建立各柔性子单元的传递矩阵，利用相邻单元公共结点实现传递性，通过力平衡方程、变形协调方程求解其末端位移与输入力之间的刚度矩阵，并提出了考虑全柔性的弹性折叠梁及弹性移动副刚度的求解方法。将传递矩阵法求解结果与有限元分析结果对比，误差在20.5%以内，在此基础上，考虑到模块化刚度分析方法将各子单元视为独立体，忽略各子单元之间的界面关联特性，提出了一种根据各子单元界面关联特性进行修正的方法，结果表明，该方法使其误差降低到10%以内，更好地满足了实际工程需求。
Abstract:A parallel micro-positioning platform with folded beams was designed, which has characteristics of large stroke and low resistance. Stiffness was solved by transfer matrix method. First, transfer matrix of flexible subunit was established. The transitivity characteristics became available by taking advantage of the common node belonging to adjacent elements. Finally, the stiffness matrix between input force and output displacement of flexible mechanism was solved according to the force balance equation and compatibility equation of deformation. A method to solve the stiffness of compliant folded beam and compliant prismatic pair considering full flexibility was put forward. The result of deformation error is less than 20.5% compared with finite element analysis. On this basis, this method ignored the correlation between the subunits because the modular stiffness analysis method regarded each subunit as independent. A modified method considering each subunit dependency was put forward, which reduced the error to less than 10% and made the results better meet the actual engineering needs.
-
Key words:
- flexible mechanism /
- stiffness /
- transfer matrix /
- interface relevance characteristics /
- revision method
-
图 10 大行程并联微定位平台参数模型
Figure 10. Large stroke parallel micro-positioning platform parameter model
图 11 大行程并联微定位平台有限元模型
Figure 11. Finite element model of large stroke parallel micro-positioning platform
表 1 传递矩阵法与有限元分析值对比
Table 1. Comparison between transfer matrix method and finite element analysis
fwy/N 0 50 100 150 200 ux有限元法/μm -2.030 63 1.556 45 5.143 53 8.730 61 12.317 70 ux修正前/μm -2.094 30 1.308 69 4.711 68 8.114 66 9.816 16 ux修正后/μm -2.013 07 1.410 88 4.834 82 8.258 77 11.682 70 ux修正前误差/% 3.135 15.918 8.396 7.055 20.309 ux修正后误差/% 0.865 9.353 6.002 5.404 5.155 uy有限元法/μm 7.184 39 6.170 25 5.156 11 4.141 96 3.127 82 uy修正前/μm 6.805 97 5.758 83 4.711 68 4.188 10 3.140 65 uy修正后/μm 6.847 90 5.841 36 4.834 82 3.828 29 2.821 75 uy修正前误差/% 5.267 6.668 8.619 1.114 0.410 uy修正后误差/% 4.684 5.330 6.231 7.573 9.785 
下载: 导出CSV
-
[1] 于靖军, 郝广波, 陈贵敏, 等.柔性机构及其应用研究进[J].机械工程学报, 2015, 51(13):53-68. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jxxb201513006&dbname=CJFD&dbcode=CJFQYU J J, HAO G B, CHEN G M, et al.State-of-art of compliant mechanisms and their applications[J].Chinese Journal of Mechanical Engineering, 2015, 51(13):53-68(in Chinese). http://kns.cnki.net/KCMS/detail/detail.aspx?filename=jxxb201513006&dbname=CJFD&dbcode=CJFQ [2] 杨启志, 郭宗和, 马履中, 等.全柔性机构在并联微动机器人中的应用[J].机械设计与研究, 2005, 21(5):45-48. https://www.wenkuxiazai.com/doc/8f3979dbad51f01dc281f167-3.htmlYANG Q Z, GUO Z H, MA L Z, et al.Application of the fullly compliant mechanism in the parallel micro-motion robots[J]. Machine Design and Research, 2005, 21(5):45-48(in Chinese). https://www.wenkuxiazai.com/doc/8f3979dbad51f01dc281f167-3.html [3] HAO G B, KONG X W.A novel large-range XY compliant parallel manipulator with enhanced out-of-plane stiffness[J].Journal of Mechanical Design, 2012, 134(6):061009. doi: 10.1115/1.4006653 [4] 艾青林, 黄伟锋, 张洪涛.并联机器人刚度与静力学研究现状与进展[J].力学进展, 2012, 42(5):583-592. doi: 10.6052/1000-0992-11-073AI Q L, HUANG W F, ZHANG H T.Review of stiffness and statics analysis of parallel robot[J].Advances in Mechanics, 2012, 42(5):583-592(in Chinese). doi: 10.6052/1000-0992-11-073 [5] STANFORD B, BERAN P.Conceptual design of compliant mechanisms for flapping wing with topology optimization[J].AIAA Journal, 2011, 49(4):855-867. doi: 10.2514/1.J050940 [6] WISSA A, TUMMALA Y, HUBBARD J E, et al.Passively morphing ornithopter wings using a novel compliant spine:Design and testing[J].Smart Materials and Structures, 2012, 21(9):094028. doi: 10.1088/0964-1726/21/9/094028 [7] 于靖军, 毕树生, 宗光华.空间全柔性机构位置分析的刚度矩阵法[J].北京航空航天大学学报, 2002, 28(3):323-326. http://bhxb.buaa.edu.cn/CN/abstract/abstract10852.shtmlYU J J, BI S S, ZONG G H.Stiffness matrix method for displacement analysis of fully spatial compliant mechanisms[J].Journal of Beijing University of Aeronautics and Astronautics, 2002, 28(3):323-326(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract10852.shtml [8] LIU X J, WANG J, GAO F, et al. On the design of 6-DOF parallel micro-motion manipulators[C]//Proceedings of the 2001 IEEE/RSJ International Conference on Intelligent Robots and Systems. Piscataway: IEEE Press, 2001: 343-348. [9] 孙立宁, 董为, 杜志江.基于大行程柔性铰链的并联机器人刚度分析[J].机械工程学报, 2005, 41(8):90-95. doi: 10.3321/j.issn:0577-6686.2005.08.015SUN L N, DONG W, DU Z J.Stiffness analysis on a wide-range flexure hinge-based parallel manipulator[J].Chinese Journal of Mechanical Engineering, 2005, 41(8):90-95(in Chinese). doi: 10.3321/j.issn:0577-6686.2005.08.015 [10] 李育文, 张华, 杨建新, 等.6-UPS并联机床静刚度的有限元分析和实验研究[J].中国机械工程, 2004, 15(2):112-115. http://www.wenkuxiazai.com/doc/e6638414eff9aef8941e0663.htmlLI Y W, ZHANG H, YANG J X, et al.Finite element analysis and experimental study for the stiffness of a 6-UPS parallel kinematics machine[J].China Mechanical Engineering, 2004, 15(2):112-115(in Chinese). http://www.wenkuxiazai.com/doc/e6638414eff9aef8941e0663.html [11] YU Y Q, FENG Z L, XU Q P.A pseudo-rigid-body 2R model of flexural beam in compliant mechanisms[J].Mechanism and Machine Theory, 2012, 55(9):19-33. https://www.sciencedirect.com/science/article/pii/S0094114X12000948 [12] 邱丽芳, 霍明磊, 李威.六杆柔顺机构的伪刚体模型[J].北京科技大学学报, 2013, 35(5):682-686. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=bjkd201305020&dbname=CJFD&dbcode=CJFQQIU L F, HUO M L, LI W.Pseudo-rigid-body model of a six-bar full-compliant mechanism[J].Journal of University of Science and Technology Beijing, 2013, 35(5):682-686(in Chinese). http://kns.cnki.net/KCMS/detail/detail.aspx?filename=bjkd201305020&dbname=CJFD&dbcode=CJFQ [13] 李青宁.变截面杆元传递矩阵法[J].西安建筑科技大学学报, 2001, 33(1):18-23. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=xajz200101004&dbname=CJFD&dbcode=CJFQLI Q N.The transfer matrix method of bar elements with variable cross-section[J].Journal of Xi'an University of Architecture & Technology, 2001, 33(1):18-23(in Chinese). http://kns.cnki.net/KCMS/detail/detail.aspx?filename=xajz200101004&dbname=CJFD&dbcode=CJFQ [14] 郑洋洋, 宫金良, 张彦斐.基于传递矩阵法的柔性杠杆放大机构刚度分析[J].北京航空航天大学学报, 2017, 43(4):849-856. http://bhxb.buaa.edu.cn/CN/abstract/abstract13950.shtmlZHENG Y Y, GONG J L, ZHANG Y F.Rigidity analysis of a flexible lever magnifying mechanism based on transfer matrix method[J].Journal of Beijing University of Aeronautics and Astronautics, 2017, 43(4):849-856(in Chinese). http://bhxb.buaa.edu.cn/CN/abstract/abstract13950.shtml [15] 杨春辉.平行板型柔性移动副的刚度计算及分析[J].现代制造工程, 2013(12):30-33. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=xxgy201312008&dbname=CJFD&dbcode=CJFQYANG C H.The stiffness design calculation and analysis of parallel plate flexible prismatic pair[J].Modern Manufacturing Engineering, 2013(12):30-33(in Chinese). http://kns.cnki.net/KCMS/detail/detail.aspx?filename=xxgy201312008&dbname=CJFD&dbcode=CJFQ -
下载:
点击查看大图
计量
- 文章访问数: 706
- HTML全文浏览量: 79
- PDF下载量: 322
- 被引次数: 0
