-
摘要:
螺栓连接是复合材料结构的薄弱环节。因此,螺栓连接部位的设计是先进复合材料结构设计的关键之一。将修正特征曲线方法和随机参数统计模型结合,建立了碳纤维增强复合材料(CFRP)螺栓连接失效载荷的概率分析模型,进而以关键影响参数为设计变量,以可靠度指标为约束,以连接结构质量为设计目标,发展了基于可靠性的CFRP螺栓连接优化设计方法。采用正交试验设计方法建立基于可靠性的螺栓连接优化设计计算方案,优化结果表明:关键影响参数
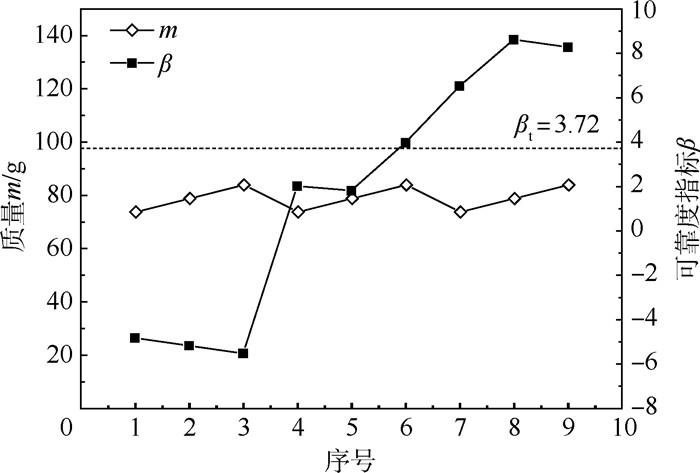
X C为2 450 MPa、t ply为0.174 mm、E 11为225 GPa时的设计方案为螺栓连接最佳设计方案,该方案使得外载荷为17.5 kN时,螺栓连接可靠度由0.998提高到0.999 999以上,同时使得连接结构的质量下降了6.44%。-
关键词:
- 复合材料螺栓连接 /
- 失效载荷 /
- 可靠度指标 /
- 基于可靠性的优化设计 /
- 特征曲线方法
Abstract:Bolted joints are the weak points of composite structures. Therefore, the design of bolted joints is one of crucial issues in the design of composite structure. In this paper, a probability analysis model for the failure load of a Carbon Fiber Reinforced Polymer (CFRP) composite bolted joint was built by combining the modified characteristic curve method and the statistical model of random parameters. The key influence parameters were regarded as the design variables, the reliability index was considered as the constraint, and the mass of the joint was the design goal. Accordingly, a reliability-based design optimization method was developed for the CFRP bolted joint. The orthogonal test design method was used to establish the reliability-based optimal design calculation scheme. The optimization results show that, when the key influence parameters
X C is 2 450 MPa,t ply is 0.174 mm, andE 11 is 225 GPa, the best design of the joint is obtained. According to this scheme, when the external load is 17.5 kN, the reliability of the joint is increased from 0.998 to above 0.999 999, and the mass of the joint is reduced by 6.44%. -
表 1 CFRP层压板几何尺寸和铺层角度的名义值
Table 1. Nominal geometrical dimensions and fiber orientation angles of CFRP laminate
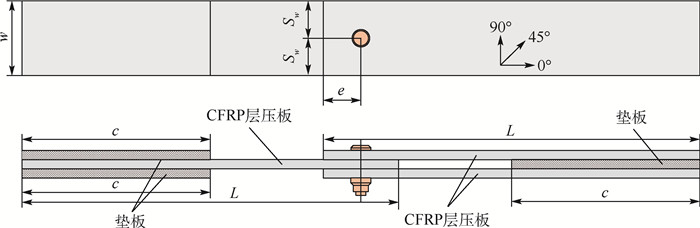
几何尺寸 w/mm Sw/mm e/mm D/mm tply/mm 数值 30 15 15 4.76 0.185 纤维铺设角度 θ1/(°) θ2/(°) θ3/(°) θ4/(°) 数值 0 90 45 -45 表 2 复合材料基本力学性能的平均值
Table 2. Means of basic mechanical properties of composites
弹性参数 E11/GPa E22/GPa G12/GPa υ12 数值 195 8.58 4.57 0.33 强度参数 XT/MPa XC/MPa YT/MPa YC/MPa S12/MPa 数值 3 071 1 747 88 271 143 表 3 螺栓连接随机参数的统计模型
Table 3. Statistical model of random parameters of bolted joint
类型 随机变量 期望值μ 标准差σ 分布类型 几何尺寸 w/mm 30 0.333 正态 Sw/mm 15 0.167 正态 e/mm 15 0.333 正态 D/mm 4.76 0.01 正态 tply/mm 设计变量 正态 纤维铺设角度 θ1/(°) 0 0.9 正态 θ2/(°) 90 0.9 正态 θ3/(°) 45 0.9 正态 θ4/(°) -45 0.9 正态 材料性能 E11/GPa 设计变量 正态 E22/GPa 8.58 0.086 正态 G12/GPa 4.57 0.098 正态 υ12 0.33 0.020 正态 XT/MPa 3 071 187.4 正态 XC/MPa 设计变量 正态 YT/MPa 88 7.7 正态 YC/MPa 271 22.4 正态 S12/MPa 143 3.9 正态 表 4 设计变量的取值
Table 4. Values of design variables
设计变量 初始值 水平1 水平2 水平3 μ σ COV μ1=μ-nσ μ2=μ μ3=μ+nσ XC 1 750 MPa 66.5 MPa 0.038 1 050 MPa 1 750 MPa 2 450 MPa tply 0.186 mm 0.004 mm 0.022 0.174 mm 0.186 mm 0.198 mm E11 195 GPa 3.8 GPa 0.02 165 GPa 195 GPa 225 GPa 表 5 基于正交试验设计的计算方案及目标函数值
Table 5. Calculation scheme based on orthogonal experimental design and objective function value
方案序号 XC/MPa tply/mm E11/GPa β m/g 1 1 050 0.174 165 -4.82 73.75 2 1 050 0.186 195 -5.17 78.83 3 1 050 0.198 225 -5.52 83.92 4 1 750 0.174 195 2.02 73.75 5 1 750 0.186 225 1.82 78.83 6 1 750 0.198 165 3.98 83.92 7 2 450 0.174 225 6.52 73.75 8 2 450 0.186 165 8.62 78.83 9 2 450 0.198 195 8.28 83.92 -
[1] MCCARTHY M A. BOJCAS: Bolted joints in composite aircraft structures[J]. Air & Space Europe, 2001, 3(3/4): 139-142. [2] MANGALGIRI P D. Design allowable considerations for use of laminated composites in aircraft structures[J]. Journal of the Indian Institute of Science, 2013, 93(4): 571-592. [3] ATAS A, SOUTIS C. Damage and failure analysis of bolted joints in composite laminates[M]//BEAUMONT P W R, SOUTIS C, HODZIC A. The structural integrity of carbon fiber composites. Berlin: Springer, 2017: 591-644. [4] 赵丽滨, 刘丰睿, 黄伟, 等. 复合材料螺栓连接失效分析研究进展[J]. 强度与环境, 2017, 44(3): 1-11.ZHAO L B, LIU F R, HUANG W, et al. Advances in failure analysis methods of bolted composite joints[J]. Structure & Environment Engineering, 2017, 44(3): 1-11(in Chinese). [5] SOARES C G. Reliability of components in composite materials[J]. Reliability Engineering and System Safety, 1997, 55(2): 171-177. doi: 10.1016/S0951-8320(96)00008-7 [6] CHIACHIO M, CHIACHIO J, RUS G. Reliability in composites-A selective review and survey of current development[J]. Composites Part B: Engineering, 2012, 43(3): 902-913. doi: 10.1016/j.compositesb.2011.10.007 [7] 王佩艳, 朱振涛, 王富生, 等. 复合材料螺栓连接性能的分散性和可靠性分析[J]. 力学季刊, 2008, 29(4): 573-577.WANG P Y, ZHU Z T, WANG F S, et al. Dispersibility and reliability analysis of composite laminates bolted joint[J]. Chinese Quarterly of Mechanics, 2008, 29(4): 573-577(in Chinese). [8] KHASHABA U A, SEBAEY T A, ALNEFAIE K A. Failure and reliability analysis of pinned-joint composite laminates: Effects of pin-hole clearance[J]. Journal of Composite Materials, 2013, 47(18): 2287-2298. doi: 10.1177/0021998312457196 [9] KHASHABA U A, SEBAEY T A, ALNEFAIE K A. Failure and reliability analysis of pinned-joints composite laminates: Effects of stacking sequences[J]. Composites Part B: Engineering, 2013, 45(1): 1694-1703. doi: 10.1016/j.compositesb.2012.09.066 [10] LI H S, LU Z Z, ZHANG Y. Probabilistic strength analysis of bolted joints in laminated composites using point estimate method[J]. Composite Structures, 2009, 88(2): 202-211. doi: 10.1016/j.compstruct.2008.03.040 [11] LI H S. Maximum entropy method for probabilistic failure load prediction of pin joints in composite laminates[J]. Composite Structures, 2013, 106: 626-634. doi: 10.1016/j.compstruct.2013.05.040 [12] LI H S, XIA S, LUO D M. A probabilistic analysis for pin joint failure load in composite laminates using subset simulation[J]. Composites Part B: Engineering, 2014, 56: 780-789. doi: 10.1016/j.compositesb.2013.09.025 [13] NAKAYAMA M, UDA N, ONO K. Probabilistic assessment of pin joint strength in CFRP laminates[J]. Composite Structures, 2011, 93(8): 2026-2030. doi: 10.1016/j.compstruct.2011.02.019 [14] NAKAYAMA M, UDA N, ONO K, et al. Design-oriented strength of mechanical joints in composite laminate structures and reliability-based design factor[J]. Composite Structures, 2015, 132: 1-11. doi: 10.1016/j.compstruct.2015.04.044 [15] 左健巍. 复合材料结构的重要性测度[C]//第四届中国航空科学技术大会, 2019: 264-277.ZUO J W. Importance measure on composite material structures[C]//Proceedings of the 4th China Aviation Science and Technology Conference, 2019: 264-277(in Chinese). [16] 高魁垠, 李海波, 吴建国, 等. 2D-C/SiC复合材料螺栓连接结构可靠性分析[J]. 强度与环境, 2020, 47(1): 33-40.GAO K Y, LI H B, WU J G, et al. Reliability analysis of 2D-C/SiC composite bolted joints[J]. Structure & Environment Engineering, 2020, 47(1): 33-40(in Chinese). [17] ZHAO L, SHAN M, LIU F, et al. A probabilistic model for strength analysis of composite double-lap single-bolt joints[J]. Composite Structures, 2017, 161: 419-427. doi: 10.1016/j.compstruct.2016.11.074 [18] LIU F, FANG Z, ZHAO L, et al. A failure-envelope-based method for the probabilistic failure prediction of composite multi-bolt double-lap joints[J]. Composites Part B: Engineering, 2019, 172: 593-602. doi: 10.1016/j.compositesb.2019.05.034 [19] YANG L, MA Z. Optimum design based on reliability for a composite structural system[J]. Computers & Structures, 1990, 36(5): 785-790. [20] MUROTSU Y, MIKI M, SHAO S. Reliability design of fiber reinforced composites[J]. Structural Safety, 1994, 15(1-2): 35-49. doi: 10.1016/0167-4730(94)90051-5 [21] CHANG F K, SCOTT R A, SPRINGER G S. Strength of mechanically fastened composite joints[J]. Journal of Composite Materials, 1982, 16(6): 470-494. doi: 10.1177/002199838201600603 [22] XIONG Y. An analytical method for failure prediction of multi-fastener composite joints[J]. International Journal of Solids and Structures, 1996, 33(29): 4395-4409. doi: 10.1016/0020-7683(95)00231-6 [23] ZHANG J M. Design and analysis of mechanically fastened composite joints and repairs[J]. Engineering Analysis with Boundary Elements, 2001, 25(6): 431-441. doi: 10.1016/S0955-7997(01)00049-2 [24] ZHANG J, LIU F, ZHAO L, et al. A novel characteristic curve for failure prediction of multi-bolt composite joints[J]. Composite Structures, 2014, 108: 129-136. doi: 10.1016/j.compstruct.2013.09.019 [25] CHOI S K, GRANDHI R V, CANFIELD R A. 结构可靠性设计[M]. 芮强, 王红岩, 译. 北京: 国防工业出版社, 2014.CHOI S K, GRANDHI R V, CANFIELD R A. Reliability-based structural design[M]. RUI Q, WANG H Y, translated. Beijing: National Defense Industry Press, 2014(in Chinese). [26] ZHANG J, LIU F, ZHAO L, et al. Investigation on characteristic length testing methods for failure prediction of composite multi-bolt joints[J]. Journal of Reinforced Plastics and Composites, 2015, 34(8): 636-648. doi: 10.1177/0731684415574871 [27] YAMADA S E, SUN C T. Analysis of laminate strength and its distribution[J]. Journal of Composite Materials, 1978, 12: 275-284. doi: 10.1177/002199837801200305 -







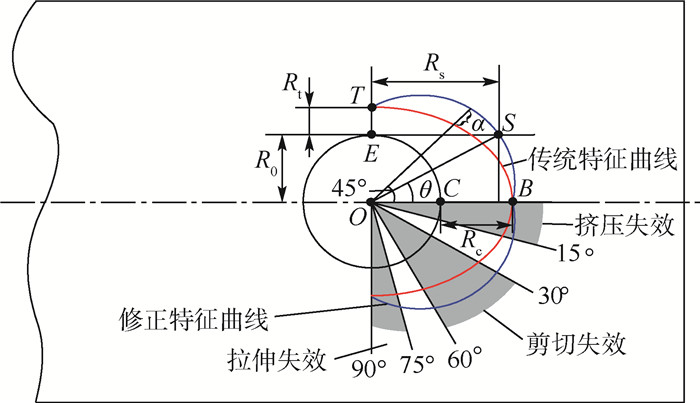
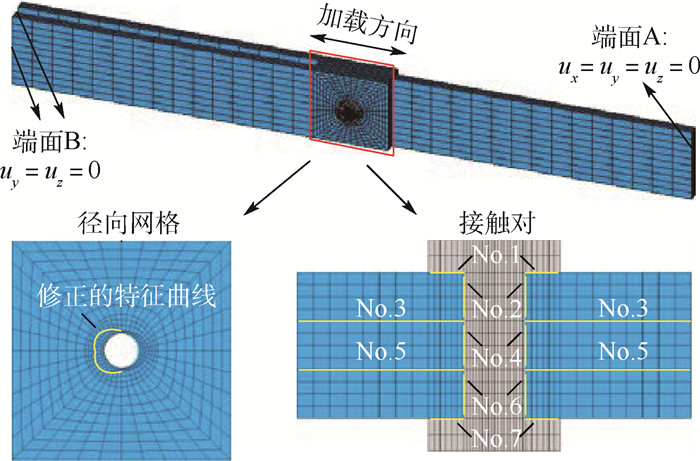
 下载:
下载: