In the ultrasonic detection for multi-layer bonding structures of metal and non-metal materials such as rubber, the signal to noise ratio of debonding defect is low, and the defect identification is difficult, due to the significant difference in acoustic impedance between media and large ultrasonic...

X-ray security image contraband classification is widely used to assist in maintaining aviation and transportation security. This paper suggests an end-to-end X-ray security inspection image classification method that takes sample imbalance into account in order to address the issues of different sc...

Arrival flight sequencing is an effective strategy to improve landing efficiency and reduce flight delays. On the basis of previous research, this paper aims to minimize the makespan of all flights landing, considers actual multi-waypoint constraints, and proposes a path and runway integrated model,...

The envelope size of the fairing will be recommended during the first stage of launch vehicle design in accordance with the satellite’s envelope size requirements, which also affects the launch vehicle’s basic configuration design. To predict the buffeting risk of this configuration, the aerodynamic...

With the rapid development of civil aviation, airport airspace has become more crowded. How airspace sector planning methods can be improved has become a key research question. The traditional method has the shortcomings of over-simplified indicators and relying on human experiences. This research o...

Regarding the problem of the angle-only orbit determination, we transformed into a method for solving the minimum value of the fitness function. The difference and relationship between the observation time step, the dynamic equation solution step and the iterative format step are analyzed. It is poi...

The diagnosis effect is unsatisfactory because it is challenging to extract high-quality fault characteristics from a single signal given the complicated working conditions of the metallurgical industry. Aiming at the problem of directly using current and vibration signals for fusion, which cannot r...

Advanced aero-engine combustor designs require precise control of turbulent flames, and existing simulation methods need to improve accuracy and efficiency. The probability density function transport equation (TPDF) turbulent combustion model possesses high accuracy and the algebraic second-order mo...

An improved active disturbance rejection control (ADRC) was proposed for quadrotor attitude control to address the extended state observer (ESO) based on the traditional fal function's problems with easy chattering and insufficient anti-interference ability when responding to complex disturbances. A...

To meet the light-weight and high-efficiency heat dissipation requirements of space optical remote sensors with large aperture, a space radiator based on high thermal conductivity graphite film is proposed for the first time. The basic physical properties, structural composition, mechanical properti...

The double charged ion in the beam of ion thruster not only restricts the working life of the grid components but also affects the actual thrust of the thruster, and its proportion in the total beam directly determines the conformity of the key performance such as thrust and life of the thruster. In...

With the development of advanced materials and microelectronic technology, the design and manufacture of flapping wing aircraft has become a research topic of great concern in recent years. The bird-like shape makes it suitable for conversion investigation and monitoring. The best shape and motion c...

In practical applications, continuous communication cannot be guaranteed due to external interference or limited communication ability. Therefore, this study investigates the tracking control problem of second-order multi-agent systems with intermittent communication. To improve the convergence perf...

Surface quality optimization is a common problem in surface reconstruction. In the design of high-end products such as aerospace and automobile, if the reconstructed surfaces are required to have high-order continuity, a lot of optimization work is often needed. In order to obtain smooth and high-qu...

In order to investigate the reliability of advanced complementary metal-oxide-semiconductor (CMOS) processes for space applications, a hardening strategy against single-event transient (SET) is investigated in P-channel fin field-effect transistor (pFinFET) devices at 14 nm. The effects of SETs are ...

Due to the connection between the front wing and the rear wing, the aerodynamic and structural characteristics of the joined wing aircraft are different from those of the conventional layout aircraft. The interconnected wings form a complex over-constrained system that has numerous layout parameters...

A new airfoil optimization method is proposed to address the inherent defects of the frozen eddy viscosity assumption widely used in airfoil optimization and the poor accuracy of aerodynamic calculation based on Spalart-Allmaras (S-A) adjoint turbulence model. This method couples the continuous adjo...

To address the challenge of rendezvousing an indeterminate number of homogeneous unmanned surface vehicles (USV) into desired formations, a distributed rendezvousing control method is introduced, leveraging multi-agent reinforcement learning (MARL). Recognizing the communication and perception const...

To improve the reliability analysis efficiency of the engine nozzle adjustment mechanism, an analysis method combining rejection sampling and active learning Kriging surrogate model is proposed. A virtual prototype simulation model of an engine nozzle adjustment mechanism was established in ADAMS, a...

SpaceWire (SpW) router is one of the key devices in the SpW network. It adopts a crossbar switching structure. Since the maximum length of the data packet of the SpW network is not fixed, the classic interative round robin matching with slip (iSlip) algorithm is not applicable. This paper studies th...

The active twist control rotor is investigated to evaluate the effectiveness in rotor vibration reduction. A numerical model for predicting the isolated rotor vibration loads in steady level flight is deployed and validated by modeling a UH-60A rotor. A parametric sweep of the amplitude and phase an...

The application of graph convolutional network (GCN) to hyperspectral image (HSI) classification is the hotspot and frontier of current research. Nevertheless, the over-smoothing, feature adaptive selection, and calculation complexity issues still exist for the graph convolution network approaches t...

A fast 3D path planning method based on a 2D connected graph is proposed in order to address the issue of the unmanned aerial vehicle 3D path planning problem's slow problem-solving speed in a complicated real environment. In order to create a multi-level equivalent 3D digital map based on digital e...

Lattice material is a new type of lightweight and multifunctional material, which has a variety of microstructures and high porosity. Excellent macroscopic properties can be obtained by designing its mesoscale features. To maximize the design potential of materials and structures, an optimization me...

To effectively reduce the design cost and cycle time of using computational fluid dynamics (CFD) methods, the reduced-order model (ROM) has gained wide attention in recent years. For complex compressible flows, using linear methods such as proper orthogonal decomposition (POD) for flow field dimensi...

Rotating disk atomization is an important method to prepare spherical metal powder. In the preparation of high-melting-point metal powders, by this method, thermal protection is required for both the turntable structure itself and the lower-end driving motor. The coupled heat transfer problem of cen...

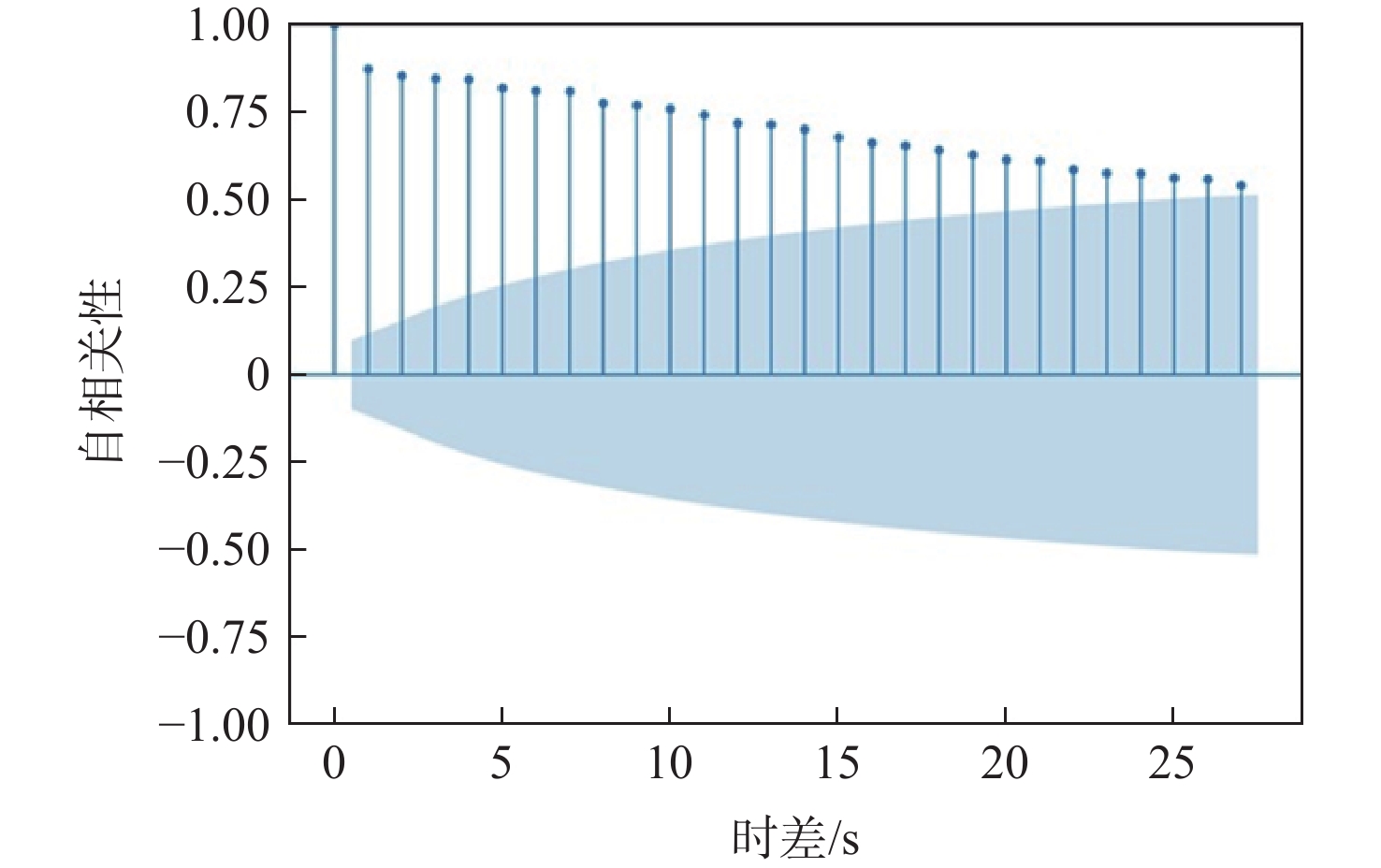
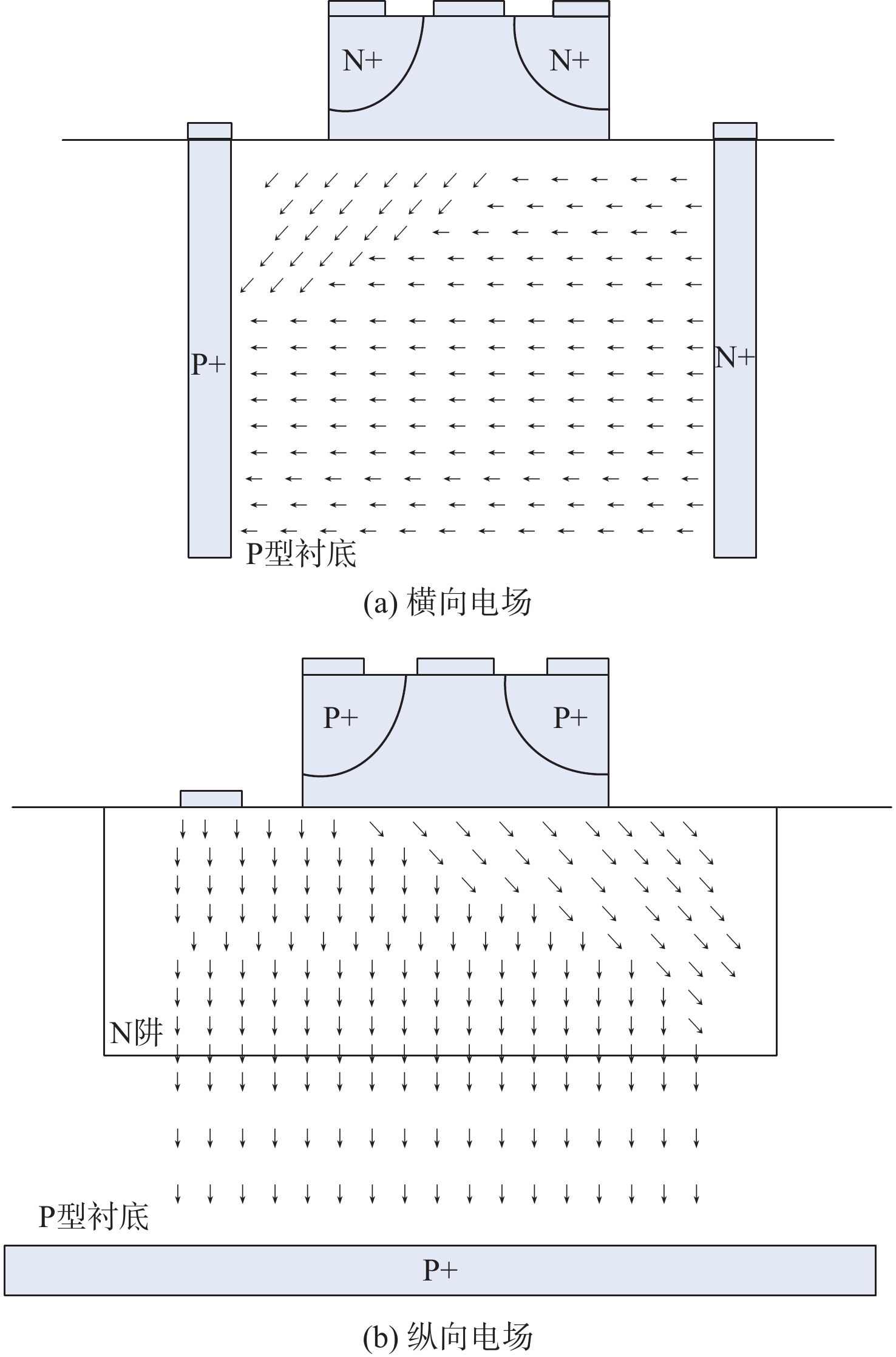
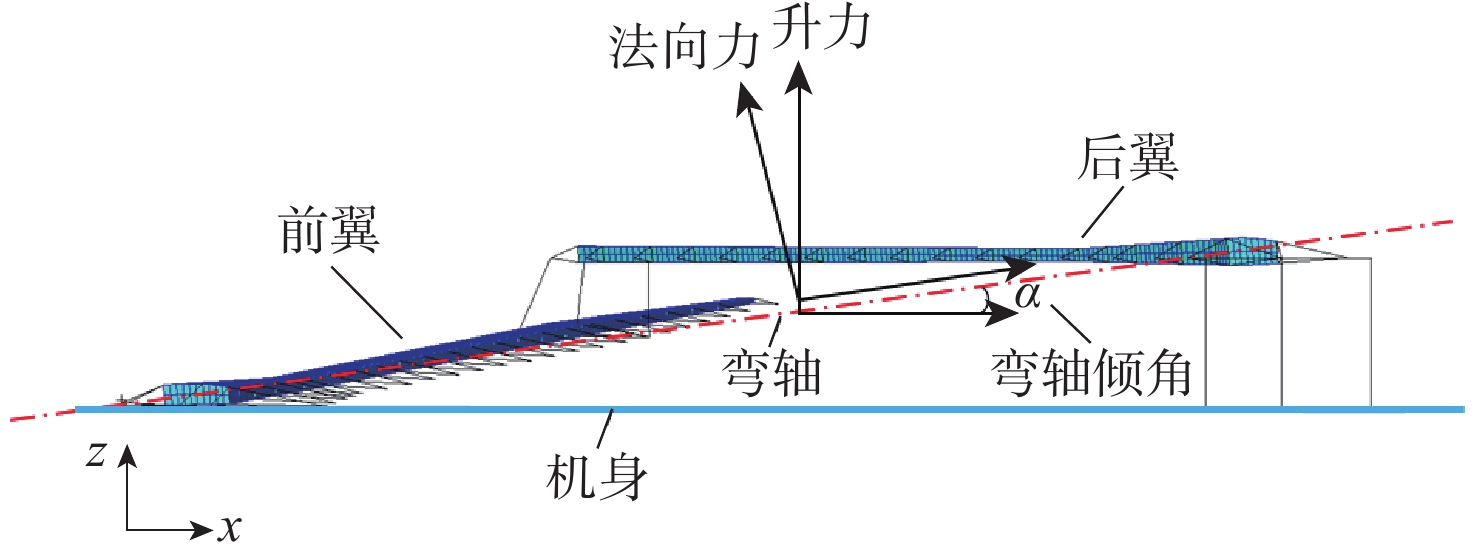
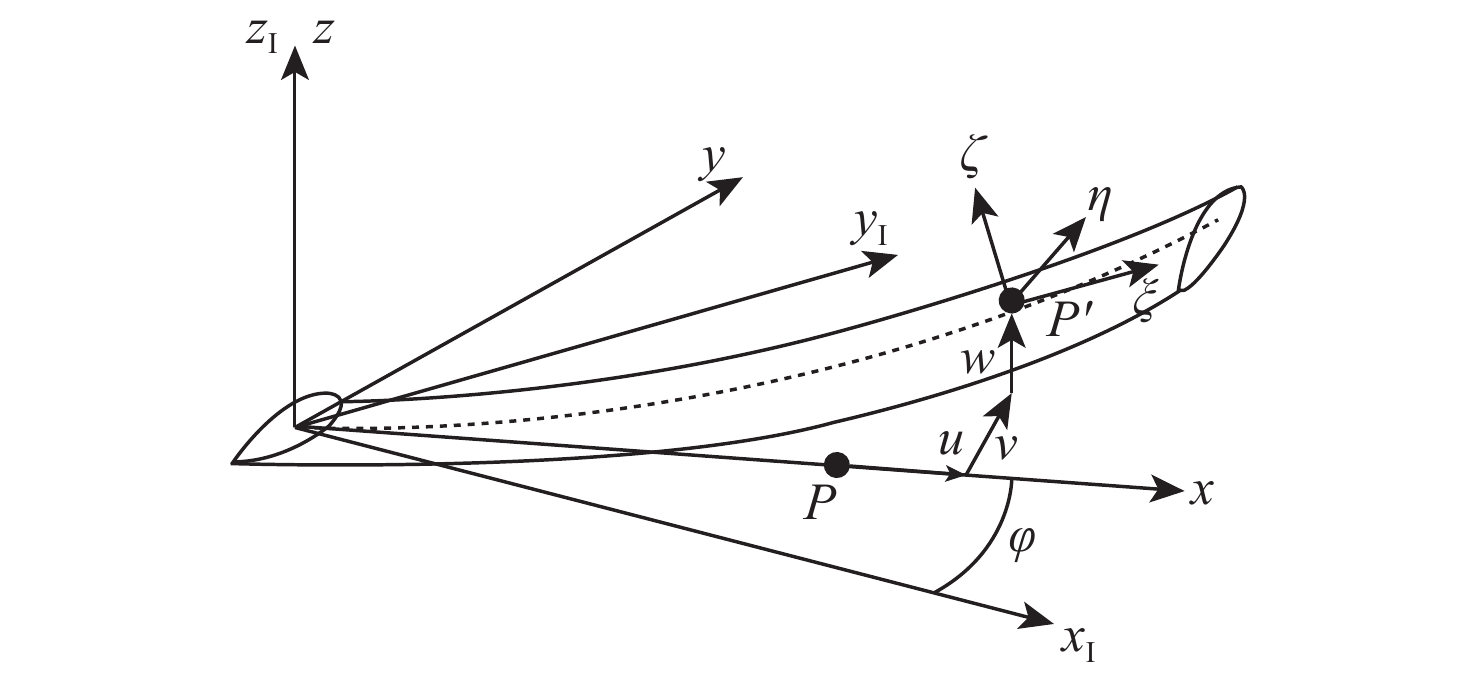
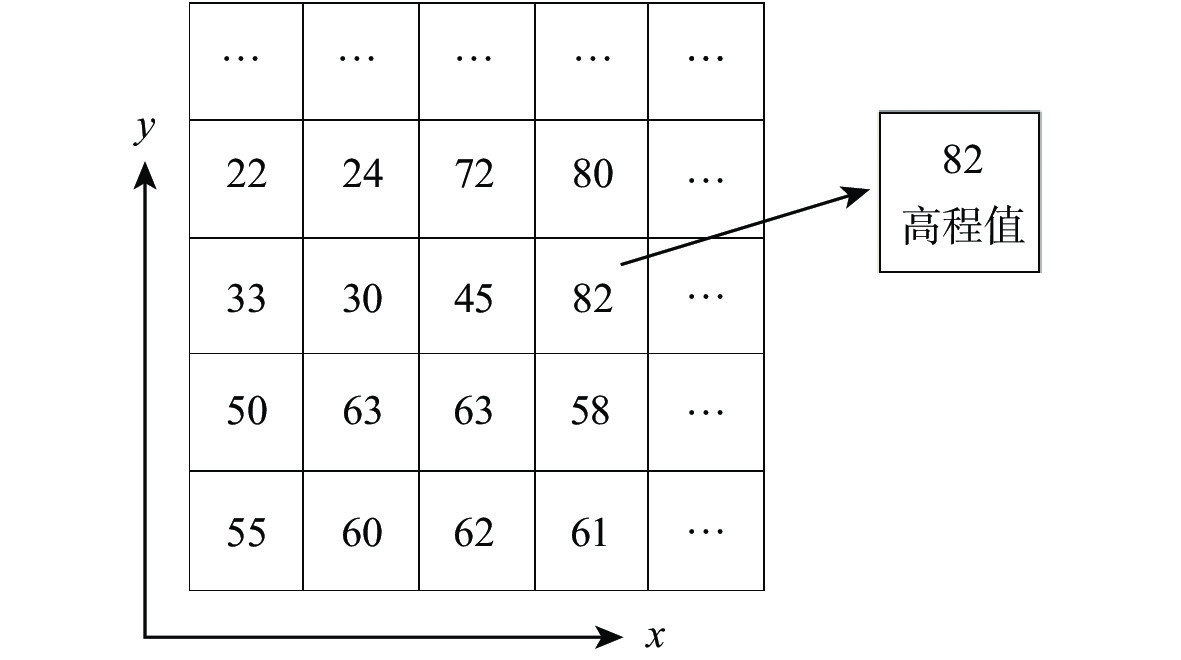
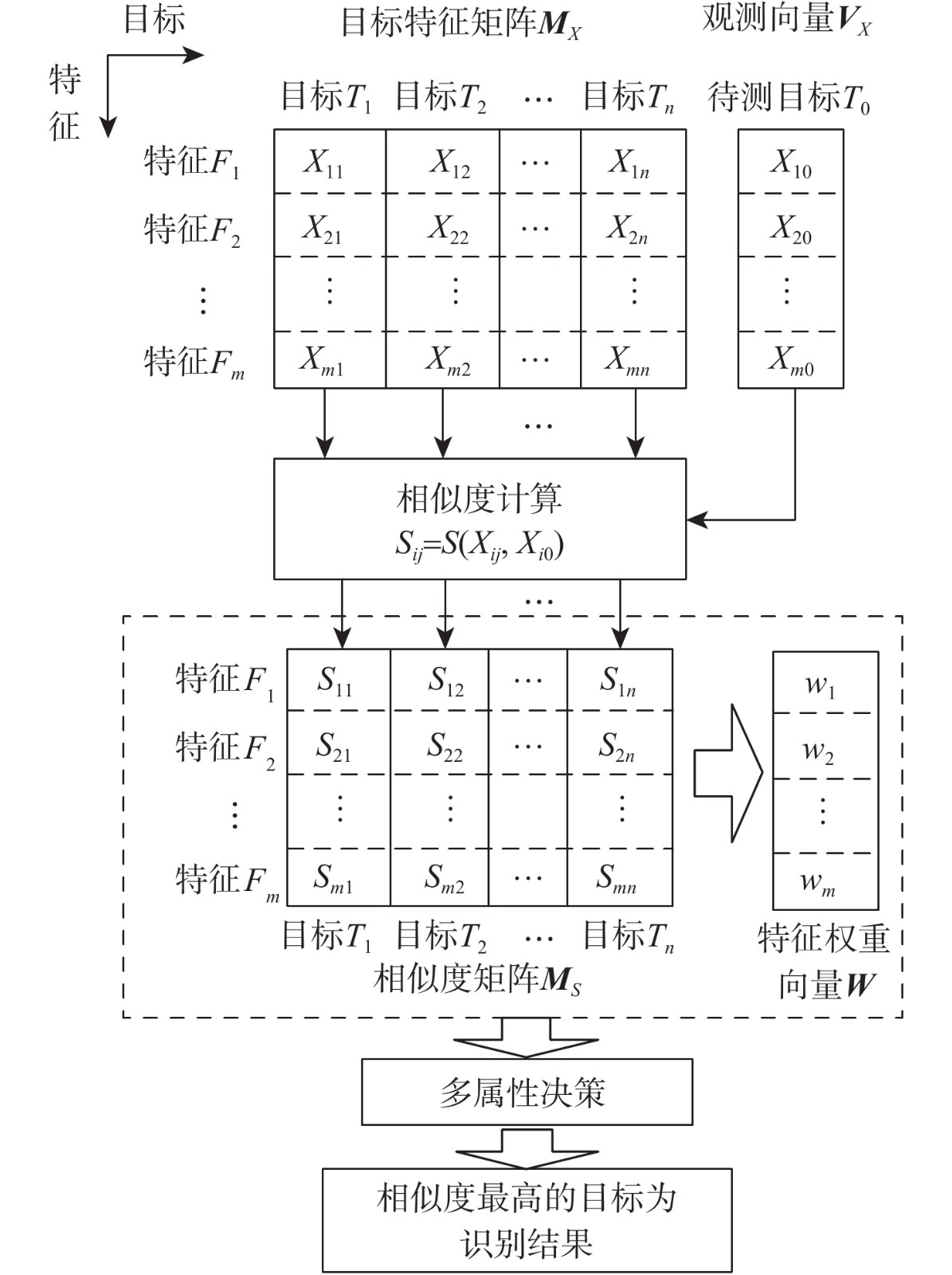
Accurate state of health (SOH) prediction for lithium-ion batteries is a key technology in battery applications. However, because of the varied failure modes, complicated electrochemical systems, and production variations, the degradation of lithium-ion batteries frequently exhibits high dispersion,...

High-speed UAV is prone to spin out of control, veer off the runway, and other major incidents because of the influence of numerous nonlinear elements such tire forces, aerodynamic forces, and rudder surface forces during the ground taxiing process. At present, the bifurcation is used to analyze the...

The development of unmanned systems and intelligent technology has presented a broad application prospect of UAV swarms, one of the typical applications of unmanned systems in both civilian and military fields. When the swarm size is large, however, the traditional networking communication method wi...

To address the uncertainty in quantitative evaluation of autonomous capability of ground-attack UAVs, an evaluation method with the cloud model is proposed based on combined weightings. Based on the cognitive control structure, the evaluation index system of autonomous capability is constructed from...

Aiming at the problems of occlusion and monotony of pedestrian discriminative feature hierarchy in person re-identification, this paper proposes a method combining random occlusion and multi-granularity feature fusion based on the IBN-Net50-a network. First, in order to enhance the robustness agains...

The temperature serves as a crucial indicator in the airworthiness compliance verification process of the fuel tank inerting system. Using MATLAB Simulink software, a mass and heat transfer model was established and validated for analyzing the behavior of gas phase space nodes and fuel nodes' temper...

After the BeiDou-3 navigation satellite system was finished, the performance of high-orbit autonomous navigation was improved, but it also occasionally resulted in the redundancy of visible satellites. In order to reduce the arithmetic operations to ensure the real-time performance, based on a multi...

In order to address the drawbacks of the traditional marginal Fisher analysis and related methods, a dimension reduction method for multivariate time series based on two-dimensional inter-class marginal Fisher analysis is proposed in this study. First, it conducts model improvement to cope with the ...

A relative entropy method combining fuzzy modeling and improved CRITIC was presented to recognize targets with fuzzy features. The observed values from multiple times were converted into fuzzy numbers through fuzzy modeling based on the statistical characteristics of multiple sets of the observed va...